8.2: Moving Money Involving Simple Interest
- Page ID
- 22111
Can you calculate the amount of money required to meet a future goal? Assume you will graduate college with your business administration diploma in a few months and have already registered at your local university to continue with your studies toward a bachelor of commerce degree. You estimate your total tuition, fees, and textbooks at $8,000. After investigating some short-term investments, you find the best simple rate of interest obtainable is 4.5%. How much money must you invest today for it to grow with interest to the needed tuition money?
In the previous section, you calculated the amount of interest earned or charged on an investment or loan. While this number is good to know, most of the time investors are interested solely in how much in total, including both principal and interest, is either owed or saved. Also, to calculate the interest amount in Formula 8.1, you must know the principal. When people plan for the future, they know the future amount of money that they need but do not know how much money they must invest today to arrive at that goal. This is the case in the opening example above, so you need a further technique for handling simple interest.
This section explores how to calculate the principal and interest together in a single calculation. It adds the flexibility of figuring out how much money there is at the beginning of the time period so long as you know the value at the end, or vice versa. In this way, you can solve almost any simple interest situation.
Maturity Value (or Future Value)
The maturity value of a transaction is the amount of money resulting at the end of a transaction, an amount that includes both the interest and the principal together. It is called a maturity value because in the financial world the termination of a financial transaction is known as the "maturing" of the transaction. The amount of principal with interest at some point in the future, but not necessarily the end of the transaction, is known as the future value.
The Formula
For any financial transaction involving simple interest, the following is true:
\[\text{Amount of money at the end }=\text{ Amount of money at the beginning }+\text{ Interest}\nonumber \]
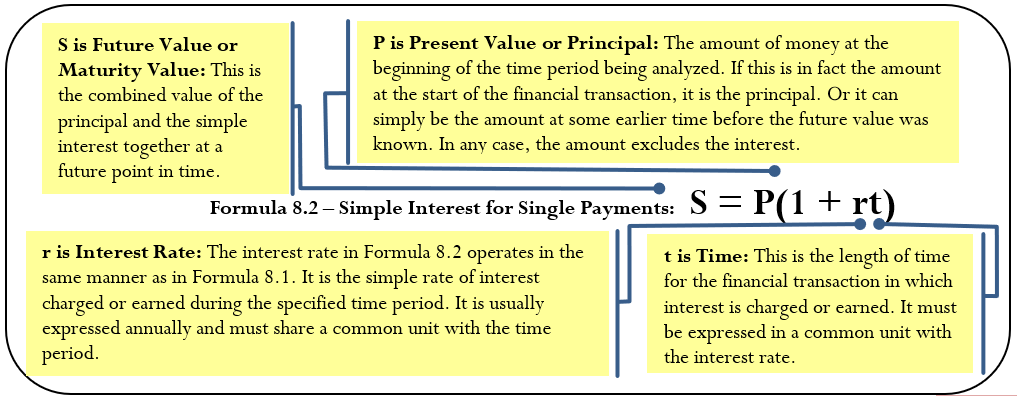
Applying algebra, you can summarize this expression by the following equation, where the future value or maturity value is commonly denoted by the symbol \(S\):
\[S=P+I\nonumber \]
Substituting in Formula 8.1, \(I = Prt\), yields the equation
\[S=P+Prt\nonumber \]
Simplify this equation by factoring \(P\) on the right-hand side to arrive at Formula 8.2.
Depending on the financial scenario, what information you know, and what variable you need to calculate, you may need a second formula offering an alternative method of calculating the simple interest dollar amount.
How It Works
Follow these steps when working with single payments involving simple interest:
Step 1: Formula 8.2 has four variables, any three of which you must identify to work with a single payment involving simple interest.
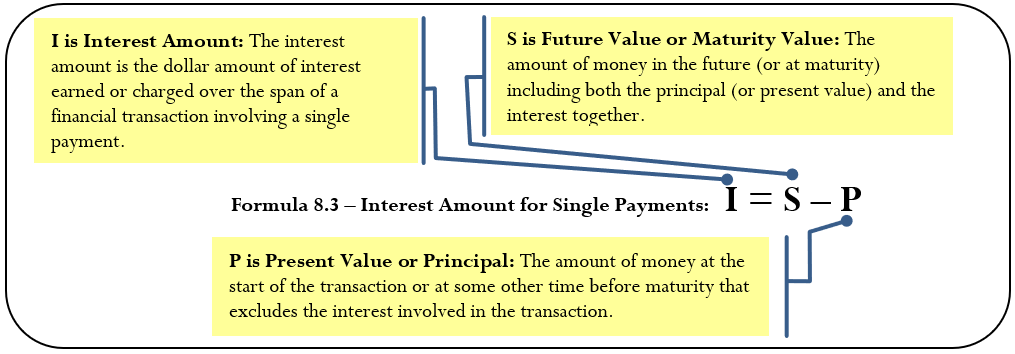
- If either the \(P\) or \(S\) is missing but the \(I\) is known, apply Formula 8.3 to determine the unknown value.
- If only the interest amount \(I\) is known and neither \(P\) nor \(S\) is identified, recall that the interest amount is a portion and that the interest rate is a product of rate and time (e.g., 6% annually for nine months is an earned interest rate of 4.5%). Apply Formula 2.2 on rate, portion, and base to solve for base, which is the present value or \(P\).
- If necessary, draw a timeline to illustrate how the money is being moved through time.
Step 2: Ensure that the simple interest rate and the time period are expressed with a common unit. If not, you need to convert one of the two variables to achieve the commonality.
Step 3: Apply Formula 8.2 and solve for the unknown variable, manipulating the formula as required.
Step 4: If you need to calculate the amount of interest, apply Formula 8.3.
Assume that today you have $10,000 that you are going to invest at 7% simple interest for 11 months. How much money will you have in total at the end of the 11 months? How much interest do you earn?
Step 1: The principal is \(P = \$10,000\), the simple interest rate is 7% per year, or \(r= 0.07\), and the time is \(t = 11\) months.
Step 2: The time is in months, but to match the rate it needs to be expressed annually as \(t=\dfrac{11}{12}\).
Step 3: Applying Formula 8.2 to calculate the future value including interest,
\(S=\$ 10,000 \times(1+0.07 \times)=\dfrac{11}{12}\$ 10,641.67\). This is the total amount after 11 months.
Step 4: Applying Formula 8.3 to calculate the interest earned, \(I=\$ 10,641.67-\$ 10,000.00=\$ 641.67\). You could also apply Formula 8.1 to calculate the interest amount; however, Formula 8.3 is a lot easier to use. The $10,000 earns $641.67 in simple interest over the next 11 months, resulting in $10,641.67 altogether.
Things To Watch Out For
As with Formula 8.1, the most common mistake in the application of Formula 8.2 is failing to ensure that the rate and time are expressed in the same units. Before you proceed, always check these two variables!
Paths To Success
When solving Formula 8.2 for either rate or time, it is generally easier to use Formula 8.3 and Formula 8.1 instead. Follow these steps to solve for rate or time:
- If you have been provided with both the present value and future value, apply Formula 8.3 to calculate the amount of interest (\(I\)).
- Apply and rearrange Formula 8.1 to solve for either rate or time as required.
In each of the following situations, determine which statement is correct.
- If you have a future value of $5,000 and interest is involved, then your present value is
- More than $5,000
- The same
- Less than $5,000
- If you had a principal of $10,000 earning 10% simple interest for any period less than a year, you earn
- More than $1,000 interest
- Exactly $1,000 interest
- Less than $1,000 interest
- If you took out a loan for $2,500 at a 5% simple interest rate for six months and you made no payments during that time frame, you owe
- More than $2,500
- Exactly $2,500
- Less than $2,500
- Answer
-
- Less than $5,000. When being charged or earning interest, the present value is always less than the future value.
- Less than $1,000 interest. The $10,000 earns exactly $1,000 interest per year, so any time frame less than a year produces a smaller interest amount.
- More than $2,500. When interest is being charged, the future value is always larger than the present value
You just inherited $35,000 from your uncle's estate and plan to purchase a house four months from today. If you use your inheritance as your down payment on the house, how much will you be able to put down if your money earns 4¼% simple interest? How much interest will you have earned?
Solution
Calculate the amount of money four months from now including both the principal and interest earned. This is the maturity value (\(S\)). Also calculate the interest earned (\(I\)).
What You Already Know
Step 1:
The principal, interest rate, and time frame are known: \(P = \$35,000, r = 4¼\%\) per year \(t = 4\) months
How You Will Get There
Step 2:
While the rate is annual, the time is in months. Convert the time to an annual number.

Step 3:
Apply Formula 8.2.
Step 4:
Apply Formula 8.3 to calculate the interest amount.
Perform
Step 2:
Four months out of 12 months in a year is \(t=\dfrac{4}{12}\)
Step 3:
\[S=\$ 35,000 \times\left(1+4\dfrac{1}{4} \% \times \dfrac{4}{12}\right)=\$ 35,000 \times(1+0.0425 \times 0 . \overline{3})=\$ 35,495.83 \nonumber \]
Step 4:
\[I = \$35,495.83 − \$35,000.00 = \$495.83 \nonumber \]
Four months from now you will have $35,495.83 as a down payment toward your house, which includes $35,000 in principal and $495.83 of interest.
Recall the section opener, where you needed $8,000 for tuition in the fall and the best simple interest rate you could find was 4.5%. Assume you have eight months before you need to pay your tuition. How much money do you need to invest today?
Solution
Calculate the principal amount of money today (\(P\)) that you must invest such that it will earn interest and end up at the $8,000 required for the tuition.
What You Already Know
Step 1:
The future value, interest rate, and time period are known: \(S = \$8,000, r = 4.5\%\) per year \(t = 8\) months
How You Will Get There
Step 2:
While the rate is annual, the time is in months. Convert the time to an annual number.

Step 3:
Apply Formula 8.2, rearranging for \(P\).
Perform
Step 2:
Eight months out of 12 months in a year is \(t=\dfrac{8}{12}\)
Step 3:
\[\begin{aligned} &\$ 8,000=P\left(1+4.5 \% \times \dfrac{8}{12}\right)\\ &P=\dfrac{\$ 8,000}{(1+0.045 \times 0.\overline {6})}=\$ 7,766.99 \end{aligned} \nonumber \]
If you place $7,766.99 into the investment, it will grow to $8,000 in the eight months.
You are sitting in an office at your local financial institution on August 4. The bank officer says to you, "We will make you a great deal. If we advance that line of credit and you borrow $20,000 today, when you want to repay that balance on September 1 you will only have to pay us $20,168.77, which is not much more!" Before answering, you decide to evaluate the statement. Calculate the simple interest rate that the bank officer used in her calculations.
Solution
Determine the rate of interest that you would be charged on your line of credit, or \(r\).
What You Already Know
Step 1:
The principal, maturity amount, and time frame are known: \(P = $20,000.00, S = $20,168.77, t =\) August 4 to September 1
How You Will Get There
Step 1 (continued):
Calculate the number of days in the transaction.
Step 2:
Since interest rates are usually expressed annually, convert the time from days to an annual number.
Step 3:
Use the combination of Formula 8.3 and Formula 8.1. First calculate the interest amount using Formula 8.3.
Step 3 (continued):
Then apply Formula 8.1, rearranging for \(r\).
Perform
Step 1 (continued):
| Start Date | End Date | Days between Dates |
|---|---|---|
| August 4 | August 31 | 31 − 4 = 27 |
| August 31 | September 1 | 1 − 0 = 1 |
| TOTAL | 27 + 1 = 28 days | |
Step 2:
28 days out of 365 days in a year is \(t=\dfrac{28}{365}\)
Step 3:
\[I = \$20,168.77 − \$20,000.00 = \$168.77 \nonumber \]
Step 3 (continued):
\[r=\dfrac{\$ 168.77}{\$ 20,000.00 \times \dfrac{28}{365}}=0.110002 \text { or } 11.0002 \% \nonumber \]
Calculator Instructions
Assume the year 2011 and use the DATE function:
| DT1 | DT2 | DBD | Mode |
|---|---|---|---|
| 08.0411 | 09.0111 | Answer: 28 | ACT |
The interest rate on the offered line of credit is 11.0002% (note that it is probably exactly 11%; the extra 0.0002% is most likely due to the rounded amount of interest used in the calculation).
Equivalent Payments
Life happens. Sometimes the best laid financial plans go unfulfilled. Perhaps you have lost your job. Maybe a reckless driver totaled your vehicle, which you now have to replace at an expense you must struggle to fit into your budget. No matter the reason, you find yourself unable to make your debt payment as promised.
On the positive side, maybe you just received a large inheritance unexpectedly. What if you bought a scratch ticket and just won $25,000? Now that you have the money, you might want to pay off that debt early. Can it be done?
Whether paying late or paying early, any amount paid must be equivalent to the original financial obligation. As you have learned, when you move money into the future it accumulates simple interest. When you move money into the past, simple interest must be removed from the money. This principle applies both to early and late payments:
- Late Payments. If a debt is paid late, then a financial penalty that is fair to both parties involved should be imposed. That penalty should reflect a current rate of interest and be added to the original payment. Assume you owe $100 to your friend and that a fair current rate of simple interest is 10%. If you pay this debt one year late, then a 10% late interest penalty of $10 should be added, making your debt payment $110. This is no different from your friend receiving the $100 today and investing it himself at 10% interest so that it accumulates to $110 in one year.
- Early Payments. If a debt is paid early, there should be some financial incentive (otherwise, why bother?). Therefore, an interest benefit, one reflecting a current rate of interest on the early payment, should be deducted from the original payment. Assume you owe your friend $110 one year from now and that a fair current rate of simple interest is 10%. If you pay this debt today, then a 10% early interest benefit of $10 should be deducted, making your debt payment today $100. If your friend then invests this money at 10% simple interest, one year from now he will have the $110, which is what you were supposed to pay.
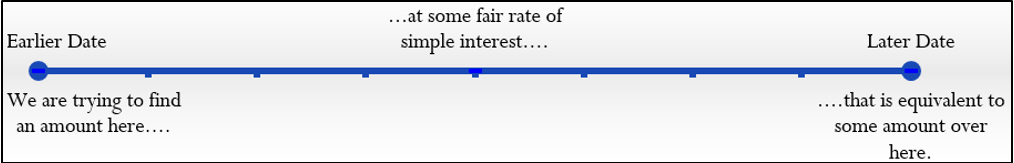
Notice in these examples that a simple interest rate of 10% means $100 today is the same thing as having $110 one year from now. This illustrates the concept that two payments are equivalent payments if, once a fair rate of interest is factored in, they have the same value on the same day. Thus, in general you are finding two amounts at different points in time that have the same value, as illustrated in the figure below.
How It Works
The steps required to calculate an equivalent payment are no different from those for single payments. If an early payment is being made, then you know the future value, so you solve for the present value (which removes the interest). If a late payment is being made, then you know the present value, so you solve for the future value (which adds the interest penalty).
Paths To Success
Being financially smart means paying attention to when you make your debt payments. If you receive no financial benefit for making an early payment, then why make it? The prudent choice is to keep the money yourself, invest it at the best interest rate possible, and pay the debt off when it comes due. Whatever interest is earned is yours to keep and you still fulfill your debt obligations in a timely manner!
Erin owes Charlotte $1,500 today. Unfortunately, Erin had some unexpected expenses and is unable to make her debt payment. After discussing the issue, they agree that Erin can make the payment nine months late and that a fair simple interest rate on the late payment is 5%. How much does Erin need to pay nine months from now? What is the amount of her late penalty?
Solution
A late payment is a future value amount (\(S\)). The late penalty is equal to the interest (\(I\)).
What You Already Know
Step 1:
The payment today, the agreed-upon interest rate, and the late payment timing are known: \(P = \$1,500, r = 5\%\) annually, \(t = 9\) months
How You Will Get There
Step 2:
While the rate is annual, the time is in months. Convert the time to an annual number.

Step 3:
Apply Formula 8.2.
Step 4:
Apply Formula 8.3 to calculate the late interest penalty amount.
Perform
Step 2:
Nine months out of 12 months in a year is \(t=\dfrac{9}{12}\).
Step 3:
\[S=\$ 1,500 \times\left(1+5 \% \times \dfrac{9}{12}\right)=\$ 1,500 \times(1+0.05 \times 0.75)=\$ 1,556.25 \nonumber \]
Step 4:
\[I = \$1,556.25 − \$1,500.00 = \$56.25 \nonumber \]
Erin's late payment is for $1,556.25, which includes a $56.25 interest penalty for making the payment nine months late.
Rupert owes Aminata two debt payments: $600 four months from now and $475 eleven months from now. Rupert came into some money today and would like to pay off both of the debts immediately. Aminata has agreed that a fair interest rate is 7%. What amount should Rupert pay today? What is the total amount of his early payment benefit?
Solution
An early payment is a present value amount (\(P\)). Both payments will be moved to today and summed. The early payment benefit will be the total amount of interest removed (\(I\)).
What You Already Know
Step 1:
The two payments, interest rate, and payment due dates are known: \(r = 7\%\) annually
First Payment: \(S = \$600, t = 4\) months from now
Second Payment: \(S = \$475, t = 11\) months from now
Replacement payment is being made today.
How You Will Get There
Do steps 2 and 3 for each payment:
Step 2:
While the rate is annual, the time is in months. Convert the time to an annual number.

Step 3:
Apply Formula 8.2, rearranging it to solve for \(P\). Once you calculate both \(P\), they can be summed to arrive at the total payment.
Step 4:
For each payment, apply Formula 8.3 to calculate the associated early interest benefit. Total the two values of \(I\) to get the early interest benefit overall.
Perform
Payment #1:
Step 2:
Four months out of 12 months in a year is \(t=\dfrac{4}{12}\)
Step 3:
\[\begin{aligned} \$ 600&=P\left(1+7 \% \times \dfrac{4}{12}\right)\\ P&=\dfrac{\$ 600}{(1+0.07 \times 0.\overline3)}\\ & =\$ 586.32 \end{aligned} \nonumber \]
Payment #2:
Step 2:
Eleven months out of 12 months in a year is \(t=\dfrac{11}{12}\)
Step 3:
\[\begin{aligned} &\$ 475=P\left(1+7 \% \times \dfrac{11}{12}\right)\\ &P=\dfrac{\$ 475}{(1+0.07 \times 0.91 \overline{6})}=\$ 446.36 \end{aligned} \nonumber \]
Totals:
\(P\) today \(= $586.32 + $446.36 = $1,032.68\)
Step 4:
Payment #1: \(I=S–P = $600 – $586.32 = $13.68 \)
Payment #2: \(I=S–P = $475 – $446.36 = $28.64 \)
\[I = \$13.68 + \$28.64 = \$42.32 \nonumber \]
To clear both debts today, Rupert pays $1,032.68, which reflects a $42.32 interest benefit reduction for the early payment.


