6.2E: Exercises for Section 6.2

Last updated
Save as PDF

Page ID: 69809

Gilbert Strang & Edwin “Jed” Herman
OpenStax

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $ $ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $$\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$ $\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$$\newcommand{\AA}{\unicode[.8,0]{x212B}}$

1) Derive the formula for the volume of a sphere using the slicing method.

2) Use the slicing method to derive the formula for the volume of a cone.

3) Use the slicing method to derive the formula for the volume of a tetrahedron with side length $a.$

4) Use the disk method to derive the formula for the volume of a trapezoidal cylinder.

5) Explain when you would use the disk method versus the washer method. When are they interchangeable?

Volumes by Slicing

For exercises 6 - 10, draw a typical slice and find the volume using the slicing method for the given volume.

6) A pyramid with height 6 units and square base of side 2 units, as pictured here.

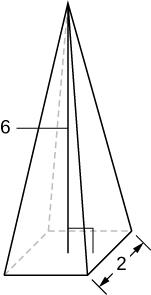
$This figure is a pyramid with base width of 2 and height of 6 units.$

Solution:: Here the cross-sections are squares taken perpendicular to the $y$-axis.
We use the vertical cross-section of the pyramid through its center to obtain an equation relating $x$ and $y$.
Here this would be the equation, $ y = 6 - 6x $. Since we need the dimensions of the square at each $y$-level, we solve this equation for $x$ to get, $x = 1 - \tfrac{y}{6}$.
This is half the distance across the square cross-section at the $y$-level, so the side length of the square cross-section is, $s = 2\left(1 - \tfrac{y}{6}\right).$
Thus, we have the area of a cross-section is,

$A(y) = \left[2\left(1 - \tfrac{y}{6}\right)\right]^2 = 4\left(1 - \tfrac{y}{6}\right)^2.$

$\begin{align*} \text{Then},\quad V &= \int_0^6 4\left(1 - \tfrac{y}{6}\right)^2 \, dy \\[5pt]
&= -24 \int_1^0 u^2 \, du, \quad \text{where} \, u = 1 - \tfrac{y}{6}, \, \text{so} \, du = -\tfrac{1}{6}\,dy, \quad \implies \quad -6\,du = dy \\[5pt]
&= 24 \int_0^1 u^2 \, du = 24\dfrac{u^3}{3}\bigg|_0^1 \\[5pt]
&= 8u^3\bigg|_0^1 \\[5pt]
&= 8\left( 1^3 - 0^3 \right) \quad= \quad 8\, \text{units}^3 \end{align*}$

7) A pyramid with height 4 units and a rectangular base with length 2 units and width 3 units, as pictured here.

$This figure is a pyramid with base width of 2, length of 3, and height of 4 units.$

8) A tetrahedron with a base side of 4 units,as seen here.

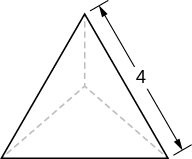
$This figure is an equilateral triangle with side length of 4 units.$

Answer: $V = \frac{32}{3\sqrt{2}} = \frac{16\sqrt{2}}{3}$ units³

9) A pyramid with height 5 units, and an isosceles triangular base with lengths of 6 units and 8 units, as seen here.

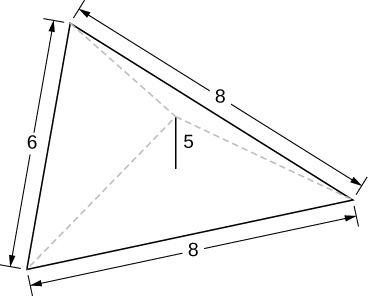
$This figure is a pyramid with a triangular base. The view is of the base. The sides of the triangle measure 6 units, 8 units, and 8 units. The height of the pyramid is 5 units.$

10) A cone of radius $ r$ and height $ h$ has a smaller cone of radius $ r/2$ and height $ h/2$ removed from the top, as seen here. The resulting solid is called a frustum.

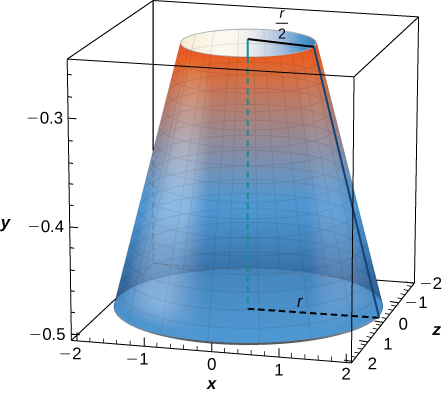
$This figure is a 3-dimensional graph of an upside down cone. The cone is inside of a rectangular prism that represents the xyz coordinate system. the radius of the bottom of the cone is “r” and the radius of the top of the cone is labeled “r/2”.$

Answer: $V = \frac{7\pi}{12} hr^2$ units³

For exercises 11 - 16, draw an outline of the solid and find the volume using the slicing method.

11) The base is a circle of radius $ a$. The slices perpendicular to the base are squares.

12) The base is a triangle with vertices $ (0,0),(1,0),$ and $ (0,1)$. Slices perpendicular to the $xy$-plane are semicircles.

Answer

$This figure shows the x-axis and the y-axis with a line starting on the x-axis at (1,0) and ending on the y-axis at (0,1). Perpendicular to the xy-plane are 4 shaded semi-circles with their diameters beginning on the x-axis and ending on the line, decreasing in size away from the origin.$

$\displaystyle V = \int_0^1 \frac{\pi(1-x)^2}{8}\, dx \quad = \quad \frac{π}{24}$ units³

13) The base is the region under the parabola $ y=1−x^2$ in the first quadrant. Slices perpendicular to the $xy$-plane are squares.

14) The base is the region under the parabola $ y=1−x^2$ and above the $x$-axis. Slices perpendicular to the $y$-axis are squares.

Answer

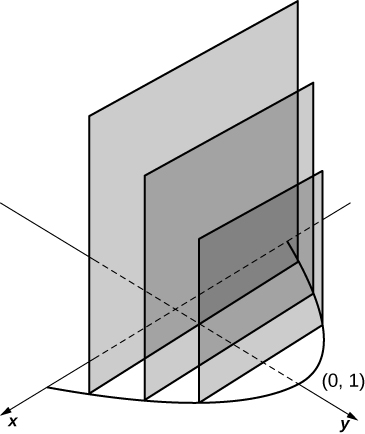
$This figure shows the x-axis and the y-axis in 3-dimensional perspective. On the graph above the x-axis is a parabola, which has its vertex at y=1 and x-intercepts at (-1,0) and (1,0). There are 3 square shaded regions perpendicular to the x y plane, which touch the parabola on either side, decreasing in size away from the origin.$

$\displaystyle V = \int_0^1 4(1 - y)\,dy \quad = \quad 2$ units³

15) The base is the region enclosed by $ y=x^2)$ and $ y=9.$ Slices perpendicular to the $x$-axis are right isosceles triangles.

16) The base is the area between $ y=x$ and $ y=x^2$. Slices perpendicular to the $x$-axis are semicircles.

Answer

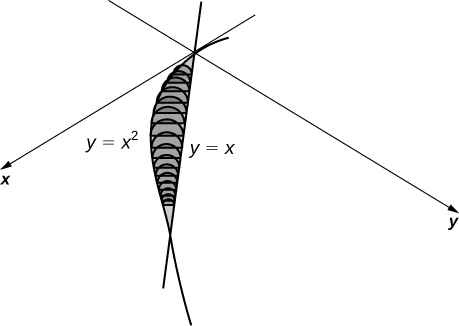
$This figure is a graph with the x and y axes diagonal to show 3-dimensional perspective. On the first quadrant of the graph are the curves y=x, a line, and y=x^2, a parabola. They intersect at the origin and at (1,1). Several semicircular-shaped shaded regions are perpendicular to the x y plane, which go from the parabola to the line and perpendicular to the line.$

$\displaystyle V = \int_0^1 \frac{\pi}{8}\left( x - x^2 \right)^2 \, dx \quad=\quad \frac{π}{240}$ units³

Disk and Washer Method

For exercises 17 - 24, draw the region bounded by the curves. Then, use the disk or washer method to find the volume when the region is rotated around the $x$-axis.

17) $ x+y=8,\quad x=0$, and $ y=0$

18) $ y=2x^2,\quad x=0,\quad x=4,$ and $ y=0$

Answer

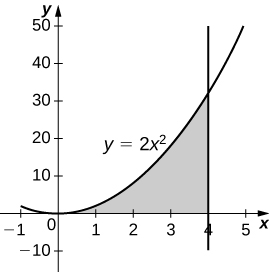
$This figure is a graph in the first quadrant. It is a shaded region bounded above by the curve y=2x^2, below by the x-axis, and to the right by the vertical line x=4.$

$\displaystyle V = \int_0^4 4\pi x^4\, dx \quad=\quad \frac{4096π}{5}$ units³

19) $ y=e^x+1,\quad x=0,\quad x=1,$ and $ y=0$

20) $ y=x^4,\quad x=0$, and $ y=1$

Answer

$This figure is a graph in the first quadrant. It is a shaded region bounded above by the line y=1, below by the curve y=x^4, and to the left by the y-axis.$

$\displaystyle V = \int_0^1 \pi\left( 1^2 - \left( x^4\right)^2\right)\, dx = \int_0^1 \pi\left( 1 - x^8\right)\, dx \quad = \quad \frac{8π}{9}$ units³

21) $ y=\sqrt{x},\quad x=0,\quad x=4,$ and $ y=0$

22) $ y=\sin x,\quad y=\cos x,$ and $ x=0$

Answer

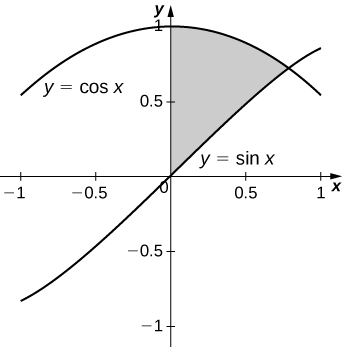
$This figure is a shaded region bounded above by the curve y=cos(x), below to the left by the y-axis and below to the right by y=sin(x). The shaded region is in the first quadrant.$

$\displaystyle V = \int_0^{\pi/4} \pi \left( \cos^2 x - \sin^2 x\right) \, dx = \int_0^{\pi/4} \pi \cos 2x \, dx \quad=\quad \frac{π}{2}$ units³

23) $ y=\dfrac{1}{x},\quad x=2$, and $ y=3$

24) $ x^2−y^2=9$ and $ x+y=9,\quad y=0$ and $ x=0$

Answer

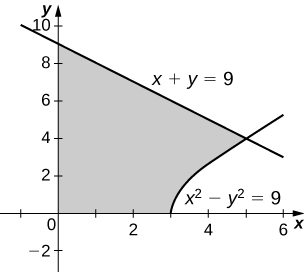
$This figure is a graph in the first quadrant. It is a shaded region bounded above by the line x + y=9, below by the x-axis, to the left by the y-axis, and to the left by the curve x^2-y^2=9.$

$V = 207π$ units³

For exercises 25 - 32, draw the region bounded by the curves. Then, find the volume when the region is rotated around the $y$-axis.

25) $ y=4−\dfrac{1}{2}x,\quad x=0,$ and $ y=0$

26) $ y=2x^3,\quad x=0,\quad x=1,$ and $ y=0$

Answer

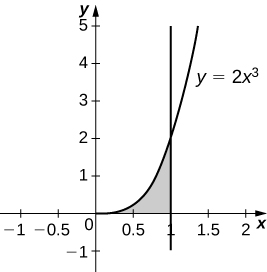
$This figure is a graph in the first quadrant. It is a shaded region bounded above by the curve y=2x^3, below by the x-axis, and to the right by the line x=1.$

$V = \frac{4π}{5}$ units³

27) $ y=3x^2,\quad x=0,$ and $ y=3$

28) $ y=\sqrt{4−x^2},\quad y=0,$ and $ x=0$

Answer

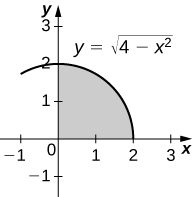
$This figure is a graph in the first quadrant. It is a quarter of a circle with center at the origin and radius of 2. It is shaded on the inside.$

$V = \frac{16π}{3}$ units³

29) $ y=\dfrac{1}{\sqrt{x+1}},\quad x=0$, and $ x=3$

30) $ x=\sec(y)$ and $ y=\dfrac{π}{4},\quad y=0$ and $ x=0$

Answer

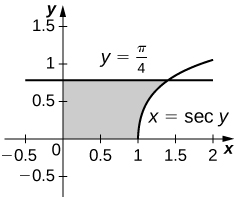
$This figure is a graph in the first quadrant. It is a shaded region bounded above by the line y=pi/4, to the right by the curve x=sec(y), below by the x-axis, and to the left by the y-axis.$

$V = π$ units³

31) $ y=\dfrac{1}{x+1},\quad x=0$, and $ x=2$

32) $ y=4−x,\quad y=x,$ and $ x=0$

Answer

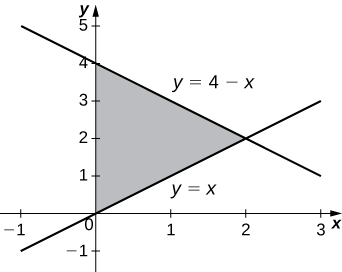
$This figure is a graph in the first quadrant. It is a shaded triangle bounded above by the line y=4-x, below by the line y=x, and to the left by the y-axis.$

$V = \frac{16π}{3}$ units³

For exercises 33 - 40, draw the region bounded by the curves. Then, find the volume when the region is rotated around the $x$-axis.

33) $ y=x+2,\quad y=x+6,\quad x=0$, and $ x=5$

34) $ y=x^2$ and $ y=x+2$

Answer

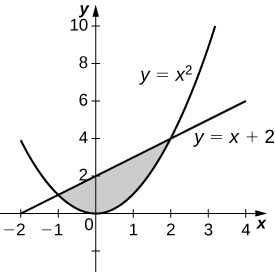
$This figure is a graph above the x-axis. It is a shaded region bounded above by the line y=x+2, and below by the parabola y=x^2.$

$V = \frac{72π}{5}$ units³

35) $ x^2=y^3$ and $ x^3=y^2$

36) $ y=4−x^2$ and $ y=2−x$

Answer

$This figure is a shaded region bounded above by the curve y=4-x^2 and below by the line y=2-x.$

$V = \frac{108π}{5}$ units³

37) [T] $ y=\cos x,\quad y=e^{−x},\quad x=0$, and $ x=1.2927$

38) $ y=\sqrt{x}$ and $ y=x^2$

Answer

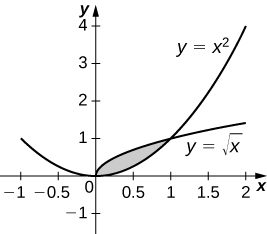
$This figure is a graph in the first quadrant. It is a shaded region bounded above by the curve y=squareroot(x), below by the curve y=x^2.$

$V = \frac{3π}{10}$ units³

39) $ y=\sin x,\quad y=5\sin x,\quad x=0$ and $ x=π$

40) $ y=\sqrt{1+x^2}$ and $ y=\sqrt{4−x^2}$

Answer

$This figure is a shaded region bounded above by the curve y=squareroot(4-x^2) and, below by the curve y=squareroot(1+x^2).$

$V = 2\sqrt{6}π$ units³

For exercises 41 - 45, draw the region bounded by the curves. Then, use the washer method to find the volume when the region is revolved around the $y$-axis.

41) $ y=\sqrt{x},\quad x=4$, and $ y=0$

42) $ y=x+2,\quad y=2x−1$, and $ x=0$

Answer

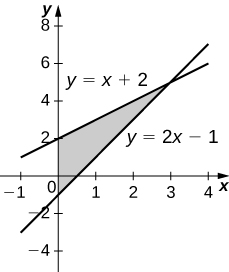
$This figure is a graph in the first quadrant. It is a shaded region bounded above by the line y=x+2, below by the line y=2x-1, and to the left by the y-axis.$

$V = 9π$ units³

43) $ y=\dfrac{3}{x}$ and $ y=x^3$

44) $ x=e^{2y},\quad x=y^2,\quad y=0$, and $ y=\ln(2)$

Answer

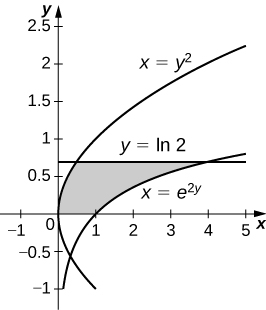
$This figure is a graph in the first quadrant. It is a shaded region bounded above by the curve y=ln(2), below by the x-axis, to the left by the curve x=y^2, and to the right by the curve x=e^(2y).$

$V = \dfrac{π}{20}(75−4\ln^5(2))$ units³

45) $ x=\sqrt{9−y^2},\quad x=e^{−y},\quad y=0$, and $ y=3$

46) Yogurt containers can be shaped like frustums. Rotate the line $ y=\left(\frac{1}{m}\right)x$ around the $y$-axis to find the volume between $ y=a$ and $ y=b$.

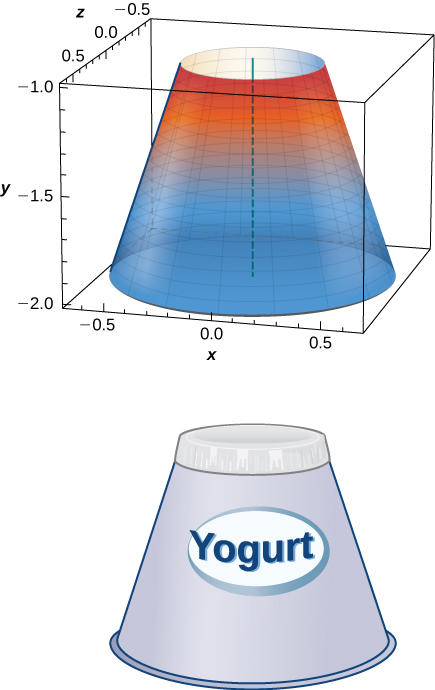
$This figure has two parts. The first part is a solid cone. The base of the cone is wider than the top. It is shown in a 3-dimensional box. Underneath the cone is an image of a yogurt container with the same shape as the figure.$

Answer: $V = \dfrac{m^2π}{3}(b^3−a^3)$ units³

47) Rotate the ellipse $ \dfrac{x^2}{a^2}+\dfrac{y^2}{b^2}=1$ around the $x$-axis to approximate the volume of a football, as seen here.

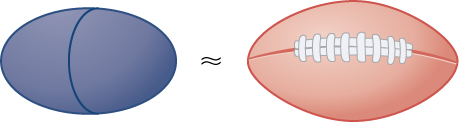
$This figure has an oval that is approximately equal to the image of a football.$

48) Rotate the ellipse $ \dfrac{x^2}{a^2}+\dfrac{y^2}{b^2}=1$ around the $y$-axis to approximate the volume of a football.

Answer: $V = \frac{4a^2bπ}{3}$ units³

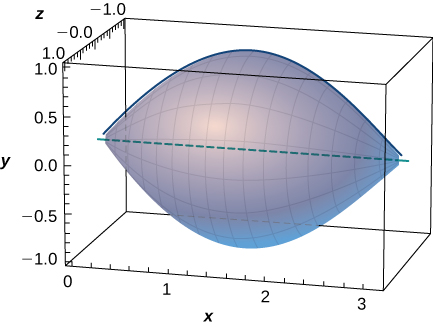
49) A better approximation of the volume of a football is given by the solid that comes from rotating $ y=\sin x) around the \(x$-axis from $ x=0$ to $ x=π$. What is the volume of this football approximation, as seen here?

$This figure has a 3-dimensional oval shape. It is inside of a box parallel to the x axis on the bottom front edge of the box. The y-axis is vertical to the solid.$

For exercises 51 - 56, find the volume of the solid described.

51) The base is the region between $ y=x$ and $ y=x^2$. Slices perpendicular to the $x$-axis are semicircles.

52) The base is the region enclosed by the generic ellipse $ \dfrac{x^2}{a^2}+\dfrac{y^2}{b^2}=1.$ Slices perpendicular to the $x$-axis are semicircles.

Answer: $V = \frac{2ab^2π}{3}$ units³

53) Bore a hole of radius a down the axis of $a$ right cone and through the base of radius $b$, as seen here.

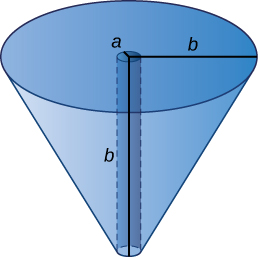
$This figure is an upside down cone. It has a radius of the top as “b”, center at “a”, and height as “b”.$

54) Find the volume common to two spheres of radius $r$ with centers that are $2h$ apart, as shown here.

$This figure has two circles that intersect. Both circles have radius “r”. There is a line segment from one center to the other. In the middle of the intersection of the circles is point “h”. It is on the line segment.$

Answer: $V = \frac{π}{12}(r+h)^2(6r−h)$ units³

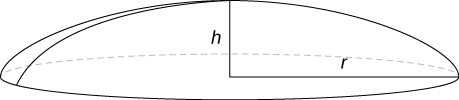
55) Find the volume of a spherical cap of height $h$ and radius $r$ where $h<r$, as seen here.

$This figure a portion of a sphere. This spherical cap has radius “r” and height “h”.$

56) Find the volume of a sphere of radius $R$ with a cap of height $h$ removed from the top, as seen here.

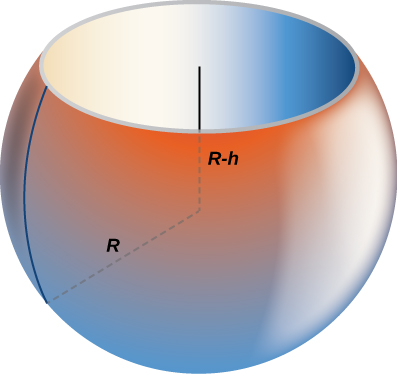
$This figure is a sphere with a top portion removed. The radius of the sphere is “R”. The distance from the center to where the top portion is removed is “R-h”.$

Answer: $V = \dfrac{π}{3}(h+R)(h−2R)^2$ units³

Contributors

Gilbert Strang (MIT) and Edwin “Jed” Herman (Harvey Mudd) with many contributing authors. This content by OpenStax is licensed with a CC-BY-SA-NC 4.0 license. Download for free at http://cnx.org.