15.6E: Exercises for Section 15.6

Last updated
Save as PDF

Page ID: 66955

Gilbert Strang & Edwin “Jed” Herman
OpenStax

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $ $ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $$\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$ $\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$$\newcommand{\AA}{\unicode[.8,0]{x212B}}$

In exercises 1 - 12, the region $R$ occupied by a lamina is shown in a graph. Find the mass of $R$ with the density function $\rho$.

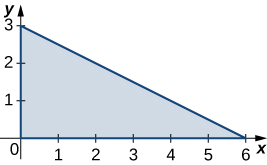
1. $R$ is the triangular region with vertices $(0,0), \space (0,3)$, and $(6,0); \space \rho (x,y) = xy$.

$A right triangle bounded by the x and y axes and the line y = negative x/2 + 3.$

Answer: $\frac{27}{2}$

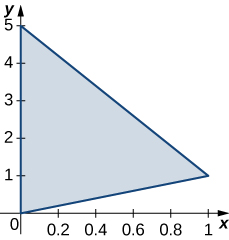
2. $R$ is the triangular region with vertices $(0,0), \space (1,1)$, and $(0,5); \space \rho (x,y) = x + y$.

$A triangle bounded by the y axis, the line x = y, and the line y = negative 4x + 5.$

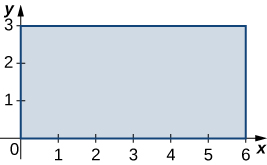
3. $R$ is the rectangular region with vertices $(0,0), \space (0,3), \space (6,3) $ and $(6,0); \space \rho (x,y) = \sqrt{xy}$.

$A rectangle bounded by the x and y axes and the lines x = 6 and y = 3.$

Answer: $24\sqrt{2}$

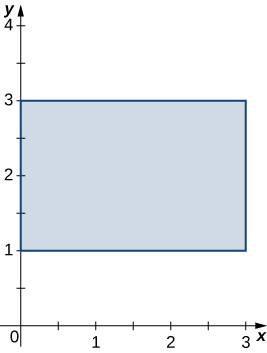
4. $R$ is the rectangular region with vertices $(0,1), \space (0,3), \space (3,3)$ and $ (3,1); \space \rho (x,y) = x^2y$.

$A rectangle bounded by the y axis, the lines y = 1 and 3, and the line x = 3.$

5. $R$ is the trapezoidal region determined by the lines $y = - \frac{1}{4}x + \frac{5}{2}, \space y = 0, \space y = 2$, and $x = 0; \space \rho (x,y) = 3xy$.

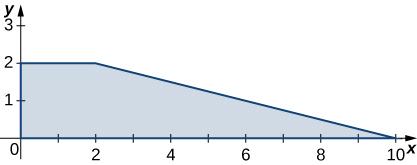
$A trapezoid bounded by the x and y axes, the line y = 2, and the line y = negative x/4 + 2.5.$

Answer: $76$

6. $R$ is the trapezoidal region determined by the lines $y = 0, \space y = 1, \space y = x$ and $y = -x + 3; \space \rho (x,y) = 2x + y$.

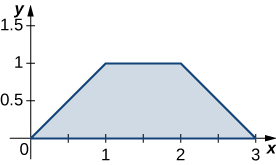
$A trapezoid bounded by the x axis, the line y = 1, the line y = x, and the line y = negative x + 3.$

7. $R$ is the disk of radius $2$ centered at $(1,2); \space \rho(x,y) = x^2 + y^2 - 2x - 4y + 5$.

Answer: $8\pi$

8. $R$ is the unit disk; $\rho (x,y) = 3x^4 + 6x^2y^2 + 3y^4$.

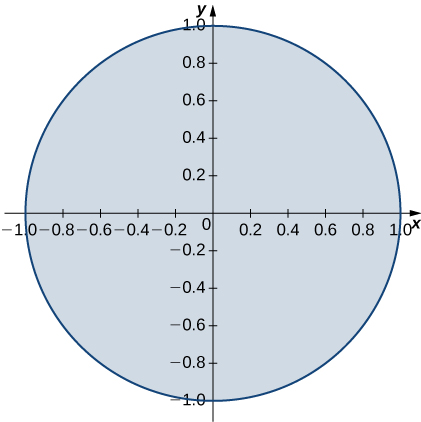
$A circle with radius 1 and center the origin.$

9. $R$ is the region enclosed by the ellipse $x^2 + 4y^2 = 1; \space \rho(x,y) = 1$.

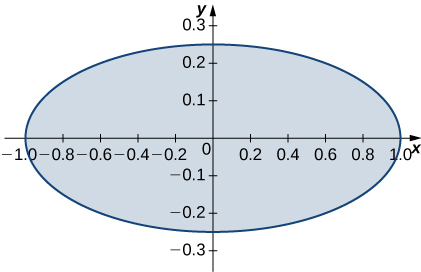
$An ellipse with center the origin, major axis 2, and minor axis 0.5.$

Answer: $\frac{\pi}{2}$

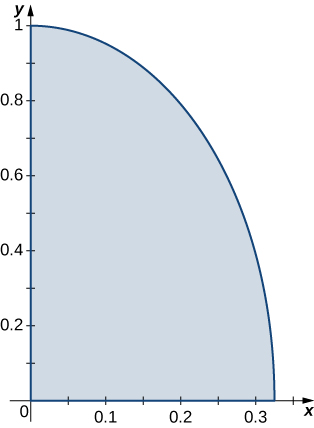
10. $R = \big\{(x,y) \,|\, 9x^2 + y^2 \leq 1, \space x \geq 0, \space y \geq 0\big\} ; \space \rho (x,y) = \sqrt{9x^2 + y^2}$.

$The quarter section of an ellipse in the first quadrant with center the origin, major axis 2, and minor axis roughly 0.64.$

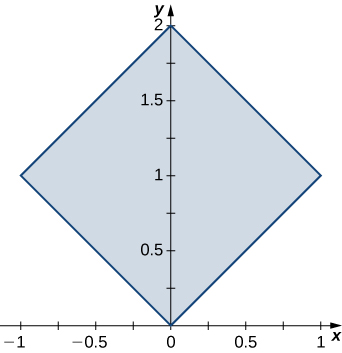
11. $R$ is the region bounded by $y = x, \space y = -x, \space y = x + 2, \space y = -x + 2; \space \rho(x,y) = 1$.

$A square with side length square root of 2 rotated 45 degrees, with corners at the origin, (2, 0), (1, 1), and (negative 1, 1).$

Answer: $2$

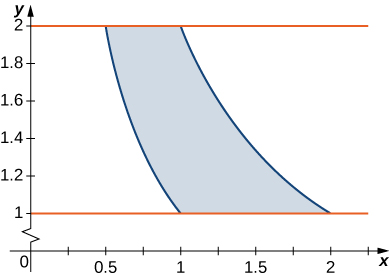
12. $R$ is the region bounded by $y = \frac{1}{x}, \space y = \frac{2}{x}, \space y = 1$, and $y = 2; \space \rho (x,y) = 4(x + y)$.

$A complex region between 2 and 1 that sweeps down and to the right with boundaries y = 1/x and y = 2/x.$

In exercises 13 - 24, consider a lamina occupying the region $R$ and having the density function $\rho$ given in the preceding group of exercises. Use a computer algebra system (CAS) to answer the following questions.

a. Find the moments $M_x$ and $M_y$ about the $x$-axis and $y$-axis, respectively.

b. Calculate and plot the center of mass of the lamina.

c. [T] Use a CAS to locate the center of mass on the graph of $R$.

13. [T] $R$ is the triangular region with vertices $(0,0), \space (0,3)$, and $(6,0); \space \rho (x,y) = xy$.

Answer

a. $M_x = \frac{81}{5}, \space M_y = \frac{162}{5}$;
b. $\bar{x} = \frac{12}{5}, \space \bar{y} = \frac{6}{5}$;
c.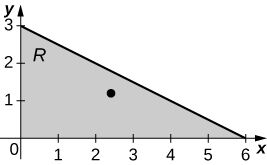

$A triangular region R bounded by the x and y axes and the line y = negative x/2 + 3, with a point marked at (12/5, 6/5).$

14. [T] $R$ is the triangular region with vertices $(0,0), \space (1,1)$, and $(0,5); \space \rho (x,y) = x + y$.

15. [T] $R$ is the rectangular region with vertices $(0,0), \space (0,3), \space (6,3)$, and $(6,0); \space \rho (x,y) = \sqrt{xy}$.

Answer

a. $M_x = \frac{216\sqrt{2}}{5}, \space M_y = \frac{432\sqrt{2}}{5}$;
b. $\bar{x} = \frac{18}{5}, \space \bar{y} = \frac{9}{5}$;
c.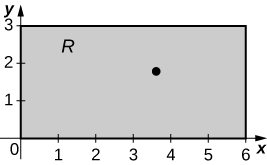

$A rectangle R bounded by the x and y axes and the lines x = 6 and y = 3 with point marked (18/5, 9/5).$

16. [T] $R$ is the rectangular region with vertices $(0,1), \space (0,3), \space (3,3)$, and $(3,1); \space \rho (x,y) = x^2y$.

17. [T] $R$ is the trapezoidal region determined by the lines $y = - \frac{1}{4}x + \frac{5}{2}, \space y = 0, \space y = 2$, and $x = 0; \space \rho (x,y) = 3xy$.

Answer

a. $M_x = \frac{368}{5}, \space M_y = \frac{1552}{5}$;
b. $\bar{x} = \frac{92}{95}, \space \bar{y} = \frac{388}{95}$;
c.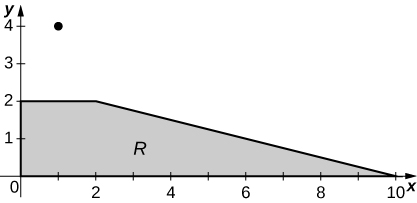

$A trapezoid R bounded by the x and y axes, the line y = 2, and the line y = negative x/4 + 2.5 with the point marked (92/95, 388/95).$

18. [T] $R$ is the trapezoidal region determined by the lines $y = 0, \space y = 1, \space y = x,$ and $y = -x + 3; \space \rho (x,y) = 2x + y$.

19. [T] $R$ is the disk of radius $2$ centered at $(1,2); \space \rho(x,y) = x^2 + y^2 - 2x - 4y + 5$.

Answer

a. $M_x = 16\pi, \space M_y = 8\pi$;
b. $\bar{x} = 1, \space \bar{y} = 2$;
c.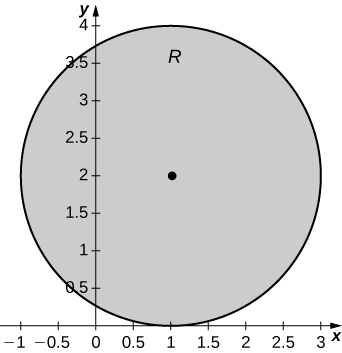

$A circle with radius 2 centered at (1, 2), which is tangent to the x axis at (1, 0) and has pointed marked at the center (1, 2).$

20. [T] $R$ is the unit disk; $\rho (x,y) = 3x^4 + 6x^2y^2 + 3y^4$.

21. [T] $R$ is the region enclosed by the ellipse $x^2 + 4y^2 = 1; \space \rho(x,y) = 1$.

Answer

a. $M_x = 0, \space M_y = 0)$;
b. $\bar{x} = 0, \space \bar{y} = 0$;
c.

$An ellipse R with center the origin, major axis 2, and minor axis 0.5, with point marked at the origin.$

22. [T] $R = \big\{(x,y) \,|\, 9x^2 + y^2 \leq 1, \space x \geq 0, \space y \geq 0\big\} ; \space \rho (x,y) = \sqrt{9x^2 + y^2}$.

23. [T] $R$ is the region bounded by $y = x, \space y = -x, \space y = x + 2$, and $y = -x + 2; \space \rho (x,y) = 1$.

Answer

a. $M_x = 2, \space M_y = 0)$;
b. $\bar{x} = 0, \space \bar{y} = 1$;
c.

$A square R with side length square root of 2 rotated 45 degrees, with corners at the origin, (2, 0), (1, 1), and (negative 1, 1). A point is marked at (0, 1).$

24. [T] $R$ is the region bounded by $y = \frac{1}{x}, \space y = \frac{2}{x}, \space y = 1$, and $y = 2; \space \rho (x,y) = 4(x + y)$.

In exercises 25 - 36, consider a lamina occupying the region $R$ and having the density function $\rho$ given in the first two groups of Exercises.

a. Find the moments of inertia $I_x$, $I_y$ and $I_0$ about the $x$-axis, $y$-axis, and origin, respectively.

b. Find the radii of gyration with respect to the $x$-axis, $y$-axis, and origin, respectively.

25. $R$ is the triangular region with vertices $(0,0), \space (0,3)$, and $(6,0); \space \rho (x,y) = xy$.

Answer: a. $I_x = \frac{243}{10}, \space I_y = \frac{486}{5}$, and $I_0 = \frac{243}{2}$;
b. $R_x = \frac{3\sqrt{5}}{5}, \space R_y = \frac{6\sqrt{5}}{5}$, and $R_0 = 3$

26. $R$ is the triangular region with vertices $(0,0), \space (1,1)$, and $(0,5); \space \rho (x,y) = x + y$.

27. $R$ is the rectangular region with vertices $(0,0), \space (0,3), \space (6,3)$, and $(6,0); \space \rho (x,y) = \sqrt{xy}$.

Answer: a. $I_x = \frac{2592\sqrt{2}}{7}, \space I_y = \frac{648\sqrt{2}}{7}$, and $I_0 = \frac{3240\sqrt{2}}{7}$;
b. $R_x = \frac{6\sqrt{21}}{7}, \space R_y = \frac{3\sqrt{21}}{7}$, and $R_0 = \frac{3\sqrt{106}}{7}$

28. $R$ is the rectangular region with vertices $(0,1), \space (0,3), \space (3,3)$, and $(3,1); \space \rho (x,y) = x^2y$.

29. $R$ is the trapezoidal region determined by the lines $y = - \frac{1}{4}x + \frac{5}{2}, \space y = 0, \space y = 2$, and x = 0; \space \rho (x,y) = 3xy\).

Answer: a. $I_x = 88, \space I_y = 1560$, and $I_0 = 1648$;
b. $R_x = \frac{\sqrt{418}}{19}, \space R_y = \frac{\sqrt{7410}}{10}$, and $R_0 = \frac{2\sqrt{1957}}{19}$

30. $R$ is the trapezoidal region determined by the lines $y = 0, \space y = 1, \space y = x$, and y = -x + 3; \space \rho (x,y) = 2x + y\).

31. $R$ is the disk of radius $2$ centered at $(1,2); \space \rho(x,y) = x^2 + y^2 - 2x - 4y + 5$.

Answer: a. $I_x = \frac{128\pi}{3}, \space I_y = \frac{56\pi}{3}$, and $I_0 = \frac{184\pi}{3}$;
b. $R_x = \frac{4\sqrt{3}}{3}, \space R_y = \frac{\sqrt{21}}{2}$, and $R_0 = \frac{\sqrt{69}}{3}$

32. $R$ is the unit disk; $\rho (x,y) = 3x^4 + 6x^2y^2 + 3y^4$.

33. $R$ is the region enclosed by the ellipse $x^2 + 4y^2 = 1; \space \rho(x,y) = 1$.

Answer: a. $I_x = \frac{\pi}{32}, \space I_y = \frac{\pi}{8}$, and $I_0 = \frac{5\pi}{32}$;
b. $R_x = \frac{1}{4}, \space R_y = \frac{1}{2}$, and $R_0 = \frac{\sqrt{5}}{4}$

34. $R = \big\{(x,y) \,|\, 9x^2 + y^2 \leq 1, \space x \geq 0, \space y \geq 0\big\} ; \space \rho (x,y) = \sqrt{9x^2 + y^2}$.

35. $R$ is the region bounded by $y = x, \space y = -x, \space y = x + 2$, and $y = -x + 2; \space \rho (x,y) = 1$.

Answer: a. $I_x = \frac{7}{3}, \space I_y = \frac{1}{3}$, and $I_0 = \frac{8}{3}$;
b. $R_x = \frac{\sqrt{42}}{6}, \space R_y = \frac{\sqrt{6}}{6}$, and $R_0 = \frac{2\sqrt{3}}{3}$

36. $R$ is the region bounded by $y = \frac{1}{x}, \space y = \frac{2}{x}, \space y = 1$, and $y = 2; \space \rho (x,y) = 4(x + y)$.

37. Let $Q$ be the solid unit cube. Find the mass of the solid if its density $\rho$ is equal to the square of the distance of an arbitrary point of $Q$ to the $xy$-plane.

Answer: $m = \frac{1}{3}$

38. Let $Q$ be the solid unit hemisphere. Find the mass of the solid if its density $\rho$ is proportional to the distance of an arbitrary point of $Q$ to the origin.

39. The solid $Q$ of constant density $1$ is situated inside the sphere $x^2 + y^2 + z^2 = 16$ and outside the sphere $x^2 + y^2 + z^2 = 1$. Show that the center of mass of the solid is not located within the solid.

40. Find the mass of the solid $Q = \big\{ (x,y,z) \,|\, 1 \leq x^2 + z^2 \leq 25, \space y \leq 1 - x^2 - z^2 \big\}$ whose density is $\rho (x,y,z) = k$, where $k > 0$.

41. [T] The solid $Q = \big\{ (x,y,z) \,|\, x^2 + y^2 \leq 9, \space 0 \leq z \leq 1, \space x \geq 0, \space y \geq 0\big\}$ has density equal to the distance to the $xy$-plane. Use a CAS to answer the following questions.

a. Find the mass of $Q$.

b. Find the moments $M_{xy}, \space M_{xz}$ and $M_{yz}$ about the $xy$-plane, $xz$-plane, and $yz$-plane, respectively.

c. Find the center of mass of $Q$.

d. Graph $Q$ and locate its center of mass.

Answer

a. $m = \frac{9\pi}{4}$;
b. $M_{xy} = \frac{3\pi}{2}, \space M_{xz} = \frac{81}{8}, \space M_{yz} = \frac{81}{8}$;
c. $\bar{x} = \frac{9}{2\pi}, \space \bar{y} = \frac{9}{2\pi}, \space \bar{z} = \frac{2}{3}$;
d.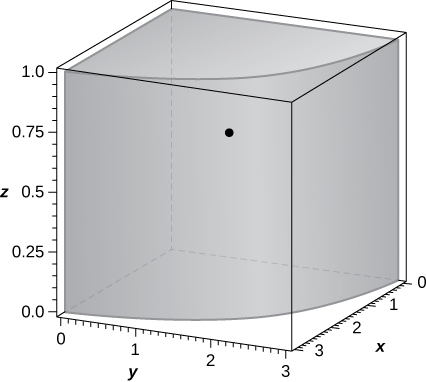

$A quarter cylinder in the first quadrant with height 1 and radius 3. A point is marked at (9/(2 pi), 9/(2 pi), 2/3).$

42. Consider the solid $Q = \big\{ (x,y,z) \,|\, 0 \leq x \leq 1, \space 0 \leq y \leq 2, \space 0 \leq z \leq 3\big\}$ with the density function $\rho(x,y,z) = x + y + 1$.

a. Find the mass of $Q$.

b. Find the moments $M_{xy}, \space M_{xz}$ and $M_{yz}$ about the $xy$-plane, $xz$-plane, and $yz$-plane, respectively.

c. Find the center of mass of $Q$.

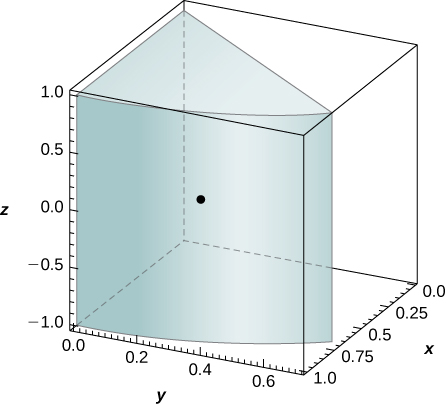
43. [T] The solid $Q$ has the mass given by the triple integral $\displaystyle \int_{-1}^1 \int_0^{\pi/4} \int_0^1 r^2 \, dr \space d\theta \space dz.$

Use a CAS to answer the following questions.

Show that the center of mass of $Q$ is located in the $xy$-plane.
Graph $Q$ and locate its center of mass.

Answer

$\bar{x} = \frac{3\sqrt{2}}{2\pi}$, $\bar{y} = \frac{3(2-\sqrt{2})}{2\pi}, \space \bar{z} = 0$; 2. the solid $Q$ and its center of mass are shown in the following figure.

$A wedge from a cylinder in the first quadrant with height 2, radius 1, and angle roughly 45 degrees. A point is marked at (3 times the square root of 2/(2 pi), 3 times (2 minus the square root of 2)/(2 pi), 0).$

44. The solid $Q$ is bounded by the planes $x + 4y + z = 8, \space x = 0, \space y = 0$, and $z = 0$. Its density at any point is equal to the distance to the $xz$-plane. Find the moments of inertia of the solid about the $xz$-plane.

45. The solid $Q$ is bounded by the planes $x + y + z = 3, \space x = 0, \space y = 0$, and $z = 0$. Its density is $\rho(x,y,z) = x + ay$, where $a > 0$. Show that the center of mass of the solid is located in the plane $z = \frac{3}{5}$ for any value of $a$.

46. Let $Q$ be the solid situated outside the sphere $x^2 + y^2 + z^2 = z$ and inside the upper hemisphere $x^2 + y^2 + z^2 = R^2$, where $R > 1$. If the density of the solid is $\rho (x,y,z) = \frac{1}{\sqrt{x^2+y^2+z^2}}$, find $R$ such that the mass of the solid is $\frac{7\pi}{2}.$

47. The mass of a solid $Q$ is given by $\displaystyle \int_0^2 \int_0^{\sqrt{4-x^2}} \int_{\sqrt{x^2+y^2}}^{\sqrt{16-x^2-y^2}} (x^2 + y^2 + z^2)^n \, dz \space dy \space dx,$ where $n$ is an integer. Determine $n$ such the mass of the solid is $(2 - \sqrt{2}) \pi$.

Answer: $n = -2$

48. Let $Q$ be the solid bounded above the cone $x^2 + y^2 = z^2$ and below the sphere $x^2 + y^2 + z^2 - 4z = 0$. Its density is a constant $k > 0$. Find $k$ such that the center of mass of the solid is situated $7$ units from the origin.

49. The solid $Q = \big\{(x,y,z) \,|\, 0 \leq x^2 + y^2 \leq 16, \space x \geq 0, \space y \geq 0, \space 0 \leq z \leq x\big\}$ has the density $\rho (x,y,z) = k$. Show that the moment $M_{xy}$ about the $xy$-plane is half of the moment $M_{yz}$ about the $yz$-plane.

50. The solid $Q$ is bounded by the cylinder $x^2 + y^2 = a^2$, the paraboloid $b^2 - z = x^2 + y^2$, and the $xy$-plane, where $0 < a < b$. Find the mass of the solid if its density is given by $\rho(x,y,z) = \sqrt{x^2 + y^2}$.

51. Let $Q$ be a solid of constant density $k$, where $k > 0$, that is located in the first octant, inside the circular cone $x^2 + y^2 = 9(z - 1)^2$, and above the plane $z = 0$. Show that the moment $M_{xy}$ about the $xy$-plane is the same as the moment $M_{yz}$ about the $xz$-plane.

52. The solid $Q$ has the mass given by the triple integral $\displaystyle \int_0^1 \int_0^{\pi/2} \int_0^{r^3} (r^4 + r) \space dz \space d\theta \space dr.$

a. Find the density of the solid in rectangular coordinates.

b. Find the moment $M_{xy}$ about the $xy$-plane.

53. The solid $Q$ has the moment of inertia $I_x$ about the $yz$-plane given by the triple integral $\displaystyle \int_0^2 \int_{-\sqrt{4-y^2}}^{\sqrt{4-y^2}} \int_{\frac{1}{2}(x^2+y^2)}^{\sqrt{x^2+y^2}} (y^2 + z^2)(x^2 + y^2) \, dz \space dx \space dy.$

a. Find the density of $Q$.

b. Find the moment of inertia $I_z$ about the $xy$-plane.

Answer: a. $\rho (x,y,z) = x^2 + y^2$;
b. $\frac{16\pi}{7}$

54. The solid $Q$ has the mass given by the triple integral $\displaystyle \int_0^{\pi/4} \int_0^{2 \space sec \space \theta} \int_0^1 (r^3 \cos \theta \sin \theta + 2r) \, dz \space dr \space d\theta.$

a. Find the density of the solid in rectangular coordinates.

b. Find the moment $M_{xz}$ about the $xz$-plane.

55. Let $Q$ be the solid bounded by the $xy$-plane, the cylinder $x^2 + y^2 = a^2$, and the plane $z = 1$, where $a > 1$ is a real number. Find the moment $M_{xy}$ of the solid about the $xy$-plane if its density given in cylindrical coordinates is $\rho(x,y,z) = \frac{d^2f}{dr^2} (r)$, where $f$ is a differentiable function with the first and second derivatives continuous and differentiable on $(0,a)$.

Answer: $M_{xy} = \pi (f(0) - f(a) + af'(a))$

56. A solid $Q$ has a volume given by $\displaystyle \iint_D \int_a^b \, dA \space dz$, where $D$ is the projection of the solid onto the $xy$-plane and $a < b$ are real numbers, and its density does not depend on the variable $z$. Show that its center of mass lies in the plane $z = \frac{a+b}{2}$.

57. Consider the solid enclosed by the cylinder $x^2 + z^2 = a^2$ and the planes $y = b$ and $y = c$, where $a > 0$ and $b < c$ are real numbers. The density of $Q$ is given by $\rho(x,y,z) = f'(y)$, where $f$ is a differential function whose derivative is continuous on $(b,c)$. Show that if $f(b) = f(c)$, then the moment of inertia about the $xz$-plane of $Q$ is null.

58. [T] The average density of a solid $Q$ is defined as $ \displaystyle \rho_{ave} = \frac{1}{V(Q)} \iiint_Q \rho(x,y,z) \, dV = \frac{m}{V(Q)},$ where $V(Q)$ and $m$ are the volume and the mass of $Q$, respectively. If the density of the unit ball centered at the origin is $\rho (x,y,z) = e^{-x^2-y^2-z^2}$, use a CAS to find its average density. Round your answer to three decimal places.

59. Show that the moments of inertia $I_x, \space I_y$, and $I_z$ about the $yz$-plane, $xz$-plane, and $xy$-plane, respectively, of the unit ball centered at the origin whose density is $\rho (x,y,z) = e^{-x^2-y^2-z^2}$ are the same. Round your answer to two decimal places.

Answer: $I_x = I_y = I_z \approx 0.84$

Contributors

Gilbert Strang (MIT) and Edwin “Jed” Herman (Harvey Mudd) with many contributing authors. This content by OpenStax is licensed with a CC-BY-SA-NC 4.0 license. Download for free at http://cnx.org.