10.3: Facts About Comparing Fractions

Assume a and c are whole numbers, and b and d are counting numbers.

Two fractions, a/b and c/d, are equivalent if and only if ad = bc. In other words,

\[ \frac{a}{b} = \frac{c}{d} \Leftrightarrow ad = bc \nonumber \]

Note: \(\Leftrightarrow\) means "if and only if", so if ad = bc, then the two fractions a/b and c/d are equivalent, and vice versa. Using this method to determine if two fractions are equivalent is called comparing cross products.

Examples: Determine if the following statements are true or false by comparing cross products. State whether the pair of fractions are equivalent or not.

Example 1

6/8 = 9/12

Solution

Since 6 \(\cdot\) 12 = 8 \(\cdot\) 9, the statement is true. Therefore, 3/4 and 9/12 are equivalent fractions.

Example 2

15/24 = 10/18

Solution

Since 15 \(\cdot\) 18 \(\neq\) 24 \(\cdot\) 10, the statement is false. Therefore, 15/24 and 10/18 are not equivalent fractions.

Exercise 31

Determine if the following statements are true or false by comparing cross products. State whether the pair of fractions are equivalent or not. Show your work and reasoning.

a. 14/25 = 28/50

b. 25/35 = 10/14

c. 12/16 = 25/40

The Fundamental Law of Fractions:

For any rational number, a/b and any integer c, a/b = ac/bc. The fractions a/b and ac/bc are called equivalent fractions.

Exercise 32

Write five equivalent fractions for each fraction given:

a. 2/3: __________________________________________________________

b. 5/7: __________________________________________________________

c. 3/8: __________________________________________________________

Two fractions, a/b and c/d, are equivalent if and only if the numerators are equal after writing each fraction with a common denominator.

Determine if the following statements are true or false. State whether the pair of fractions are equivalent or not.

Example 1

6/8 = 9/12

Solution

Write each fraction with a common denominator of 24 by applying the fundamental law of fractions: 6/8 = 18/24, and 9/12 = 18/24. Since the numerators are equal when each fraction is written with a common denominator, 6/8 and 9/12 are equivalent.

Example 2

15/24 = 10/18

Solution

Write each fraction with a common denominator of 72 by applying the fundamental law of fractions: 15/24 = 45/72, and 10/18 = 40/72. Since the numerators are not equal when each fraction is written with a common denominator, 15/24 and 10/18 are not equivalent.

Exercise 33

Determine if the following statements are true or false by writing each fraction with a common denominator. State whether the pair of fractions are equivalent or not. Show your work and reasoning.

a. 14/25 = 28/50

b. 25/35 = 10/14

c. 12/16 = 25/40

A fraction, a/b, is in simplest (reduced) form if GCF(a,b) = 1.

One way to simplify fractions is to prime factor the numerator and denominator, and then "cancel out" any common factors. Another way is to

(1) find the GCF of the numerator and denominator,

(2) rewrite both the numerator and denominator as the product of the GCF(a,b) and another factor, and then

(3) "cancel out" any common factors. When you cancel out everything from either the numerator and/or denominator, there is always a factor of 1 that is still there.

Example

Write 315/350 in simplest form by using each of the two methods just described.

Method 1: Prime factorization: \[\frac{315}{350} = \frac{3 \times 3 \times 5 \times 7}{2 \times 5 \times 5 \times 7} = \frac{9}{10} \nonumber \]

Method 2: GCF(315, 350) = 35: \[\frac{315}{350} = \frac{9 \times 35}{10 \times 35} = \frac{9}{10} \nonumber \]

Exercise 34

Write each fraction in simplest form using each of the two methods:

(1) prime factorization and

(2) finding GCF as shown in the previous example.

Show the actual factorization for each method, and then reduce to lowest terms.

a. Method 1: \(\frac{378}{675}\)

Method 2: \(\frac{378}{675}\)

b. Method 1: \(\frac{247}{323}\)

Method 2: \(\frac{247}{323}\)

Two fractions, a/b and c/d, are equivalent if the two fractions are equal when they are both written in simplest form.

Examples: Determine if the following statements are true or false. State whether the pair of fractions are equivalent or not.

Example 1

6/8 = 9/12

Solution

In simplest form, 6/8 = 3/4, and 9/12 = 3/4. Therefore, 6/8 and 9/12 are equivalent.

Example 2

15/24 = 10/18

Solution

In simplest form, 15/24 = 5/8, and 10/18 = 5/9. Therefore, 15/24 and 10/18 are not equivalent.

Exercise 35

35. Determine if the following statements are true or false by writing each fraction in simplest form. State whether the pair of fractions are equivalent or not. Show your work and reasoning.

a. 14/25 = 28/50

b. 25/35 = 10/14

c. 12/16 = 25/40

Below is a way to compare two fractions that are unequal by using cross products.

\[ \begin{aligned} \frac{a}{b} < \frac{c}{d} \Leftrightarrow ad < bc && \text{ and } && \frac{a}{b} > \frac{c}{d} \Leftrightarrow ad > bc \end{aligned} \nonumber \]

As you write the fractions, a/b < c/d, a is the first number written and d is the last number written. a and d are called the extremes (outside numbers when written as a/b < c/d), and their product is written on the left of the inequality sign when you take the cross products. The other two numbers, b and c, are called the means (when written as a/b < c/d, these are the inside numbers), and their product is written on the right of the inequality sign when you take the cross products. There are only three cases possible when comparing two fractions, a/b and c/d. Either the first fraction (a/b) is equal to the second (c/d), in which case ad = bc; the first (a/b) is less than the second (c/d), in which case ad < bc; or the first (a/b) is more than the second (c/d), in which case ad > bc.

Example 1

Compare 2/5 and 3/7 using cross products.

Solution

Multiply the extremes (2 and 7) and put on the left. Multiply the means (5 and 3) and put on the right. Compare the products.
Since 2\(\cdot\)7 < 5 \(\cdot\) 3, then 2/5 < 3/7.

Example 2

Compare 6/7 and 5/6 using cross products.

Solution

Multiply the outside numbers (6 and 6) and put on the left. Multiply the inside numbers (7 and 5) and put on the right. Compare the products.
Since 6\(\cdot\)6 > 7\(\cdot\)5, then 6/7 > 5/6.

Exercise 36

Use cross products to compare each of the following fractions. Use < or >.

a. 4/5 and 5/8

b. 12/35 and 11/18

c. 13/15 and 14/17

At the beginning of this exercise set, you compared several fractions using the fraction circles and fraction array. One problem was to compare 1/2, 1/3, 1/4, 1/5, 1/6, 1/8, 1/9 , 1/10, and 1/12, and put them in order from smallest to largest using the less than symbol.

The answer was: 1/12 < 1/10 < 1/9 < 1/8 < 1/6 < 1/5 < 1/4 < 1/3 < 1/2

This can easily be checked using cross products. You can check one at a time: 1/12 < 1/10 since 10 < 12, and 1/10 < 1/9 because 9 < 10, etc.

Exercise 37

Check the validity of the answer you got for exercise 9a. Write the answer. Then check using cross products.

Exercise 38

Check the validity of the answer you got for exercise 9b. Write the answer. Then check using cross products.

Adding and Subtracting Fractions

In order to add or subtract fractions, the fractions must have a common denominator. Below is the rule for adding or subtracting fractions that have a common denominator.

\[ \begin{aligned} \frac{a}{b} + \frac{c}{b} = \frac{a+c}{b} && \text{ and } && \frac{a}{b} - \frac{c}{b} = \frac{a-c}{b} \end{aligned} \nonumber \]

In order to add fractions that do not have a common denominator, you must first rewrite each fraction as an equivalent fraction so that both fractions have a common denominator, OR you may use the following rule for addition of fractions.

\[\frac{a}{b} + \frac{c}{d} = \frac{ad + bc}{bd} \nonumber \]

Note: If you are going to add fractions by rewriting each fraction with a common denominator, it is usually preferable to find the least common denominator, which is the least common multiple of the two denominators. Whether or not you do this, you should always write the answer in simplest form after adding or subtracting.

Multiplying two fractions

The rule for multiplying two fractions is to multiply the numerators together to obtain the new numerator, and multiply the denominators together to obtain the new denominator.

\[ \frac{a}{b} \cdot \frac{c}{d} = \frac{a \cdot c}{b \cdot d} \nonumber \]

It is easier to first "cancel out" any common factors before multiplying. This can be done by first prime factoring the numerators and denominators, and writing all the factors of the numerator in the numerator of the fraction, and all the factors of the denominator in the denominator of the fraction. Then, cancel any common factors before multiplying. If you do this, the fraction will already be in simplest form.

Dividing two fractions

To understand the rule for dividing two fractions, see if you can follow the reasoning below.

\[\frac{a}{b} \div \frac{c}{d} = \frac{\frac{a}{b}}{\frac{c}{d}} \cdot \frac{\frac{d}{c}}{\frac{d}{c}} = \frac{\frac{a}{b} \cdot \frac{d}{c}}{\frac{c}{d} \cdot \frac{d}{c}} = \frac{\frac{a}{b} \cdot \frac{d}{c}}{1} = \frac{a}{b} \cdot \frac{d}{c} \nonumber \]

The rule for dividing two fractions is this: Dividing by a fraction is the same as multiplying by the reciprocal of that fraction. Then, use the rule for multiplying as just explained.

\[\frac{a}{b} \div \frac{c}{d} = \frac{a}{b} \cdot \frac{d}{c} \nonumber \]

Exercise 39

Compute each of the following using the order of operations. Write each answer in simplest terms. Show all work.

a. \[ \frac{3}{4} \times \frac{4}{5} - \frac{5}{6} \div \frac{7}{3} \nonumber \]

b. \[\frac{5}{6} \div \frac{4}{5} + \frac{5}{6} \left( \frac{1}{3} - \frac{2}{5}\right) \nonumber \]

Unlike integers, there are infinitely many rational numbers between any two rational numbers. It doesn't make sense to talk about two consecutive rational numbers. For instance, between 4/9 and 5/9, there are infinitely many rational numbers. One easy way to list a few is to write equivalent fractions for 7/9 and 8/9. A simple common denominator would be 90, so look at 70/90 and 80/90. It's easy to see these rational numbers between 70/90 and 80/90: 71/90, 72/90, 73/90, 74/90, 75/90, 76/90, 77/90, 78/90, 79/90. Of course, had I chosen equivalent fractions with a larger denominator (900 or 900,000, etc.), you would easily be able to list many more rational numbers between 7/9 and 8/9.

If you wanted to list just one rational number between 7/9 and 8/9, you could choose one of the ones from the above list, or you could simply find the midpoint (or average) of the two. To find the average of any two numbers, add and divide by 2. Since dividing by 2 is the same as multiplying by one-half, add and multiply by one-half. Note the average of 7/9 and 8/9 is 1/2(7/9 + 8/9) = 1/2(15/9) = 15/18 or 5/6.

Exercise 40

Find 5 rational numbers, written with a common denominator, between 2/5 and 3/5.

Exercise 41

Find the average of 5/11 and 6/11.

Exercise 42

Find the average of 3/7 and 4/7

If you are asked to find several rational numbers between two rational numbers that do not have a common denominator, first you should rewrite each fraction as equivalent fractions having a common denominator. For instance, to find 4 rational numbers between 4/7 and 5/6, first rewrite 4/7 and 5/6 with a common denominator of 42: 24/42 and 35/42. In this case, it is easy to find 4 rational numbers between 4/7 and 5/6: 25/42, 26/42, 27/42, etc.

Here's another one: Find 5 rational numbers between 3/4 and 4/5. First, rewrite 3/4 and 4/5 with a common denominator of 20: 15/20 and 16/20. Then, find equivalent fractions with a larger denominator than 20: 150/200 and 160/200 are easy choices, although you could have picked 90/120 and 96/120. In any case, there are infinitely many choices. I probably would choose 151/200, 152/200, 153/200, 154/200 and 155/200.

Exercise 43

Find 3 rational numbers, written with a common denominator, between 1/3 and 2/5.

Exercise 44

Find 3 rational numbers, written with a common denominator, between 1/2 and 1/3. (Be careful: Which number is smaller and which is larger?)

Exercise 45

Find 5 rational numbers, written with a common denominator, between 5/6 and 4/5.

(Be careful: Which number is smaller and which is larger?)

Exercise 46

Find the average of 5/8 and 6/7.

Exercise 47

Find the average of 5/7 and 5/8.

Now, we'll work on some word problems where we can use the meaning of fractions to easily solve the problems. We'll use the fraction circles as manipulatives.

Problem: One day, 24 of my students showed up for class. This only represented 3/4 of my students. How many students were enrolled in the class? How many were absent?

Solution: We know that 24 is 3/4 of the total students in the class. That means 4/4 makes up all the students. Get out the fraction circle where 4 equal parts make up a whole. You know three of those parts represent 24 students. If 3 of those parts represent 24 students, then each of the equal parts represents 8 students. Since 4 equal parts represents the whole class, then there must be 8 · 4, or 32 students enrolled. One of the equal parts represents the absent students, so 8 students are absent.

You could also show this pictorially – you see circles in place of the fraction circles.

Step 1: 4 equal parts make up a whole.
Step 2: 3 equal parts, or 3/4, represents 24 students. The part left over represents 1/4 of the students.
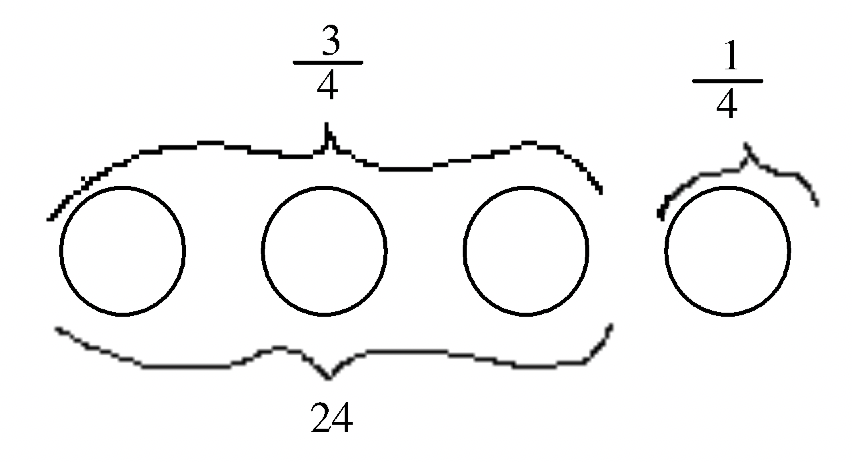Step 3: If those three circles represent 24, then 8 must be in each circle. Now it is clear there are 32 students in the class, and 8 are absent.

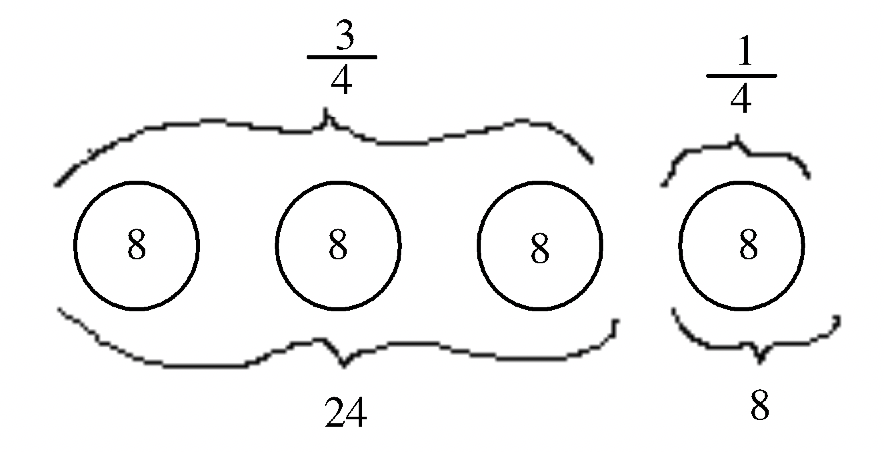The picture in Step 3 is what the final picture looks like.

Here is another example. 14 teachers were absent one day. This represents 2/11 of the teachers working at the school. How many teachers work at the school? Since 11 equal parts make up a whole, and 2 of those parts represent 14, then each of the 11 equal parts represents 7 teachers. So, 11 of the equal parts represents 77 teachers.

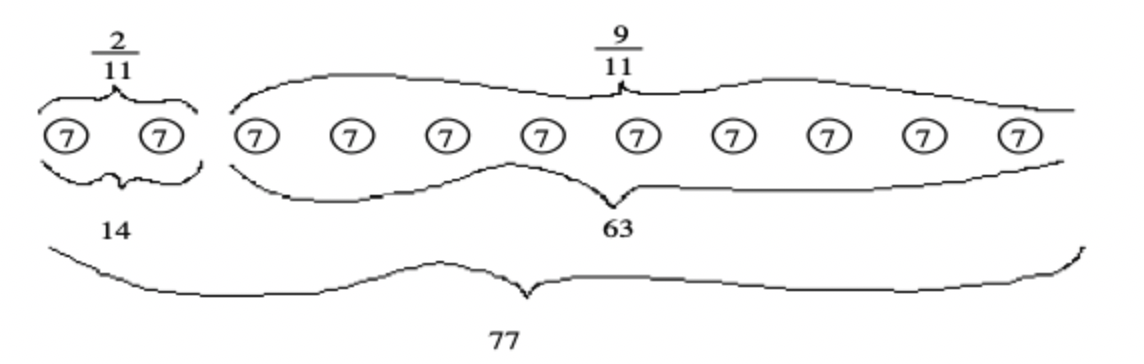
Here is one more example:

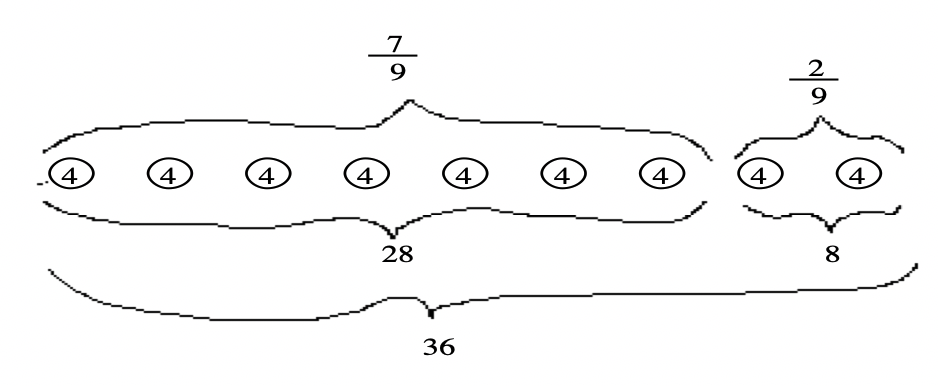
One day, 7/9 of the people at a local business came to work. 36 people worked there. How many people came to work?

First, since 9 equal parts make up a whole, draw 9 circles. You know 7 of those represent 7/9 and 2 of those represent 2/9. You also know, the total equals 36, which means 4 goes into each equal part. Therefore, 28 came to work, and 8 did not. Here is the picture:

For each of the following word problems, draw model similar to the previous two examples to solve the problem. You may want to use fraction circles as well.

Exercise 48

66 students in my class passed the first test. That represents 11/12 of my students. How many students did not pass?

Exercise 49

36 students in first grade brought a lunchbox to school one day. This was 3/7 of the first graders. How many did not buy lunch?

Exercise 50

I have 120 students this semester. 5/8 of my students are female. How many are female and how many are male?

Exercise 51

Make up a word problem to solve using fractions. Make sure you ask a question. Then solve the problem using models. Explain how the model works.

Write the word problem here:

Solve the problem using models here: