1.1: Introduction to concept of a limit
- Page ID
- 11015
This page is a draft and is under active development.
Learning Objectives
- Using correct notation, describe the limit of a function.
- Use a table of values to estimate the limit of a function or to identify when the limit does not exist.
- Use a graph to estimate the limit of a function or to identify when the limit does not exist.
- Define one-sided limits and provide examples.
- Explain the relationship between one-sided and two-sided limits.
- Using correct notation, describe an infinite limit.
- Define a vertical asymptote.
Limits
Two key problems led to the initial formulation of calculus:
(1) the tangent problem, or how to determine the slope of a line tangent to a curve at a point;
and (2) the area problem, or how to determine the area under a curve.
The concept of a limit or limiting process, essential to the understanding of calculus, has been around for thousands of years. In fact, early mathematicians used a limiting process to obtain better and better approximations of areas of circles. Yet, the formal definition of a limit—as we know and understand it today—did not appear until the late 19th century. We, therefore, begin our quest to understand limits, as our mathematical ancestors did, by using an intuitive approach.
We begin our exploration of limits by taking a look at the graphs of the functions
- \(f(x)=\dfrac{x^2−4}{x−2}\),
- \(g(x)=\dfrac{|x−2|}{x−2}\), and
- \(h(x)=\dfrac{1}{(x−2)^2}\),
which are shown in Figure \(\PageIndex{1}\). In particular, let’s focus our attention on the behavior of each graph at and around \(x=2\).
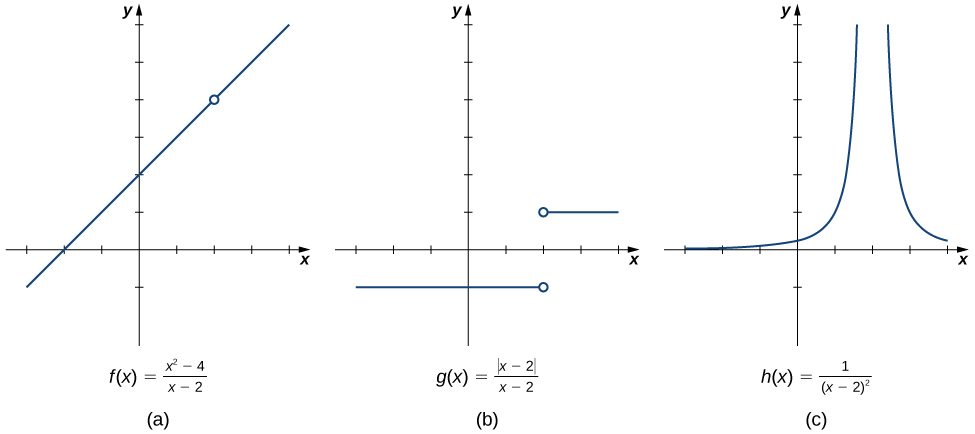
Each of the three functions is undefined at \(x=2\), but if we make this statement and no other, we give a very incomplete picture of how each function behaves in the vicinity of \(x=2\). To express the behavior of each graph in the vicinity of \(2\) more completely, we need to introduce the concept of a limit.
Intuitive Definition of a Limit
Let’s first take a closer look at how the function \(f(x)=(x^2−4)/(x−2)\) behaves around \(x=2\) in Figure \(\PageIndex{1}\). As the values of \(x\) approach \(2\) from either side of \(2\), the values of \(y=f(x)\) approach \(4\). Mathematically, we say that the limit of \(f(x)\) as \(x\) approaches \(2\) is \(4\). Symbolically, we express this limit as
\(\displaystyle \lim_{x \to 2} f(x)=4\).
From this very brief informal look at one limit, let’s start to develop an intuitive definition of the limit. We can think of the limit of a function at a number a as being the one real number \(L\) that the functional values approach as the \(x\)-values approach a, provided such a real number \(L\) exists. Stated more carefully, we have the following definition:
Definition(Intuitive): Limit
Let \(f(x)\) be a function defined at all values in an open interval containing \(a\), with the possible exception of a itself, and let \(L\) be a real number. If all values of the function \(f(x)\) approach the real number \(L\) as the values of \(x(≠a)\) approach the number a, then we say that the limit of \(f(x)\) as \(x\) approaches \(a\) is \(L\). (More succinct, as \(x\) gets closer to \(a\), \(f(x)\) gets closer and stays close to \(L\).) Symbolically, we express this idea as
\[\lim_{x \to a} f(x)=L.\]
We can estimate limits by constructing tables of functional values and by looking at their graphs. This process is described in the following Problem-Solving Strategy.
Problem-Solving Strategy: Evaluating a Limit Using a Table of Functional Values
1. To evaluate \(\displaystyle \lim_{x \to a} f(x)\), we begin by completing a table of functional values. We should choose two sets of \(x\)-values—one set of values approaching \(a\) and less than \(a\), and another set of values approaching \(a\) and greater than \(a\). Table \(\PageIndex{1}\) demonstrates what your tables might look like.
| \(x\) | \(f(x)\) | \(x\) | \(f(x)\) |
|---|---|---|---|
| \(a-0.1\) | \(f(a-0.1)\) | \(a+0.1\) | \(f(a+0.1)\) |
| \(a-0.01\) | \(f(a-0.01)\) | \(a+0.001\) | \(f(a+0.001)\) |
| \(a-0.001\) | \(f(a-0.001)\) | \(a+0.0001\) | \(f(a+0.001)\) |
| \(a-0.0001\) | \(f(a-0.0001)\) | \(a+0.00001\) | \(f(a+0.0001)\) |
| Use additional values as necessary. | Use additional values as necessary. | ||
2. Next, let’s look at the values in each of the \(f(x)\) columns and determine whether the values seem to be approaching a single value as we move down each column. In our columns, we look at the sequence \(f(a−0.1)\), \(f(a−0.01)\), \(f(a−0.001)\), \(f(a−0.0001)\), and so on, and \(f(a+0.1), \;f(a+0.01), \;f(a+0.001), \;f(a+0.0001)\), and so on. (Note: Although we have chosen the \(x\)-values \(a±0.1, \;a±0.01, \;a±0.001, \;a±0.0001\), and so forth, and these values will probably work nearly every time, on very rare occasions we may need to modify our choices.)
3. If both columns approach a common \(y\)-value \(L\), we state \(\displaystyle \lim_{x \to a}f(x)=L\). We can use the following strategy to confirm the result obtained from the table or as an alternative method for estimating a limit.
4. Using a graphing calculator or computer software that allows us graph functions, we can plot the function \(f(x)\), making sure the functional values of \(f(x)\) for \(x\)-values near a are in our window. We can use the trace feature to move along the graph of the function and watch the \(y\)-value readout as the \(x\)-values approach a. If the \(y\)-values approach \(L\) as our \(x\)-values approach \(a\) from both directions, then \(\displaystyle \lim_{x \to a}f(x)=L\). We may need to zoom in on our graph and repeat this process several times.
We apply this Problem-Solving Strategy to compute a limit in Examples \(\PageIndex{1A}\) and \(\PageIndex{1B}\).
Example \(\PageIndex{1A}\): Evaluating a Limit Using a Table of Functional Values
Evaluate \(\displaystyle \lim_{x \to 0}\frac{\sin x}{x}\) using a table of functional values.
Solution
We have calculated the values of \(f(x)=\dfrac{\sin x}{x}\) for the values of \(x\) listed in Table \(\PageIndex{2}\).
| \(x\) | \(\frac{\sin x}{x}\) | \(x\) | \(\frac{\sin x}{x}\) |
|---|---|---|---|
| -0.1 | 0.998334166468 | 0.1 | 0.998334166468 |
| -0.01 | 0.999983333417 | 0.01 | 0.999983333417 |
| -0.001 | 0.999999833333 | 0.001 | 0.999999833333 |
| -0.0001 | 0.999999998333 | 0.0001 | 0.999999998333 |
Note: The values in this table were obtained using a calculator and using all the places given in the calculator output.
As we read down each \(\dfrac{\sin x}{x}\) column, we see that the values in each column appear to be approaching one. Thus, it is fairly reasonable to conclude that \(\displaystyle \lim_{x\to0}\frac{\sin x}{x}=1\). A calculator-or computer-generated graph of \(f(x)=\dfrac{\sin x}{x}\) would be similar to that shown in Figure \(\PageIndex{2}\), and it confirms our estimate.
![A graph of f(x) = sin(x)/x over the interval [-6, 6]. The curving function has a y intercept at x=0 and x intercepts at y=pi and y=-pi.](https://math.libretexts.org/@api/deki/files/7963/imageedit_1_8651812985.png?revision=1)
Example \(\PageIndex{1B}\): Evaluating a Limit Using a Table of Functional Values
Evaluate \(\displaystyle \lim_{x\to4}\frac{\sqrt{x}−2}{x−4}\) using a table of functional values.
Solution
As before, we use a table—in this case, Table \(\PageIndex{3}\)—to list the values of the function for the given values of \(x\).
| \(x\) | \(\frac{\sqrt{x}−2}{x−4}\) | \(x\) | \(\frac{\sqrt{x}−2}{x−4}\) |
|---|---|---|---|
| 3.9 | 0.251582341869 | 4.1 | 0.248456731317 |
| 3.99 | 0.25015644562 | 4.01 | 0.24984394501 |
| 3.999 | 0.250015627 | 4.001 | 0.249984377 |
| 3.9999 | 0.250001563 | 4.0001 | 0.249998438 |
| 3.99999 | 0.25000016 | 4.00001 | 0.24999984 |
After inspecting this table, we see that the functional values less than 4 appear to be decreasing toward 0.25 whereas the functional values greater than 4 appear to be increasing toward 0.25. We conclude that \(\displaystyle \lim_{x\to4}\frac{\sqrt{x}−2}{x−4}=0.25\). We confirm this estimate using the graph of \(f(x)=\dfrac{\sqrt{x}−2}{x−4}\) shown in Figure \(\PageIndex{3}\).
![A graph of the function f(x) = (sqrt(x) – 2 ) / (x-4) over the interval [0,8]. There is an open circle on the function at x=4. The function curves asymptotically towards the x axis and y axis in quadrant one.](https://math.libretexts.org/@api/deki/files/7964/imageedit_5_9266726966.png?revision=1)
Exercise \(\PageIndex{1}\)
Estimate \(\displaystyle \lim_{x \to 1} \frac{\frac{1}{x}−1}{x−1}\) using a table of functional values. Use a graph to confirm your estimate.
- Hint
-
Use 0.9, 0.99, 0.999, 0.9999, 0.99999 and 1.1, 1.01, 1.001, 1.0001, 1.00001 as your table values.
- Answer
-
\[\lim_{x\to1}\frac{\frac{1}{x}−1}{x−1}=−1\nonumber\]
At this point, we see from Examples \(\PageIndex{1A}\) and \(\PageIndex{1b}\) that it may be just as easy, if not easier, to estimate a limit of a function by inspecting its graph as it is to estimate the limit by using a table of functional values. In Example \(\PageIndex{2}\), we evaluate a limit exclusively by looking at a graph rather than by using a table of functional values.
Example \(\PageIndex{2}\): Evaluating a Limit Using a Graph
For \(g(x)\) shown in Figure \(\PageIndex{4}\), evaluate \(\displaystyle \lim_{x\to−1}g(x)\).
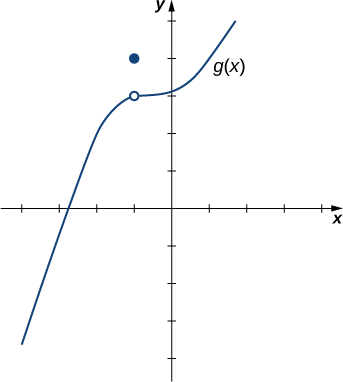
Solution:
Despite the fact that \(g(−1)=4\), as the \(x\)-values approach \(−1\) from either side, the \(g(x)\) values approach \(3\). Therefore, \(\displaystyle \lim_{x\to−1}g(x)=3\). Note that we can determine this limit without even knowing the algebraic expression of the function.
Based on Example \(\PageIndex{2}\), we make the following observation: It is possible for the limit of a function to exist at a point, and for the function to be defined at this point, but the limit of the function and the value of the function at the point may be different.
Exercise \(\PageIndex{2}\)
Use the graph of \(h(x)\) in Figure \(\PageIndex{5}\) to evaluate \(\displaystyle \lim_{x \to 2}h(x)\), if possible.
![A graph of the function h(x), which is a parabola graphed over [-2.5, 5]. There is an open circle where the vertex should be at the point (2,-1).](https://math.libretexts.org/@api/deki/files/7966/imageedit_13_2727890618.png?revision=1)
- Hint
-
What \(y\)-value does the function approach as the \(x\)-values approach \(2\)?
- Solution
-
\(\displaystyle \lim_{x \to 2}h(x)=−1.\)
Looking at a table of functional values or looking at the graph of a function provides us with useful insight into the value of the limit of a function at a given point. However, these techniques rely too much on guesswork. We eventually need to develop alternative methods of evaluating limits. These new methods are more algebraic in nature and we explore them in the next section; however, at this point we introduce two special limits that are foundational to the techniques to come.
Two Important Limits
Let \(a\) be a real number and \(c\) be a constant.
- \(\displaystyle \lim_{x \to a}x=a\)
- \(\displaystyle \lim_{x \to a}c=c\)
We can make the following observations about these two limits.
- For the first limit, observe that as \(x\) approaches \(a\), so does \(f(x)\), because \(f(x)=x\). Consequently, \(\displaystyle \lim_{x \to a}x=a\).
- For the second limit, consider Table \(\PageIndex{4}\).
| \(x\) | \(f(x)=c\) | \(x\) | \(f(x)=c\) |
|---|---|---|---|
| \(a-0.1\) | \(c\) | \(a+0.1\) | \(c\) |
| \(a-0.01\) | \(c\) | \(a+0.01\) | \(c\) |
| \(a-0.001\) | \(c\) | \(a+0.001\) | \(c\) |
| \(a-0.0001\) | \(c\) | \(a+0.0001\) | \(c\) |
Observe that for all values of \(x\) (regardless of whether they are approaching \(a\)), the values \(f(x)\) remain constant at \(c\). We have no choice but to conclude \(\displaystyle \lim_{x \to a}c=c\).
The Existence of a Limit
As we consider the limit in the next example, keep in mind that for the limit of a function to exist at a point, the functional values must approach a single real-number value at that point. If the functional values do not approach a single value, then the limit does not exist.
Example \(\PageIndex{3}\): Evaluating a Limit That Fails to Exist
Evaluate \(\displaystyle\lim_{x \to 0}\sin(1/x)\) using a table of values.
Solution
Table \(\PageIndex{5}\) lists values for the function \(\sin(1/x)\) for the given values of \(x\).
| \(x\) | \(\sin(1/x)\) | \(x\) | \(\sin(1/x)\) |
|---|---|---|---|
| -0.1 | 0.544021110889 | 0.1 | −0.544021110889 |
| -0.01 | 0.50636564111 | 0.01 | −0.50636564111 |
| -0.001 | −0.8268795405312 | 0.001 | 0.8268795405312 |
| -0.0001 | 0.305614388888 | 0.0001 | −0.305614388888 |
| -0.00001 | −0.035748797987 | 0.00001 | 0.035748797987 |
| -0.000001 | 0.349993504187 | 0.000001 | −0.349993504187 |
After examining the table of functional values, we can see that the \(y\)-values do not seem to approach any one single value. It appears the limit does not exist. Before drawing this conclusion, let’s take a more systematic approach. Take the following sequence of \(x\)-values approaching \(0\):
\[\frac{2}{π},\;\frac{2}{3π},\;\frac{2}{5π},\;\frac{2}{7π},\;\frac{2}{9π},\;\frac{2}{11π},\;….\nonumber\]
The corresponding \(y\)-values are
\[1,\;-1,\;1,\;-1,\;1,\;-1,\;....\nonumber\]
At this point we can indeed conclude that \(\displaystyle \lim_{x \to 0} \sin(1/x)\) does not exist. (Mathematicians frequently abbreviate “does not exist” as DNE. Thus, we would write \(\displaystyle \lim_{x \to 0} \sin(1/x)\) DNE.) The graph of \(f(x)=\sin(1/x)\) is shown in Figure \(\PageIndex{6}\) and it gives a clearer picture of the behavior of \(\sin(1/x)\) as \(x\) approaches \(0\). You can see that \(\sin(1/x)\) oscillates ever more wildly between \(−1\) and \(1\) as \(x\) approaches \(0\).
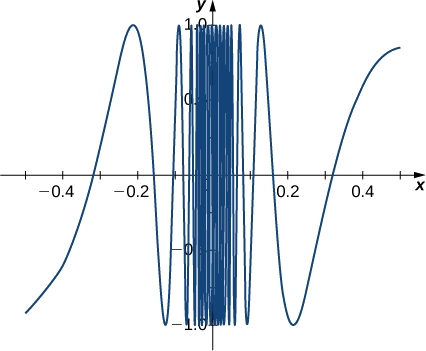
Exercise \(\PageIndex{3}\)
Use a table of functional values to evaluate \(\displaystyle \lim_{x \to 2}\frac{∣x^2−4∣}{x−2}\), if possible.
- Hint
-
Use \(x\)-values 1.9, 1.99, 1.999, 1.9999, 1.9999 and 2.1, 2.01, 2.001, 2.0001, 2.00001 in your table.
- Answer
-
\(\displaystyle \lim_{x \to 2}\frac{∣x^2−4∣}{x−2}\) does not exist.
Contributors and Attributions
Gilbert Strang (MIT) and Edwin “Jed” Herman (Harvey Mudd) with many contributing authors. This content by OpenStax is licensed with a CC-BY-SA-NC 4.0 license. Download for free at http://cnx.org.
Pamini Thangarajah (Mount Royal University, Calgary, Alberta, Canada)

