10.2: Bessel’s Equation

Last updated
Save as PDF

Page ID: 8332

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $ $ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $$\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$ $\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$$\newcommand{\AA}{\unicode[.8,0]{x212B}}$

Bessel’s equation of order $\nu$ is given by \[x^2 y'' + x y' + (x^2-\nu^2) y = 0. \nonumber \] Clearly $x=0$ is a regular singular point, so we can solve by Frobenius’ method. The indicial equation is obtained from the lowest power after the substitution $y=x^\gamma$, and is

\[\gamma^2-\nu^2=0 \nonumber \]

So a generalized series solution gives two independent solutions if $\nu \neq \frac{1}{2} n$. Now let us solve the problem and explicitly substitute the power series,

\[y = x^\nu \sum_n a_n x^n. \nonumber \]

From Bessel’s equation we find

\[\sum_n(n+\nu)(n+\nu-1) a_\nu x^{m+\nu} +\sum_n(n+\nu)a_\nu x^{m+\nu} +\sum_n(x^2-\nu^2)a_\nu = 0 \nonumber \]

which leads to

\[[(m+\nu)^2-\nu^2] a_m= -a_{m-2} \nonumber \] or \[a_m= -\frac{1}{m(m+2\nu)}a_{m-2}. \nonumber \]

If we take $\nu=n>0$, we have

\[a_m= -\frac{1}{m(m+2n)}a_{m-2}. \nonumber \]

This can be solved by iteration,

\[\begin{aligned} a_{2k} &= -\frac{1}{4}\frac{1}{k(k+n)}a_{2(k-1)}\nonumber\\ &= \left(\frac{1}{4}\right)^2\frac{1}{k(k-1)(k+n)(k+n-1)}a_{2(k-2)} \nonumber\\ &= \left(-\frac{1}{4}\right)^k\frac{n!}{k!(k+n)!}a_{0}.\end{aligned} \nonumber \]

If we choose¹ $a_0 = \frac{1}{n!2^n}$ we find the Bessel function of order $n$

\[J_n(x) = \sum_{k=0}^\infty \frac{(-1)^k}{k!(k+n)!} \left(\frac{x}{2}\right)^{2k+n}. \nonumber \]

There is another second independent solution (which should have a logarithm in it) with goes to infinity at $x=0$.

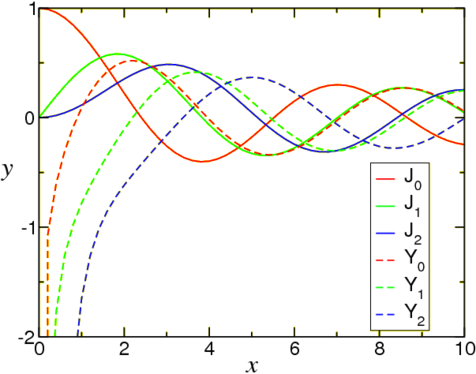
$A plot of the first three Bessel functions $J_n$ and $Y_n$.$

Figure $\PageIndex{1}$: A plot of the first three Bessel functions $J_n$ and $Y_n$.

The general solution of Bessel’s equation of order $n$ is a linear combination of $J$ and $Y$, \[y(x) = A J_n(x)+B Y_n(x). \nonumber \]

This can be done since Bessel’s equation is linear, i.e., if $g(x)$ is a solution $C g(x)$ is also a solution.↩