7.6.E: Problems on Measures and Outer Measures
- Last updated
- Save as PDF
- Page ID
- 32351
Exercise \(\PageIndex{1}\)
Show that formulas (1) and (2) are equivalent.
[Hints: (i) Assume (1) and let \(X \subseteq A, Y \subseteq-A .\)
As \(X\) in (1) is arbitrary, we may replace it by \(X \cup Y.\) Simplifying, obtain (2) on noting that \(X \cap A=X, X \cap-A=\emptyset, Y \cap A=\emptyset,\) and \(Y \cap-A=Y\).
(ii) Assume (2). Take any \(X\) and substitute \(X \cap A\) and \(X-A\) for \(X\) and \(Y\) in (2).]
Exercise \(\PageIndex{2}\)
Given an outer measure space \(\left(S, \mathcal{M}^{*}, m^{*}\right)\) and \(A \subseteq S,\) set
\[A \cap \mathcal{M}^{*}=\left\{A \cap X | X \in \mathcal{M}^{*}\right\}\] (SYMBOL!)
(all sets of the form \(A \cap X\) with \(X \in \mathcal{M}^{*}\)).
Prove that \(A \cap \mathcal{M}^{*}\) is a \(\sigma\)-field in \(A,\) and \(m^{*}\) is \(\sigma\)-additive on it. (SYMBOL!)
[Hint: Use Lemma 4, with \(X_{k}=A \cap A_{k} \in A \cap \mathcal{M}^{*}\).] (SYMBOL!)
Exercise \(\PageIndex{3}\)
Prove Lemmas 1 and 2, using formula (1).
Exercise \(\PageIndex{3'}\)
Prove Corollary 1.
Exercise \(\PageIndex{4}\)
Verify Examples (b),(c), and (d). Why is \(m\) an outer measure as well?
[Hint: Use Corollary 2 in §5.]
Exercise \(\PageIndex{5}\)
Fill in all details (induction, etc.) in the proofs of this section.
Exercise \(\PageIndex{6}\)
Verify that \(m^{*}\) is an outer measure and describe \(\mathcal{M}^{*}\) under each of the following conditions.
(a) \(m^{*} A=1\) if \(\emptyset \subset A \subseteq S; m^{*} \emptyset=0\).
(b) \(m^{*} A=1\) if \(\emptyset \subset A \subset S; m^{*} S=2; m^{*} \emptyset=0\).
(c) \(m^{*} A=0\) if \(A \subseteq S\) is countable; \(m^{*} A=1\) otherwise (\(S\) is uncountable).
(d) \(S=N\) (naturals); \(m^{*} A=1\) if \(A\) is infinite; \(m^{*} A=\frac{n}{n+1}\) if \(A\) has \(n\) elements.
Exercise \(\PageIndex{7}\)
Prove the following.
(i) An outer measure \(m^{*}\) is \(\mathcal{M}^{*}\)-regular (Definition 5 in §5) iff
\[(\forall A \subseteq S)\left(\exists B \in \mathcal{M}^{*}\right) \quad A \subseteq B \text { and } m^{*} A=m B.\]
\(B\) is called a measurable cover of \(A\).
[Hint: If
\[m^{*} A=\inf \left\{m X | A \subseteq X \in \mathcal{M}^{*}\right\},\]
then
\[(\forall n)\left(\exists X_{n} \in \mathcal{M}^{*}\right) \quad A \subseteq X_{n} \text { and } m X_{n} \leq m^{*} A+\frac{1}{n}.\]
Set \(B=\bigcap_{n=1}^{\infty} X_{n}\).]
(ii) If \(m^{*}\) is as in Definition 3 of §5, with \(\mathcal{C} \subseteq \mathcal{M}^{*},\) then \(m^{*}\) is \(\mathcal{M}^{*}\)-regular.
Exercise \(\PageIndex{8}\)
Show that if \(m^{*}\) is \(\mathcal{M}^{*}\)-regular (Problem 7), it is left continuous.
[Hints: Let \(\left\{A_{n}\right\} \uparrow;\) let \(B_{n}\) be a measurable cover of \(A_{n};\) set
\[C_{n}=\bigcap_{k=n}^{\infty} B_{k}.\]
Verify that \(\left\{C_{n}\right\} \uparrow, B_{n} \supseteq C_{n} \supseteq A_{n},\) and \(m C_{n}=m^{*} A_{n}\).
By the left continuity of \(m\) (Theorem 2 in §4),
\[\lim m^{*} A_{n}=\lim m C_{n}=m \bigcup_{n=1}^{\infty} C_{n} \geq m^{*} \bigcup_{n=1}^{\infty} A_{n}.\]
Prove the reverse inequality as well.]
Exercise \(\PageIndex{9}\)
Continuing Problems 6-8, verify the following.
(i) In 6(a), with \(S=N, m^{*}\) is \(\mathcal{M}^{*}\)-regular, but not right continuous.
Hint: Take \(A_{n}=\{x \in N | x \geq n\}\).
(ii) In 6(b), with \(S=N, m^{*}\) is neither \(\mathcal{M}^{*}\)-regular nor left continuous.
(iii) In 6(d), \(m^{*}\) is not \(\mathcal{M}^{*}\)-regular; yet it is left continuous. (Thus Problem 8 is not a necessary condition.)
Exercise \(\PageIndex{10}\)
In Problem 2, let \(n^{*}\) be the restriction of \(m^{*}\) to \(2^{A}.\) Prove the following.
(a) \(n^{*}\) is an outer measure in \(A\).
(b) \(A \cap \mathcal{M}^{*} \subseteq \mathcal{N}^{*}=\left\{n^{*} \text {-measurable sets}\right\}\). (SYMBOL!)
(c) \(A \cap \mathcal{M}^{*}=\mathcal{N}^{*}\) if \(A \in \mathcal{M}^{*},\) or if \(m^{*}\) is \(\mathcal{M}^{*}\)-regular (see Problem 7) and finite. (SYMBOL!)
(d) \(n^{*}\) is \(\mathcal{N}^{*}\)-regular if \(m^{*}\) is \(\mathcal{M}^{*}\)-regular.
Exercise \(\PageIndex{11}\)
Show that if \(m^{*}\) is \(\mathcal{M}^{*}\)-regular and finite, then \(A \subseteq S\) is \(m^{*}\)-measurable iff
\[m S=m^{*} A+m^{*}(-A).\]
[Hint: Assume the latter. By Problem 7,
\[(\forall X \subseteq S)\left(\exists B \in \mathcal{M}^{*}, B \supseteq X\right) \quad m^{*} X=m B;\]
so
\[m^{*} A=m^{*}(A \cap B)+m^{*}(A-B).\]
Similarly for \(-A.\) Deduce that
\[m^{*}(A \cap B)+m^{*}(A-B)+m^{*}(B-A)+m^{*}(-A-B)=m S=m B+m(-B);\]
hence
\[m^{*} X=m B \geq m^{*}(B \cap A)+m^{*}(B-A) \geq m^{*}(X \cap A)+m^{*}(X-A),\]
so \(A \in \mathcal{M}^{*}\).]
Exercise \(\PageIndex{12}\)
Using Problem 15 in §5, prove that if \(m^{*}\) has the CP then each open set \(G \subseteq S\) is in \(\mathcal{M}^{*}\).
[Outline: Show that
\[(\forall X \subseteq G)(\forall Y \subseteq-G) \quad m^{*}(X \cup Y) \geq m^{*} X+m^{*} Y,\]
assuming \(m^{*} X<\infty.\) (Why?) Set
\[D_{0}=\{x \in X | \rho(x,-G) \geq 1\}\]
and
\[D_{k}=\left\{x \in X | \frac{1}{k+1} \leq \rho(x,-G)<\frac{1}{k}\right\}, \quad k \geq 1.\]
Prove that
\[X=\bigcup_{k=0}^{\infty} D_{k}\]
and
\[\rho\left(D_{k}, D_{k+2}\right)>0;\]
so by Problem 15 in §5,
\[\sum_{n=0}^{\infty} m^{*} D_{2 n}=m^{*} \bigcup_{n=0}^{\infty} D_{2 n} \leq m^{*} \bigcup_{n=0}^{\infty} D_{n}=m^{*} X<\infty.\]
Similarly,
\[\sum_{n=0}^{\infty} m^{*} D_{2 n+1} \leq m^{*} X<\infty.\]
Hence
\[\sum_{n=0}^{\infty} m^{*} D_{n}<\infty;\]
so
\[\lim _{n \rightarrow \infty} \sum_{k=n}^{\infty} m^{*} D_{k}=0.\]
(Why?) Thus
\[(\forall \varepsilon>0)(\exists n) \sum_{k=n}^{\infty} m^{*} D_{k}<\varepsilon.\]
Also,
\[X=\bigcup_{k=0}^{\infty} D_{k}=\bigcup_{k=0}^{n-1} D_{k} \cup \bigcup_{k=n}^{\infty} D_{k};\]
so
\[m^{*} X \leq m^{*} \bigcup_{k=0}^{n-1} D_{k}+\sum_{k=n}^{\infty} m^{*} D_{k}<m^{*} \bigcup_{k=0}^{n-1} D_{k}+\varepsilon.\]
Adding \(m^{*} Y\) on both sides, get
\[m^{*} X+m^{*} Y \leq m^{*} \bigcup_{k=0}^{n-1} D_{k}+m^{*} Y+\varepsilon.\]
Moreover,
\[\rho\left(\bigcup_{k=0}^{n-1} D_{k}, Y\right)>0,\]
for \(Y \subseteq-G\) and
\[\rho\left(D_{k},-G\right) \geq \frac{1}{k+1}.\]
Hence by the CP,
\[m^{*} Y+\sum_{k=0}^{n-1} m^{*} D_{k}=m^{*}\left(Y \cup \bigcup_{k=0}^{n-1} D_{k}\right)<m^{*}(Y \cup X).\]
(Why?) Combining with (iii), obtain
\[m^{*} X+m^{*} Y \leq m^{*}(X \cup Y)+\varepsilon.\]
Now let \(\varepsilon \rightarrow 0\).]
Exercise \(\PageIndex{13}\)
\(\Rightarrow\) Show that if \(m : \mathcal{M} \rightarrow E^{*}\) is a measure, there is \(P \in \mathcal{M},\) with
\[m P=\max \{m X | X \in \mathcal{M}\}.\]
[Hint: Let
\[k=\sup \{m X | X \in \mathcal{M}\}\]
in \(E^{*}.\) As \(k \geq 0,\) there is a sequence \(r_{n} \nearrow k, r_{n}<k.\) (If \(k=\infty,\) set \(r_{n}=n;\) if \(\left.k<\infty, r_{n}=k-\frac{1}{n}.\right)\) By lub properties,
\[(\forall n)\left(\exists X_{n} \in \mathcal{M}\right) \quad r_{n}<m X_{n} \leq k,\]
with \(\left\{X_{n}\right\} \uparrow\) (Problem 9 in §3). Set
\[P=\bigcup_{n=1}^{\infty} X_{n}.\]
Show that
\[m P=\lim _{n \rightarrow \infty} m X_{n}=k.]\]
Exercise \(\PageIndex{14}\)
\(\Rightarrow^{*}\) Given a measure \(m : \mathcal{M} \rightarrow E^{*},\) let
\[\overline{\mathcal{M}}=\{\text {all sets of the form } X \cup Z \text { where } X \in \mathcal{M} \text { and } Z \text { is } m \text{-null}\}.\]
Prove that \(\overline{\mathcal{M}}\) is a \(\sigma\)-ring \(\supseteq \mathcal{M}\).
[Hint: To prove that
\[(\forall A, B \in \overline{\mathcal{M}}) \quad A-B \in \overline{\mathcal{M}},\]
suppose first \(A \in \mathcal{M}\) and \(B\) is "null," i.e., \(B \subseteq U \in \mathcal{M}, m U=0\).
Show that
\[A-B=X \cup Z,\]
with \(X=A-U \in \mathcal{M}\) and \(Z=A \cap U-B m\)-null (\(Z\) is shaded in Figure 31).
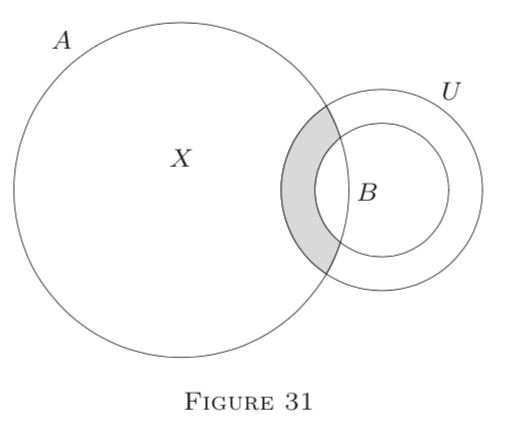
Next, if \(A, B \in \overline{\mathcal{M}},\) let \(A=X \cup Z\) \(B=X^{\prime} \cup Z^{\prime},\) where \(X, X^{\prime} \in \mathcal{M}\) and \(Z, Z^{\prime}\) are \(m\)-null. Hence
\[\begin{aligned} A-B &=(X \cup Z)-B \\ &=(X-B) \cup(Z-B) \\ &=(X-B) \cup Z^{\prime \prime}, \end{aligned}\]
where
\[Z^{\prime \prime}=Z-B\]
is \(m\)-null. Also, \(B=X^{\prime} \cup Z^{\prime}\) implies
\[X-B=\left(X-X^{\prime}\right)-Z^{\prime} \in \overline{\mathcal{M}},\]
by the first part of the proof.
Deduce that
\[A-B=(X-B) \cup Z^{\prime \prime} \in \overline{\mathcal{M}}\]
(after checking closure under unions).]
Exercise \(\PageIndex{15}\)
\(\Rightarrow^{*}\) Continuing Problem 14, define \(\overline{m} : \overline{\mathcal{M}} \rightarrow E^{*}\) by setting \(\overline{m} A=m X\) whenever \(A=X \cup Z,\) with \(X \in \mathcal{M}\) and \(Z\) \(m\)-null. (Show that \(\overline{m} A\) does not depend on the particular representation of \(A\) as \(X \cup Z\).)
Prove the following.
(i) \(\overline{m}\) is a complete measure (called the completion of \(m\)), with \(\overline{m}=m\) on \(\mathcal{M}.\)
(ii) \(\overline{m}\) is the least complete extension of \(m;\) that is, if \(n : \mathcal{N} \rightarrow E^{*}\) is another complete measure, with \(\mathcal{M} \subseteq \mathcal{N}\) and \(n=m\) on \(\mathcal{M},\) then \(\overline{\mathcal{M}} \subseteq \mathcal{N}\) and \(n=\overline{m}\) on \(\overline{\mathcal{M}}.\)
(iii) \(m=\overline{m}\) iff \(m\) is complete.
Exercise \(\PageIndex{16*}\)
Show that if \(m : \mathcal{M}^{*} \rightarrow E^{*}\) is induced by an \(\mathcal{M}^{*}\)-regular outer measure \(\mu^{*},\) then \(m\) equals its Lebesgue extension \(m^{\prime}\) and completion \(\overline{m}\) (see Problem 15).
[Hint: By Definition 3 in §5, \(m\) induces an outer measure \(m^{*}.\) By Theorem 3 in §5,
\[m^{*} A=\inf \left\{m X | A \subseteq X \in \mathcal{M}^{*}\right\}=\mu^{*} A\]
(for \(\mu^{*}\) is \(\mathcal{M}^{*}\)-regular).
As \(m^{*}=\mu^{*},\) we get \(m^{\prime}=m.\) Also, \(m=\overline{m},\) by Problem 15(iii).]
Exercise \(\PageIndex{17*}\)
Prove that if a measure \(\mu : \mathcal{M} \rightarrow E^{*}\) is \(\sigma\)-finite (Definition 4 in §5), with \(S \in \mathcal{M},\) then its Lebesgue extension \(m : \mathcal{M}^{*} \rightarrow E^{*}\) equals its completion \(\overline{\mu}\) (see Problem 15).
[Outline: It suffices to prove \(\mathcal{M}^{*} \subseteq \overline{\mathcal{M}}.\) (Why?)
To start with, let \(A \in \mathcal{M}^{*}, m A<\infty.\) By Problem 12 in §5,
\[(\exists B \in \mathcal{M}) \quad A \subseteq B \text { and } m^{*} A=m A=m B<\infty;\]
so
\[m(B-A)=m B-m A=0.\]
Also,
\[(\exists H \in \mathcal{M}) \quad B-A \subseteq H \text { and } \mu H=m(B-A)=0.\]
Thus \(B-A\) is \(\mu\)-null; so \(B-A \in \overline{\mathcal{M}}.\) (Why?) Deduce that
\[A=B-(B-A) \in \overline{\mathcal{M}}.\]
Thus \(\overline{\mathcal{M}}\) contains any \(A \in \mathcal{M}^{*}\) with \(m A<\infty.\) Use the \(\sigma\)-finiteness of \(\mu\) to show
\[\left.\left(\forall x \in \mathcal{M}^{*}\right)\left(\exists\left\{A_{n}\right\} \subseteq \mathcal{M}^{*}\right) \quad m A_{n}<\infty \text { and } X=\bigcup_{n} A_{n} \in \overline{\mathcal{M}}.\right]\]

