6.1: Inverse Trigonometric Functions

Last updated
Save as PDF

Page ID: 34924

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $ $ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $$\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$ $\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$$\newcommand{\AA}{\unicode[.8,0]{x212B}}$

Learning Objectives

Understand and use the inverse sine, cosine, and tangent functions.
Find the exact value of expressions involving the inverse sine, cosine, and tangent functions.
Use a calculator to evaluate inverse trigonometric functions.
Find exact values of composite functions with inverse trigonometric functions.

For any right triangle, given one other angle and the length of one side, we can figure out what the other angles and sides are. But what if we are given only two sides of a right triangle? We need a procedure that leads us from a ratio of sides to an angle. This is where the notion of an inverse to a trigonometric function comes into play. In this section, we will explore the inverse trigonometric functions.

Understanding and Using the Inverse Sine, Cosine, and Tangent Functions

In order to use inverse trigonometric functions, we need to understand that an inverse trigonometric function “undoes” what the original trigonometric function “does,” as is the case with any other function and its inverse. In other words, the domain of the inverse function is the range of the original function, and vice versa, as summarized in Figure $\PageIndex{1a}$.

A chart that says “Trig Functinos”, “Inverse Trig Functions”, “Domain: Measure of an angle”, “Domain: Ratio”, “Range: Ratio”, and “Range: Measure of an angle”. — Figure $\PageIndex{1a}$

For example, if $f(x)=\sin\space x$, then we would write $f^{−1}(x)={\sin}^{−1}x$. Be aware that ${\sin}^{−1}x$ does not mean $\dfrac{1}{\sin\space x}$. The following examples illustrate the inverse trigonometric functions:

Since $\sin\left(\dfrac{\pi}{6}\right)=\dfrac{1}{2}$, then $\dfrac{\pi}{6}={\sin}^{−1}\left(\dfrac{1}{2}\right)$.
Since $\cos(\pi)=−1$, then $\pi={\cos}^{−1}(−1)$.
Since $\tan\left (\dfrac{\pi}{4}\right )=1$, then $\dfrac{\pi}{4}={\tan}^{−1}(1)$.

In previous sections, we evaluated the trigonometric functions at various angles, but at times we need to know what angle would yield a specific sine, cosine, or tangent value. For this, we need inverse functions. Recall that, for a one-to-one function, if $f(a)=b$, then an inverse function would satisfy $f^{−1}(b)=a$.

Bear in mind that the sine, cosine, and tangent functions are not one-to-one functions. The graph of each function would fail the horizontal line test. In fact, no periodic function can be one-to-one because each output in its range corresponds to at least one input in every period, and there are an infinite number of periods. As with other functions that are not one-to-one, we will need to restrict the domain of each function to yield a new function that is one-to-one. We choose a domain for each function that includes the number 0. Figure $\PageIndex{1b}$ shows how the domains are restricted to obtain one-to-one functions.

屏幕快照 2019-07-09 上午4.16.33.png - Three side-by-side graphs. The first graph, graph A, shows half of a period of the function sine of x. The second graph, graph B, shows half a period of the function cosine of x. The third graph shows one period of tangent of x, from -pi/2 to pi/2. — Figure $\PageIndex{1b}$: Domain restrictions applied to sine, cosine and tangent in order to define inverse functions for these trigonometric functions.

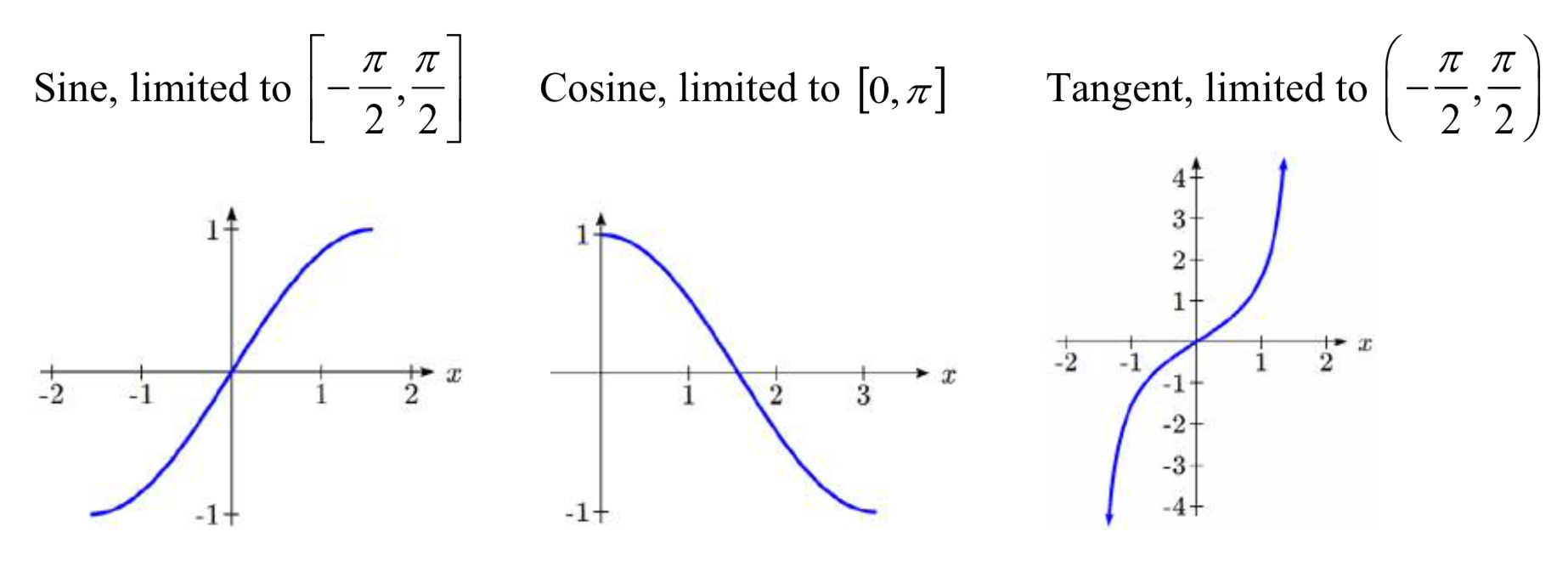
These conventional choices for the restricted domain are somewhat arbitrary, but they have important, helpful characteristics. Each domain includes the origin and some positive values, and most importantly, each results in a one-to-one function that is invertible. The conventional choice for the restricted domain of the tangent function also has the useful property that it extends from one vertical asymptote to the next instead of being divided into two parts by an asymptote.

On these restricted domains, we can define the inverse trigonometric functions.

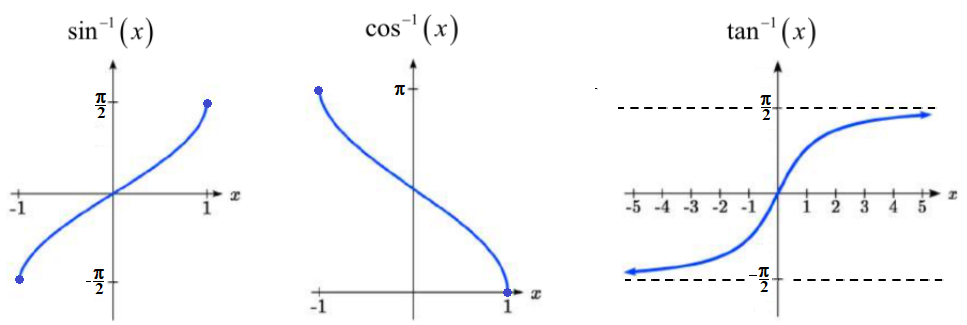
The inverse sine function $y={\sin}^{−1}x$ means $x=\sin\space y$. The inverse sine function is sometimes called the arcsine function, and notated $\arcsin\space x$.
$y={\sin}^{−1}x$ has domain $[−1,1]$ and range $\left[−\frac{\pi}{2},\frac{\pi}{2}\right]$
The inverse cosine function $y={\cos}^{−1}x$ means $x=\cos\space y$. The inverse cosine function is sometimes called the arccosine function, and notated $\arccos\space x$.
$y={\cos}^{−1}x$ has domain $[−1,1]$ and range $[0,π]$
The inverse tangent function $y={\tan}^{−1}x$ means $x=\tan\space y$. The inverse tangent function is sometimes called the arctangent function, and notated $\arctan\space x$.
$y={\tan}^{−1}x$ has domain $(−\infty,\infty)$ and range $\left(−\frac{\pi}{2},\frac{\pi}{2}\right)$

The graphs of the inverse functions are shown in Figure $\PageIndex{1c}$. Notice that the output of each of these inverse functions is an angle in radian measure. We see that ${\sin}^{−1}x$ has domain $[ −1,1 ]$ and range $\left[ −\dfrac{\pi}{2},\dfrac{\pi}{2} \right]$, ${\cos}^{−1}x$ has domain $[ −1,1 ]$ and range $[0,\pi]$, and ${\tan}^{−1}x$ has domain of all real numbers and range $\left(−\dfrac{\pi}{2},\dfrac{\pi}{2}\right)$. To find the domain and range of inverse trigonometric functions, switch the domain and range of the original domain restricted functions.

inverse trig graphs.png - inverse sine, inverse cosine and inverse tangent function graphs — Figure $\PageIndex{1c}$: The inverse sine , inverse cosine, and inverse tangent

Recall we previously defined a reference angle, often denoted $\alpha$, to be an angle between $0$ and $\dfrac{\pi}{2}$. Any defined inverse trigonometric function with a positive argument will return an angle that can be regarded as a reference angle in Quadrant I. If the argument is negative, the result will be an angle in standard position either in Quadrant IV ( $\sin^{-1}$ or $\tan^{-1}$ ) or in Quadrant II ($\cos^{-1}$). A summary of these definitions is below.

INVERSE SINE, COSINE, AND TANGENT FUNCTIONS

Given that the reference angle of $y$ is $ \alpha $,

\( \begin{array} {lclll}
{\sin}^{−1}x=y & \leftrightarrow & \sin y=x \text{,  } −\dfrac{\pi}{2} \le y \le \dfrac{\pi}{2} & \quad\text{ So } {\sin}^{−1}x =
{ \begin{cases}
\text{undefined} & \mbox{if } |x| > 1 & \text{ because } -1 \le \sin y \le 1 \\
\alpha & \text{if } x \ge 0, &  \alpha \text{ is in QI} \\
-\alpha & \mbox{if } x < 0, &   -\alpha \text{ is in QIV}\\
\end{cases}
} \\[4pt]
{\cos}^{−1}x=y & \leftrightarrow & \cos y=x \text{, }\;\;\;\;\; 0 \le y \le \pi & \quad\text{ So } {\cos}^{−1}x =
{ \begin{cases}
\text{undefined} & \mbox{if } |x| > 1 & \text{ because } -1 \le \cos y \le 1\\
\alpha & \mbox{if } x \ge 0, &  \alpha \text{ is in QI} \\
\pi -\alpha & \mbox{if } x < 0, &  \pi -\alpha  \text{ is in QII} \\
\end{cases}
} \\[4pt]
{\tan}^{−1}x=y & \leftrightarrow & \tan y=x \text{, } −\dfrac{\pi}{2} < y < \dfrac{\pi}{2} & \quad\text{ So } {\tan}^{−1}x =
{ \begin{cases}
\alpha \qquad \quad \;\; & \text{if } x \ge 0, &  \alpha \text{ is in QI} \\
-\alpha & \mbox{if } x < 0, & -\alpha \text{ is in QIV}\\
\end{cases}
}
\end{array} \)

Example $\PageIndex{1}$: Write a Trig Function in its Inverse Form

Given $\sin\left(\dfrac{5\pi}{12}\right)≈0.96593$, write a relation involving the inverse sine.

Solution

Use the relation for the inverse sine. If $\sin y=x$, then ${\sin}^{−1}x=y$.

In this problem, $x=0.96593$, and $y=\dfrac{5\pi}{12}$.

${\sin}^{−1}(0.96593)≈\dfrac{5\pi}{12}$

$try-it.png$ Try It $\PageIndex{1}$

Given $\cos(0.5)≈0.8776$, write a relation involving the inverse cosine.

Answer: $\arccos(0.8776)≈0.5$

Find Exact Special Angle Values for Inverse Sine, Cosine, and Tangent Functions

Now that we can identify inverse functions, we will learn to evaluate them. For most values in their domains, we must evaluate the inverse trigonometric functions by using a calculator, interpolating from a table, or using some other numerical technique. Just as we did with the original trigonometric functions, we can give exact values for the inverse functions when we are using the special angles, specifically $\dfrac{\pi}{6}$ (or 30°), $\dfrac{\pi}{4}$ (or 45°), and $\dfrac{\pi}{3}$ (or 60°), and their reflections into other quadrants.

Given a “special” input value, evaluate an inverse trigonometric function.

Find angle $x$ for which the original trigonometric function has an output equal to the given input for the inverse trigonometric function.
If $x$ is not in the defined range of the inverse, find another angle $y$ that is in the defined range and has the same sine, cosine, or tangent as $x$, depending on which corresponds to the given inverse function.

Example $\PageIndex{2}$: Evaluating Inverse Trigonometric Functions for Special Input Values

Evaluate each of the following.

${\sin}^{−1}\left(\dfrac{1}{2}\right)$

${\sin}^{−1}\left(−\dfrac{\sqrt{2}}{2}\right)$

${\cos}^{−1}\left(−\dfrac{\sqrt{3}}{2}\right)$

${\tan}^{−1}(1)$

Solution

Evaluating ${\sin}^{−1}\left(\dfrac{1}{2}\right)$ is the same as determining the angle that would have a sine value of $\dfrac{1}{2}$. In other words, what angle $x$ would satisfy $\sin(x)=\dfrac{1}{2}$? There are multiple values that would satisfy this relationship, such as $\dfrac{\pi}{6}$ and $\dfrac{5\pi}{6}$, but we know we need the angle in the interval $\left[ −\dfrac{\pi}{2},\dfrac{\pi}{2} \right]$, so the answer will be ${\sin}^{−1}\left (\dfrac{1}{2}\right)=\dfrac{\pi}{6}$. Remember that the inverse is a function, so for each input, we will get exactly one output.
To evaluate ${\sin}^{−1}\left(−\dfrac{\sqrt{2}}{2}\right)$, we know that $\dfrac{5\pi}{4}$ and $\dfrac{7\pi}{4}$ both have a sine value of $-\dfrac{\sqrt{2}}{2}$, but neither is in the interval $\left[ −\dfrac{\pi}{2},\dfrac{\pi}{2} \right]$. For that, we need the negative angle coterminal with $\dfrac{7\pi}{4}$: ${\sin}^{−1}\left(−\dfrac{\sqrt{2}}{2}\right)=−\dfrac{\pi}{4}$.
To evaluate ${\cos}^{−1}\left(−\dfrac{\sqrt{3}}{2}\right)$, we are looking for an angle in the interval $[ 0,\pi ]$ with a cosine value of $-\dfrac{\sqrt{3}}{2}$. The angle that satisfies this is ${\cos}^{−1}\left(−\dfrac{\sqrt{3}}{2}\right)=\dfrac{5\pi}{6}$.
Evaluating ${\tan}^{−1}(1)$, we are looking for an angle in the interval $\left(−\dfrac{\pi}{2},\dfrac{\pi}{2}\right)$ with a tangent value of $1$. The correct angle is ${\tan}^{−1}(1)=\dfrac{\pi}{4}$.

$try-it.png$ Try It $\PageIndex{2}$

Evaluate each of the following.

${\sin}^{−1}(−1)$

Answer a: $-\dfrac{\pi}{2}$

${\tan}^{−1}(−1)$

Answer b: $-\dfrac{\pi}{4}$

${\cos}^{−1}(−1)$

Answer c: $\pi$

${\cos}^{−1}\left(\dfrac{1}{2}\right)$

Answer d: $\dfrac{\pi}{3}$

Use a Calculator to Evaluate Inverse Trigonometric Functions

To evaluate inverse trigonometric functions that do not involve the special angles discussed previously, we will need to use a calculator or other type of technology. Most scientific calculators and calculator-emulating applications have specific keys or buttons for the inverse sine, cosine, and tangent functions. These may be labeled, for example, SIN^-1, ARCSIN, or ASIN.

In the previous chapter, we worked with trigonometry on a right triangle to solve for the sides of a triangle given one side and an additional angle. Using the inverse trigonometric functions, we can solve for the angles of a right triangle given two sides, and we can use a calculator to find the values to several decimal places.

In these examples and exercises, the answers will be interpreted as angles and we will use $\theta$ as the independent variable. The value displayed on the calculator may be in degrees or radians, so be sure to set the mode appropriate to the application.

Example $\PageIndex{3}$: Evaluate Inverse Sine on a Calculator

Evaluate ${\sin}^{−1}(0.97)$ using a calculator.

Solution

Because the output of the inverse function is an angle, the calculator will give us a degree value if in degree mode and a radian value if in radian mode. Calculators also use the same domain restrictions on the angles as we are using.

In radian mode, ${\sin}^{−1}(0.97)≈1.3252$. In degree mode, ${\sin}^{−1}(0.97)≈75.93°$. Note that in calculus and beyond we will use radians in almost all cases.

$try-it.png$ Try It $\PageIndex{3}$

Evaluate ${\cos}^{−1}(−0.4)$ using a calculator.

Answer: $1.9823 \;$ or $113.578^{\circ}$

Given two sides of a right triangle, find an angle.

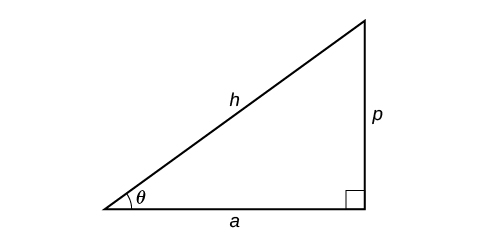An illustration of a right triangle with an angle theta. Adjacent to theta is the side a, opposite theta is the side p, and the hypoteneuse is side h.

$h \quad$ is the length of the hypotenuse

$a \quad$ is the length of the side adjacent to the desired angle

$p \quad$ is the length of the side opposite to the desired angle

$\theta \quad$ is the "desired" angle with unknown measure between the hypotenuse and the adjacent side

Given $h$ and $a$, $\quad$ find the angle using $\theta={\cos}^{−1}\left(\dfrac{a}{h}\right)$ $\quad$and the other side using $ p = \sqrt{h^2-a^2}$.
Given $h$ and $p$, $\quad$ find the angle using $\theta={\sin}^{−1}\left(\dfrac{p}{h}\right)$ $\quad$and the other side using $ a = \sqrt{h^2-p^2}$.
Given $p$ and $a$ $\quad$ find the angle using $\theta={\tan}^{−1}\left(\dfrac{p}{a}\right)$ $\quad$and the other side using $ h = \sqrt{p^2+a^2}$.

Example $\PageIndex{4}$: Applying the Inverse Cosine to a Right Triangle

Solve the triangle in Figure $\PageIndex{4}$ for the angle $\theta$.

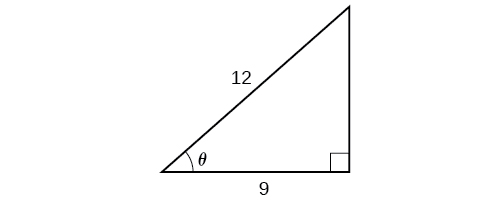
An illustration of a right triangle with the angle theta. Adjacent to the angle theta is a side with a length of 9 and a hypoteneuse of length 12. — Figure $\PageIndex{4}$

Solution

Because we know the hypotenuse and the side adjacent to the angle, it makes sense for us to use the cosine function.

\[\begin{align*} \cos \theta&= \dfrac{9}{12}\\
\theta &= {\cos}^{-1}\left(\dfrac{9}{12}\right) && \text{Apply definition of the inverse}\\
\theta &\approx 0.7227 &&\text{or about } 41.4096^{\circ}\end{align*}\]

$try-it.png$ Try It $\PageIndex{4}$

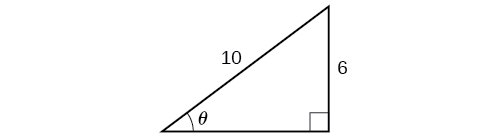
An illustration of a right triangle with the angle theta. Opposite to the angle theta is a side with a length of 6 and a hypoteneuse of length 10. — Figure $\PageIndex{4t}$

Solve the triangle in Figure $\PageIndex{4t}$ for the angle $\theta$.

Answer: ${\sin}^{−1}(0.6)=36.87°=0.6435$ radians

Finding Exact Values of Composite Functions with Inverse Trigonometric Functions

There are times when we need to compose a trigonometric function with an inverse trigonometric function. In these cases, we can usually find exact values for the resulting expressions without resorting to a calculator. Even when the input to the composite function is a variable or an expression, we can often find an expression for the output. To help sort out different cases, let $f(x)$ and $g(x)$ be two different trigonometric functions belonging to the set{ $\sin(x)$, $\cos(x)$, $\tan(x)$ } and let $f^{-1}(y)$ and $g^{-1}(y)$ be their inverses.

Evaluating Compositions of the Form $f(f^{-1}(y))$ and $f^{-1}(f(x))$

For any trigonometric function, $f(f^{-1}(y))=y$ for all $y$ in the proper domain for the given function. This follows from the definition of the inverse and from the fact that the range of $f$ was defined to be identical to the domain of $f^{−1}$. However, we have to be a little more careful with expressions of the form $f^{-1}(f(x))$.

COMPOSITIONS OF A TRIGONOMETRIC FUNCTION AND ITS INVERSE

For all $x$ below, $\alpha$ is the reference angle for $x$

$ \begin{array} {lclll}
\\ {\sin}(\sin^{−1}(x)) =
{ \begin{cases}
    x \qquad & \mbox{if } −1 \le x \le 1 \\
    \text{Undefined} & \mbox{if } |x| > 1 \\
\end{cases}
} \\[20pt]
{\cos}(\cos^{−1}(x)) =
{ \begin{cases}
    x \qquad & \mbox{if } −1 \le x \le 1 \\
    \text{Undefined} & \mbox{if } |x| > 1 \\
\end{cases}
} \\[20pt]
{\tan}(\tan^{−1}(x)) = \;\;\;x \qquad \qquad \;\;\;\text{ for all real numbers } x
\end{array} $

$ \begin{array} {lclll}
{\sin}^{−1}(\sin(x)) =
{ \begin{cases}
    x \qquad & \mbox{if } −\dfrac{\pi}{2} \le x \le \dfrac{\pi}{2} \\
    \alpha  & \text{if } x \ge 0\\
    -\alpha & \mbox{if } x < 0\\
\end{cases}
} \\[6pt]
{\cos}^{−1}(\cos(x)) =
{ \begin{cases}
   x & \mbox{if } |0 \le x \le \pi \\
    \alpha       & \mbox{if } x \ge 0\\
    \pi -\alpha & \mbox{if } x < 0\\
\end{cases}
} \\[6pt]
{\tan}^{−1}(\tan(x)) =
{ \begin{cases}
    x \qquad & \mbox{if } −\dfrac{\pi}{2} \le x \le \dfrac{\pi}{2} \\
    \alpha  & \text{if } x \ge 0\\
    -\alpha & \mbox{if } x < 0\\
\end{cases}
}
\end{array} $

$QA.png$ Is it correct that $ \sin ^{-1} ( \sin x ) = x $ ?

No. This equation is correct only if $x$ belongs to the restricted domain$\left[−\dfrac{\pi}{2},\dfrac{\pi}{2}\right]$, but sine is defined for all real input values, and for $x$ outside the restricted interval, the equation is not correct because its inverse always returns a value in $\left[ −\dfrac{\pi}{2},\dfrac{\pi}{2} \right]$. The situation is similar for cosine and tangent and their inverses. For example, ${\sin}^{−1}\left(\sin\left(\dfrac{3\pi}{4}\right)\right)=\dfrac{\pi}{4}$.

Given an expression of the form $f^{-1}(f(\theta))$ where $f(\theta)=\sin \theta$, $\cos \theta$, or $\tan \theta$, evaluate.

If $\theta$ is in the restricted domain of $f$, then $f^{−1}(f(\theta))=\theta$.
If not, then find an angle $\phi$ within the restricted domain of $f$ such that $f(\phi)=f(\theta)$. Then $f^{−1}(f(\theta))=\phi$.

Example $\PageIndex{5}$: Using Inverse Trigonometric Functions

Evaluate the following:

${\sin}^{−1}\left (\sin \left(\dfrac{\pi}{3}\right )\right ) $

${\sin}^{−1}\left (\sin \left(\dfrac{2\pi}{3}\right ) \right)$

${\cos}^{−1}\left (\cos \left (\dfrac{2\pi}{3}\right )\right)$

$ {\cos}^{−1}\left (\cos \left (−\dfrac{\pi}{3}\right )\right)$

Solution

$\dfrac{\pi}{3}$ is in $\left[−\dfrac{\pi}{2},\dfrac{\pi}{2}\right]$, so ${\sin}^{−1}\left(\sin\left(\dfrac{\pi}{3}\right)\right)=\dfrac{\pi}{3}$.
$\dfrac{2\pi}{3}$ is not in $\left[−\dfrac{\pi}{2},\dfrac{\pi}{2}\right]$, but $\sin\left(\dfrac{2\pi}{3}\right)=\sin\left(\dfrac{\pi}{3}\right)$, so ${\sin}^{−1}\left(\sin\left(\dfrac{2\pi}{3}\right)\right)=\dfrac{\pi}{3}$.
$\dfrac{2\pi}{3}$ is in $[ 0,\pi ]$, so ${\cos}^{−1}\left(\cos\left(\dfrac{2\pi}{3}\right)\right)=\dfrac{2\pi}{3}$.
$-\dfrac{\pi}{3}$ is not in $[ 0,\pi ]$, but $\cos\left(−\dfrac{\pi}{3}\right)=\cos\left(\dfrac{\pi}{3}\right)$ because cosine is an even function. $\dfrac{\pi}{3}$ is in $[ 0,\pi ]$, so ${\cos}^{−1}\left(\cos\left(−\dfrac{\pi}{3}\right)\right)=\dfrac{\pi}{3}$.

$try-it.png$ Try It $\PageIndex{5}$

Evaluate ${\tan}^{−1}\left(\tan\left(\dfrac{\pi}{8}\right)\right)$ and ${\tan}^{−1}\left(\tan\left(\dfrac{11\pi}{9}\right)\right)$.

Answer: $\dfrac{\pi}{8}$; $\dfrac{2\pi}{9}$

Evaluating Compositions of the Form $f^{-1}(g(x))$

Now that we can compose a trigonometric function with its inverse, we can explore how to evaluate a composition of a trigonometric function and the inverse of another trigonometric function. We will begin with compositions of the form $f^{-1}(g(x))$. For special values of $x$, we can exactly evaluate the inner function and then the outer, inverse function.

Example $\PageIndex{6}$: Evaluating the Composition of an Inverse Sine with a Cosine

Evaluate ${\sin}^{−1}\left(\cos\left(\dfrac{13\pi}{6}\right)\right)$

Solution

We can directly evaluate the inside of the composition. $ \cos \left( \dfrac{13\pi}{6} \right) = \cos \left( \dfrac{\pi}{6} +2\pi \right) = \cos\left (\dfrac{\pi}{6}\right ) = \dfrac{\sqrt{3}}{2}$

Now, we can evaluate the inverse function as we did earlier. ${\sin}^{−1}\left(\cos\left(\dfrac{13\pi}{6}\right)\right) = {\sin}^{−1}\left (\dfrac{\sqrt{3}}{2}\right )=\dfrac{\pi}{3}$

$try-it.png$ Try It $\PageIndex{6}$

Evaluate ${\cos}^{−1}\left (\sin\left (−\dfrac{11\pi}{4}\right )\right )$.

Answer: $\dfrac{3\pi}{4}$

Evaluating Compositions of the Form $f(g^{−1}(x))$

To evaluate compositions of the form $f(g^{−1}(x))$, where $f$ and $g$ are any two of the functions sine, cosine, or tangent and $x$ is any input in the domain of $g^{−1}$, sometimes Identities can be used, like the Pythagorean identity, ${\sin}^2 x+{\cos}^2 x=1$. Another approach uses the fact that the inner function is an inverse trigonometric function, and therefore tells us the quadrant the angle is in and the value of a trigonometric ratio for that angle. From this information, the other side of the triangle, and any other trigonometric ratio can be obtained.

Example $\PageIndex{7}$: Evaluating the Composition of a Sine with an Inverse Cosine

Find an exact value for $\sin\left({\cos}^{−1}\left(\dfrac{4}{5}\right)\right)$.

Solution

A restatement of the problem is: Find $\sin \theta $, given that $ \theta ={\cos}^{−1}\left(\dfrac{4}{5}\right)$.

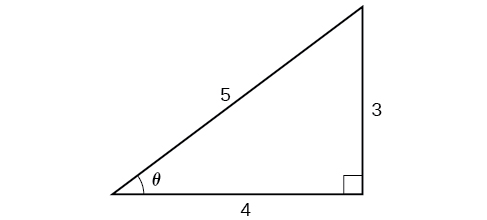
An illustration of a right triangle with an angle theta. Oppostie the angle theta is a side with length 3. Adjacent the angle theta is a side with length 4. The hypoteneuse has angle of length 5. — Figure $\PageIndex{7}$: Right triangle illustrating
that if $\cos \theta=\dfrac{4}{5}$, then $\sin \theta=\dfrac{3}{5}$

Method I. Using Definitions

The inverse cosine equation gives us two pieces of information.

The cosine ratio ( $4/5 $ ) is positive, so the angle $\theta $ is in Quadrant I, where $x$ and $y$ are both positive.
$\cos \theta = \dfrac {4}{5} = \dfrac{x}{r} $ so $\boxed{x=4}$ and $\boxed{r=5}$

Use the Pythagorean Theorem to find the third side.
$y$ will be positive because $\theta$ is in Quadrant I.
$x^2+y^2=r^2 \rightarrow 4^2+y^2=5^2 \rightarrow y^2=25-16,$ so $y = \pm 3 $ but since $\theta$ is in Quadrant I, $\boxed{y=3}$.

Use the definition of the sine ratio: $ \sin \theta = \dfrac{y}{r} = \dfrac{3}{5} $.

Method II. Using Identities.

We can say that $\theta={\cos}^{−1}\left (\dfrac{4}{5}\right )$ means $\cos \theta=\dfrac{4}{5}$.

We are looking for $\sin \theta$. We can use the Pythagorean identity to do this.

\[\begin{align*} {\sin}^2 \theta+{\cos}^2 \theta&= 1\qquad \text{Use our known value for cosine}\\ {\sin}^2 \theta+{\left (\dfrac{4}{5} \right )}^2&= 1\qquad \text{Solve for sine}\\ {\sin}^2 \theta&= 1-\dfrac{16}{25}\\ \sin \theta&=\pm \dfrac{9}{25}\\ &= \pm \dfrac{3}{5} \end{align*}\]

We know that the inverse cosine always gives an angle on the interval $[ 0,\pi ]$, so we know that the sine of that angle must be positive. Since $\theta={\cos}^{−1}\left (\dfrac{4}{5}\right )$ is in quadrant I, $\sin \theta$ must be positive, so the solution is \(\dfrac{3}{5}\

$try-it.png$ Try It $\PageIndex{7}$

Evaluate $\cos \left ({\tan}^{−1} \left (\dfrac{5}{12} \right ) \right )$.

Answer: $\frac{12}{13}$

Example $\PageIndex{8}$: Evaluating the Composition of a Sine with an Inverse Tangent

Find an exact value for $\sin\left({\tan}^{−1}\left(-\dfrac{7}{4}\right)\right)$.

Solution

Restating the problem: Find $\sin \theta $, given that $ \theta = {\tan}^{−1}\left(-\dfrac{7}{4}\right) $.

From $ \theta = {\tan}^{−1}\left(-\dfrac{7}{4}\right) $ we can obtain 2 pieces of information.

Inverse tangent always gives an angle in QI or QIV, but since the tangent ratio ( $-7/4 $ ) is negative, the angle is in Quadrant IV where $x$ is positive and $y$ is negative.
Rewriting the inverse tangent expression, and applying the definition of tangent, we can obtain $\tan \theta = -\dfrac{7}{4} = \dfrac{y}{x=4} $ so $\boxed{x=4}$ and $\boxed{y=-7}$

Use the Pythagorean Theorem $r^2 = x^2+y^2$ to find $r$.
$r = \sqrt{(4)^2+(-7)^2} = \sqrt{16+49}$ .
$r$ is always positive so $ \boxed{r = \sqrt{65}}$.

Using the definition of the sine function, and the diagram of angle $\theta$ in standard position, we find $ \sin \theta = \dfrac{y}{r} = \dfrac{-7}{\sqrt{65}} = -\dfrac{7\sqrt{65}}{65} $.

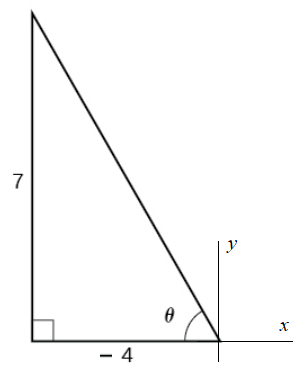
$6.3 triangle QII.png$

Figure $\PageIndex{8}$: A right triangle with two sides known

$try-it.png$ Try It $\PageIndex{8}$

Evaluate $\cos\left({\sin}^{−1}\left(-\dfrac{7}{9}\right)\right)$.

Answer: $\dfrac{4\sqrt{2}}{9}$

Example $\PageIndex{9}$: Finding the Cosine of the Inverse Sine of an Algebraic Expression

Find a simplified expression for $\cos\left({\sin}^{−1}\left(\dfrac{u}{3}\right)\right)$. State any restrictions to $u$.

Solution

Restating the problem: Find an expression for $\cos\theta$ given $\theta = {\sin}^{−1}\left(\dfrac{u}{3}\right) $

Method using Definitions

The inverse sine function gives us two pieces of information:

$\theta$ will be in either the first or fourth quadrants, where we know $x$ is only positive.
$\sin \theta = \left(\dfrac{u}{3}\right) =\left(\dfrac{y}{r}\right)$, so therefore, $\boxed{y = u}$, and $\boxed{r = 3} $.

Use the Pythagorean Theorem to find $x$:
$x^2+y^2=r^2 \rightarrow x^2+u^2 = (3)^2 \rightarrow x^2 = 9-u^2$.
Therefore, $\boxed{x=\sqrt{9-u^2}}$

Finally, using the definition for cosine, we obtain $\cos\theta = \dfrac{x}{r}= \dfrac{\sqrt{9-u^2}}{3}$

Method Using Identities

We know there is an angle $\theta$ such that $\sin \theta=\dfrac{u}{3}$.

\[\begin{align*} {\sin}^2 \theta+{\cos}^2 \theta&= 1\qquad \text{Use the Pythagorean Theorem}\\ {\left (\dfrac{u}{3}\right )}^2+{\cos}^2 \theta&= 1\qquad \text{Solve for cosine}\\ {\cos}^2 \theta&= 1-\dfrac{u^2}{9}\\ \cos \theta &= \pm \sqrt{\dfrac{9-u^2}{9}}\\ &= \pm \dfrac{\sqrt{9-u^2}}{3} \end{align*}\]

Because we know that the inverse sine must give an angle on the interval $[ −\dfrac{\pi}{2},\dfrac{\pi}{2} ]$, we can deduce that the cosine of that angle must be positive.

$\cos\left({\sin}^{−1}\left(\dfrac{u}{3}\right)\right)=\dfrac {\sqrt{9-u^2}}{3} $

Given $ \sin^{−1}\left( \dfrac{u}{3} \right) $ then $ \sin \theta = \dfrac{u}{3} $. Since $ −1 ≤ \sin \theta ≤ 1 $ then $ −1 ≤ \dfrac{u}{3} ≤ 1 $ and thus $−3≤u≤3$.

$try-it.png$ Try It $\PageIndex{9}$

Find a simplified expression for $\sin({\tan}^{−1}(4u))$. State any restrictions to $u$.

Answer: $\dfrac{4u}{\sqrt{16^2+1}}$. No restrictions to $u$ because $ \tan \theta $ can be any real number.

Key Concepts

An inverse function is one that “undoes” another function. The domain of an inverse function is the range of the original function and the range of an inverse function is the domain of the original function.
Because the trigonometric functions are not one-to-one on their natural domains, their domains are restricted so that inverse trigonometric functions can be defined for them.
For any trigonometric function $f(x)$, if $x=f^{−1}(y)$, then $f(x)=y$. However, $f(x)=y$ only implies $x=f^{−1}(y)$ if $x$ is in the restricted domain of $f$.
Special angles are the outputs of inverse trigonometric functions for special input values; for example, $\frac{\pi}{4}={\tan}^{−1}(1)$ and $\frac{\pi}{6}={\sin}^{−1}(\frac{1}{2})$.
A calculator will return an angle within the restricted domain of the original trigonometric function.
Inverse functions allow us to find an angle when given two sides of a right triangle.
In function composition, if the inside function is an inverse trigonometric function, then the result of the composition is an exact expression; for example,$\sin({\cos}^{−1}(x))=\sqrt{1−x^2}$.
When evaluating the composition of a trigonometric function with an inverse trigonometric function, draw a reference triangle to assist in determining the ratio of sides that represents the output of the trigonometric function.
When evaluating the composition of a trigonometric function with an inverse trigonometric function, you may use trig identities to assist in determining the ratio of sides.

Contributors

Jay Abramson (Arizona State University) with contributing authors. Textbook content produced by OpenStax College is licensed under a Creative Commons Attribution License 4.0 license. Download for free at https://openstax.org/details/books/precalculus.

Understanding and Using the Inverse Sine, Cosine, and Tangent Functions

Find Exact Special Angle Values for Inverse Sine, Cosine, and Tangent Functions

Use a Calculator to Evaluate Inverse Trigonometric Functions

Finding Exact Values of Composite Functions with Inverse Trigonometric Functions

Evaluating Compositions of the Form \(f(f^{-1}(y))\) and \(f^{-1}(f(x))\)

Evaluating Compositions of the Form \(f^{-1}(g(x))\)

Evaluating Compositions of the Form \(f(g^{−1}(x))\)

Key Concepts

Contributors