3.6E: Exercises
This page is a draft and is under active development.
( \newcommand{\kernel}{\mathrm{null}\,}\)
Exercise \PageIndex{1}
For the following exercises, use implicit differentiation to find \dfrac{dy}{dx}.
1) x^2−y^2=4
2) 6x^2+3y^2=12
3) x^2y=y−7
4) 3x^3+9xy^2=5x^3
5) xy−cos(xy)=1
6) y\sqrt{x+4}=xy+8
7) −xy−2=\dfrac{x}{7}
8) ysin(xy)=y^2+2
9) (xy)^2+3x=y^2
10) x^3y+xy^3=−8
- Answer
-
1. \dfrac{dy}{dx}=\dfrac{x}{y}
2. \dfrac{dy}{dx}=\dfrac{−2x}{y}
3. \dfrac{dy}{dx}=\dfrac{-2xy}{x^2-1}
4. \dfrac{dy}{dx}=\dfrac{x}{3y}−\dfrac{y}{2x}
5. \dfrac{dy}{dx}=\dfrac{-y}{x}
6. \dfrac{dy}{dx}=\dfrac{y−\dfrac{y}{2\sqrt{x+4}}}{\sqrt{x+4}−x}
7. \dfrac{dy}{dx}=-\dfrac{1+7y}{7x}
8. \dfrac{dy}{dx}=\dfrac{y^2cos(xy)}{2y−sin(xy)−xycosxy}
9. \dfrac{dy}{dx}=\dfrac{-2xy^2-3}{2y(x^2-1)}
10. \dfrac{dy}{dx}=\dfrac{−3x^2y−y^3}{x^3+3xy^2}
- Solution
-
1. x^2−y^2=4
2x-2y \dfrac{dy}{dx}=0
2y \dfrac{dy}{dx}=2x
\dfrac{dy}{dx}=\dfrac{2x}{2y}
\dfrac{dy}{dx}=\dfrac{x}{y}, y\ne 0
2. 6x^2+3y^2=12
12x+6y \dfrac{dy}{dx}=0
6y \dfrac{dy}{dx}=-12x
\dfrac{dy}{dx}=\dfrac{-12x}{6y}
\dfrac{dy}{dx}=\dfrac{-2x}{y}\, , y\ne 0
3. x^2y=y−7
2xy+x^2\dfrac{dy}{dx}=\dfrac{dy}{dx}-0
x^2 \dfrac{dy}{dx}-\dfrac{dy}{dx}=-2xy
\dfrac{dy}{dx}(x^2-1)=-2xy
\dfrac{dy}{dx}=\dfrac{-2xy}{x^2-1} , x^2-1\ne 0
4. 3x^3+9xy^2=5x^3
9x^2+9y^2+18xy\dfrac{dy}{dx}=15x^2
18xy\dfrac{dy}{dx}=3x^2-9y^2
\dfrac{dy}{dx}=\dfrac{3x^2-9y^2}{18xy}
\dfrac{dy}{dx}=\dfrac{x}{3y}-\dfrac{y}{2x}, y\ne 0,x\ne 0
5. xy−cos(xy)=1
x\dfrac{dy}{dx}+y+sin(xy)(x(\dfrac{dy}{dx})+y)=0
x\dfrac{dy}{dx}+x(\dfrac{dy}{dx})sin(xy)+ysin(xy)=-y
x\dfrac{dy}{dx}(1+sin(xy))=-y-ysin(xy)
x\dfrac{dy}{dx}(1+sin(xy))=-y(1+sin(xy))
x\dfrac{dy}{dx}=-y
\dfrac{dy}{dx}=\dfrac{-y}{x},, x\ne 0
6. y\sqrt{x+4}=xy+8
y(x+4)^{(1/2)}=xy+8
\dfrac{1}{2}y(x+4)^{(-1/2)}+\dfrac{dy}{dx}(x+4)^{(1/2)}=x\dfrac{dy}{dx}+y
\dfrac{dy}{dx}\sqrt{x+4}-x\dfrac{dy}{dx}=y-\dfrac{y}{2\sqrt{x+4}}
\dfrac{dy}{dx}(\sqrt{x+4}-x)=y-\dfrac{y}{2\sqrt{x+4}}
\dfrac{dy}{dx}=\dfrac{y-\dfrac{y}{2\sqrt{x+4}}}{\sqrt{x+4}-x}, \sqrt{x+4}-x\ne 0.
7. −xy−2=\dfrac{x}{7}
-x\dfrac{dy}{dx}-y-0=\dfrac{1}{7}
-x\dfrac{dy}{dx}=\dfrac{1}{7}+y
\dfrac{dy}{dx}=\dfrac{\dfrac{1}{7}+y}{-x}
\dfrac{dy}{dx}=-\dfrac{1+7y}{7x}, x\ne 0.
8. ysin(xy)=y^2+2
ycos(xy)\left(x\dfrac{dy}{dx}+y\right)+\dfrac{dy}{dx}sin(xy)=2y\dfrac{dy}{dx}
\dfrac{dy}{dx}xycos(xy)+y^2cos(xy)+\dfrac{dy}{dx}sin(xy)-2y\dfrac{dy}{dx}=0
\dfrac{dy}{dx}xycos(xy)+\dfrac{dy}{dx}sin(xy)-2y\dfrac{dy}{dx}=-y^2cos(xy)
\dfrac{dy}{dx}(xycos(xy)+sin(xy)-2y)=-y^2cos(xy)
\dfrac{dy}{dx}=\dfrac{-y^2cos(xy)}{-2y+sin(xy)+xycos(xy)}
\dfrac{dy}{dx}=\dfrac{y^2cos(xy)}{2y−sin(xy)−xycosxy}, 2y−sin(xy)−xycosxy \ne 0.
9. (xy)^2+3x=y^2
2xy\left(x\dfrac{dy}{dx}+y\right)+3=2y\dfrac{dy}{dx}
2x^2y\dfrac{dy}{dx}+2xy^2-2y\dfrac{dy}{dx}+3=0
2x^2y\dfrac{dy}{dx}+2xy^2-2y\dfrac{dy}{dx}+3=0
\dfrac{dy}{dx}(2x^2y-2y)=-2xy^2-3
\dfrac{dy}{dx}=\dfrac{-2xy^2-3}{2y(x^2-1)}, 2y(x^2-1)\ne 0.
10. x^3y+xy^3=−8
3x^2y+x^3\dfrac{dy}{dx}+y^3+3xy^2\dfrac{dy}{dx}=0
x^3\dfrac{dy}{dx}+3xy^2\dfrac{dy}{dx}=-3x^2y-y^3
\dfrac{dy}{dx}(x^3+3xy^2)=-3x^2y-y^3
\dfrac{dy}{dx}=\dfrac{-3x^2y-y^3}{x^3+3xy^2}, x \ne 0.
Exercise \PageIndex{2}
For the following exercises, find the equation of the tangent line to the graph of the given equation at the indicated point. Use a calculator or computer software to graph the function and the tangent line.
1) x^4y−xy^3=−2,(−1,−1)
2) x^2y^2+5xy=14,(2,1)
3) xy^2+sin(πy)−2x^2=10,(2,−3)
4) \dfrac{x}{y}+5x−7=−\dfrac{3}{4}y,(1,2)
5) xy+sin(x)=1,(\dfrac{π}{2},0)
- Answer
-
1.y=-\dfrac{5}{4}x-\dfrac{9}{4}
2. y=−\dfrac{1}{2}x+2
3. y=\dfrac{1}{π+12}x−\dfrac{3π+38}{π+12}
4.y=-\dfrac{11}{2}x+\dfrac{15}{2}
5. y=0\
- Solution
-
1. x^4y−xy^3=−2,(−1,−1)
Take the derivative:
4x^3y+x^4\dfrac{dy}{dx}-y^3-3xy^2\dfrac{dy}{dx}=0
Input x and y values to solve for \dfrac{dy}{dx} (this will be the slope of the tangent line):
4(-1)^3(-1)+(-1)^4\dfrac{dy}{dx}-(-1)^3-3(-1)(-1)^2\dfrac{dy}{dx}=0
4+\dfrac{dy}{dx}+1+3\dfrac{dy}{dx}=0
\dfrac{dy}{dx} (1+3)=-4-1
\dfrac{dy}{dx}=\dfrac{-5}{4}
Now use this slope and the x and y values provided to solve the slope point intercept equation:
y=\dfrac{-5}{4}x+b
-1=\dfrac{-5}{4}(-1)+b
-1=\dfrac{5}{4}+b
-1-\dfrac{5}{4}=b
b=\dfrac{-9}{4}
Therefore the equation of the tangent line is:
y=-\dfrac{5}{4}x-\dfrac{9}{4}
2. x^2y^2+5xy=14,(2,1)
Take the derivative:
2xy^2+2yx^2\dfrac{dy}{dx}+5y+5x\dfrac{dy}{dx}=0
Input x and y values to solve for \dfrac{dy}{dx} (this will be the slope of the tangent line):
2(2)(1)^2+2(1)(2)^2\dfrac{dy}{dx}+5(1)+5(2)\dfrac{dy}{dx}=0
4+8\dfrac{dy}{dx}+5+10\dfrac{dy}{dx}=0
18\dfrac{dy}{dx}=-9
\dfrac{dy}{dx}=\dfrac{-1}{2}
Now use this slope and the x and y values provided to solve the slope point intercept equation:
y=\dfrac{-1}{2}x+b
1=\dfrac{-1}{2}(2)+b
1=-1+b
2=b
Therefore, the equation of the tangent line is:
y=\dfrac{-1}{2}+2
3. xy^2+sin(πy)−2x^2=10,(2,−3)
Take the derivative:
y^2+2xy\dfrac{dy}{dx}+\pi cos(\pi y) \dfrac{dy}{dx} -4x=0
Input x and y values to solve for \dfrac{dy}{dx} (this will be the slope of the tangent line):
(-3)^2+2(2)(-3)\dfrac{dy}{dx}+\pi cos(-3\pi)\dfrac{dy}{dx}-4(2)=0
9-12\dfrac{dy}{dx}0-\pi \dfrac{dy}{dx}-8=0
-12-\pi(\dfrac{dy}{dx})=-1
\dfrac{dy}{dx}=\dfrac{1}{12+\pi}
Now use this slope and the x and y values provided to solve the slope point intercept equation:
y=\dfrac{1}{12+ \pi}x+b
-3=(\dfrac{1}{12+ \pi})2+b
\dfrac{-3(12+\pi)-2}{12+ \pi}=b
\dfrac{-38-3\pi}{12+\pi}=b
Therefore the equation of the tangent line is:
y=\dfrac{1}{π+12}x−\dfrac{3π+38}{π+12}
Exercise \PageIndex{3}
The graph of a folium of Descartes with equation 2x^3+2y^3−9xy=0 is given in the following graph.
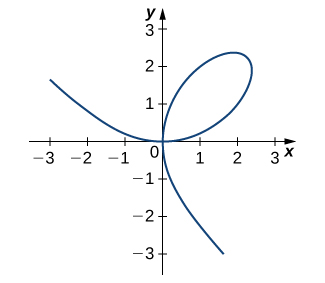
a. Find the equation of the tangent line at the point (2,1). Graph the tangent line along with the folium.
b. Find the equation of the normal line to the tangent line in a. at the point (2,1).
- Answer
-
Under Construction
Exercise \PageIndex{4}
For the equation x^2+2xy−3y^2=0,
a. Find the equation of the normal to the tangent line at the point (1,1).
b. At what other point does the normal line in a. intersect the graph of the equation?
- Answer
-
a. y=−x+2
b. (3,−1)
Exercise \PageIndex{5}
Find all points on the graph of y^3−27y=x^2−90 at which the tangent line is vertical.
For the equation x^2+xy+y^2=7,
a. Find the x-intercept(s).
b.Find the slope of the tangent line(s) at the x-intercept(s).
c. What does the value(s) in b. indicate about the tangent line(s)?
- Answer
-
a. (±7√,0)
b. −2
c. They are parallel since the slope is the same at both intercepts.
Exercise \PageIndex{6}
Find the equation of the tangent line to the graph of the equation sin^{−1}x+sin^{−1}y=\dfrac{π}{6} at the point (0,\dfrac{1}{2}).
- Answer
-
Under Construction
Exercise \PageIndex{7}
Find the equation of the tangent line to the graph of the equation \tan^{−1}(x+y)=x^2+\frac{π}{4} at the point (0,1).
- Answer
-
y=−x+1
Exercise \PageIndex{8}
Find y′ and y'' for x^2+6xy−2y^2=3.
- Answer
-
Under Construction
Exercise \PageIndex{9}
The number of cell phones produced when x dollars is spent on labor and y dollars is spent on capital invested by a manufacturer can be modeled by the equation 60x^{3/4}y^{1/4}=3240.
a. Find \dfrac{dy}{dx} and evaluate at the point (81,16).
b. Interpret the result of a.
- Answer
-
a. −0.5926
b. When $81 is spent on labour, and $16 is spent on capital, the amount spent on capital decreases by $0.5926 per $1 spent on labour.
Exercise \PageIndex{10}
The number of cars produced when x dollars is spent on labor and y dollars is spent on capital invested by a manufacturer can be modeled by the equation 30x^{1/3}y^{2/3}=360.
(Both xand y are measured in thousands of dollars.)
a. Find \dfrac{dy}{dx} and evaluate at the point (27,8).
b. Interpret the result of a.
- Answer
-
Under Construction
Exercise \PageIndex{11}
The volume of a right circular cone of radius x and height y is given by V=\dfrac{1}{3}πx^2y. Suppose that the volume of the cone is 85πcm^3. Find \dfrac{dy}{dx} when x=4 and y=16.
- Answer
-
−8
Exercise \PageIndex{12}
For the following exercises, consider a closed rectangular box with a square base with side x and height y.
1) Find an equation for the surface area of the rectangular box, S(x,y).
2) If the surface area of the rectangular box is 78 square feet, find \dfrac{dy}{dx} when x=3 feet and y=5 feet.
- Answers to even numbered questions
-
2. −2.67
Exercise \PageIndex{13}
For the following exercises, use implicit differentiation to determine y′. Does the answer agree with the formulas we have previously determined?
1) x=siny
2) x=cosy
3) x=tany
- Answers to even numbered questions
-
2. y′=−\dfrac{1}{\sqrt{1−x^2}}

