8.1: Example
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
Separation of variables in polar coordinates
Example
Consider a circular plate of radius \(c \text{ m}\), insulated from above and below. The temperature on the circumference is \(100^\circ~\text{C}\) on half the circle, and \(0^\circ~\text{C}\) on the other half.
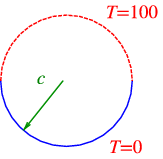
The differential equation to solve is \[\rho^2 \pdrhor{u} + \rho \pdrho{u} + \pdphip{u} = 0,\] with boundary conditions \[u(c,\phi) = \begin{cases} 100 & \text{if $0 < \phi < \pi$} \\ 0 & \text{if $\pi < \phi < 2\pi $} \end{cases}\quad .\]
Periodic BC
There is no real boundary in the \(\phi\) direction, but we introduce one, since we choose to let \(\phi\) run from \(0\) to \(2\pi\) only. So what kind of boundary conditions do we apply? We would like to see “seamless behaviour”, which specifies the periodicity of the solution in \(\phi\), \[\begin{aligned} u(\rho,\phi+2\pi)&=&u(\rho,\phi),\\ \pdphi{u}(\rho,\phi+2\pi)&=&\pdphi{u}(\rho,\phi).\end{aligned}\] If we choose to put the seem at \(\phi=-\pi\) we have the periodic boundary conditions \[\begin{aligned} u(\rho,2\pi)&=&u(\rho,0),\\ \pdphi{u}(\rho,2\pi)&=&\pdphi{u}(\rho,0).\end{aligned}\]
We separate variables, and take, as usual \[u(\rho,\phi) = R(\rho) \Phi(\phi).\] This gives the usual differential equations \[\begin{aligned} \Phi''-\lambda\Phi &=&0,\\ \rho^2 R'' + \rho R' + \lambda R &=& 0.\end{aligned}\] Our periodic boundary conditions gives a condition on \(\Phi\), \[\Phi(0)=\Phi(2\pi),\;\;\Phi'(0)=\Phi'(2\pi). \label{eq:phiBC}\] The other boundary condition involves both \(R\) and \(\Phi\).
Three cases for \(\lambda\)
As usual we consider the cases \(\lambda>0\), \(\lambda<0\) and \(\lambda=0\) separately. Consider the \(\Phi\) equation first, since this has the most restrictive explicit boundary conditions ([eq:phiBC]).
We have to solve \[\Phi'' = \alpha^2\Phi,\] which has as a solution \[\Phi(\phi) = A \cos \alpha \phi + B \sin \alpha \phi.\] Applying the boundary conditions, we get \[\begin{aligned}
A &=& A \cos(2\alpha\pi)+ B\sin(2\alpha\pi),
\\
B \alpha &=&
-A \alpha\sin(2\alpha\pi)+ B\alpha\cos(2\alpha\pi).\end{aligned}\] If we eliminate one of the coefficients from the equation, we get \[A = A \cos(2\alpha\pi)-A\sin(2\alpha\pi)^2/(1-cos(2\alpha\pi))\] which leads to \[\sin(2\alpha\pi)^2=-(1-cos(2\alpha\pi))^2,\] which in turn shows \[2\cos(2\alpha\pi)=2,\] and thus we only have a non-zero solution for \(\alpha=n\), an integer. We have found \[\lambda_n=n^2,\;\;\Phi_n(\phi) =
A_n \cos n \phi + B_n \sin n \phi.\] We have \[\Phi'' = 0 .\] This implies that \[\Phi = A\phi + B.\] The boundary conditions are satisfied for \(A=0\), \[\Phi_0(\phi)=B_n.\] The solution (hyperbolic sines and cosines) cannot satisfy the boundary conditions.
height 1mm width 5cm
Now let me look at the solution of the \(R\) equation for each of the two cases (they can be treated as one), \[\rho^2 R''(\rho) + \rho R'(\rho) -n^2R(\rho)=0.\] Let us attempt a power-series solution (this method will be discussed in great detail in a future lecture) \[R(\rho) =\rho^\alpha.\] We find the equation \[\rho^\alpha[\alpha(\alpha-1)+\alpha^2-n^2]= \rho^\alpha[\alpha^2-n^2]=0\] If \(n\neq 0\) we thus have two independent solutions (as should be) \[R_n(\rho) = C\rho^{-n}+D\rho^n\] The term with the negative power of \(\rho\) diverges as \(\rho\) goes to zero. This is not acceptable for a physical quantity (like the temperature). We keep the regular solution, \[R_n(\rho) = \rho^n.\] For \(n=0\) we find only one solution, but it is not very hard to show (e.g., by substitution) that the general solution is \[R_0(\rho) = C_0 + D_0\ln(\rho).\] We reject the logarithm since it diverges at \(\rho=0\).
Putting it all together
In summary, we have \[u(\rho,\phi) = \frac{A_0}{2} + \sum_{n=1}^\infty \rho^n \left(A_n\cos n\phi+B_n\sin n \phi \right).\] The one remaining boundary condition can now be used to determine the coefficients \(A_n\) and \(B_n\), \[\begin{aligned} U(c,\phi) &=& \frac{A_0}{2} + \sum_{n=1}^\infty c^n \left(A_n\cos n\phi+B_n\sin n \phi \right)\nonumber\\ &=& \begin{cases} 100 & \text{if $0 < \phi < \pi$} \\ 0 & \text{if $\pi < \phi < 2\pi$} \end{cases}\quad.\end{aligned}\] We find \[\begin{aligned} A_0 &=& \frac{1}{\pi} \int_0^\pi 100\, d\phi = 100, \nonumber\\ c^n A_n &=& \frac{1}{\pi}\int_0^\pi 100\cos n\phi\, d\phi = \frac{100}{n\pi} \sin(n\phi)|^\pi_0=0,\nonumber\\ c^n B_n &=& \frac{1}{\pi}\int_0^\pi 100\sin n\phi\, d\phi = -\frac{100}{n\pi} \cos(n\phi)|^\pi_0 \nonumber\\&=& \begin{cases} 200/(n\pi) & \text{if $n$ is odd}\\ 0 & \text{if $n$ is even} \end{cases}\quad.\end{aligned}\] In summary \[u(\rho,\phi) = 50 + \frac{200}{\pi} \sum_{n~\rm odd} \left(\frac{\rho}{c}\right)^n \frac{\sin n \phi}{n}. \label{eq:T0-100}\] We clearly see the dependence of \(u\) on the pure number \(r/c\), rather than \(\rho\). A three dimensional plot of the temperature is given in Fig. [fig:T0-100].