1.5: Chapter 1 Exercises with Solutions
( \newcommand{\kernel}{\mathrm{null}\,}\)
In Exercises
80
- Answer
-
108
180
- Answer
-
160
128
- Answer
-
192
32
- Answer
-
72
In Exercises
0.648
- Answer
-
There are three decimal places, so
0.62
0.240
- Answer
-
There are three decimal places, so
0.90
0.14
- Answer
-
There are two decimal places, so
0.760
0.888
- Answer
-
There are three decimal places, so
0.104
In Exercises
- Answer
-
Let
yields
- Answer
-
Let
yields
- Answer
-
Let
yields
- Answer
-
Let
yields
Prove that
- Answer
-
Suppose that
Square both sides,
then clear the equation of fractions by multiplying both sides by
Now p and q each have their own unique prime factorizations. Both
Therefore, our assumption that
Prove that
In Exercises
| 0 | |||||
| -2 | |||||
| -2/3 | |||||
| 0.15 | |||||
- Answer
-
0 x x x x -2 x x x -2/3 x x 0.15 x x x x x
| 10/2 | |||||
| -6 | |||||
| 0.37 |
| -4/3 | |||||
| 12 | |||||
| 0 | |||||
| 6/2 |
- Answer
-
-4/3 x x 12 x x x x x 0 x x x x x x x 6/2 x x x x x
| -3/5 | |||||
| 1.625 | |||||
| 10/2 | |||||
| 0/5 | |||||
| 11 |
In Exercises
All natural numbers are whole numbers.
- Answer
-
True. The only difference between the two sets is that the set of whole numbers contains the number 0.
All whole numbers are rational numbers.
All rational numbers are integers.
- Answer
-
False. For example,
All rational numbers are whole numbers.
Some natural numbers are irrational.
- Answer
-
False. All natural numbers are rational, and therefore not irrational.
Some whole numbers are irrational.
Some real numbers are irrational.
- Answer
-
True. For example, π and √2 are real numbers which are irrational.
All integers are real numbers.
All integers are rational numbers.
- Answer
-
True. Every integer b can be written as a fraction b/1.
No rational numbers are natural numbers.
No real numbers are integers.
- Answer
-
False. For example, 2 is a real number that is also an integer.
All whole numbers are natural numbers.
In Exercises
45x + 12 = 0
- Answer
-
76x − 55 = 0
x − 7 = −6x + 4
- Answer
-
−26x + 84 = 48
37x + 39 = 0
- Answer
-
−48x + 95 = 0
74x − 6 = 91
- Answer
-
−7x + 4 = −6
−88x + 13 = −21
- Answer
-
−14x − 81 = 0
19x + 35 = 10
- Answer
-
−2x + 3 = −5x − 2
In Exercises
6 − 3(x + 1) = −4(x + 6) + 2
- Answer
-
(8x + 3) − (2x + 6) = −5x + 8
−7 − (5x − 3) = 4(7x + 2)
−3 − 4(x + 1) = 2(x + 4) + 8
9 − (6x − 8) = −8(6x − 8)
- Answer
-
−9 − (7x − 9) = −2(−3x + 1)
(3x − 1) − (7x − 9) = −2x − 6
- Answer
-
−8 − 8(x − 3) = 5(x + 9) + 7
(7x − 9) − (9x + 4) = −3x + 2
- Answer
-
(−4x − 6) + (−9x + 5) = 0
−5 − (9x + 4) = 8(−7x − 7)
- Answer
-
(8x − 3) + (−3x + 9) = −4x − 7
In Exercises
−3.7x − 1 = 8.2x − 5
- Answer
-
First clear decimals by multiplying by 10.
Here is a check of the solutions on the graphing calculator. The left-hand side of the equation is evaluated at the solution in (a), the right-hand side of the equation is evaluated at the solution in (b). Note that they match.

8.48x − 2.6 = −7.17x − 7.1
- Answer
-
First clear fractions by multiplying by 15.
Here is a check of the solutions on the graphing calculator. The left-hand side of the equation is evaluated at the solution in (a), the right-hand side of the equation is evaluated at the solution in (b). Note that they match.
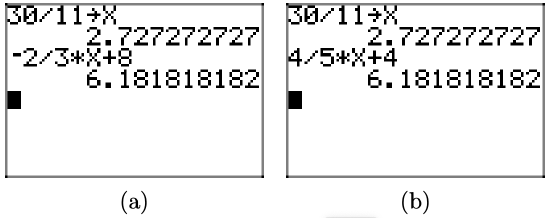
−8.4x = −4.8x + 2
- Answer
-
First clear fractions by multiplying by 4.
Here is a check of the solutions on the graphing calculator. The left-hand side of the equation is evaluated at the solution in (a), the right-hand side of the equation is evaluated at the solution in (b). Note that they match.

2.9x − 4 = 0.3x − 8
5.45x + 4.4 = 1.12x + 1.6
- Answer
-
First clear decimals by multiplying by 100.
Here is a check of the solutions on the graphing calculator. The left-hand side of the equation is evaluated at the solution in (a), the right-hand side of the equation is evaluated at the solution in (b). Note that they match.

- Answer
-
First clear fractions by multiplying by 10.
Here is a check of the solutions on the graphing calculator. The left-hand side of the equation is evaluated at the solution in (a), the right-hand side of the equation is evaluated at the solution in (b). Note that they match.

−4.34x − 5.3 = 5.45x − 8.1
- Answer
-
First clear decimals by multiplying by 100.
Here is a check of the solutions on the graphing calculator. The left-hand side of the equation is evaluated at the solution in (a), the right-hand side of the equation is evaluated at the solution in (b). Note that they match.

In Exercises
P = IRT for R
- Answer
-
d = vt for t
- Answer
-
Ax + By = C for y
- Answer
-
y = mx + b for x
- Answer
-
- Answer
-
- Answer
-
- Answer
-
Cross multiply, then divide by the coefficient of
Tie a ball to a string and whirl it around in a circle with constant speed. It is known that the acceleration of the ball is directly toward the center of the circle and given by the formula
i. Solve formula (1) for r.
ii. Given that the acceleration of the ball is 12 m/s2 and the speed is 8 m/s, find the radius of the circle of motion.
- Answer
-
Cross multiply, then divide by the coefficient of r.
To find the radius, substitute the acceleration
Hence, the radius is
A particle moves along a line with constant acceleration. It is known the velocity of the particle, as a function of the amount of time that has passed, is given by the equation
i. Solve formula (2) for t.
ii. You know that the current velocity of the particle is 120 m/s. You also know that the initial velocity was 40 m/s and the acceleration has been a constant
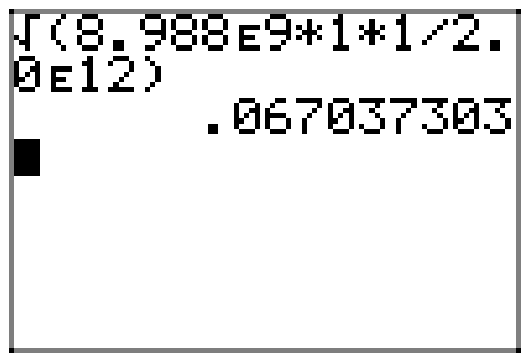
Like Newton’s Universal Law of Gravitation, the force of attraction (repulsion) between two unlike (like) charged particles is proportional to the product of the charges and inversely proportional to the distance between them.
i. Solve formula (3) for r.
ii. Given a force
- Answer
-
Cross multiply, then divide by the coefficient of r.
Finally, to find r, take the square root.
To find the distance between the charged particles, substitute
A calculator produces an approximation,
Perform each of the following tasks in Exercises
i. Write out in words the meaning of the symbols which are written in set-builder notation.
ii. Write some of the elements of this set.
iii. Draw a real line and plot some of the points that are in this set.
- Answer
-
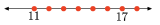
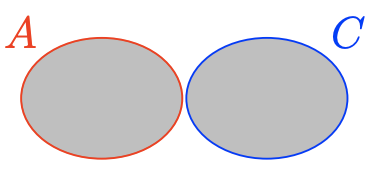
i. A is the set of all
ii.
iii.
- Answer
-
i. C is the set of all
ii.
iii.

In Exercises
- Answer
-
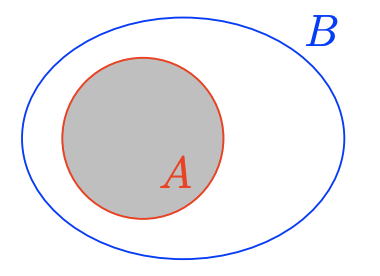
- Answer
-
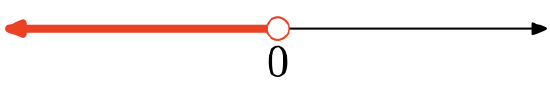
In Exercises

- Answer
-
The filled circle at the endpoint 3 indicates this point is included in the set. Thus, the set in interval notation is
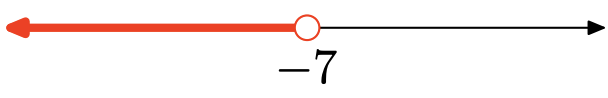
- Answer
-
The empty circle at the endpoint −7 indicates this point is not included in the set. Thus, the set in interval notation is
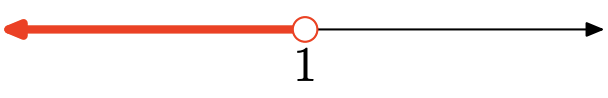
- Answer
-
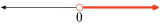
The empty circle at the endpoint 0 indicates this point is not included in the set. Thus, the set in interval notation is


- Answer
-
The empty circle at the endpoint −8 indicates this point is not included in the set. Thus, the set in interval notation is
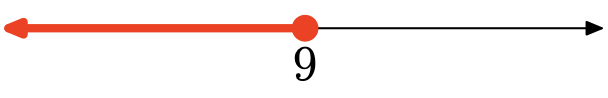
In Exercises
- Answer
-

- Answer
-

- Answer
-

- Answer
-

In Exercises
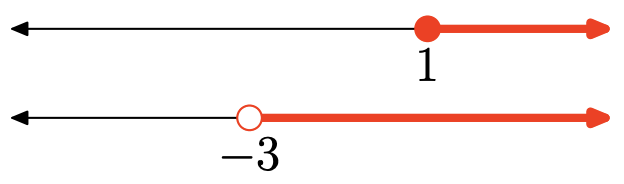
- Answer
-
The intersection is the set of points that are in both intervals (shaded on both graphs). Graph of the intersection:

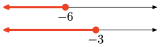

- Answer
-
There are no points that are in both intervals (shaded in both), so there is no intersection. Graph of the intersection:

no intersection
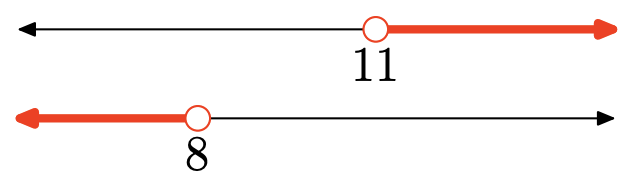
- Answer
-
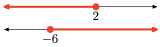
The intersection is the set of points that are in both intervals (shaded in both). Graph of the intersection:


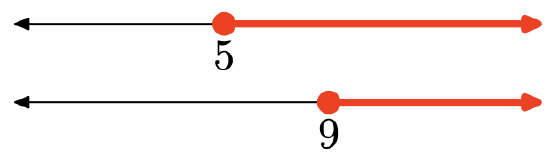
- Answer
-
The intersection is the set of points that are in both intervals (shaded in both). Graph of the intersection:

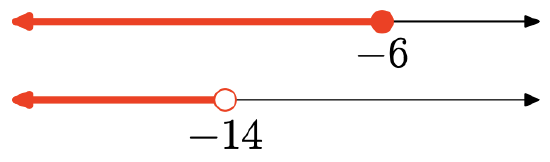
In Exercises
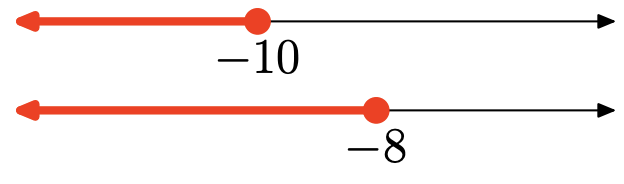
- Answer
-
The union is the set of all points that are in one interval or the other (shaded in either graph). Graph of the union:


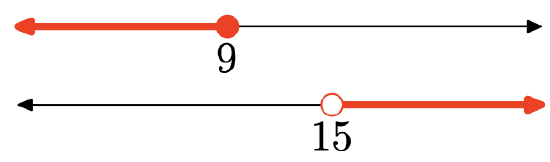
- Answer
-
The union is the set of all points that are in one interval or the other (shaded in either graph). Graph of the union:

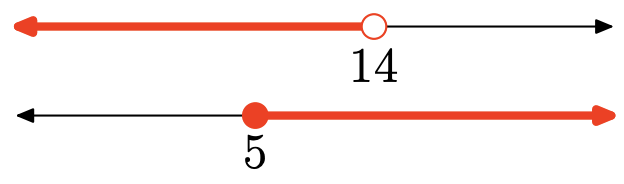
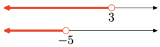
- Answer
-
The union is the set of all points that are in one interval or the other (shaded in either). Graph of the union:



- Answer
-
The union is the set of all points that are in one interval or the other (shaded in either). Graph of the union:


In Exercises
- Answer
-
This set is the same as

- Answer
-
Every real number is in one or the other of the two intervals. Therefore, the set is the set of all real numbers

- Answer
-
This set is the same as

- Answer
-
This set is the same as

- Answer
-
This set is the same as

- Answer
-
This set is the union of two intervals,

- Answer
-
There are no numbers that satisfy both inequalities. Thus, there is no intersection. Graph of the set:

- Answer
-
This set is the same as

In Exercises
- Answer
-
Thus, the solution interval is
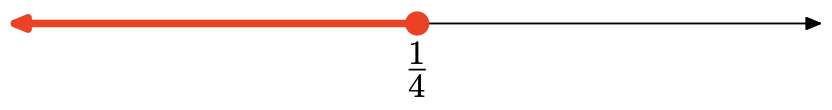
- Answer
-
Thus, the solution interval is
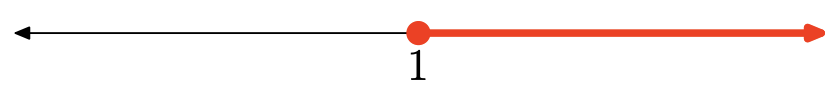
- Answer
-
Thus, the solution interval is
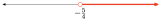
- Answer
-
Thus, the solution interval is
- Answer
-
Thus, the solution interval is
- Answer
-
Thus, the solution interval is
In Exercises 13-50, solve the compound inequality. Express your answer in both interval and set notations, and shade the solution on a number line.
- Answer
-

For the union, shade anything shaded in either graph. The solution is the set of all real numbers

- Answer
-
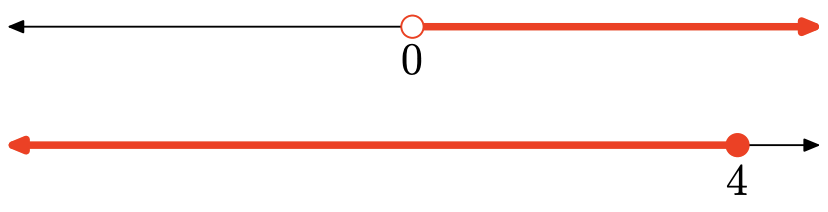
The intersection is all points shaded in both graphs, so the solution is
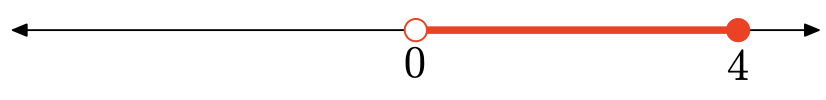
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
In Exercises 51-62, solve the given inequality for x. Graph the solution set on a number line, then use interval and set-builder notation to describe the solution set.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
- Answer
-
Add texts here. Do not delete this text first.
Aeron has arranged for a demonstration of “How to make a Comet” by Professor O’Commel. The wise professor has asked Aeron to make sure the auditorium stays between 15 and 20 degrees Celsius (C). Aeron knows the thermostat is in Fahrenheit (F) and he also knows that the conversion formula between the two temperature scales is C = (5/9)(F − 32).
a) Setting up the compound inequality for the requested temperature range in Celsius, we get
b) Solve the compound inequality in part (a) for F. Write your answer in set notation.
c) What are the possible temperatures (integers only) that Aeron can set the thermostat to in Fahrenheit?
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.
Add exercises text here.
- Answer
-
Add texts here. Do not delete this text first.

