1.5E: Exercises

Last updated
Save as PDF

Page ID: 30291

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$ \newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\id}{\mathrm{id}}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\kernel}{\mathrm{null}\,}$

$ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$

$ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$

$ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\AA}{\unicode[.8,0]{x212B}}$

$ \newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$ \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$ \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vectorC}[1]{\textbf{#1}} $

$ \newcommand{\vectorD}[1]{\overrightarrow{#1}} $

$ \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} $

$ \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} $

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$\newcommand{\avec}{\mathbf a}$ $\newcommand{\bvec}{\mathbf b}$ $\newcommand{\cvec}{\mathbf c}$ $\newcommand{\dvec}{\mathbf d}$ $\newcommand{\dtil}{\widetilde{\mathbf d}}$ $\newcommand{\evec}{\mathbf e}$ $\newcommand{\fvec}{\mathbf f}$ $\newcommand{\nvec}{\mathbf n}$ $\newcommand{\pvec}{\mathbf p}$ $\newcommand{\qvec}{\mathbf q}$ $\newcommand{\svec}{\mathbf s}$ $\newcommand{\tvec}{\mathbf t}$ $\newcommand{\uvec}{\mathbf u}$ $\newcommand{\vvec}{\mathbf v}$ $\newcommand{\wvec}{\mathbf w}$ $\newcommand{\xvec}{\mathbf x}$ $\newcommand{\yvec}{\mathbf y}$ $\newcommand{\zvec}{\mathbf z}$ $\newcommand{\rvec}{\mathbf r}$ $\newcommand{\mvec}{\mathbf m}$ $\newcommand{\zerovec}{\mathbf 0}$ $\newcommand{\onevec}{\mathbf 1}$ $\newcommand{\real}{\mathbb R}$ $\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$ $\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$ $\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$ $\newcommand{\laspan}[1]{\text{Span}\{#1\}}$ $\newcommand{\bcal}{\cal B}$ $\newcommand{\ccal}{\cal C}$ $\newcommand{\scal}{\cal S}$ $\newcommand{\wcal}{\cal W}$ $\newcommand{\ecal}{\cal E}$ $\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$ $\newcommand{\gray}[1]{\color{gray}{#1}}$ $\newcommand{\lgray}[1]{\color{lightgray}{#1}}$ $\newcommand{\rank}{\operatorname{rank}}$ $\newcommand{\row}{\text{Row}}$ $\newcommand{\col}{\text{Col}}$ $\renewcommand{\row}{\text{Row}}$ $\newcommand{\nul}{\text{Nul}}$ $\newcommand{\var}{\text{Var}}$ $\newcommand{\corr}{\text{corr}}$ $\newcommand{\len}[1]{\left|#1\right|}$ $\newcommand{\bbar}{\overline{\bvec}}$ $\newcommand{\bhat}{\widehat{\bvec}}$ $\newcommand{\bperp}{\bvec^\perp}$ $\newcommand{\xhat}{\widehat{\xvec}}$ $\newcommand{\vhat}{\widehat{\vvec}}$ $\newcommand{\uhat}{\widehat{\uvec}}$ $\newcommand{\what}{\widehat{\wvec}}$ $\newcommand{\Sighat}{\widehat{\Sigma}}$ $\newcommand{\lt}{<}$ $\newcommand{\gt}{>}$ $\newcommand{\amp}{&}$ $\definecolor{fillinmathshade}{gray}{0.9}$

Practice Makes Perfect

Round Decimals

In the following exercises, round each number to the nearest ⓐ hundredth ⓑ tenth ⓒ whole number.

1. $5.781$

Answer: ⓐ $5.78$ ⓑ $5.8$ ⓒ $6$

2. $1.638$

3. $0.299$

Answer: ⓐ $0.30$ ⓑ $0.3$ ⓒ $0$

4. $0.697$

5. $63.479$

Answer: ⓐ $63.48$ ⓑ $63.5$ ⓒ $63$

6. $84.281$

Add and Subtract Decimals

In the following exercises, add or subtract.

7. $−16.53−24.38$

Answer: $−40.91$

8. $−19.47−32.58$

9. $−38.69+31.47$

Answer: $−7.22$

10. $−29.83+19.76$

11. $72.5-100$

Answer: $-27.5$

12. $86.2-100$

13. $91.75−(−10.462)$

Answer: $02.212$

14. $94.69−(−12.678)$

15. $55.01−3.7$

Answer: $51.31$

16. $59.08−4.6$

17. $2.51−7.4$

Answer: $−4.89$

18. $3.84−6.1$

Multiply and Divide Decimals

In the following exercises, multiply.

19. $94.69−(−12.678)$

Answer: $−11.653$

20. $(−8.5)(1.69)$

21. $(−5.18)(−65.23)$

Answer: $337.8914$

22. $(−9.16)(−68.34)$

23. $(0.06)(21.75)$

Answer: $1.305$

24. $(0.08)(52.45)$

25. $(9.24)(10)$

Answer: $92.4$

26. $(6.531)(10)$

27. $(0.025)(100)$

Answer: $2.5$

28. $(0.037)(100)$

29. $(55.2)(1000)$

Answer: $55200$

30. $(99.4)(1000)$

In the following exercises, divide. Round money monetary answers to the nearest cent.

31. $$117.25÷48$

Answer: $$2.44$

32. $$109.24÷36$

33. $1.44÷(−0.3)$

Answer: $−4.8$

34. $−1.15÷(−0.05)$

35. $5.2÷2.5$

Answer: $2.08$

36. $14÷0.35$

Convert Decimals, Fractions and Percents

In the following exercises, write each decimal as a fraction.

37. $0.04$

Answer: $\frac{1}{25}$

38. $1.464$

39. $0.095$

Answer: $\frac{19}{200}$

40. $−0.375$

In the following exercises, convert each fraction to a decimal.

41. $\frac{17}{20}$

Answer: $0.85$

42. $\frac{17}{4}$

43. $−\frac{310}{25}$

Answer: $−12.4$

44. $−\frac{18}{11}$

In the following exercises, convert each percent to a decimal.

45. $71 \%$

Answer: $0.71$

46. $150 \%$

47. $39.3 \% $

Answer: $0.393$

48. $7.8 \% $

In the following exercises, convert each decimal to a percent.

49. $1.56$

Answer: $156 \% $

50. $3$

51. $0.0625$

Answer: $6.25 \% $

52. $2.254$

Simplify Expressions with Square Roots

In the following exercises, simplify.

53. $\sqrt{64}$

Answer: $8$

54. $\sqrt{169}$

55. $\sqrt{144}$

Answer: $12$

56. $−\sqrt{4}$

57. $−\sqrt{100}$

Answer: $−10$

58. $−\sqrt{121}$

Identify Integers, Rational Numbers, Irrational Numbers, and Real Numbers

In the following exercises, list the ⓐ whole numbers, ⓑ integers, ⓒ rational numbers, ⓓ irrational numbers, ⓔ real numbers for each set of numbers.

59. $−8,0,1.95286...,\frac{12}{5},\sqrt{36},9$

Answer: ⓐ Whole numbers: $0,\sqrt{36},9$
ⓑ Integers: $−8,0,\sqrt{36},9$
ⓒ Rational numbers: $−8,0,\sqrt{36},9$
ⓓ Irrational numbers: $1.95286...,$
ⓔ Real numbers: $−8,0,1.95286...,\frac{12}{5},\sqrt{36},9$

60. $−9,−3\frac{4}{9},−\sqrt{9},0.40 \overline{9},\frac{11}{6},7$

61. $−\sqrt{100},−7,−\frac{8}{3},−1,0.77,3\frac{1}{4}$

Answer: ⓐ Whole numbers: none
ⓑ Integers: $−\sqrt{100},−7,−1$
ⓒ Rational numbers: $−\sqrt{100},−7,−\frac{8}{3},−1,0.77,3\frac{1}{4}$
ⓓ Irrational numbers: none
ⓔ Real numbers: $−\sqrt{100},−7,−\frac{8}{3},−1,0.77,3\frac{1}{4}$

62. $−6,−\frac{5}{2},0,0. \overline{714285},2\frac{1}{5},\sqrt{14}$

Locate Fractions and Decimals on the Number Line

In the following exercises, locate the numbers on a number line.

63. $\frac{3}{10},\frac{7}{2},\frac{11}{6},4$

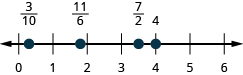
Answer: $Figure shows a number line with numbers ranging from 0 to 6. Some values are highlighted. From left to right, these are: 3 by 10, 11 by 6, 7 by 2 and 4.$

64. $\frac{7}{10},\frac{5}{2},\frac{13}{8},3$

65. $\frac{3}{4},−\frac{3}{4},1\frac{2}{3},−1\frac{2}{3},\frac{5}{2},−\frac{5}{2}$

Answer: $Figure shows a number line with numbers ranging from minus 4 to 4. Some values are highlighted. From left to right, these are: minus 5 by 2, minus 1 and two thirds, minus 3 by 4, 3 by 4, 1 and two thirds, and 5 by 2.$

66. $\frac{2}{5},−\frac{2}{5},1\frac{3}{4},−1\frac{3}{4},\frac{8}{3},−\frac{8}{3}$

67. ⓐ $0.8$ ⓑ $−1.25$

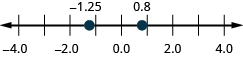
Answer: $Figure shows a number line with numbers ranging from minus 4 to 4. Two values are highlighted. One is between minus 2 and minus 1. The other is between 0 and 1.$

68. ⓐ $−0.9$ ⓑ $−2.75$

69. ⓐ $−1.6$ ⓑ $3.25$

Answer: $Figure shows a number line with numbers ranging from minus 4 to 4. Two values are highlighted. One is between minus 2 and minus 1. The other is between 3 and 4.$

70. ⓐ $3.1$ ⓑ $−3.65$

Writing Exercises

71. How does knowing about U.S. money help you learn about decimals?

Answer: Answers will vary.

72. When the Szetos sold their home, the selling price was 500% of what they had paid for the house 30 years ago. Explain what 500% means in this context.Szetos sold their home, the selling price was 500% of what they had paid for the house 30 years ago. Explain what 500% means in this context.

73. In your own words, explain the difference between a rational number and an irrational number.

Answer: Answers will vary.

74. Explain how the sets of numbers (counting, whole, integer, rational, irrationals, reals) are related to each other.

Self Check

ⓐ Use this checklist to evaluate your mastery of the objectives of this section.

$This table has 4 columns, 6 rows and a header row. The header row labels each column: I can, confidently, with some help and no, I don’t get it. The statements in the first column are: round decimals, add and subtract decimals, multiply and divide decimals, convert decimals, fractions and percents, simplify expressions with square roots, identify integers, rational numbers, irrational numbers and real numbers, locate fractions and decimals on the number line. The remaining columns are blank.$

ⓑ On a scale of 1-10, how would you rate your mastery of this section in light of your responses on the checklist? How can you improve this?

Search

Text Color

Text Size

Margin Size

Font Type