9.4: Measuring Volume

Figure 9.8 Packing cartons sit on a loading dock ready to be filled. (credit: “boxing day” by Erich Ferdinand/Flickr, CC BY 2.0)

Learning Objectives

After completing this section, you should be able to:

1. Identify reasonable values for volume applications.
2. Convert between like units of measures of volume.
3. Convert between different unit values.
4. Solve application problems involving volume.

Volume is a measure of the space contained within or occupied by three-dimensional objects. It could be a box, a pool, a storage unit, or any other three-dimensional object with attributes that can be measured in the metric unit for distance–meters. For example, when purchasing an SUV, you may want to compare how many cubic units of cargo the SUV can hold.

Cubic units indicate that three measures in the same units have been multiplied together. For example, to find the volume of a rectangular prism, you would multiply the length units by the width units and the height units to determine the volume in square units:

$$1\mathrm{cm}\times 1\mathrm{cm}\times 1\mathrm{cm}=1$$

Note that to accurately calculate volume, each of the measures being multiplied must be of the same units. For example, to find a volume in cubic centimeters, each of the measures must be in centimeters.

FORMULA

The formula used to determine volume depends on the shape of the three-dimensional object. Here we will limit our discussions to the area to rectangular prisms like the one in Figure 9.9 Given this limitation, the basic formula for volume is:

$$\text{Volume}=\text{length}\left(l\right)\times \text{width}\left(w\right)\times \text{height}(h)$$

$$\begin{array}{c}\text{or}\\ V\\ =\end{array}$$

Figure 9.9 Rectangular Prism with Height $\left(h\right)$, Length $\left(l\right)$, and Width $\left(w\right)$ Labeled.

Reasonable Values for Volume

Because volume is determined by multiplying three lengths, the magnitude of difference between different cubic units is exponential. In other words, while a meter is 100 times greater in length than a centimeter, a cubic meter, m³, is $100\times 100\times 100,\mathrm{or}\mathrm{1,000,000}$ times greater in area than a cubic centimeter, cm³. This relationship between benchmark metric volume units is shown in the following table.

Units	Relationship	Conversion Rate
km^3 to m^3	$\mathrm{km}\times \mathrm{km}\times \mathrm{km}=$ $1\mathrm{km}=\mathrm{1,000}\mathrm{m}$ $\mathrm{1,000}\mathrm{m}\times \mathrm{1,000}\mathrm{m}\times \mathrm{1,000}\mathrm{m}=\mathrm{1,000,000,000}{\mathrm{m}}^3$	1 km^3 = 1,000,000,000 m^3
m^3 to dm^3	$\mathrm{m}\times \mathrm{m}\times \mathrm{m}={\mathrm{m}}^3$ $1\mathrm{m}=10\mathrm{dm}$ $10\mathrm{dm}\times 10\mathrm{dm}\times 10\mathrm{dm}=\mathrm{1,000}{\mathrm{dm}}^3$	1 m^3 = 1,000 dm^3
dm^3 to cm^3	$\mathrm{dm}\times \mathrm{dm}\times \mathrm{dm}={\mathrm{dm}}^3$ $1\mathrm{dm}=10\mathrm{cm}$ $10\mathrm{cm}\times 10\mathrm{cm}\times 10\mathrm{cm}=\mathrm{1,000,000}{\mathrm{cm}}^3$	1 cm^3 = 1,000 dm^3
cm^3 to mm^3	$\mathrm{cm}\times \mathrm{cm}\times \mathrm{cm}={\mathrm{cm}}^3$ $1\mathrm{cm}=10\mathrm{mm}$ $10\mathrm{mm}\times 10\mathrm{mm}\times 10\mathrm{mm}=\mathrm{1,000}{\mathrm{mm}}^3$	1 cm^3 = 1,000 mm^3

To have an essential understanding of metric volume, you must be able to identify reasonable values for volume. When testing for reasonableness you should assess both the unit and the unit value. Only by examining both can you determine whether the given volume is reasonable for the situation.

Example 9.19

Determining Reasonable Values for Volume

A grandparent wants to send cookies to their grandchild away at college. Which represents a reasonable value for the volume of a box to ship the cookies:

• 3,375 km³,
• 3,375 m³, or
• 3,375 cm³?

Answer

A volume of 3,375 km³ is equivalent to a rectangular prism with dimensions of $15\mathrm{km}\times 15\mathrm{km}\times 15\mathrm{km},$ which is far too large for a shipping box. An area of 3,375 m³ is equivalent to a surface of $15\mathrm{m}\times 15\mathrm{m}\times 15\mathrm{m},$ which is also too large. A reasonable value for the volume of the box is 3,375 cm³.

Your Turn 9.19

1.

Which represents a reasonable value for the volume of a storage area:
8 m³, 8 cm³, or 8 mm³?

Example 9.20

Identifying Reasonable Values for Volume

A food manufacturer is prototyping new packaging for one of its most popular products. Which represents a reasonable value for the volume of the box:

• 2 dm³,
• 2 cm³, or
• 2 mm³?

Answer

A decimeter is equal to 10 centimeters. A box with a volume of 2 dm³ might have the dimensions $1\mathrm{dm}\times 1\mathrm{dm}\times 2\mathrm{dm}$, or $10\mathrm{cm}\times 10\mathrm{cm}\times 20\mathrm{cm}$, which is reasonable. A box with a volume of 2 cm³ or 2 mm³ would be too small.

Your Turn 9.20

1.

Which represents a reasonable value for the volume of a fish tank:
40,000 mm³, 40,000 cm³, or 40,000 m³?

Example 9.21

Explaining Reasonable Values for Volume

A farmer has a hay loft. They calculate the volume of the hayloft as 64 cm³. Does the calculation make sense? Explain your answer.

Answer

No. Centimeters are used to determine smaller distances, such as the length of a pencil. A hayloft is more than 64 centimeters long, so a more reasonable unit of value would be m². A volume of 64 m³ can be calculated using the dimensions 4 meters by 4 meters by 4 meters, which are reasonable dimensions for a hayloft. So, a more reasonable value for the volume of the hayloft is 64 m³.

Your Turn 9.21

1.

An artist creates a glass paperweight. They decide they want to box and ship the paperweight, so they measure and determine that the volume of the cubic box is 125,000 mm³. Does their calculation make sense? Explain your answer.

Converting Like Units of Measures for Volume

Just like converting units of measure for distance, you can convert units of measure for volume. However, the conversion factor, the number used to multiply or divide to convert from one volume unit to another, is different from the conversion factor for metric distance units. Recall that the conversion factor for volume is exponentially relative to the conversion factor for distance. The most frequently used conversion factors are illustrated in Figure 9.10.

Figure 9.10 Common Conversion Factors for Metric Volume Units

Example 9.22

Converting Like Units of Measure for Volume Using Multiplication

A pencil case has a volume of 1,700 cm³. What is the volume in cubic millimeters?

Answer

Use multiplication to convert from a larger metric volume unit to a smaller metric volume unit. To convert from cm³ to mm³, multiply the value of the volume by 1,000.

$$\mathrm{1,700}\times \mathrm{1,000}=\mathrm{1,700,000}$$

The pencil case has a volume of 1,700,000 mm³.

Your Turn 9.22

1.

A jewelry box has a volume of 8 cm³. What is the volume of the jewelry box in cubic millimeters?

Video

How to Convert Cubic Centimeters to Cubic Meters

Example 9.23

Converting Like Units of Measure for Volume Using Multi-Step Multiplication

A shipping container has a volume of 33.2 m³. What is the volume in cubic centimeters?

Answer

Use multiplication to convert a larger metric volume unit to a smaller metric volume unit. To convert from m³ to cm³, first multiply the value of the volume by 1,000 to convert from m³ to dm³, and then multiply again by 1,000 to convert from dm³ to cm³.

$$33.2\times \mathrm{1,000}\times \mathrm{1,000}=\mathrm{33,200,000}$$

The shipping container has a volume of 32,200,000 cm³.

Your Turn 9.23

1.

A gasoline storage tank has a volume of 37.854 m³. What is the volume of the storage tank in cubic centimeters?

Example 9.24

Converting Like Units of Measure for Volume Using Multi-Step Division

A holding tank at the local aquarium has a volume of 22,712,000,000 cm³. What is the volume in cubic meters?

Answer

Figure 9.10 indicates that when converting from a smaller metric volume unit to a larger metric volume unit you divide using the given conversion factor. To convert from cm³ to m³, divide the value of the volume by 1,000 to first convert from cm³ to dm³, then divide again to convert from dm³ to m³.

$$\begin{array}{c}{\mathrm{cm}}^3{\mathrm{to\; dm}}^3:\frac{\mathrm{22,712,000,000}}{\mathrm{1,000}}=\mathrm{22,712,000}\\ {\mathrm{dm}}^3{\mathrm{to\; m}}^3:\frac{\mathrm{22,712,000}}{\mathrm{1,000}}=\mathrm{22,712}\end{array}$$

The holding tank has a volume of 22,712 m³.

Your Turn 9.24

1.

A warehouse has a volume of 465,000,000 cm³. What is the volume of the warehouse in cubic meters?

Understanding Other Metric Units of Volume

When was the last time you purchased a bottle of soda? Was the volume of the bottle expressed in cubic centimeters or liters? The liter (L) is a metric unit of capacity often used to express the volume of liquids. A liter is equal in volume to 1 cubic decimeter. A milliliter is equal in volume to 1 cubic centimeter. So, when a doctor orders 10 cc (cubic centimeters) of saline to be administered to a patient, they are referring to 10 mL of saline.

The most frequently used factors for converting from cubic meters to liters are listed in Table 9.2.

m^3 to L	m^3 to mL
$1{\mathrm{dm}}^3=1\mathrm{L}$	$1{\mathrm{dm}}^3=\mathrm{1,000}\mathrm{mL}$
$\mathrm{1,000}{\mathrm{cm}}^3=1\mathrm{L}$	$1{\mathrm{cm}}^3=1\mathrm{mL}$
$\mathrm{1,000,000}{\mathrm{mm}}^3=1\mathrm{L}$	$1{\mathrm{mm}}^3=0.001\mathrm{mL}$

Table 9.2 Relationships Between Metric Volume and Metric Capacity Units

Video

Converting Metric Units of Volume

Example 9.25

Converting Different Units of Measure for Volume

A holding tank at the local aquarium has a volume of 22,712,000,000 cm³? What is the capacity of the holding tank in liters?

Answer

Use division to convert from cubic centimeters to liters. To determine the equivalent volume in liters, convert from cm³ to L by dividing the value of the volume in cm³ by 1,000.

$$\frac{\mathrm{22,712,000,000}{\mathrm{cm}}^3}{\mathrm{1,000}}=\mathrm{22,712,000}\mathrm{L}$$

The holding tank holds 22,712,000 L of water.

Your Turn 9.25

1.

A gas can has a volume of 19,000 cm³. How much gas, in liters, does the gas can hold?

Example 9.26

Converting Different Units of Measure for Volume Using Multiplication

An airplane used 150 m³ of fuel to fly from New York to Hawaii. How many liters of fuel did the airplane use?

Answer

Because 1 liter is equivalent to 1 cubic decimeter, use multiplication to convert from m³ to dm³. Multiply the value of the volume by 1,000 to convert from m³ to dm³. Because $1{\mathrm{dm}}^3=1\mathrm{L}$, the resulting value is equivalent to the number of liters used.

$$150\times \mathrm{1,000}=\mathrm{150,000}{\mathrm{dm}}^3=\mathrm{150,000}\mathrm{L}$$

The airplane used 150,000 liters of fuel.

Your Turn 9.26

1.

A gasoline storage tank has a volume of 37.854 m³. What is the volume of the storage tank in liters?

Example 9.27

Converting Different Units of Measure for Volume Using Multi-Step Division

How many liters can a pitcher with a volume of 8,000,000 mm³ hold?

Answer

Use division to convert from a smaller metric volume unit to a larger metric volume unit. To convert from mm³ to dm³,

Step 1: Divide by 1,000 to convert from mm³ to cm³.

Step 2: Divide again by 1,000 to convert from cm³ to dm³.

Step 3: Use the unit value to express the volume in terms of liters.

$$\begin{array}{ccc}\hfill \frac{\mathrm{8,000,000}{\mathrm{mm}}^3}{\mathrm{1,000}}& \hfill =\hfill & \mathrm{8,000}{\mathrm{cm}}^3\hfill \\ \hfill \frac{\mathrm{8,000}{\mathrm{cm}}^3}{\mathrm{1,000}}& \hfill =\hfill & 8{\mathrm{dm}}^3=8\mathrm{L}\hfill \end{array}$$

The pitcher can hold 8 liters of liquid.

Your Turn 9.27

1.

A glass jar has a volume of 800,000 mm³. How many mL of liquid can the glass jar hold?

Solving Application Problems Involving Volume

Knowing the volume of an object lets you know just how much that object can hold. When making a bowl of punch you might want to know the total amount of liquid a punch bowl can hold. Knowing how many liters of gasoline a car’s tank can hold helps determine how many miles a car can drive on a full tank. Regardless of the application, understanding volume is essential to many every day and professional tasks.

Example 9.28

Using Volume to Solve Problems

A cubic shipping carton’s dimensions measure $2\mathrm{m}\times 2\mathrm{m}\times 2\mathrm{m}$. A company wants to fill the carton with smaller cubic boxes that measure $10\mathrm{cm}\times 10\mathrm{cm}\times 10\mathrm{cm}$. How many of the smaller boxes will fit in each shipping carton?

Answer

Step 1: Determine the volume of the shipping carton.

$$2\mathrm{m}\times 2\mathrm{m}\times 2\mathrm{m}=8{\mathrm{m}}^3$$

Step 2: Use the appropriate conversion factor to convert the volume of the shipping carton from m³ to cm³.

$$\begin{array}{c}8{\mathrm{m}}^3\times \mathrm{1,000}=\mathrm{8,000}{\mathrm{dm}}^3\\ \mathrm{8,000}{\mathrm{dm}}^3\times \mathrm{1,000}=\mathrm{8,000,000}{\mathrm{cm}}^3\end{array}$$

Step 3: Determine the volume of the smaller boxes.

$$10\mathrm{cm}\times 10\mathrm{cm}\times 10\mathrm{cm}=\mathrm{1,000}{\mathrm{cm}}^3$$

Step 4: Divide the volume of the shipping carton, in cm³, by the volume of the smaller box, in cm³.

$$\frac{\mathrm{8,000,000}{\mathrm{cm}}^3}{\mathrm{1,000}{\mathrm{cm}}^3}=\mathrm{8,000}$$

The shipping carton will hold 8,000 smaller boxes.

Your Turn 9.28

1.

A factory can mill 300 cubic meters of flour each day. They package the flour in boxes that measure /**/20\,{\text{cm}} \times 5\,{\text{cm}} \times 30\,{\text{cm}}/**/. How many boxes of flour does the factory produce each day?

Example 9.29

Solving Volume Problems with Different Units

A carton of juice measures 6 cm long, 6 cm wide and 20 cm tall. A factory produces 28,800 liters of orange juice each day. How many cartons of orange juice are produced each day?

Answer

Step 1: Find the volume of the carton in cubic centimeters.

$$6\mathrm{cm}\times 6\mathrm{cm}\times 20\mathrm{cm}=720{\mathrm{cm}}^3$$

Step 2: Convert the volume in cm³ to liters.

$$\begin{array}{ccc}\hfill 1{\mathrm{cm}}^3& \hfill =\hfill & 1\mathrm{mL}\hfill \\ \hfill 720{\mathrm{cm}}^3& \hfill =\hfill & 720\mathrm{mL}\hfill \\ \hfill \frac{720\mathrm{mL}}{\mathrm{1,000}}& \hfill =\hfill & 0.72\mathrm{L}\hfill \end{array}$$

Step 3: Divide the number of liters of orange juice produced each day by the volume of each carton.

$$\frac{\mathrm{28,800}\mathrm{L}}{0.72\mathrm{L}}=\mathrm{40,000}$$

The factory produces 40,000 cartons of orange juice each day.

Your Turn 9.29

1.

An ice cream maker boxes frozen yogurt mix in boxes that measure 25 cm long, 8 cm wide and 35 cm tall. They produce 42,000 liters of frozen yogurt mix each day. How many boxes of frozen yogurt mix are produced each day?

Example 9.30

Solving Complex Volume Problems

A fish tank measures 60 cm long, 15 cm wide and 34 cm tall (Figure 9.11). The tank is 25 percent full. How many liters of water are needed to completely fill the tank?

Figure 9.11

Answer

Step 1: Determine the volume of the fish tank in cubic centimeters.

$$60\mathrm{cm}\times 15\mathrm{cm}\times 34\mathrm{cm}=\mathrm{30,600}{\mathrm{cm}}^3$$

Step 2: Convert the volume in cm³ to volume in liters.

$$\begin{array}{ccc}\hfill 1{\mathrm{cm}}^3& \hfill =\hfill & 1\mathrm{mL}\hfill \\ \hfill \mathrm{30,600}{\mathrm{cm}}^3& \hfill =\hfill & \mathrm{30,600}\mathrm{mL}\hfill \\ \hfill \frac{\mathrm{30,600}\mathrm{mL}}{\mathrm{1,000}}& \hfill =\hfill & 30.6\mathrm{L}\hfill \end{array}$$

Step 3: Since the tank is 25 percent full, the tank is 75 percent empty. Convert 75 percent to its decimal equivalent. Multiply the total volume by 75 percent expressed in decimal form to determine how many liters of water are required to fill the tank.

$$\begin{array}{ccc}\hfill 75\%& \hfill =\hfill & 0.75\hfill \\ \hfill 30.6\times 0.75& \hfill =\hfill & 22.95\hfill \end{array}$$

So, 22.95 liters of water are needed to fill the tank.

Your Turn 9.30

1.

A fish tank measures 75 cm long, 20 cm wide and 25 cm tall. The tank is 50 percent full. How many liters of water are needed to completely fill the tank?

Figure 9.12

WORK IT OUT

How Does Shape Affect Volume?

Take two large sheets of card stock. Roll one piece to tape the longer edges together to make a cylinder. Tape the cylinder to the other piece of card stock which serves as the base of the cylinder. Fill the cylinder to the top with cereal. Pour the cereal from the cylinder into a plastic storage or shopping bag. Remove the cylinder from the base and the tape from the cylinder. Re-roll the cylinder along the shorter edges a tape together. Attach the new cylinder to the base. Pour the cereal from the plastic bag into the cylinder. What do you observe? How does the shape of a container affect its volume?

Check Your Understanding

For the following exercises, determine the most reasonable value for each volume.

16.

Terrarium: 50,000 km³, 50,000 m³, 50,000 cm³, or 50,000 mm³

17.

Milk carton: 236,000 L, 236 L, 236,000 mL, or 236 mL

18.

Box of crackers: 1,500 km³, 1,500 m³, 1,500 cm³, or 1,500 mm³

For the following exercises, Convert the given volume to the units shown.

19.

42,500 mm³ = __________ cm³

20.

1.5 dm³ = __________ mL

21.

6.75 cm³ = __________ mm³

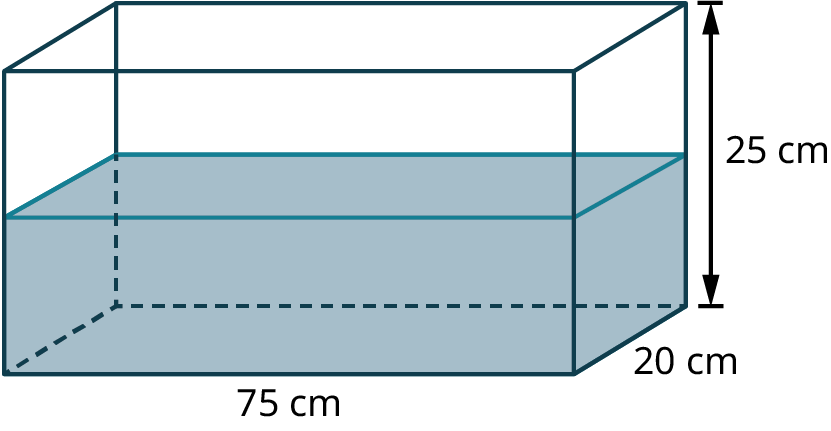
For the following exercises, determine the volume of objects with the dimensions shown.

22.

/**/20\,{\text{cm}} \times 20\,{\text{m}} \times 20\,\text{cm}/**/
/**/V =/**/ ________ L

23.

/**/12\,{\text{mm}} \times 5\,{\text{cm}} \times 1.7\,{\text{cm}}/**/
/**/V =/**/ ________ mL

24.

/**/7.3\,{\text{m}} \times 3.2\,{\text{m}} \times 7\,{\text{m}}/**/
/**/V =/**/ ________ m³

Section 9.3 Exercises

For the following exercises, determine the most reasonable value for each volume.

1.

Fish tank:
71,120 km³, 71,120 m³, 71,120 cm³, or 71,120 mm³

2.

Juice box:
125,000 L, 125 L, 125,000 mL, or 125 mL

3.

Box of cereal:
2,700 km³, 2,700 m³, 2,700 cm³, or 2,700 mm³

4.

Water bottle:
5 L, 0.5 L, 5 mL, or 0.5 mL

5.

Shoe box:
3,600 km³, 3.6 m³, 3,600 cm³, or 3,600 mm³

6.

Swimming pool:
45 L, 45,000 L, 45 mL, or 45,000 mL

For the following exercises, convert the given volume to the units shown.

7.

38,861 mm³ = __________ cm³

8.

13 dm³ = __________ mL

9.

874 cm³ = __________ mm³

10.

4 m³ = __________ cm³

11.

0.00003 m³ = _________ mm³

12.

57,500 mm³ = _______ L

13.

0.007 m³ = __________ L

14.

8,600 cm³ = _________ m³

15.

45.65 m³ = _______ cm³

16.

0.06 m³ = __________ dm³

17.

0.081 m³ = _________ mL

18.

3,884,000 mm³ = _______ m³

For the following exercises, determine the volume of objects with the dimensions shown.

19.

/**/30\,{\text{cm}} \times 20\,{\text{m}} \times 10\,{\text{cm}}/**/
/**/V =/**/ ________ L

20.

/**/17\,{\text{mm}} \times 3\,{\text{cm}} \times 2.5\,{\text{cm}}/**/
/**/V =/**/ ________ mL

21.

/**/3.4\,{\text{m}} \times 2.5\,{\text{m}} \times 10\,{\text{m}}/**/
/**/V =/**/ ________ m³

22.

/**/325\,{\text{mm}} \times 20\,{\text{cm}} \times 0.05\,{\text{m}}/**/
/**/V =/**/ ________ cm³

23.

/**/3.7\,{\text{m}} \times 4\,{\text{m}} \times 5.5\,{\text{m}}/**/
/**/V =/**/ ________ m³

24.

/**/18\,{\text{dm}} \times 0.8\,{\text{m}} \times 150\,{\text{cm}}/**/
/**/V =/**/ ________ L

25.

/**/15\,{\text{cm}} \times 400\,{\text{mm}} \times 3\,{\text{dm}}/**/
/**/V =/**/ ________ mL

26.

/**/3.5\,{\text{cm}} \times 200\,{\text{mm}} \times 0.7\,{\text{dm}}/**/
/**/V =/**/ ________ cm³

27.

/**/35\,{\text{m}} \times 1.2\,{\text{m}} \times 0.007\,{\text{km}}/**/
/**/V =/**/ ________ m³

28.

A box has dimensions of /**/20\,{\text{cm}} \times 15\,{\text{cm}} \times 30\,{\text{cm}}/**/. The box currently holds 1,250 cm³ of rice. How many cubic centimeters of rice are needed to completely fill the box?

29.

The dimensions of a medium storage unit are /**/4\,{\text{m}} \times 4\,{\text{m}} \times 8\,{\text{m}}/**/. What is the volume of a small storage area with dimensions half the size of the medium unit?

30.

How much liquid, in liters, can a container with dimensions of /**/40\,{\text{cm}} \times 20\,{\text{cm}} \times 120\,{\text{cm}}/**/ hold?

31.

What is the volume of the rectangular prism that is shown?

32.

A box is 15 centimeters long and 5 centimeters wide. The volume of the box is 225 cm³. What is the height of the box?

33.

Kareem mixed two cartons of orange juice, three 2-liter bottles of soda water and six cans of cocktail fruits to make a fruit punch for a party. The cartons of orange juice and cans of cocktail fruits each have a volume of 500 cm³. How much punch, in liters, did Kareem make?

34.

A holding tank has dimensions of /**/16\,{\text{m}} \times 8\,{\text{m}} \times 8\,{\text{m}}/**/. If the tank is half-full, how more liters of liquid can the tank hold?

35.

A large plastic storage bin has dimensions of /**/16\,{\text{cm}} \times 16\,{\text{cm}} \times 16\,{\text{cm}}/**/. A medium bin’s dimensions are half the size of the large bin. A small bin’s dimensions are the size of the medium bin. If the storage bins come in a set of 3—small, medium, and large—what is the total volume of the storage bin set in cubic centimeters?

36.

A soft serve ice cream machine holds a 19.2 liter bag of ice cream mix. If the average serving size of an ice cream cone is 120 mL, how many cones can be made from each bag of mix?

37.

A shipping carton has dimensions of /**/0.5\,{\text{m}} \times 0.5\,{\text{m}} \times 0.5\,{\text{m}}/**/. How many boxes with dimensions of /**/50\,{\text{mm}} \times 50\,{\text{mm}} \times 50\,{\text{mm}}/**/ will fit in the shipping carton?

38.

A recipe for chili makes 3.5 liters of chili. If a restaurant serves chili in 250 mL bowls, how many bowls of chili can they serve?

39.

A contractor is building an in-ground pool. They excavate a pit that measures /**/12\,{\text{m}} \times 9\,{\text{m}} \times 2.5\,{\text{m}}/**/. The dirt is being taken away in a truck that holds 30 m³. How many trips will the truck have to make to cart away all of the dirt?

40.

A juice dispenser measures /**/30\,{\text{cm}} \times 30\,{\text{cm}} \times 30\,{\text{cm}}/**/. How many 375 mL servings will a full dispenser serve?