14.1E: Exercises for Section 14.1
- Page ID
- 67808
For the following exercises, evaluate each function at the indicated values.
1) \( W(x,y)=4x^2+y^2.\) Find \( W(2,−1), W(−3,6)\).
- Answer
- \( W(2,−1) = 17,\quad W(−3,6) = 72\)
2) \( W(x,y)=4x^2+y^2\). Find \( W(2+h,3+h).\)
3) The volume of a right circular cylinder is calculated by a function of two variables, \( V(x,y)=πx^2y,\) where \( x\) is the radius of the right circular cylinder and \( y\) represents the height of the cylinder. Evaluate \( V(2,5)\) and explain what this means.
- Answer
- \( V(2,5) = 20π\,\text{units}^3\) This is the volume when the radius is \( 2\) and the height is \( 5\).
4) An oxygen tank is constructed of a right cylinder of height \( y\) and radius \( x\) with two hemispheres of radius \( x\) mounted on the top and bottom of the cylinder. Express the volume of the cylinder as a function of two variables, \( x\) and \( y\), find \( V(10,2)\), and explain what this means.
For exercises 5 - 10, find the domain and range of the given function. State the domain in set-builder notation and the range in interval notation.
5) \( V(x,y)=4x^2+y^2\)
- Answer
- Domain: \(\big\{(x, y) \, | \, x \in \rm I\!R, y \in \rm I\!R\big\}\) That is, all points in the \(xy\)-plane
Range: \( [0, \infty) \)
6) \( f(x,y)=\sqrt{x^2+y^2−4}\)
- Answer
- Domain: \( \big\{(x, y) \, | \, x^2+y^2 \ge 4\big\}\)
Range: \( [0, \infty) \)
7) \( f(x,y)=4\ln(y^2−x)\)
- Answer
- Domain: \( \big\{(x, y) \, | \, x<y^2 \big\}\)
Range: \( (-\infty, \infty) \)
8) \( g(x,y)=\sqrt{16−4x^2−y^2}\)
- Answer
- Domain: \( \big\{(x, y) \, | \, \dfrac{x^2}{4} + \dfrac{y^2}{16} \le 1\big\}\)
Range: \( [0, 4] \)
9) \( z=\arccos(y−x)\)
- Answer
- Domain: \( \big\{(x, y) \, | \, x - 1 \le y \le x + 1\big\}\) That is, all points between the graphs of \(y = x -1\) and \(y = x +1 \).
Range: \( [0, \pi] \)
10) \( f(x,y)=\dfrac{y+2}{x^2}\)
- Answer
- Domain: \( \big\{(x, y) \, | \, x\neq 0 \big\}\)
Range: \( (-\infty, \infty) \)
Find the range of the functions.
11) \( g(x,y)=\sqrt{16−4x^2−y^2}\)
- Answer
- \( \big\{z \, | \, 0≤z≤4\big\}\) or in interval notation: \([0,4]\)
12) \( V(x,y)=4x^2+y^2\)
13) \( z=y^2−x^2\)
- Answer
- The set \(\rm I\!R\)
In exercises 14 - 29, find the level curves of each function at the indicated values of \( c\) to visualize the given function. Sketch a contour plot for those exercises where you are asked for more than 3 values of \(c\).
14) \( z(x,y)=y^2−x^2, \quad c=1\)
15) \( z(x,y)=y^2−x^2,\quad c=4\)
- Answer
- \( y^2−x^2=4,\) a hyperbola
16) \( g(x,y)=x^2+y^2;\quad c=0, 1, 2, 3, 4, 9\)
17) \( g(x,y)=4−x−y;\quad c=0,1, 2, 3, 4\)
- Answer
- Level curves are lines with \( y = -x + (4 - c) \).
For each value of \(c\) these are:
\( c = 0: \, y = -x + 4\),
\( c = 1: \, y = -x + 3\),
\( c = 2: \, y = -x + 2\),
\( c = 3: \, y = -x + 1\),
\( c = 4: \, y = -x \).
The contour plot consists of a series of parallel lines.
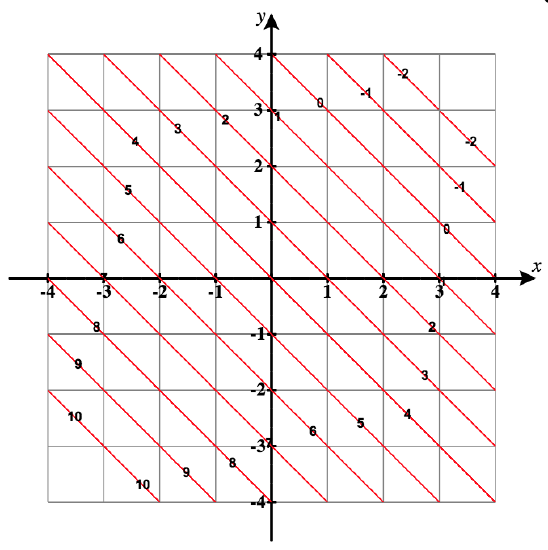
18) \( f(x,y)=xy;c=1;\quad c=−1\)
19) \( h(x,y)=2x−y;\quad c=-2,0,2\)
- Answer
- \( 2x−y=0,2x−y=−2,2x−y=2;\) three lines
20) \( f(x,y)=x^2−y;\quad c=1,2\)
21) \( g(x,y)=\dfrac{x}{x+y};c=−1,0,1,2\)
- Answer
- Level curves are lines with the form \( y = x \left( \dfrac{1-c}{c} \right) \). At \(c = 0\), we solve it directly from the equation \(\dfrac{x}{x+y}=0\) to get \(x = 0\).
For each value of \(c\) these are:
\( c = -1: \, y = -2x\),
\( c = 0: \, x = 0,\text{ with }y \ne 0\),
\( c = 1: \, y = 0,\text{ with }x \ne 0\),
\( c = 2: \, y = -\frac{1}{2}x\).
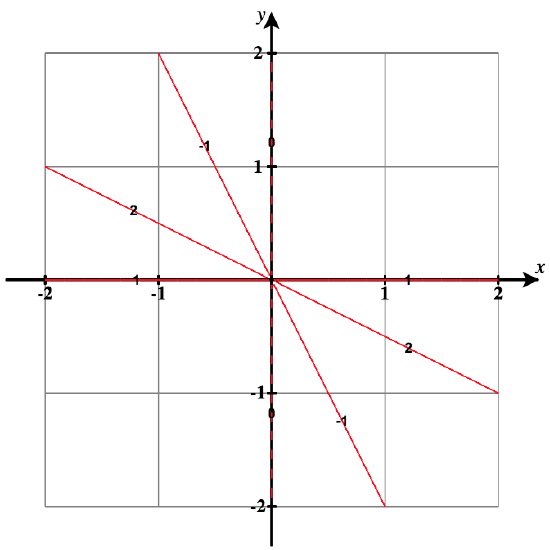
22) \( g(x,y)=x^3−y;\quad c=−1,0,2\)
23) \( g(x,y)=e^{xy};\quad c=\frac{1}{2},3\)
- Answer
- The level curves have the form, \( y = \dfrac{\ln c}{x}\).
For each value of \(c\) these are:
\( c = \frac{1}{2}: \, y = \dfrac{\ln \frac{1}{2}}{x}\) that can be rewritten as, \(y = -\dfrac{\ln 2}{x}\)
\( c = 3: \, y = \dfrac{\ln 3}{x}\).
24) \( f(x,y)=x^2;\quad c=4,9\)
25) \( f(x,y)=xy−x;\quad c=−2,0,2\)
- Answer
- Level curves have the form: \( y = \dfrac{c}{x} + 1\).
Here \(y = \dfrac{-2}{x} + 1,\quad y = 1,\quad y = \dfrac{2}{x} + 1\) or \( xy−x=−2,\,xy−x=0,\,xy−x=2\)
26) \( h(x,y)=\ln(x^2+y^2);\quad c=−1,0,1\)
27) \( g(x,y)=\ln\left(\dfrac{y}{x^2}\right);\quad c=−2,0,2\)
- Answer
- The level curves have the form, \( y =e^c x^2\).
For each value of \(c\) these are:
\( c = -2: \, y = e^{-2} x^2 \),
\( c = 0: \, y = x^2 \),
\( c = 2: \, y = e^{2} x^2 \).
28) \( z=f(x,y)=\sqrt{x^2+y^2},\quad c=3\)
29) \( f(x,y)=\dfrac{y+2}{x^2},\quad c=\) any constant
- Answer
- The level curves are parabolas of the form \( y=cx^2−2,\text{ with }x \ne 0\).
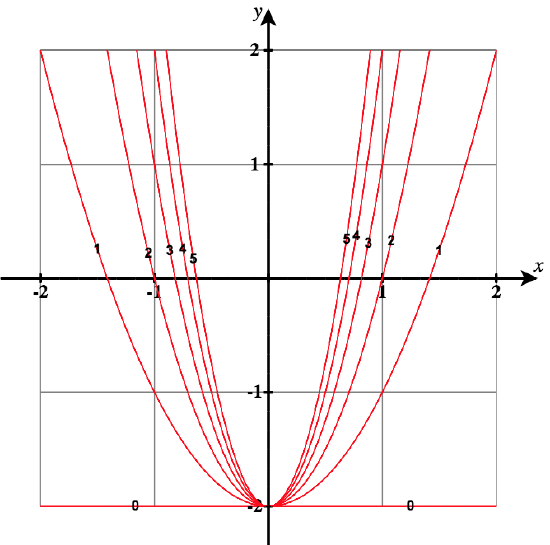
In exercises 30-32, find the vertical traces of the functions at the indicated values of \( x\) and \( y\), and plot the traces.
30) \( z=4−x−y, \quad x=2\)
31) \( f(x,y)=3x+y^3, \quad x=1\)
- Answer
-
\( z=3+y^3,\) a curve in the \(zy\)-plane with rulings parallel to the \(x\)-axis
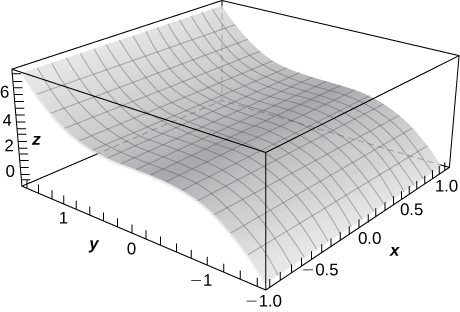
32) \( z=\cos\sqrt{x^2+y^2}, \quad x=1\)
In exercises 33 - 38, find the domain and range of each function.
33) \( z=\sqrt{100−4x^2−25y^2}\)
- Answer
- Domain: \( \big\{(x, y) \, | \, \dfrac{x^2}{25}+\dfrac{y^2}{4}≤1\big\}\)
Range: \( [0, 10] \)
34) \( z=\ln(x−y^2)\)
35) \( f(x,y,z)=\dfrac{1}{\sqrt{36−4x^2−9y^2−z^2}}\)
- Answer
- Domain: \( \big\{(x, y, z) \, | \, \dfrac{x^2}{9}+\dfrac{y^2}{4}+\dfrac{z^2}{36}<1\big\}\)
Range: \( \big[\frac{1}{6}, \infty\big) \)
36) \( f(x,y,z)=\sqrt{49−x^2−y^2−z^2}\)
37) \( f(x,y,z)=\sqrt[3]{16−x^2−y^2−z^2}\)
- Answer
- Domain: All points in \( xyz\)-space
Range: \( \big(-\infty, \sqrt[3]{16}\,\big] \)
38) \( f(x,y)=\cos\sqrt{x^2+y^2}\)
In exercises 39 - 40, plot a graph of the function.
39) \( z=f(x,y)=\sqrt{x^2+y^2}\)
- Answer
-
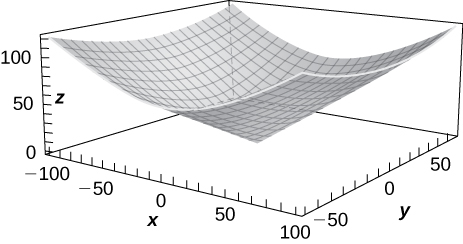
40) \( z=x^2+y^2\)
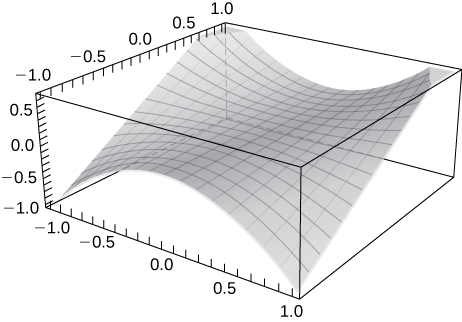
41) Use technology to graph \( z=x^2y.\)
- Answer
-
In exercises 42 - 46, sketch the function by finding its level curves. Verify the graph using technology, such as CalcPlot3D.
42) \( f(x,y)=\sqrt{4−x^2−y^2}\)
43) \( f(x,y)=2−\sqrt{x^2+y^2}\)
- Answer
-
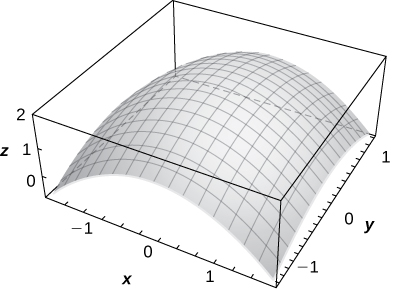
44) \( z=1+e^{−x^2−y^2}\)
45) \( z=\cos\sqrt{x^2+y^2}\)
- Answer
-
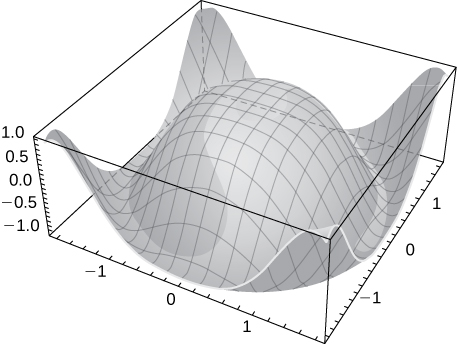
46) \( z=y^2−x^2\)
47) Describe the contour lines for several values of \( c\) for \( z=x^2+y^2−2x−2y.\)
- Answer
- The contour lines are concentric circles centered at the point, \( (1, 1) \).
You can see this by completing the square after setting this function equal to \(c\).
That is, we write \( x^2-2x+1+y^2−2y+1 = c + 2 \) which can be rewritten as, \( (x - 1)^2 + (y - 1)^2 = c + 2 \).
This gives us circles centered at the point, \( (1, 1) \), each with a radius of \( \sqrt{c+2} \).
In exercises, 48 - 52, find the level surface for the given value of \(c\) for each function of three variables and describe it.
48) \( w(x,y,z)=x−2y+z,\quad c=4\)
49) \( w(x,y,z)=x^2+y^2+z^2,\quad c=9\)
- Answer
- \( x^2+y^2+z^2=9\), a sphere of radius \( 3\)
50) \( w(x,y,z)=x^2+y^2−z^2,\quad c=−4\)
51) \( w(x,y,z)=x^2+y^2−z^2,\quad c=4\)
- Answer
- \( x^2+y^2−z^2=4,\) a hyperboloid of one sheet
52) \( w(x,y,z)=9x^2−4y^2+36z^2,\quad c=0\)
In exercises 53 - 55, find an equation of the level curve of \( f\) that contains the point \( P\).
53) \( f(x,y)=1−4x^2−y^2,\quad P(0,1)\)
- Answer
- \( 4x^2+y^2=1,\)
54) \( g(x,y)=y^2\arctan x,\quad P(1,2)\)
55) \( g(x,y)=e^{xy}(x^2+y^2),\quad P(1,0)\)
- Answer
- \( 1=e^{xy}(x^2+y^2)\)
56) The strength \( E\) of an electric field at point \( (x,y,z)\) resulting from an infinitely long charged wire lying along the \(y\)-axis is given by \( E(x,y,z)=k/\sqrt{x^2+y^2}\), where \( k\) is a positive constant. For simplicity, let \( k=1\) and find the equations of the level surfaces for \( E=10\) and \( E=100.\)
57) A thin plate made of iron is located in the \(xy\)-plane The temperature \( T\) in degrees Celsius at a point \( P(x,y)\) is inversely proportional to the square of its distance from the origin. Express \( T\) as a function of \( x\) and \( y\).
- Answer
- \( T(x,y)=\dfrac{k}{x^2+y^2}\)
58) Refer to the preceding problem. Using the temperature function found there, determine the proportionality constant if the temperature at point \( P(1,2)\) is \( 50°C.\) Use this constant to determine the temperature at point \( Q(3,4).\)
59) Refer to the preceding problem. Find the level curves for \( T=40°C\) and \( T=100°C,\) and describe what the level curves represent.
- Answer
- \( x^2+y^2=\dfrac{k}{40}, \quad x^2+y^2=\dfrac{k}{100}\). The level curves represent circles of radii \( \sqrt{10k}/20\) and \( \sqrt{k}/10\)
Contributors
Gilbert Strang (MIT) and Edwin “Jed” Herman (Harvey Mudd) with many contributing authors. This content by OpenStax is licensed with a CC-BY-SA-NC 4.0 license. Download for free at http://cnx.org.
- Paul Seeburger (Monroe Community College) added the contour plots to answers for problems 17, 21 and 29.

