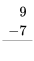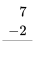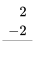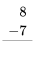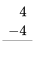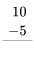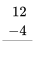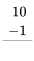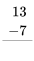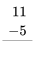3.1: Topic A- Subtraction

Last updated
Save as PDF

Page ID: 95401

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$ \newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\id}{\mathrm{id}}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\kernel}{\mathrm{null}\,}$

$ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$

$ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$

$ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\AA}{\unicode[.8,0]{x212B}}$

$ \newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$ \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$ \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vectorC}[1]{\textbf{#1}} $

$ \newcommand{\vectorD}[1]{\overrightarrow{#1}} $

$ \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} $

$ \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} $

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$\newcommand{\avec}{\mathbf a}$ $\newcommand{\bvec}{\mathbf b}$ $\newcommand{\cvec}{\mathbf c}$ $\newcommand{\dvec}{\mathbf d}$ $\newcommand{\dtil}{\widetilde{\mathbf d}}$ $\newcommand{\evec}{\mathbf e}$ $\newcommand{\fvec}{\mathbf f}$ $\newcommand{\nvec}{\mathbf n}$ $\newcommand{\pvec}{\mathbf p}$ $\newcommand{\qvec}{\mathbf q}$ $\newcommand{\svec}{\mathbf s}$ $\newcommand{\tvec}{\mathbf t}$ $\newcommand{\uvec}{\mathbf u}$ $\newcommand{\vvec}{\mathbf v}$ $\newcommand{\wvec}{\mathbf w}$ $\newcommand{\xvec}{\mathbf x}$ $\newcommand{\yvec}{\mathbf y}$ $\newcommand{\zvec}{\mathbf z}$ $\newcommand{\rvec}{\mathbf r}$ $\newcommand{\mvec}{\mathbf m}$ $\newcommand{\zerovec}{\mathbf 0}$ $\newcommand{\onevec}{\mathbf 1}$ $\newcommand{\real}{\mathbb R}$ $\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$ $\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$ $\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$ $\newcommand{\laspan}[1]{\text{Span}\{#1\}}$ $\newcommand{\bcal}{\cal B}$ $\newcommand{\ccal}{\cal C}$ $\newcommand{\scal}{\cal S}$ $\newcommand{\wcal}{\cal W}$ $\newcommand{\ecal}{\cal E}$ $\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$ $\newcommand{\gray}[1]{\color{gray}{#1}}$ $\newcommand{\lgray}[1]{\color{lightgray}{#1}}$ $\newcommand{\rank}{\operatorname{rank}}$ $\newcommand{\row}{\text{Row}}$ $\newcommand{\col}{\text{Col}}$ $\renewcommand{\row}{\text{Row}}$ $\newcommand{\nul}{\text{Nul}}$ $\newcommand{\var}{\text{Var}}$ $\newcommand{\corr}{\text{corr}}$ $\newcommand{\len}[1]{\left|#1\right|}$ $\newcommand{\bbar}{\overline{\bvec}}$ $\newcommand{\bhat}{\widehat{\bvec}}$ $\newcommand{\bperp}{\bvec^\perp}$ $\newcommand{\xhat}{\widehat{\xvec}}$ $\newcommand{\vhat}{\widehat{\vvec}}$ $\newcommand{\uhat}{\widehat{\uvec}}$ $\newcommand{\what}{\widehat{\wvec}}$ $\newcommand{\Sighat}{\widehat{\Sigma}}$ $\newcommand{\lt}{<}$ $\newcommand{\gt}{>}$ $\newcommand{\amp}{&}$ $\definecolor{fillinmathshade}{gray}{0.9}$

Topic A: Subtraction

Subtraction takes an amount away from another amount. The result of subtraction is called the difference.

The minus sign − means to subtract.

$nine minus three is six$

says nine minus three equals six or nine take away three is six.

The difference between 9 and 3 is 6.

Subtraction is the opposite of addition.

Look at the examples:

\begin{array}{cc}
5 + 4 = 9 & 9-4 = 5\\
4+5 = 9 & 9-5 = 4
\end{array}

\begin{array}{cc}
\begin{aligned}
8 \\
+3 \\
\hline 11
\end{aligned} &
\begin{aligned}
11 \\
-3 \\
\hline 8
\end{aligned} \\
\begin{aligned}
3 \\
+8 \\
\hline 11
\end{aligned} &
\begin{aligned}
11 \\
-8 \\
\hline 3
\end{aligned}
\end{array}

Subtraction facts are a tool that you will use to do subtraction questions.

Exercise 1

Check out your subtraction facts by doing the following exercises as quickly as you can. Use your addition facts to help find the subtraction facts. Check your work using the answer key at the end of the exercises. Then, make a list of any subtraction facts you do not know or which are slow for you and practice them.

$\begin{align}5\\-4\\ \hline\\ \end{align}$
$\begin{align}3\\-2\\ \hline\\ \end{align}$
$\begin{align}7\\-7\\ \hline\\ \end{align}$
$\begin{align}1\\-0\\ \hline\\ \end{align}$
$\begin{align}8\\-2\\ \hline\\ \end{align}$
$\begin{align}9\\-7\\ \hline\\ \end{align}$
$\begin{align}4\\-3\\ \hline\\ \end{align}$
$\begin{align}6\\-1\\ \hline\\ \end{align}$
$\begin{align}7\\-2\\ \hline\\ \end{align}$
$\begin{align}2\\-2\\ \hline\\ \end{align}$
$\begin{align}7\\-6\\ \hline\\ \end{align}$
$\begin{align}8\\-7\\ \hline\\ \end{align}$

Answers to Exercise 1

Exercise 2

$\begin{align}8\\-4\\ \hline\\ \end{align}$
$\begin{align}9\\-1\\ \hline\\ \end{align}$
$\begin{align}7\\-5\\ \hline\\ \end{align}$
$\begin{align}6\\-4\\ \hline\\ \end{align}$
$\begin{align}9\\-4\\ \hline\\ \end{align}$
$\begin{align}5\\-2\\ \hline\\ \end{align}$
$\begin{align}2\\-0\\ \hline\\ \end{align}$
$\begin{align}6\\-3\\ \hline\\ \end{align}$
$\begin{align}8\\-3\\ \hline\\ \end{align}$
$\begin{align}6\\-5\\ \hline\\ \end{align}$
$\begin{align}4\\-4\\ \hline\\ \end{align}$
$\begin{align}9\\-0\\ \hline\\ \end{align}$

Answers to Exercise 2

Exercise 3

$\begin{align}8\\-4\\ \hline\\ \end{align}$
$\begin{align}5\\-5\\ \hline\\ \end{align}$
$\begin{align}2\\-1\\ \hline\\ \end{align}$
$\begin{align}4\\-3\\ \hline\\ \end{align}$
$\begin{align}3\\-3\\ \hline\\ \end{align}$
$\begin{align}6\\-3\\ \hline\\ \end{align}$
$\begin{align}7\\-6\\ \hline\\ \end{align}$
$\begin{align}9\\-2\\ \hline\\ \end{align}$
$\begin{align}9\\-0\\ \hline\\ \end{align}$
$\begin{align}5\\-4\\ \hline\\ \end{align}$
$\begin{align}8\\-8\\ \hline\\ \end{align}$
$\begin{align}4\\-2\\ \hline\\ \end{align}$
$\begin{align}7\\-7\\ \hline\\ \end{align}$
$\begin{align}2\\-0\\ \hline\\ \end{align}$
$\begin{align}6\\-1\\ \hline\\ \end{align}$
$\begin{align}9\\-8\\ \hline\\ \end{align}$

Answers to Exercise 3

Exercise 4

$\begin{align}11\\-7\\ \hline\\ \end{align}$
$\begin{align}10\\-4\\ \hline\\ \end{align}$
$\begin{align}12\\-7\\ \hline\\ \end{align}$
$\begin{align}8\\-6\\ \hline\\ \end{align}$
$\begin{align}10\\-8\\ \hline\\ \end{align}$
$\begin{align}7\\-4\\ \hline\\ \end{align}$
$\begin{align}9\\-3\\ \hline\\ \end{align}$
$\begin{align}9\\-5\\ \hline\\ \end{align}$

Answers to Exercise 4

Exercise 5

$\begin{align}12\\-3\\ \hline\\ \end{align}$
$\begin{align}9\\-6\\ \hline\\ \end{align}$
$\begin{align}11\\-9\\ \hline\\ \end{align}$
$\begin{align}10\\-5\\ \hline\\ \end{align}$
$\begin{align}8\\-8\\ \hline\\ \end{align}$
$\begin{align}10\\-4\\ \hline\\ \end{align}$
$\begin{align}12\\-4\\ \hline\\ \end{align}$
$\begin{align}7\\-6\\ \hline\\ \end{align}$

Answers to Exercise 5

Exercise 6

$\begin{align}11\\-7\\ \hline\\ \end{align}$
$\begin{align}12\\-6\\ \hline\\ \end{align}$
$\begin{align}10\\-9\\ \hline\\ \end{align}$
$\begin{align}8\\-3\\ \hline\\ \end{align}$
$\begin{align}12\\-5\\ \hline\\ \end{align}$
$\begin{align}10\\-4\\ \hline\\ \end{align}$
$\begin{align}9\\-7\\ \hline\\ \end{align}$
$\begin{align}7\\-3\\ \hline\\ \end{align}$
$\begin{align}8\\-4\\ \hline\\ \end{align}$
$\begin{align}11\\-9\\ \hline\\ \end{align}$
$\begin{align}9\\-5\\ \hline\\ \end{align}$
$\begin{align}7\\-2\\ \hline\\ \end{align}$

Answers to Exercise 6

Exercise 6

$\begin{align}11\\-7\\ \hline\\ \end{align}$
$\begin{align}12\\-6\\ \hline\\ \end{align}$
$\begin{align}10\\-9\\ \hline\\ \end{align}$
$\begin{align}8\\-3\\ \hline\\ \end{align}$
$\begin{align}12\\-5\\ \hline\\ \end{align}$
$\begin{align}10\\-4\\ \hline\\ \end{align}$
$\begin{align}9\\-7\\ \hline\\ \end{align}$
$\begin{align}7\\-3\\ \hline\\ \end{align}$
$\begin{align}8\\-4\\ \hline\\ \end{align}$
$\begin{align}11\\-9\\ \hline\\ \end{align}$
$\begin{align}6\\-5\\ \hline\\ \end{align}$
$\begin{align}7\\-2\\ \hline\\ \end{align}$

Answers to Exercise 6

Exercise 7

$\begin{align}13\\-5\\ \hline\\ \end{align}$
$\begin{align}10\\-1\\ \hline\\ \end{align}$
$\begin{align}9\\-4\\ \hline\\ \end{align}$
$\begin{align}5\\-4\\ \hline\\ \end{align}$
$\begin{align}9\\-9\\ \hline\\ \end{align}$
$\begin{align}16\\-8\\ \hline\\ \end{align}$
$\begin{align}11\\-7\\ \hline\\ \end{align}$
$\begin{align}6\\-3\\ \hline\\ \end{align}$
$\begin{align}18\\-9\\ \hline\\ \end{align}$
$\begin{align}7\\-2\\ \hline\\ \end{align}$
$\begin{align}13\\-7\\ \hline\\ \end{align}$
$\begin{align}8\\-6\\ \hline\\ \end{align}$

Answers to Exercise 7

Practice your subtraction facts using dominoes. Place all the dominoes face down.

Exercise 8

$\begin{align}12\\-3\\ \hline\\ \end{align}$
$\begin{align}6\\-2\\ \hline\\ \end{align}$
$\begin{align}10\\-4\\ \hline\\ \end{align}$
$\begin{align}11\\-9\\ \hline\\ \end{align}$
$\begin{align}1\\-0\\ \hline\\ \end{align}$
$\begin{align}8\\-1\\ \hline\\ \end{align}$
$\begin{align}12\\-5\\ \hline\\ \end{align}$
$\begin{align}11\\-2\\ \hline\\ \end{align}$
$\begin{align}3\\-2\\ \hline\\ \end{align}$
$\begin{align}11\\-8\\ \hline\\ \end{align}$
$\begin{align}14\\-7\\ \hline\\ \end{align}$
$\begin{align}8\\-3\\ \hline\\ \end{align}$
$\begin{align}15\\-9\\ \hline\\ \end{align}$
$\begin{align}9\\-7\\ \hline\\ \end{align}$
$\begin{align}7\\-1\\ \hline\\ \end{align}$
$\begin{align}11\\-5\\ \hline\\ \end{align}$

Answers to Exercise 8

Need some extra practice?

Find a partner and play this card game.
Using a regular deck of cards, a jack will be eleven, a queen will be twelve and a king will be thirteen.
Shuffle the cards and deal them out. Keep your cards in a pile in front of you.
Each player flips over a card.
Take turns subtracting the numbers on the cards. If the person gets the right answer that person gets to keep the cards. If the person get the wrong answer the other player gets the cards.
The person who collects all the cards is the winner.
You could also set a time limit and the person with the most cards when time is up is the winner.

Subtracting Across

So far you have only been subtracting numbers when they are up and down or vertical.

Example:

\begin{aligned}
9 \\
-5 \\
\hline 4
\end{aligned}

Another way to subtract numbers is across or horizontally.

$9 − 5 = 4$

When you subtract numbers across, you work from left to right.

Exercise 9

Practice subtracting across or horizontally. Check your work using the answer key at the end of the exercise.

6 − 3 =
12 − 8 =
4 − 1 =
8 − 6 =
18 − 9 =
11 − 4 =
7 − 2 =
16 − 7 =
10 − 5 =
2 − 0 =
9 − 5 =
17 − 8 =

Answers to Exercise 9

Exercise 10

Practice subtracting across or horizontally. Check your work using the answer key at the end of the exercise.

9 − 6 =
14 − 5 =
8 − 4 =
7 − 1 =
11 − 7 =
5 − 0 =
4 − 3 =
15 − 8 =
11 − 9 =
10 − 2 =
9 − 2 =
8 − 3 =

Answers to Exercise 10

Exercise 11

Practice subtracting across or horizontally. Check your work using the answer key at the end of the exercise.

3 − 2 =
17 − 9 =
14 − 7 =
9 − 3 =
12 − 5 =
8 − 8 =
6 − 1 =
13 − 4 =
11 − 6 =
4 − 0 =
8 − 1 =
16 − 9 =
7 − 0 =
13 − 8 =
12 − 3 =
9 − 4 =
15 − 7 =
10 − 6 =
11 − 5 =
5 − 2 =

Answers to Exercise 11

Word Problems

Learning subtraction facts is very important because once you know them all they become a tool to use when solving problems.

Words such as:

less than
minus
subtracted from
how many more
how much more, and
difference

tell you to subtract the numbers.

Look for these words when reading word problems and underline them before trying to solve a problem. Circle the information that is given.

Example:

There were 14 nails in a box. Lu used 7 of them. How many nails were still in the box?

You have circled 14 nails and 7. This is the information you will use to find the answer.

You have underlined “how many”. These words tell you to subtract.

\begin{aligned}
14 \text{ nails} \\
-7 \text{ nails} \\
\hline 7 \text{ nails}
\end{aligned}

Exercise 12

Wolfgang walked 11 blocks. Ingrid walked 6 blocks. Wolfgang walked how much farther than Ingrid?
Mika and her father went fishing. Mika caught 18 fish and her father caught 9 fish. How many more fish did Mika catch?
Kuan-Lin was making moon cakes for the class party. She needed 15 cakes for the party. On Monday she had made 7 moon cakes. How many moon cakes did she still need to make?
Malik counted 12 cars in the parking lot where he worked. One hour later, he counted only 4 cars. How many cars left?

Answers to Exercise 12

5 blocks
9 fish
8 moon cakes
8 cars

Topic A Self-Test

Mark /21 Aim 18/27

Find the difference. Be sure to check your answers. (9 marks)
1. $\begin{align}16\\-8\\ \hline\\ \end{align}$
2. $\begin{align}18\\-9\\ \hline\\ \end{align}$
3. $\begin{align}14\\-8\\ \hline\\ \end{align}$
4. $\begin{align}11\\-4\\ \hline\\ \end{align}$
5. $\begin{align}9\\-3\\ \hline\\ \end{align}$
6. $\begin{align}17\\-9\\ \hline\\ \end{align}$
7. $\begin{align}10\\-6\\ \hline\\ \end{align}$
8. $\begin{align}7\\-5\\ \hline\\ \end{align}$
9. $\begin{align}15\\-6\\ \hline\\ \end{align}$
Find the difference. Be sure to check your answers. (6 marks)
1. 3 − 2 =
2. 17 − 9 =
3. 14 − 7 =
4. 9 − 3 =
5. 12 − 5 =
6. 8 − 8 =
Solve each of the following word problems. Be sure to include the unit of measure in your answer. Be sure to circle the information and underline what’s being asked.(6 marks, 2 marks each)
1. Shada caught 17 fish. She gave 8 fish to her grandmother. How many fish did she have left?
2. Yuan went to the store with $15 to buy some rice. The rice cost $6. How much did he have left?
3. Carlo had 13 metres of fencing. He used 8 metres around his flower garden. How many metres did he have left?

Answers to Topic A Self-Test

1. 8
2. 9
3. 6
4. 7
5. 6
6. 8
7. 4
8. 2
9. 9
1. 1
2. 8
3. 7
4. 6
5. 7
6. 0
1. 9 fish
2. $9
3. 5 metres

Search

Text Color

Text Size

Margin Size

Font Type

Topic A: Subtraction