6.1.1: Organizing Data

Lesson

Let's find ways to show patterns in data

Exercise \(\PageIndex{1}\): Notice and Wonder: Messy Data

Here is a table of data. Each row shows two measurements of a triangle.

Table \(\PageIndex{1}\)

length of short side (cm)	length of perimeter (cm)
0.25	1
2	7.5
6.5	22
3	9.5
0.5	2
1.25	3.5
3.5	12.5
1.5	5
4	14
1	2.5

What do you notice? What do you wonder?

Exercise \(\PageIndex{2}\): Seeing the Data

Here is the table of isosceles right triangle measurements from the warm-up and an empty table.

Table \(\PageIndex{2}\)

length of short sides (cm)	length of perimeter (cm)	length of short sides (cm)	length of perimeter (cm)
0.25	1
2	7.5
6.5	22
3	9.5
0.5	2
1.25	3.5
3.5	12.5
1.5	5
4	14
1	2.5

1. How can you organize the measurements from the first table so that any patterns are easier to see? Write the organized measurements in the empty table.
2. For each of the following lengths, estimate the perimeter of an isosceles right triangle whose short sides have that length. Explain your reasoning for each triangle.
   1. length of short sides is 0.75 cm
   2. length of short sides is 5 cm
   3. length of short sides is 10 cm

Are you ready for more?

In addition to the graphic representations of data you have learned, there are others that make sense in other situations. Examine the maps showing the results of the elections for United States president for 2012 and 2016. In red are the states where a majority of electorate votes were cast for the Republican nominee. In blue are the states where a majority of the electorate votes were cast for the Democrat nominee.

Figure \(\PageIndex{1}\)

1. What information can you see in these maps that would be more difficult to see in a bar graph showing the number of electorate votes for the 2 main candidates?
2. Why are these representations appropriate for the data that is shown?

Exercise \(\PageIndex{3}\): Tables and Their Scatter Plots

Here are four scatter plots. Your teacher will give you four tables of data.

• Match each table with one of the scatter plots.
• Use information from the tables to label the axes for each scatter plot.

Figure \(\PageIndex{2}\): Four scatterplots. First, x, 0 to 150,000 by 30,000, y, 6,000 to 21,000 by 3,000. Points begin around 2,000 comma 16,000 and trend down and right toward 120,000 comma 7,000. Second, x, 72 to 102 by 6, y, 15 to 45 by 5. Points begin around 76 comma 18 and trend up and right toward 100 comma 40. Third, x, point 9 to 2 point 1 by point 2 4, y 2,000 to 10,000 by 2,000. Points begin around point 1 and trend up and right toward 1 point 8 6 comma 8,000. Fourth, x, 1,000 to 2,500 by 250, y, 14 to 32 by 2. Points begin around 1,100 comma 29 and trend down and right toward 2,300 comma 16.

Summary

Consider the data collected from pulling back a toy car and then letting it go forward. In the first table, the data may not seem to have an obvious pattern. The second table has the same data and shows that both values are increasing together.

Table \(\PageIndex{3}\): Unorganized Table

distance pulled back (in)	distance traveled (in)
8	23.57
4	18.48
10	38.66
8	31.12
2	13.86
1	8.95

Table \(\PageIndex{4}\): Organized Table

distance pulled back (in)	distance traveled (in)
1	8.95
2	13.86
4	18.48
6	23.57
8	31.12
10	38.66

A scatter plot of the data makes the pattern clear enough that we can estimate how far the car will travel when it is pulled back 5 inches.

Patterns in data can sometimes become more obvious when reorganized in a table or when represented in scatter plots or other diagrams. If a pattern is observed, it can sometimes be used to make predictions.

Figure \(\PageIndex{3}\)

Glossary Entries

Definition: Scatter Plot

A scatter plot is a graph that shows the values of two variables on a coordinate plane. It allows us to investigate connections between the two variables.

Each plotted point corresponds to one dog. The coordinates of each point tell us the height and weight of that dog.

Figure \(\PageIndex{4}\)

Practice

Exercise \(\PageIndex{4}\)

Here is data on the number of cases of whooping cough from 1939 to 1955.

Table \(\PageIndex{5}\)

year	number of cases
1941	222,202
1950	120,718
1945	133,792
1942	191,383
1953	37,129
1939	103,188
1951	68,687
1948	74,715
1955	62,786
1952	45,030
1940	183,866
1954	60,866
1944	109,873
1946	109,860
1943	191,890
1949	69,479
1947	156,517

1. Make a new table that orders the data by year.
2. Circle the years in your table that had fewer than 100,000 cases of whooping cough.
3. Based on this data, would you expect 1956 to have closer to 50,000 cases or closer to 100,000 cases?

Exercise \(\PageIndex{5}\)

In volleyball statistics, a block is recorded when a player deflects the ball hit from the opposing team. Additionally, scorekeepers often keep track of the average number of blocks a player records in a game. Here is part of a table that records the number of blocks and blocks per game for each player in a women’s volleyball tournament. A scatter plot that goes with the table follows.

Table \(\PageIndex{6}\)

blocks	blocks per game
13	1.18
1	0.17
5	0.42
0	0
0	0
7	0.64

Figure \(\PageIndex{5}\)

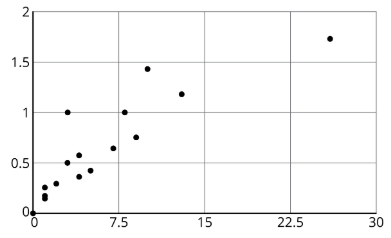
Label the axes of the scatter plot with the necessary information.

Exercise \(\PageIndex{6}\)

A cylinder has a radius of 4 cm and a height of 5 cm.

1. What is the volume of the cylinder?
2. What is the volume of the cylinder when its radius is tripled?
3. What is the volume of the cylinder when its radius is halved?

(From Unit 5.5.2)