4.2.1: Increasing and Decreasing

Last updated
Save as PDF

Page ID: 38308

Illustrative Mathematics
OpenUp Resources

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$ \newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\id}{\mathrm{id}}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\kernel}{\mathrm{null}\,}$

$ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$

$ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$

$ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\AA}{\unicode[.8,0]{x212B}}$

$ \newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$ \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$ \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vectorC}[1]{\textbf{#1}} $

$ \newcommand{\vectorD}[1]{\overrightarrow{#1}} $

$ \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} $

$ \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} $

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$\newcommand{\avec}{\mathbf a}$ $\newcommand{\bvec}{\mathbf b}$ $\newcommand{\cvec}{\mathbf c}$ $\newcommand{\dvec}{\mathbf d}$ $\newcommand{\dtil}{\widetilde{\mathbf d}}$ $\newcommand{\evec}{\mathbf e}$ $\newcommand{\fvec}{\mathbf f}$ $\newcommand{\nvec}{\mathbf n}$ $\newcommand{\pvec}{\mathbf p}$ $\newcommand{\qvec}{\mathbf q}$ $\newcommand{\svec}{\mathbf s}$ $\newcommand{\tvec}{\mathbf t}$ $\newcommand{\uvec}{\mathbf u}$ $\newcommand{\vvec}{\mathbf v}$ $\newcommand{\wvec}{\mathbf w}$ $\newcommand{\xvec}{\mathbf x}$ $\newcommand{\yvec}{\mathbf y}$ $\newcommand{\zvec}{\mathbf z}$ $\newcommand{\rvec}{\mathbf r}$ $\newcommand{\mvec}{\mathbf m}$ $\newcommand{\zerovec}{\mathbf 0}$ $\newcommand{\onevec}{\mathbf 1}$ $\newcommand{\real}{\mathbb R}$ $\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$ $\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$ $\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$ $\newcommand{\laspan}[1]{\text{Span}\{#1\}}$ $\newcommand{\bcal}{\cal B}$ $\newcommand{\ccal}{\cal C}$ $\newcommand{\scal}{\cal S}$ $\newcommand{\wcal}{\cal W}$ $\newcommand{\ecal}{\cal E}$ $\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$ $\newcommand{\gray}[1]{\color{gray}{#1}}$ $\newcommand{\lgray}[1]{\color{lightgray}{#1}}$ $\newcommand{\rank}{\operatorname{rank}}$ $\newcommand{\row}{\text{Row}}$ $\newcommand{\col}{\text{Col}}$ $\renewcommand{\row}{\text{Row}}$ $\newcommand{\nul}{\text{Nul}}$ $\newcommand{\var}{\text{Var}}$ $\newcommand{\corr}{\text{corr}}$ $\newcommand{\len}[1]{\left|#1\right|}$ $\newcommand{\bbar}{\overline{\bvec}}$ $\newcommand{\bhat}{\widehat{\bvec}}$ $\newcommand{\bperp}{\bvec^\perp}$ $\newcommand{\xhat}{\widehat{\xvec}}$ $\newcommand{\vhat}{\widehat{\vvec}}$ $\newcommand{\uhat}{\widehat{\uvec}}$ $\newcommand{\what}{\widehat{\wvec}}$ $\newcommand{\Sighat}{\widehat{\Sigma}}$ $\newcommand{\lt}{<}$ $\newcommand{\gt}{>}$ $\newcommand{\amp}{&}$ $\definecolor{fillinmathshade}{gray}{0.9}$

Lesson

Let's use percentages to describe increases and decreases.

Exercise $\PageIndex{1}$: Improving Their Game

Here are the scores from 3 different sports teams from their last 2 games.

Table $\PageIndex{1}$
sports team	total points in game 1	total points in game 2
football team	$22$	$30$
basketball team	$100$	$108$
baseball team	$4$	$12$

What do you notice about the teams' scores? What do you wonder?
Which team improved the most? Explain your reasoning.

Exercise $\PageIndex{2}$: More Cereal and a Discounted Shirt

1. A cereal box says that now it contains 20% more. Originally, it came with 18.5 ounces of cereal. How much cereal does the box come with now?

2. The price of a shirt is $18.50, but you have a coupon that lowers the price by 20%. What is the price of the shirt after using the coupon?

Exercise $\PageIndex{3}$: Using Tape Diagrams

1. Match each situation to a diagram. Be prepared to explain your reasoning.

Compared with last year’s strawberry harvest, this year’s strawberry harvest is a 25% increase.
This year’s blueberry harvest is 75% of last year’s.
Compared with last year, this year’s peach harvest decreased 25%.
This year’s plum harvest is 125% of last year’s plum harvest.

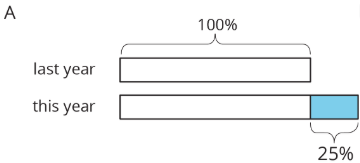
2. Draw a diagram to represent these situations.

The number of ducks living at the pond increased by 40%.
The number of mosquitoes decreased by 80%.

Are you ready for more?

What could it mean to say there is a 100% decrease in a quantity? Give an example of a quantity where this makes sense.

Exercise $\PageIndex{4}$: Agree or Disagree: Percentages

Do you agree or disagree with each statement? Explain your reasoning.

Employee A gets a pay raise of 50%. Employee B gets a pay raise of 45%. So Employee A gets the bigger pay raise.
Shirts are on sale for 20% off. You buy two of them. As you pay, the cashier says, “20% off of each shirt means 40% off of the total price.”

Summary

Imagine that it takes Andre $\frac{3}{4}$ more than the time it takes Jada to get to school. Then we know that Andre’s time is $1\frac{3}{4}$ or 1.75 times Jada’s time. We can also describe this in terms of percentages:

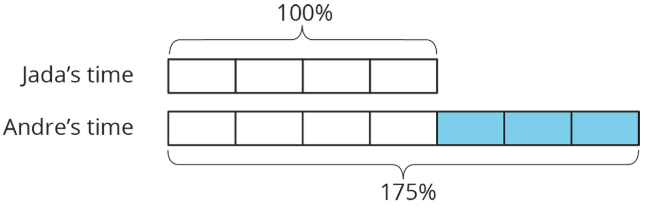
We say that Andre’s time is 75% more than Jada’s time. We can also see that Andre’s time is 175% of Jada’s time. In general, the terms percent increase and percent decrease describe an increase or decrease in a quantity as a percentage of the starting amount.

For example, if there were 500 grams of cereal in the original package, then “20% more” means that 20% of 500 grams has been added to the initial amount, $500+(0.2)\cdot 500=600$, so there are 600 grams of cereal in the new package.

We can see that the new amount is 120% of the initial amount because

$500+(0.2)\cdot 500=(1+0.2)500$

Figure $\PageIndex{7}$: Tape diagram. 6 equal sections, 1 shaded blue. 120 percent labels the entire tape. 100 percent labels 5 sections. 20 percent labels the one blue shaded section.

Glossary Entries

Definition: Percentage Decrease

A percentage decrease tells how much a quantity went down, expressed as a percentage of the starting amount.

For example, a store had 64 hats in stock on Friday. They had 48 hats left on Saturday. The amount went down by 16.

This was a 25% decrease, because 16 is 25% of 64.

Definition: Percentage Increase

A percentage increase tell how much a quantity went up, expressed as a percentage of the starting amount.

For example, Elena had $50 in the bank on Monday. She had $56 on Tuesday. The amount went up by $6.

This was a 12% increase, because 6 is 12% of 50.

Practice

Exercise $\PageIndex{5}$

For each diagram, decide if $y$ is an increase or a decrease relative to $x$. Then determine the percent increase or decrease.

Figure $\PageIndex{10}$: Tape diagram A. 4 green equal sections, all labeled x and 3 labeled y. Tape diagram B. 5 equal sections. 4 green and labeled x. 1 white section. The entire tape diagram labeled y.

Exercise $\PageIndex{6}$

Draw diagrams to represent the following situations.

The amount of flour that the bakery used this month was a 50% increase relative to last month.
The amount of milk that the bakery used this month was a 75% decrease relative to last month.

Exercise $\PageIndex{7}$

Write each percent increase or decrease as a percentage of the initial amount. The first one is done for you.

This year, there was 40% more snow than last year.
The amount of snow this year is 140% of the amount of snow last year.
This year, there were 25% fewer sunny days than last year.
Compared to last month, there was a 50% increase in the number of houses sold this month.
The runner’s time to complete the marathon was a 10% less than the time to complete the last marathon.

Exercise $\PageIndex{8}$

The graph shows the relationship between the diameter and the circumference of a circle with the point $(1,\pi$ shown. Find 3 more points that are on the line.

(From Unit 3.1.3)

Exercise $\PageIndex{9}$

Priya bought $x$ grams of flour. Clare bought $\frac{3}{8}$ more than that. Select all equations that represent the relationship between the amount of flour that Priya bought, $x$, and the amount of flour that Clare bought, $y$.

$y=\frac{3}{8}x$
$y=\frac{5}{8}x$
$y=x+\frac{3}{8}x$
$y=x-\frac{3}{8}x$
$y=\frac{11}{8}x$

(From Unit 4.1.4)

Search

Text Color

Text Size

Margin Size

Font Type

sports team	total points in game 1	total points in game 2
football team	\(22\)	\(30\)
basketball team	\(100\)	\(108\)
baseball team	\(4\)	\(12\)