5.2.4: Representing Subtraction
- Page ID
- 38326
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
Lesson
Let's subtract signed numbers.
Exercise \(\PageIndex{1}\): Equivalent Equations
Consider the equation \(2+3=5\). Here are some more equations, using the same numbers, that express the same relationship in a different way:
\(3+2=5\qquad\qquad 5-3=2\qquad\qquad 5-2=3\)
For each equation, write two more equations, using the same numbers, that express the same relationship in a different way.
- \(9+(-1)=8\)
- \(-11+x=7\)
Exercise \(\PageIndex{2}\): Subtraction with Number Lines
1. Here is an unfinished number line diagram that represents a sum of 8.

- How long should the arrow be?
- For an equation that goes with this diagram, Mai writes \(3+?=8\).
Tyler writes \(8-3=?\). Do you agree with either of them? - What is the unknown number? How do you know?
2. Here are two more unfinished diagrams that represent sums.


For each diagram:
- What equation would Mai write if she used the same reasoning as before?
- What equation would Tyler write if he used the same reasoning as before?
- How long should the other arrow be?
- What number would complete each equation? Be prepared to explain your reasoning.
3. Draw a number line diagram for \((-8)-(3)=?\) What is the unknown number? How do you know?
Exercise \(\PageIndex{3}\): We can Add Instead
1. Match each diagram to one of these expressions:
\(3+7\qquad\qquad 3-7\qquad\qquad 3+(-7)\qquad\qquad 3-(-7)\)
a.

b.

c.
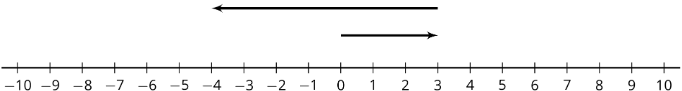
d.

2. Which expressions in the first question have the same value? What do you notice?
3. Complete each of these tables. What do you notice?
| expression | value |
|---|---|
| \(8+(-8)\) | |
| \(8-8\) | |
| \(8+(-5)\) | |
| \(8-5\) | |
| \(8+(-12)\) | |
| \(8-12\) |
| expression | value |
|---|---|
| \(-5+5\) | |
| \(-5-(-5)\) | |
| \(-5+9\) | |
| \(-5-(-9)\) | |
| \(-5+2\) | |
| \(-5-(-2)\) |
Are you ready for more?
It is possible to make a new number system using only the numbers 0, 1, 2, and 3. We will write the symbols for adding and subtracting in this system like this: \(2\oplus 1=3\) and \(2\ominus 1=1\). The table shows some of the sums.
| \(\oplus\) | 0 | 1 | 2 | 3 |
|---|---|---|---|---|
| 0 | 0 | 1 | 2 | 3 |
| 1 | 1 | 2 | 3 | 0 |
| 2 | 2 | 3 | 0 | 1 |
| 3 |
- In this system, \(1\oplus 2=3\) and \(2\oplus 3=1\). How can you see that in the table?
- What do you think \(3\oplus 1\) should be?
- What about \(3\oplus 3\)?
- What do you think \(3\ominus 1\) should be?
- What about \(2\ominus 3\)?
- Can you think of any uses for this number system?
Summary
The equation \(7-5=?\) is equivalent to \(?+5=7\). The diagram illustrates the second equation.
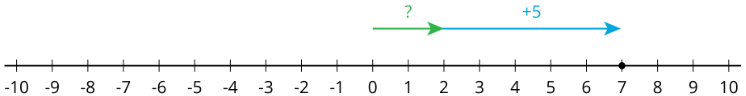
Notice that the value of \(7+(-5)\) is 2.
We can solve the equation \(?+5=7\) by adding -5 to both sides. This shows that \(7-5=7+(-5)\)
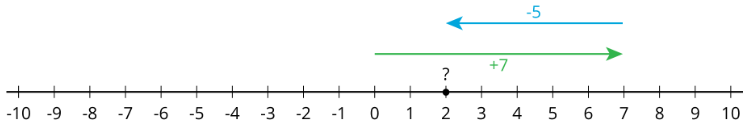
Likewise, \(3-5=?\) is equivalent to \(?+5=3\).
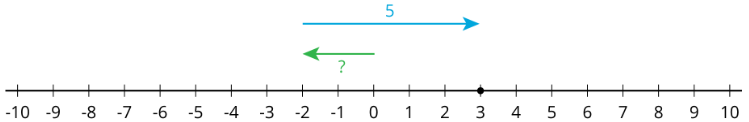
Notice that the value of \(3+(-5)\) is \(-2\).

We can solve the equation \(?+5=3\) by adding -5 to both sides. This shows that \(3-5=3+(-5)\)
In general:
\(a-b=a+(-b)\)
If \(a-b=x\), then \(x+b=a\). We can add \(-b\) to both sides of this second equation to get that \(x=a+(-b)\)
Glossary Entries
Definition: Deposit
When you put money into an account, it is called a deposit.
For example, a person added $60 to their bank account. Before the deposit, they had $435. After the deposit, they had $495, because \(435+60=495\).
Definition: Withdrawal
When you take money out of an account, it is called a withdrawal.
For example, a person removed $25 from their bank account. Before the withdrawal, they had $350. After the withdrawal, they had $325, because \(350-25=325\).
Practice
Exercise \(\PageIndex{4}\)
Write each subtraction equation as an addition equation.
- \(a-9=6\)
- \(p-20=-30\)
- \(z-(-12)=15\)
- \(x-(-7)=-10\)
Exercise \(\PageIndex{5}\)
Find each difference. If you get stuck, consider drawing a number line diagram.
- \(9-4\)
- \(4-9\)
- \(9-(-4)\)
- \(-9-(-4)\)
- \(-9-4\)
- \(4-(-9)\)
- \(-4-(-9)\)
- \(-4-9\)
Exercise \(\PageIndex{6}\)
A restaurant bill is $59 and you pay $72. What percentage gratuity did you pay?
(From Unit 4.3.1)
Exercise \(\PageIndex{7}\)
Find the solution to each equation mentally.
- \(30+a=40\)
- \(500+b=200\)
- \(-1+c=-2\)
- \(d+3,567=0\)
Exercise \(\PageIndex{8}\)
One kilogram is 2.2 pounds. Complete the tables. What is the interpretation of the constant of proportionality in each case?
| pounds | kilograms |
|---|---|
| \(2.2\) | \(1\) |
| \(11\) | |
| \(5.5\) | |
| \(1\) |
______ kilogram per pound
| kilograms | pounds |
|---|---|
| \(1\) | \(2.2\) |
| \(7\) | |
| \(30\) | |
| \(0.5\) |
______ pounds per kilogram
(From Unit 2.1.3)


