9.1E: Ellipses (Exercises)

Last updated
Save as PDF

Page ID: 20758

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $ $ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $$\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$ $\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$$\newcommand{\AA}{\unicode[.8,0]{x212B}}$

section 9.1 exercises

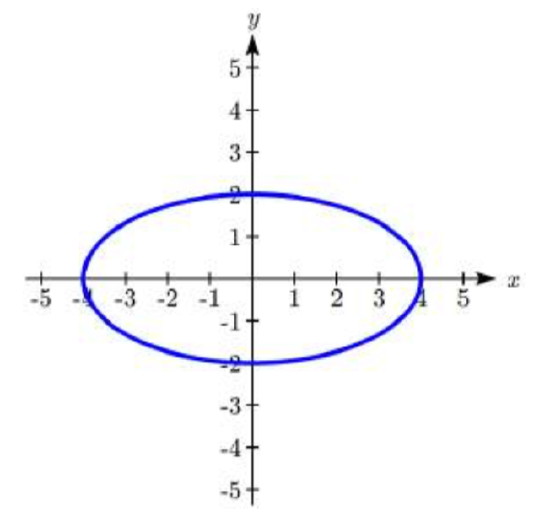
In problems 1–4, match each graph with one of the equations A–D.
A. $\dfrac{x^2}{4} + \dfrac{y^2}{9} = 1$

B. $\dfrac{x^2}{9} + \dfrac{y^2}{4} = 1$

C. $\dfrac{x^2}{9} + {y^2} = 1$

D. ${x^2} + \dfrac{y^2}{9} = 1$

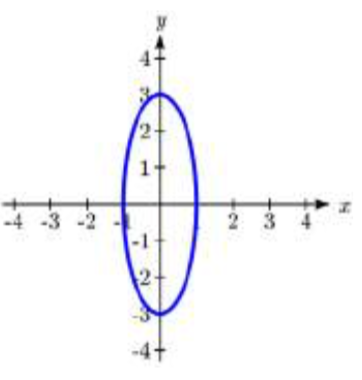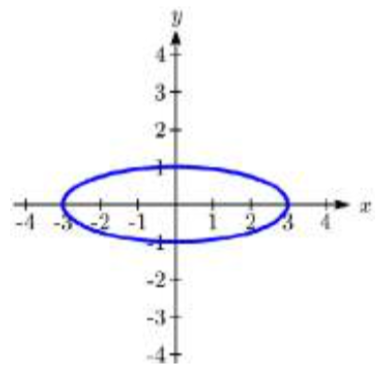1. $屏幕快照 2019-07-30 上午12.53.34.png$ 2. $屏幕快照 2019-07-30 上午12.53.52.png$ 3. $屏幕快照 2019-07-30 上午12.54.34.png$ 4. $屏幕快照 2019-07-30 上午12.55.27.png$

In problems 5–14, find the vertices, the minor axis endpoints, length of the major axis, and length of the minor axis. Sketch the graph. Check using a graphing utility.
5. $\dfrac{x^2}{4} + \dfrac{y^2}{25} = 1$

6. $\dfrac{x^2}{16} + \dfrac{y^2}{4} = 1$

7. $\dfrac{x^2}{4} + y^2 = 1$

8. $x^2 + \dfrac{y^2}{25} = 1$

9. $x^2+ 25y^2 = 25$

10. $16x^2 + y^2 = 16$

11. $16x^2 + 9y^2 = 144$

12. $16x^2 + 25y^2 = 400$

13. $9x^2 + y^2 = 18$

14. $x^2 + 4y^2 = 12$

In problems 15–16, write an equation for the graph.

15. $屏幕快照 2019-07-30 上午12.56.57.png$ 16. $屏幕快照 2019-07-30 上午12.57.36.png$

In problems 17–20, find the standard form of the equation for an ellipse satisfying the given conditions.

17. Center (0,0), horizontal major axis length 64, minor axis length 14

18. Center (0,0), vertical major axis length 36, minor axis length 18

19. Center (0,0), vertex (0,3), $b = 2$

20. Center (0,0), vertex (4,0), $b = 3$

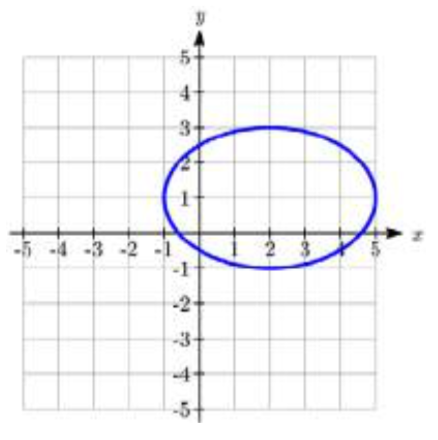
In problems 21–28, match each graph to equations A-H.
A. $\dfrac{\left( {x - 2} \right)^2}{4} + \dfrac{{(y - 1)}^2}{9} = 1$

E. $\dfrac{\left( {x + 2} \right)^2}{4} + \dfrac{{(y + 1)}^2}{9} = 1$
B. $\dfrac{\left( {x - 2} \right)^2}{4} + \dfrac{{(y - 1)}^2}{16} = 1$

F. $\dfrac{\left( {x + 2} \right)^2}{4} + \dfrac{{(y + 1)}^2}{16} = 1$
C. $\dfrac{\left( {x - 2} \right)^2}{16} + \dfrac{{(y - 1)}^2}{4} = 1$

G. $\dfrac{\left( {x + 2} \right)^2}{16} + \dfrac{{(y + 1)}^2}{4} = 1$
D. $\dfrac{\left( {x - 2} \right)^2}{9} + \dfrac{{(y - 1)}^2}{4} = 1$

H. $\dfrac{\left( {x + 2} \right)^2}{9} + \dfrac{{(y + 1)}^2}{4} = 1$

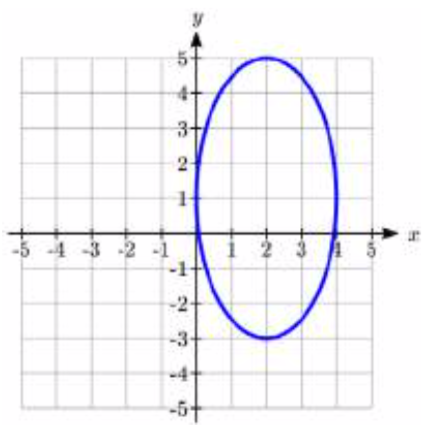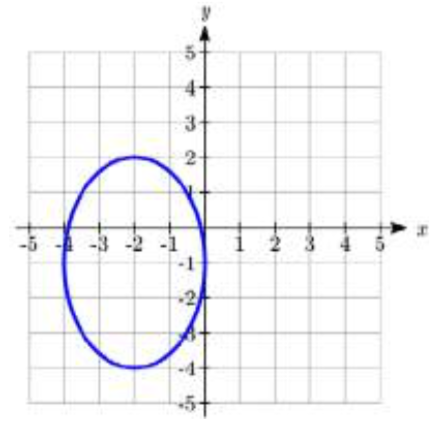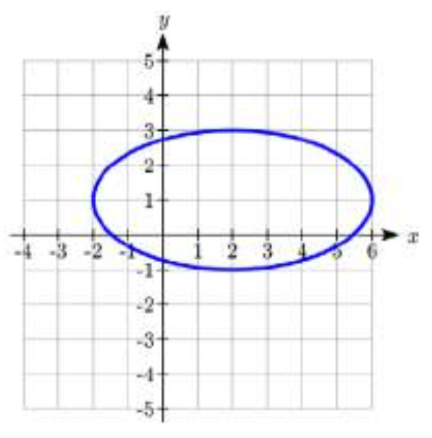21. $屏幕快照 2019-07-30 上午12.59.57.png$ 22. $屏幕快照 2019-07-30 上午1.01.19.png$ 23. $屏幕快照 2019-07-30 上午1.02.03.png$ 24. $屏幕快照 2019-07-30 上午1.02.24.png$

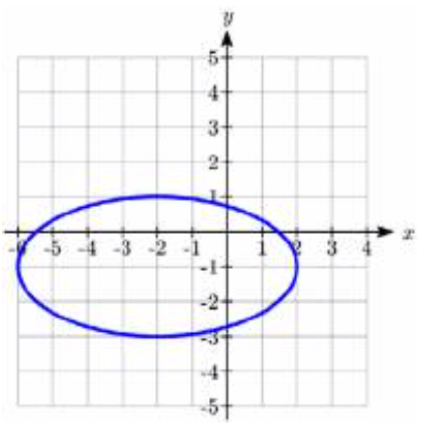25. $屏幕快照 2019-07-30 上午1.02.44.png$ 26. $屏幕快照 2019-07-30 上午1.03.28.png$ 27. $屏幕快照 2019-07-30 上午1.04.09.png$ 28. $屏幕快照 2019-07-30 上午1.04.30.png$

In problems 29–38, find the vertices, the minor axis endpoints, length of the major axis, and length of the minor axis. Sketch the graph. Check using a graphing utility.

29. $\dfrac{(x - 1)^2}{25} + \dfrac{(y + 2)^2}{4} = 1$

30. $\dfrac{(x + 5)^2}{16} + \dfrac{(y - 3)^2}{36} = 1$

31. $(x + 2)^2 + \dfrac{(y - 3)^2}{25} = 1$

32. $\dfrac{(x - 1)^2}{25} + (y - 6)^2 = 1$

33. $4x^2 + 8x + 4 + y^2 = 16$

34. $x^2 + 4y^2 + 16y + 16 = 36$

35. $x^2 + 2x + 4y^2 + 16y = - 1$

36. $4x^2 + 16x + y^2 - 8y = 4$

37. $9x^2 - 36x + 4y^2 + 8y = 104$

38. $4x^2 + 8x + 9y^2 + 36y = - 4$

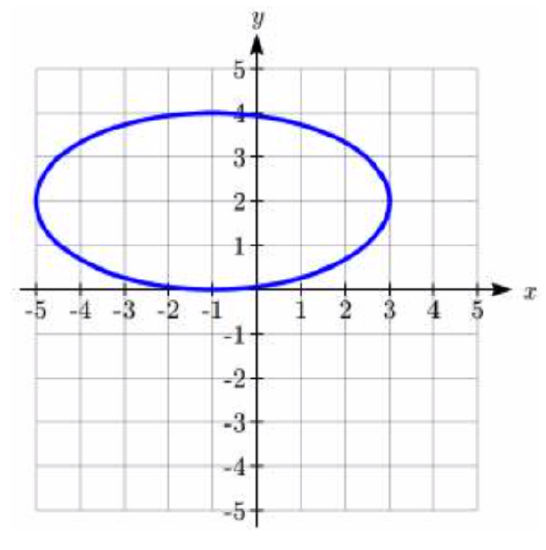
In problems 39–40, write an equation for the graph.

39. 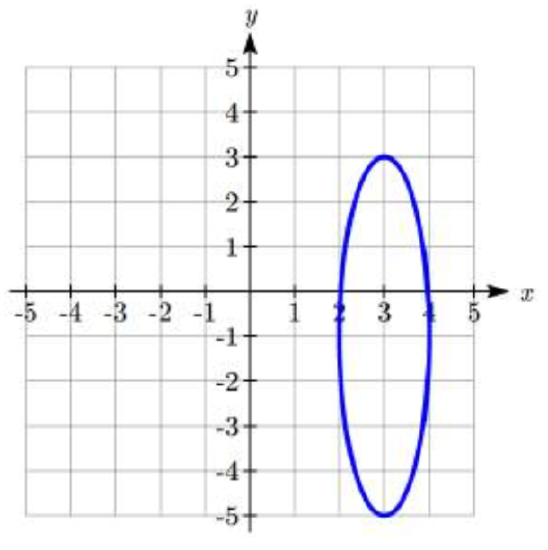$屏幕快照 2019-07-30 上午1.06.55.png$ 40. $屏幕快照 2019-07-30 上午1.07.24.png$

In problems 41–42, find the standard form of the equation for an ellipse satisfying the given conditions.

41. Center (-4, 3), vertex(-4, 8), point on the graph (0, 3)

42. Center (1, -2), vertex(-5, -2), point on the graph (1, 0)

43. Window A window in the shape of a semiellipse is 12 feet wide and 4 feet high. What is the height of the window above the base 5 feet from the center ?

44. Window A window in the shape of a semiellipse is 16 feet wide and 7 feet high. What is the height of the window above the base 4 feet from the center?

45. Bridge A bridge over a river is supported by a semielliptical arch. The river is 150 feet wide. At the center, the arch rises 60 feet above the river. The roadway is 5 feet above the center of the arch. What is the vertical distance between the roadway and the arch 45 feet from the center?

46. Bridge A bridge over a river is supported by a semielliptical arch. The river is 1250 feet wide. At the center, the arch rises 175 feet above the river. The roadway is 3 feet above the center of the arch. What is the vertical distance between the roadway and the arch 600 feet from the center?

47. Racetrack An elliptical racetrack is 100 feet long and 90 feet wide. What is the width of the racetrack 20 feet from a vertex on the major axis?

48. Racetrack An elliptical racetrack is 250 feet long and 150 feet wide. What is the width of the racetrack 25 feet from a vertex on the major axis?

In problems 49-52, find the foci.

49. $\dfrac{x^2}{19} + \dfrac{y^2}{3} = 1$ 50. $\dfrac{x^2}{2} + \dfrac{y^2}{38} = 1$

51. $(x + 6)^2 + \dfrac{(y - 1)^2}{26} + = 1$ 52. $\dfrac{(x - 3)^2}{10} + (y + 5)^2 = 1$

In problems 53-72, find the standard form of the equation for an ellipse satisfying the given conditions.

53. Major axis vertices ($\pm$3,0), $c= 2$

54. Major axis vertices (0, $\pm$7), $c= 4$

55. Foci (0, $\pm$5) and major axis length 12

56. Foci ($\pm$3, 0) and major axis length 8

57. Foci ($\pm$5, 0), vertices ($\pm$7, 0)

58. Foci (0,$\pm$2), vertices (0,$\pm$3)

59. Foci (0, $\pm$4) and $x$-intercepts ($\pm$2, 0)

60. Foci ($\pm$3, 0) and $y$-intercepts (0, $\pm$1)

61. Center (0, 0), major axis length 8, foci on $x$-axis, passes through point $\left( 2,\sqrt 6 \right)$

62. Center (0, 0), major axis length 12, foci on $y$-axis, passes through point $\left( \sqrt {10} ,4 \right)$

63. Center (-2, 1), vertex (-2, 5), focus (-2, 3)

64. Center (-1, -3), vertex (-7, -3), focus (-4, -3)

65. Foci (8, 2) and (-2, 2), major axis length 12

66. Foci (-1, 5) and (-1, -3), major axis length 14

67. Vertices (3, 4) and (3, -6), $c= 2$

68. Vertices (2, 2) and (-4, 2), $c= 2$

69. Center (1, 3), focus (0, 3), passes through point (1, 5)

70. Center (-1, -2), focus (1, -2), passes through point (2, -2)

71. Focus (-15, -1), vertices (-19, -1) and (15, -1)

72. Focus (-3, 2), vertices (-3, 4) and (-3, -8)

73. Whispering Gallery If an elliptical whispering gallery is 80 feet long and 25 feet wide, how far from the center of room should someone stand on the major axis of the ellipse to experience the whispering effect? Round to two decimal places.

74. Billiards Some billiards tables are elliptical and have the foci marked on the table. If such a one is 8 feet long and 6 feet wide, how far are the foci from the center of the ellipse? Round to two decimal places.

75. Planetary Orbits The orbits of planets around the sun are approximately elliptical with the sun as a focus. The aphelion is a planet’s greatest distance from the sun and the perihelion is its shortest. The length of the major axis is the sum of the aphelion and the perihelion. Earth’s aphelion is 94.51 million miles and its perihelion is 91.40 million miles. Write an equation for Earth’s orbit.

76. Satellite Orbits The orbit of a satellite around Earth is elliptical with Earth’s center as a focus. The satellite’s maximum height above the Earth is 170 miles and its minimum height above the Earth is 90 miles. Write an equation for the satellite’s orbit. Assume Earth is spherical and has a radius of 3960 miles.

77. Eccentricity $e$ of an ellipse is the ratio $\dfrac{c}{a}$ where $c$ is the distance of a focus from the center and a is the distance of a vertex from the center. Write an equation for an ellipse with eccentricity 0.8 and foci at (-4, 0) and (4, 0).

78. Confocal ellipses have the same foci. Show that, for $k > 0$, all ellipses of the form $\dfrac{x^2}{6 + k} + \dfrac{y^2}{k} = 1$ are confocal.

79. The latus rectum of an ellipse is a line segment with endpoints on the ellipse that passes through a focus and is perpendicular to the major axis. Show that $\dfrac{2b^2}{a}$ is the length of the latus rectum of $\dfrac{x^2}{a^2} + \dfrac{y^2}{b^2} = 1$ where $a > b$.

Answer

1. D

3. B

5. Vertices $(0, \pm 5)$, minor axis endpoints $(\pm 2, 0)$, major length = 10, minor length = 4

$Screen Shot 2019-10-17 at 7.58.53 AM.png$

7. Vertices $(\pm 5, 0)$, minor axis endpoints $(0, \pm 1)$, major length = 4, minor length = 2

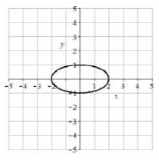
$Screen Shot 2019-10-17 at 7.59.37 AM.png$

9. Vertices $(\pm 5, 0)$, minor axis endpoints $(0, \pm 1)$, major length = 10, minor length = 2

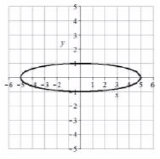
$Screen Shot 2019-10-17 at 8.00.37 AM.png$

11. Vertices $(0, \pm 4)$, minor axis endpoints $(\pm 3, 0)$, major length = 8, minor length = 6

$Screen Shot 2019-10-17 at 8.01.19 AM.png$

13. Vertices $(0, \pm 3\sqrt{2})$, minor axis endpoints $(\pm \sqrt{2}, 0)$, major length = $6\sqrt{2}$, minor length = $2\sqrt{2}$

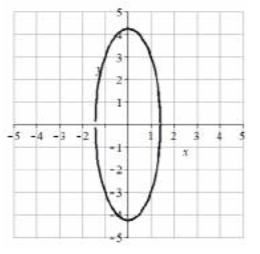
$Screen Shot 2019-10-17 at 8.02.06 AM.png$

15. $\dfrac{x^2}{16} + \dfrac{y^2}{4} = 1$

17. $\dfrac{x^2}{1024} + \dfrac{y^2}{49} = 1$

19. $\dfrac{x^2}{4} + \dfrac{y^2}{9} = 1$

21. B

23. C

25. F

27. G

29. Center (1, -2), vertices (6, -2) and (-4, -2), minor axis endpoints (1, 0) and (1, -4), major length= 10, minor length = 4

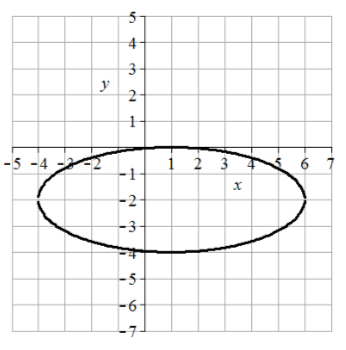
$Screen Shot 2019-10-17 at 8.03.50 AM.png$

31. Center (-2, 3), vertices (-2, 8) and (-2, -2), minor axis endpoints (-1, 3) and (-3, 3), major length = 10, minor length = 2

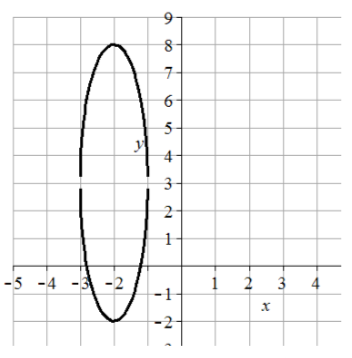
$Screen Shot 2019-10-17 at 8.04.27 AM.png$

33. Center (-1, 0), vertices (-1, 4) and (-1, -4), minor axis endpoints (-1, 0) and (3, 0), major length = 8, minor length = 4

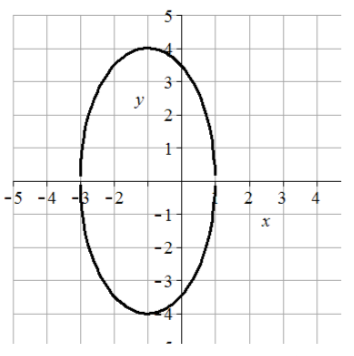
$Screen Shot 2019-10-17 at 8.05.06 AM.png$

35. Center (-1, -2), vertices (3, -2) and (-5, -2), minor axis endpoints (-1, 0) and (-1, -4), major length = 8, minor length = 4

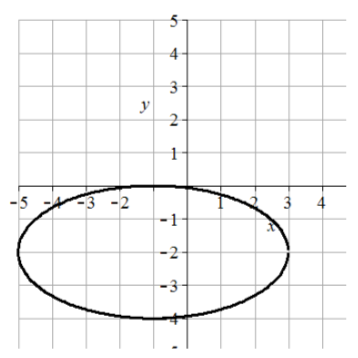
$Screen Shot 2019-10-17 at 8.05.40 AM.png$

37. Center (2, -1), vertices (2, 5) and (2, -7), minor axis endpoints (6, -1) and (-2, -1), major length = 12, minor length = 8

$Screen Shot 2019-10-17 at 8.06.09 AM.png$

39. $(x - 3)^2 + \dfrac{(y + 1)^2}{16} = 1$

41. $\dfrac{(x + 4)^2}{16} + \dfrac{(y - 3)^2}{25} = 1$

43. 2.211083 feet

45. 17 feet

47. 64 feet

49. $(\pm 4, 0)$

51. (-6, 6) and (-6, -4)

53. $\dfrac{x^2}{9} + \dfrac{y^2}{5} = 1$

55. $\dfrac{x^2}{11} + \dfrac{y^2}{36} = 1$

57. $\dfrac{x^2}{49} + \dfrac{y^2}{24} = 1$

59. $\dfrac{x^2}{4} + \dfrac{y^2}{20} = 1$

61. $\dfrac{x^2}{16} + \dfrac{y^2}{8} = 1$

63. $\dfrac{(x + 2)^2}{12} + \dfrac{(y - 1)^2}{16} = 1$

65. $\dfrac{(x - 3)^2}{36} + \dfrac{(y - 2)^2}{11} = 1$

67. $\dfrac{(x - 3)^2}{21} + \dfrac{(y + 1)^2}{25} = 1$

69. $\dfrac{(x - 1)^2}{4} + \dfrac{(y - 3)^2}{5} = 1$

71. $\dfrac{(x + 2)^2}{289} + \dfrac{(y + 1)^2}{120} = 1$

73. 31.22 feet

75. $\dfrac{x^2}{8640.632025} + \dfrac{y^2}{8638.214} = 1$

77. $\dfrac{x^2}{25} + \dfrac{y^2}{9} = 1$

79. The center is at (0, 0). Since $a > b$, the ellipse is horizontal. Let ($c$, 0) be the focus on the positive x-axis. Let ($c, h$) be the endpoint in Quadrant 1 of the latus rectum passing through ($c$,0).

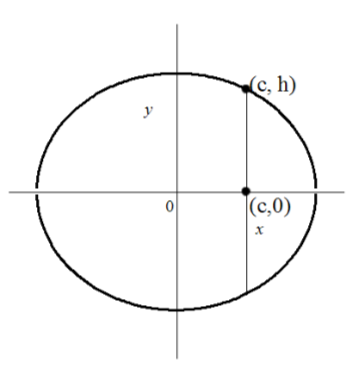
$Screen Shot 2019-10-17 at 8.25.17 AM.png$

The distance between the focus and latus rectum endpoint can be found by substituting ($c$,0) and $(c,h)$ into the distance formula $h = \sqrt{(x_1 - x_2)^2 + (y_1 - y_2)^2}$ which yields $h = \sqrt{(c - c)^2 + (h - 0)^2} = h$. So $h$ is half the latus rectum distance. Substituting ($c$, $h$) into the ellipse equation to find $h$ gives $\dfrac{c^2}{a^2} + \dfrac{h^2}{b^2} = 1$. Solve for $h$ yields $h^2 = b^2(1 - \dfrac{c^2}{a^2}) = b^2 (\dfrac{a^2}{a^2} - \dfrac{c^2}{a^2}) = b^2 (\dfrac{a^2 - c^2}{a^2}) = b^2(\dfrac{b^2}{a^2}) = \dfrac{b^4}{a^2}$. so $h = \sqrt{\dfrac{b^4}{a^2}} = \dfrac{b^2}{a}$. The distance of the latus rectum is $2h = \dfrac{2b^2}{a}$.