1.4E: Composition of Functions

SECTION 1.4 EXERCISE

Given each pair of functions, calculate \(f(g(0))\) and \(g(f(0))\).

1. \(f(x) = 4x + 8, g(x) = 7 - x^{2}\)

2. \(f(x) = 5x + 7, g(x) = 4 - 2x^{2}\)

3. \(f(x) = \sqrt{x + 4} , g(x) = 12 - x^{3}\)

4. \(f(x) = \dfrac{1}{x + 2} , g(x) = 4x + 3\)

Use the table of values to evaluate each expression

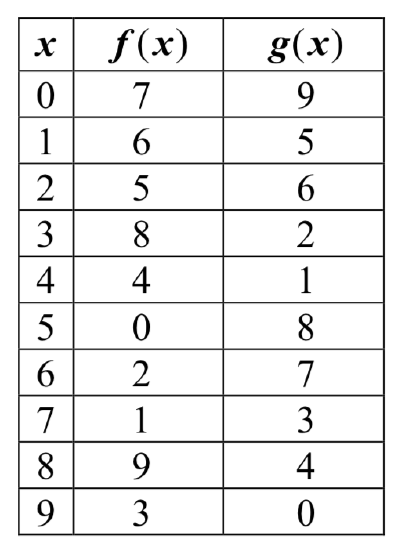
5. \(f(g(8))\)

6. \(f(g(5))\)

7. \(g(f(5))\)

8. \(g(f(3))\)

9. \(f(f(4))\)

10. \(f(f(1))\)

11. \(g(g(2))\)

12. \(g(g(6))\)

Use the graphs to evaluate the expressions below.

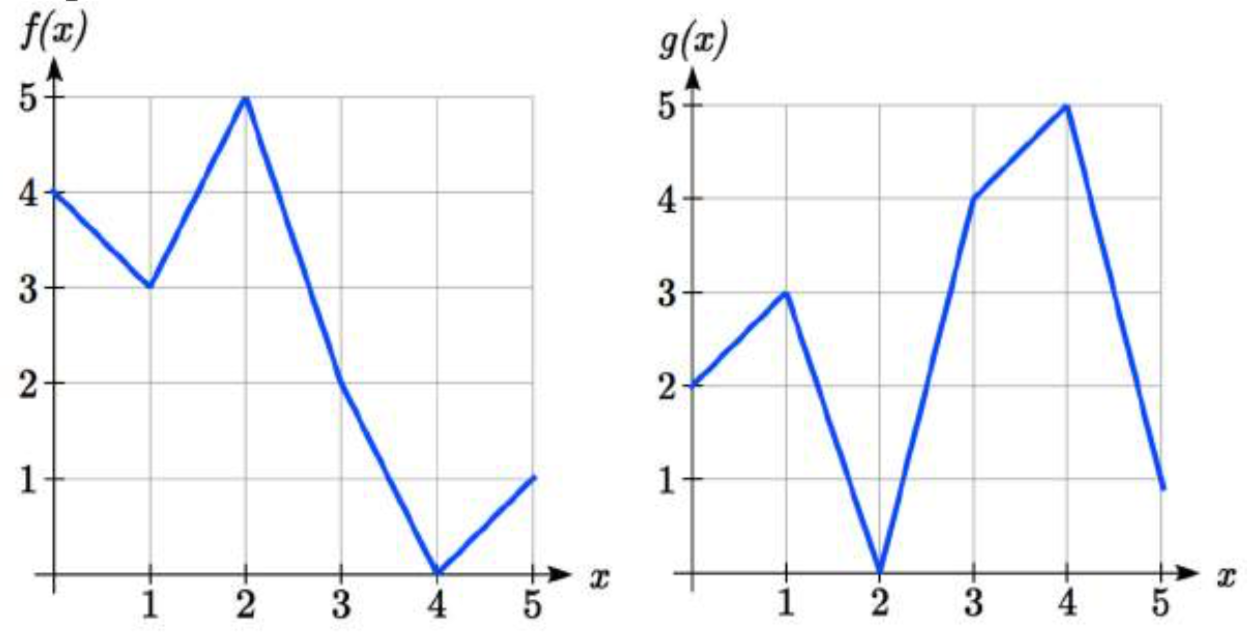
13. \(f(g(3))\)

14. \(f(g(1))\)

15. \(g(f(1))\)

16. \(g(f(0))\)

17. \(f(f(5))\)

18. \(f(f(4))\)

19. \(g(g(2))\)

20. \(g(g(0))\)

For each pair of functions, find \(f(g(x))\) and \(g(f(x))\). Simplify your answers.

21. \(f(x) = \dfrac{1}{x - 6}, g(x) = \dfrac{7}{x} + 6\)

22. \(f(x) = \dfrac{1}{x-4} , g(x) = \dfrac{2}{x} + 4\)

23. \(f(x) = x^{2} + 1, g(x) = \sqrt{x+2}\)

24. \(f(x) = \sqrt{x} +2, g(x) = x^{2} +3\)

25. \(f(x) = |x|, g(x) = 5x + 1\)

26. \(f(x)=\sqrt[{3}]{x} , g(x) = \dfrac{x+1}{x^{3} }\)

27. If \(f(x) = x^{4} +6\), \(g(x) = x - 6\) and \(h(x) = \sqrt{x}\), find \(f(g(h(x)))\)

28. If \(f(x) = x^{2} +1\), \(g(x) = \dfrac{1}{x}\) and \(h(x) = x + 3\), find \(f(g(h(x)))\)

29. The function \(D(p)\) gives the number of items that will be demanded when the price is \(p\). The production cost, \(C(x)\) is the cost of producing \(x\) items. To determine the cost of production when the price is $6, you would do which of the following:

a. Evaluate \(D(C(6))\)
b. Evaluate \(C(D(6))\)
c. Solve \(D(C(x)) = 6\)
d. Solve \(C(D(p)) = 6\)

20. The function \(A(d)\) gives the pain level on a scale of 0-10 experienced by a patient with \(d\) milligrams of a pain reduction drug in their system. The milligrams of drug in the patient’s system after t minutes is modeled by \(m(t)\). To determine when the patient will be at a pain level of 4, you would need to:

a. Evaluate \(A(m(4))\)
b. Evaluate \(m(A(4))\)
c. Solve \(A(m(t) = 4\)
d. Solve \(m(A(d)) = 4\)

31. The radius \(r\), in inches, of a spherical balloon is related to the volume, \(V\), by \(r(V)=\sqrt[{3}]{\dfrac{3V}{4\pi } }\). Air is pumped into the balloon, so the volume after \(t\) seconds is given by \(V(t) = 10 + 20t\).

a. Find the composite function \(r(V(t))\)

b. Find the radius after 20 seconds

32. The number of bacteria in a refrigerated food product is given by \(N(T) = 23T^{2} - 56T + 1\), \(3 < T < 33\), where \(T\) is the temperature of the food. When the food is removed from the refrigerator, the temperature is given by \(T(t) = 5t + 1.5\), where t is the time in hours.

a. Find the composite function \(N\left(T\left(t\right)\right)\)
b. Find the bacteria count after 4 hours

33. Given \(p(x) = \dfrac{1}{\sqrt{x} }\) and \(m(x) = x^{2} -4\), find the domain of \(m(p(x)\).

34. Given \(p(x) = \dfrac{1}{\sqrt{x} }\) and \(m(x) = 9 - x^{2}\), find the domain of \(m(p(x))\).

35. Given \(f(x) = \dfrac{1}{x+3}\) and \(g(x) = \dfrac{2}{x - 1}\), find the domain of \(f(g(x))\).

36. Given \(f(x) = \dfrac{x}{x+1}\) and \(g(x)=\dfrac{4}{x}\), find the domain of \(f(g(x))\).

37. Given \(f(x)=\sqrt{x-2}\) and \(g(x)=\dfrac{2}{x^{2} -3}\), find the domain of \(g(f(x))\).

38. Given \(f(x)=\sqrt{4-x}\) and \(g(x)=\dfrac{1}{x^{2} -2}\), find the domain of \(g(f(x))\).

Find functions \(f(x)\) and \(g(x)\) so the given function can be expressed as \(h(x)=f(g(x))\).

39. \(h(x)=(x+2)^{2}\)

40. \((x)=(x-5)^{3}\)

41. \((x)=\dfrac{3}{x-5}\)

42. \(h(x)=\dfrac{4}{(x+2)^{2}}\)

43. \(h(x)=3+\sqrt{x-2}\)

44. \(h(x)=4+\sqrt[{3}]{x}\)

45. Let \(f(x)\) be a linear function, with form \(f(x)=ax+b\) for constants \(a\) and \(b\). [UW]

a. Show that \(f\left(f\left(x\right)\right)\) is a linear function
b. Find a function \(g(x)\) such that \(g\left(g\left(x\right)\right)=6x-8\)

46. Let \(f(x)=\dfrac{1}{2} x+3\) [UW]

a. Sketch the graphs of \(f(x)\), \(f(f(x))\), \(f(f(f(x)))\) on the interval \(-2 \le x \le 10\)
b. Your graphs should all intersect at the point (6, 6). The value x = 6 is called a fixed point of the function \(f(x)\) since \(f(6) = 6\); that is, 6 is fixed - it doesn’t move when \(f\) is applied to it. Give an explanation for why 6 is a fixed point for any function \(f(f(f(...f(x)...)))\).
c. Linear functions (with the exception of \(f(x)=x\)) can have at most one fixed point. Quadratic functions can have at most two. Find the fixed points of the function \(g(x)=x^{2} -2\).
d. Give a quadratic function whose fixed points are \(x = -2\) and \(x = 3\).

47. A car leaves Seattle heading east. The speed of the car in mph after \(m\) minutes is given by the function \(C(m)=\dfrac{70m^{2} }{10+m^{2} }\). [UW]

a. Find a function \(m=f(s)\) that converts seconds \(s\) into minutes \(m\). Write out the formula for the new function \(C(f(s))\); what does this function calculate?
b. Find a function \(m=g(h\)) that converts hours \(h\) into minutes \(m\). Write out the formula for the new function \(C(g(h))\); what does this function calculate?
c. Find a function \(z=v(s)\) that converts mph \(s\) into ft/sec \(z\). Write out the formula for the new function \(v(C(m)\); what does this function calculate?

Answer

1. \(f(g(0)) = 36\). \(g(f(0)) = -57\)

3. \(f(g(0)) = 4\). \(g(f(0)) = 4\)

5. 4

7. 9

11. 7

13. 0

15. 4

17. 3

19. 2

21. \(f(g(x)) = \dfrac{x}{7}\) \(g(f(x)) = 7x - 36\)

23. \(f(g(x)) = x + 3\) \(g(f(x)) = \sqrt{x^2 + 3}\)

25. \(f(g(x)) = |5x + 1|\) \(g(f(x)) = 5|x| + 1\)

27. \(f(g(h(x))) = (\sqrt{x} - 6)^4 + 6\)

29. b

31. a. \(r(V(t)) = \sqrt[3]{\dfrac{3(10 + 20t)}{4\pi}}\)
b. 4.609 in

33. \((0, \infty)\)

35. \((-\infty, \dfrac{1}{3}) \cup (\dfrac{1}{3}, 1) \cup (1, \infty)\)

37. \([2, 5) \cup (5, \infty)\)

39. \(g(x) = x + 2\), \(f(x) = x^2\)

41. \(f(x) = \dfrac{3}{x}\), \(g(x) = x - 5\)

43. \(f(x) = 3 + \sqrt{x}\), \(g(x) = x - 2\), or \(f(x) = 3 + x\), \(g(x) = \sqrt{x - 2}\)

45. a. \(f(f(x)) = a(ax + b) + b = (a^2)x + (ab + b)\)
b. \(g(x) = \sqrt{6} x - \dfrac{8}{\sqrt{6} + 1}\) or \(g(x) = -\sqrt{6} x - \dfrac{8}{1 - \sqrt{6}}\)

47. a. \(C(f(s)) = \dfrac{70(\dfrac{s}{60})^2}{10 + (\dfrac{s}{60})^2}\)
b. \(C(g(h)) = \dfrac{70(60h)^2}{10 + (60h)^2}\)
c. \(v(C(m)) = \dfrac{5280}{3600} (\dfrac{70m^2}{10 + m^2})\)