1.5.1: Transformation of Functions
( \newcommand{\kernel}{\mathrm{null}\,}\)
Section 1.5 Exercises
Describe how each function is a transformation of the original function f(x)
1. f(x−49)
2. f(x+43)
3. f(x+3)
4. f(x−4)
5. f(x)+5
6. f(x)+8
7. f(x)−2
8. f(x)−7
9. f(x−2)+3
10. f(x+4)−1
11. Write a formula for f(x)=√x shifted up 1 unit and left 2 units.
12. Write a formula for f(x)=|x| shifted down 3 units and right 1 unit.
13. Write a formula for f(x)=1x shifted down 4 units and right 3 units.
14. Write a formula for f(x)=1x2 shifted up 2 units and left 4 units.
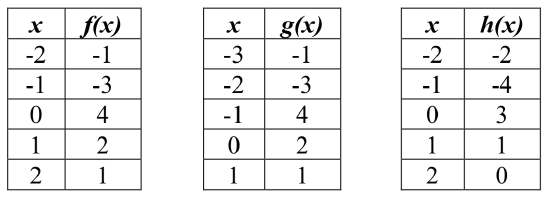
15. Tables of values for f(x), g(x), and h(x) are given below. Write g(x) and h(x) as transformations of f(x).
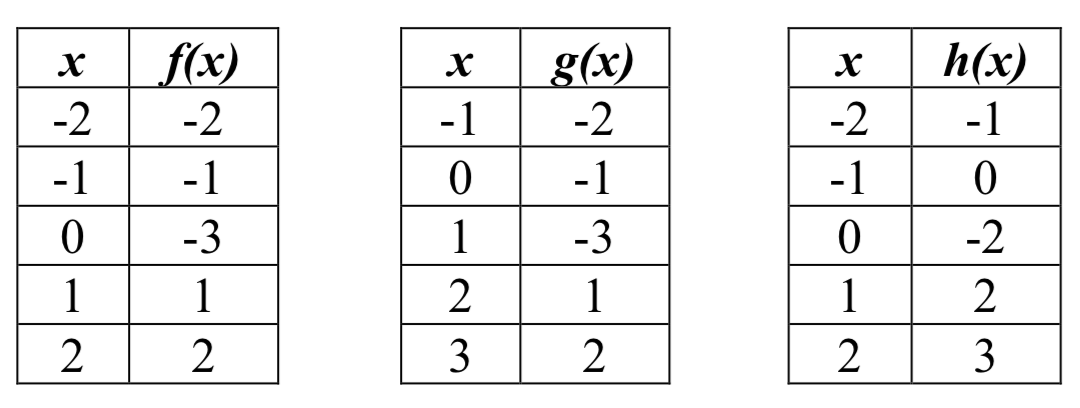
16. Tables of values for f(x), g(x), and h(x) are given below. Write g(x) and h(x) as transformations of f(x).
The graph of f(x)=2x is shown. Sketch a graph of each transformation of f(x).
17. g(x)=2x+1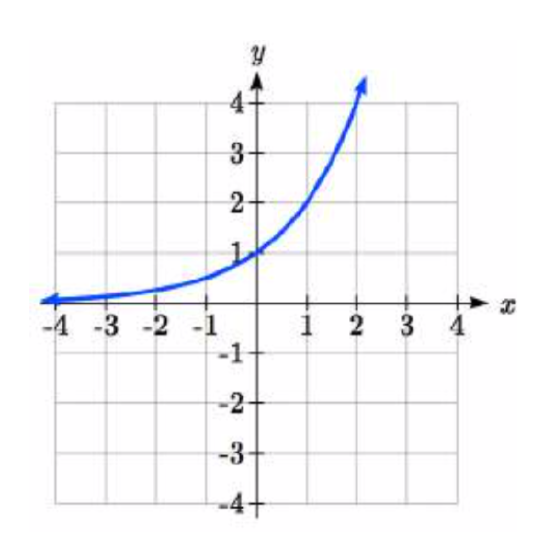
18. h(x)=2x−3
19. w(x)=2x−1
20. q(x)=2x+3
Sketch a graph of each function as a transformation of a toolkit function.
21. f(t)=(t+1)2−3
22. h(x)=|x−1|+4
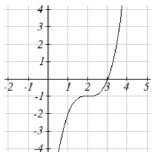
23. k(x=(x−2)3−1
24. m(t)=3+√t+2
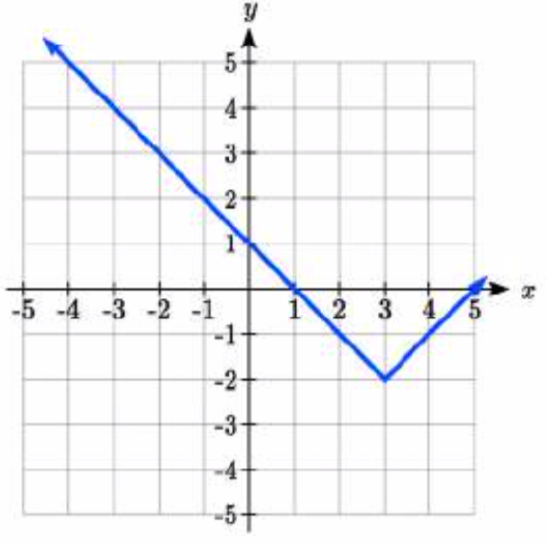
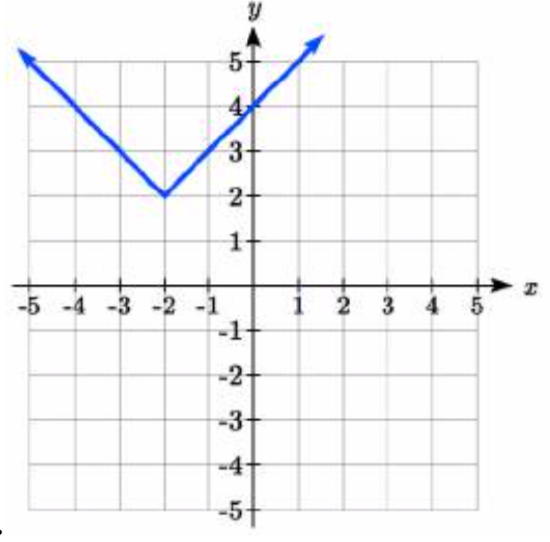
Write an equation for each function graphed below.
25. 
26. 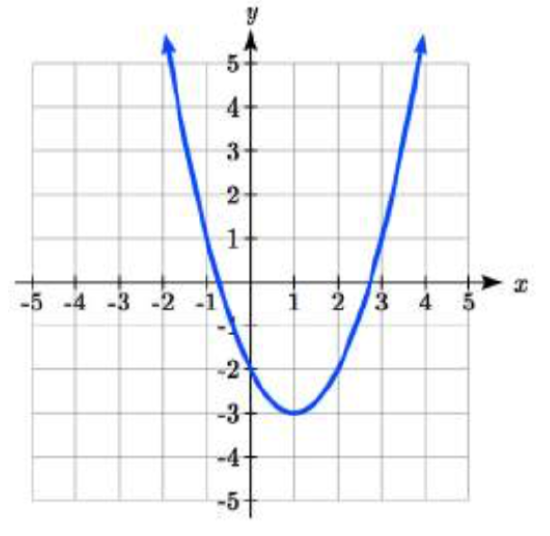
27. 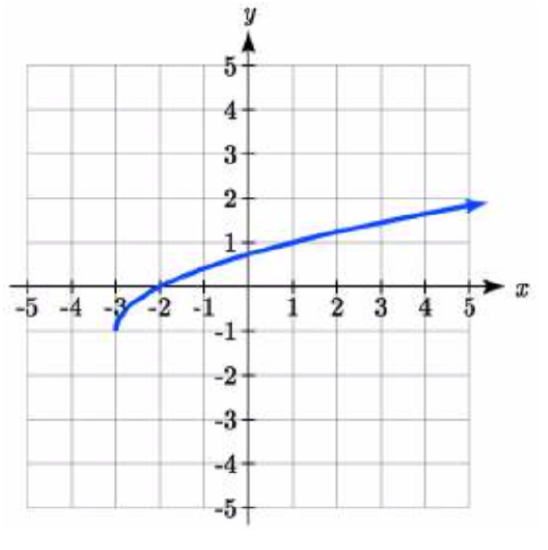
28.
Find a formula for each of the transformations of the square root whose graphs are given below.
29. 
30. 
The graph of f(x)=2x is shown. Sketch a graph of each transformation of f(x)

31. g(x)=−2x+1
32. h(x)=2−x
33. Starting with the graph of f(x)=6x write the equation of the graph that results from
a. reflecting f(x) about the x-axis and the y-axis
b. reflecting f(x) about the x-axis, shifting left 2 units, and down 3 units
34. Starting with the graph of f(x)=4x write the equation of the graph that results from
a. reflecting f(x) about the x-axis
b. reflecting f(x) about the y-axis, shifting right 4 units, and up 2 units
Write an equation for each function graphed below.
35. 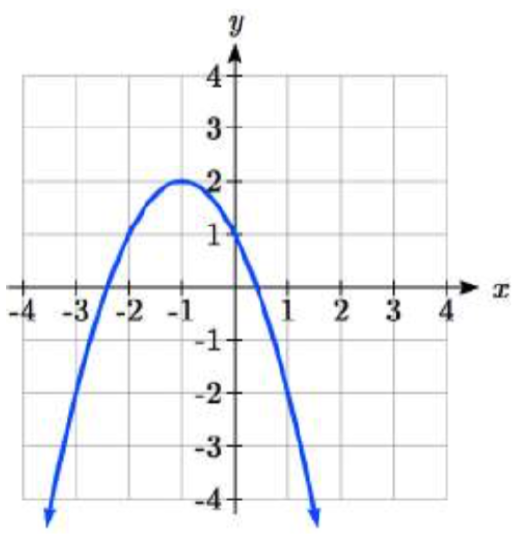 36.
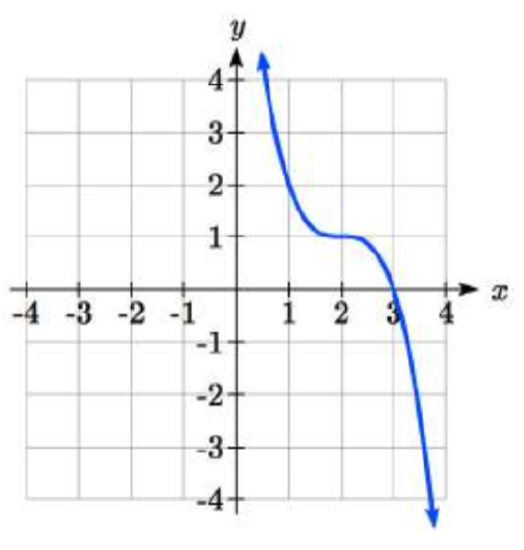
36. 
37.  38.
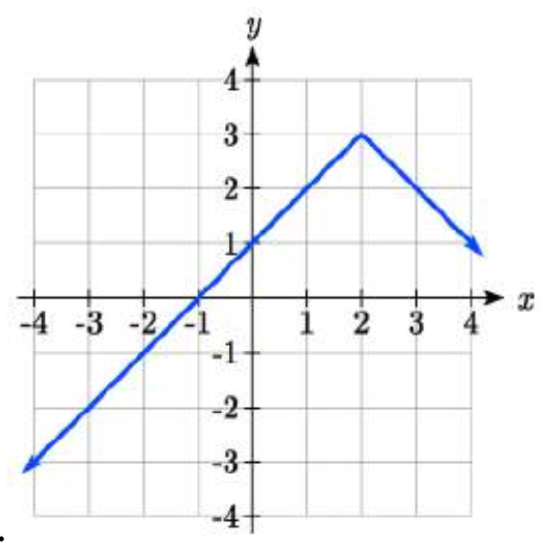
38.
39. For each equation below, determine if the function is Odd, Even, or Neither.
a. f(x)=3x4
b. g(x)=√x
c. h(x)=1x+3x
40. For each equation below, determine if the function is Odd, Even, or Neither.
a. f(x)=(x−2)2
b. g(x)=2x4
c. h(x)=2x−x3
Describe how each function is a transformation of the original function f(x).
41. −f(x)
42. f(−x)
43. 4f(x)
44. 6f(x)
45. f(5x)
46. f(2x)
47. f(13x)
48. f(15x)
49. 3f(−x)
50. −f(3x)
Write a formula for the function that results when the given toolkit function is transformed as described.
51. f(x)=|x| reflected over the y axis and horizontally compressed by a factor of 14.
52. f(x)=√x reflected over the x axis and horizontally stretched by a factor of 2.
53. f(x)=1x2 vertically compressed by a factor of 13, then shifted to the left 2 units and down 3 units.
54. f(x)=1x vertically stretched by a factor of 8, then shifted to the right 4 units and up 2 units.
55. f(x)=x2 horizontally compressed by a factor of 12, then shifted to the right 5 units and up 1 unit.
56. f(x)=x2 horizontally stretched by a factor of 3, then shifted to the left 4 units and down 3 units.
Describe how each formula is a transformation of a toolkit function. Then sketch a graph of the transformation.
57. f(x)=4(x+1)2−5
58. g(x)=5(x+3)2−2
59. h(x)=−2|x−4|+3
60. k(x)=−3√x−1
61. m(x)=12x3
62. n(x)=13|x−2|
63. p(x)=(13x)2−3
64. q(x)=(14x)3+1
65. a(x)=√−x+4
66. b(x)=3√−x−6
Determine the interval(s) on which the function is increasing and decreasing.
67. f(x)=4(x+1)2−5
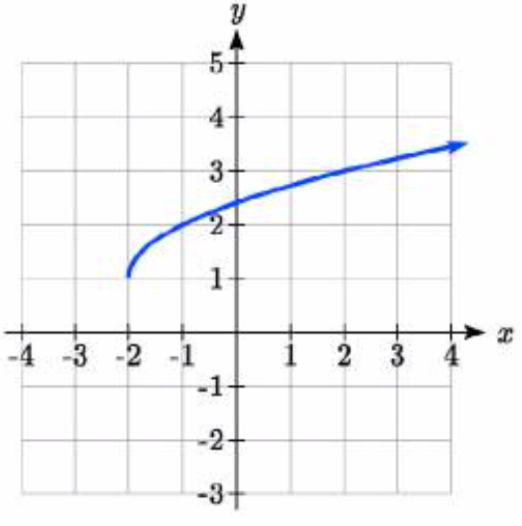
68. g(x)=5(x+3)2−2
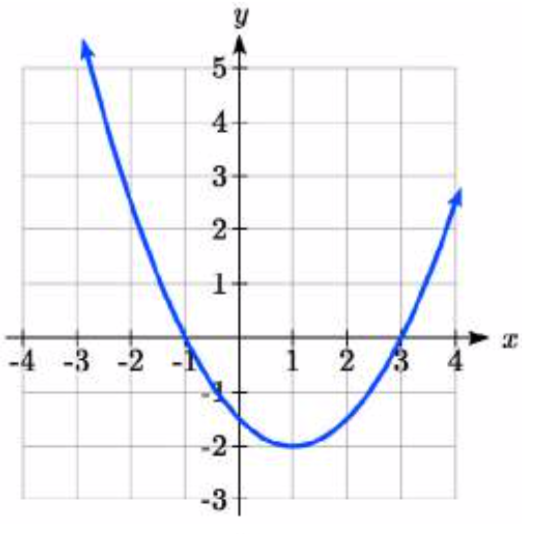
69. a(x)=√−x+4
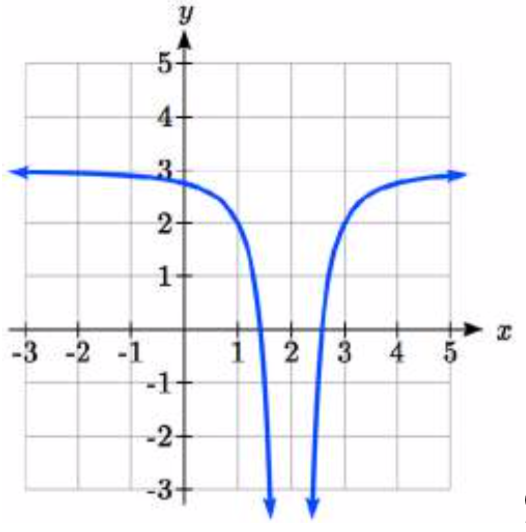
70. k(x)=−3√x−1
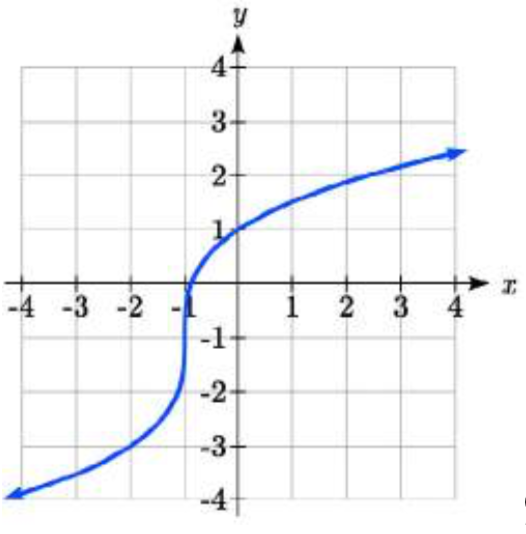
Determine the interval(s) on which the function is concave up and concave down.
71. m(x)=−2(x+3)3+1
72. b(x)=3√−x−6
73. p(x)=(13x)2−3
74. k(x)=−3√x−1
The function f(x) is graphed here. Write an equation for each graph below as a transformation of f(x).
75.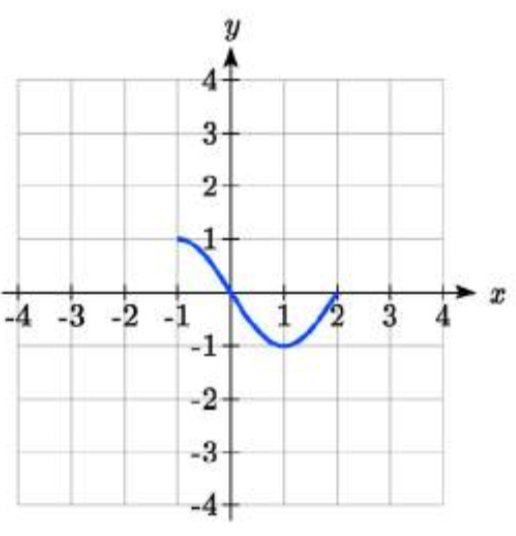 76.
76.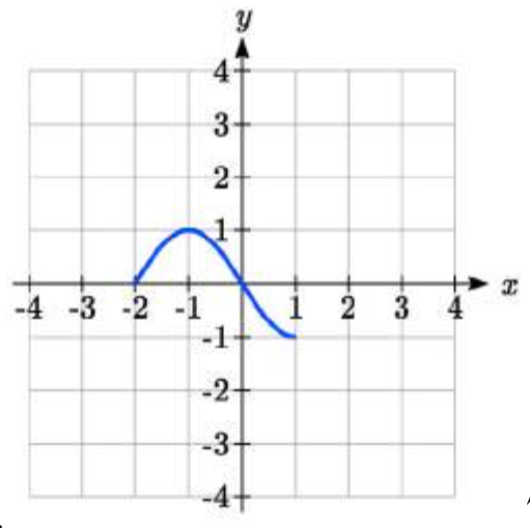 77.
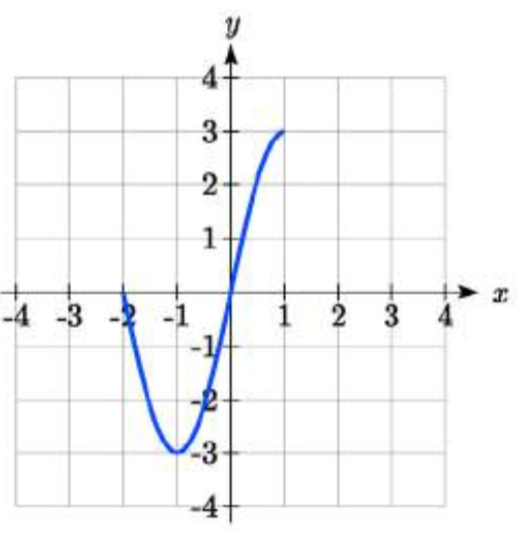
77.
78.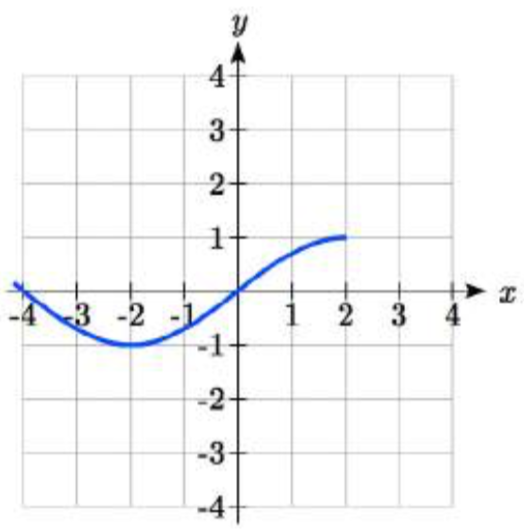 79.
79. 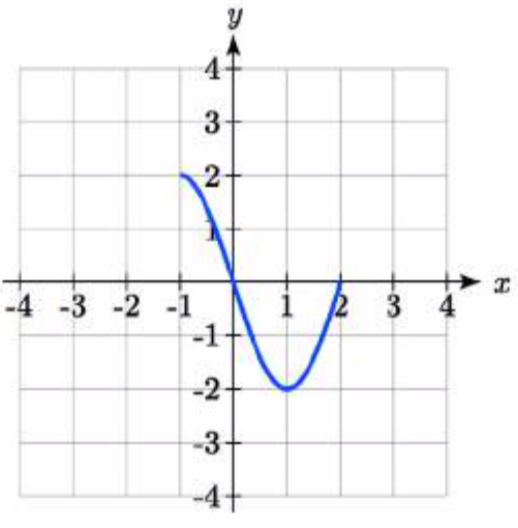 80.
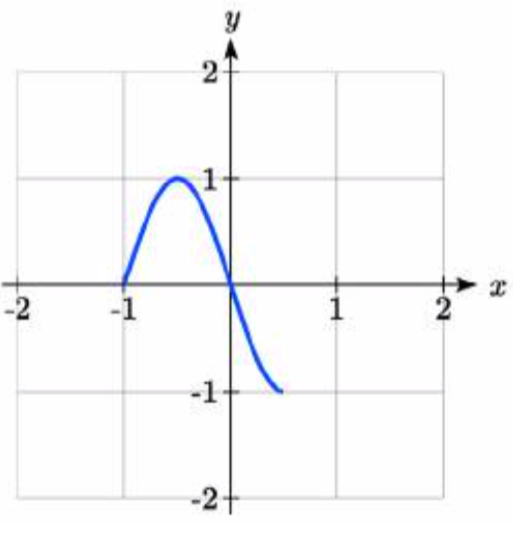
80. 
81.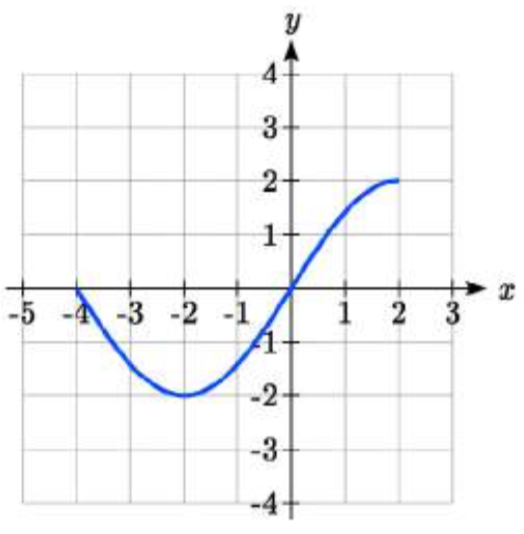 82.
82.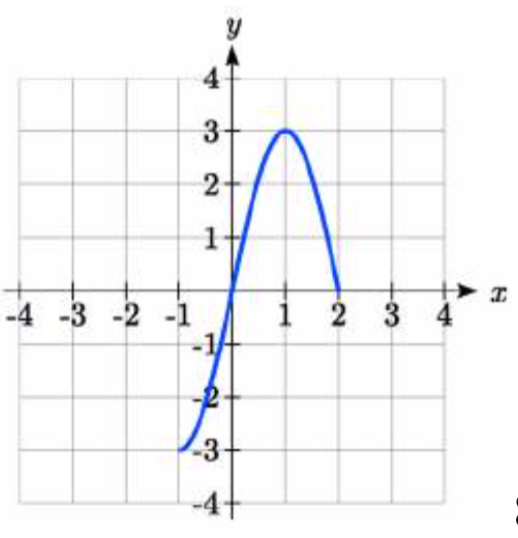 83.
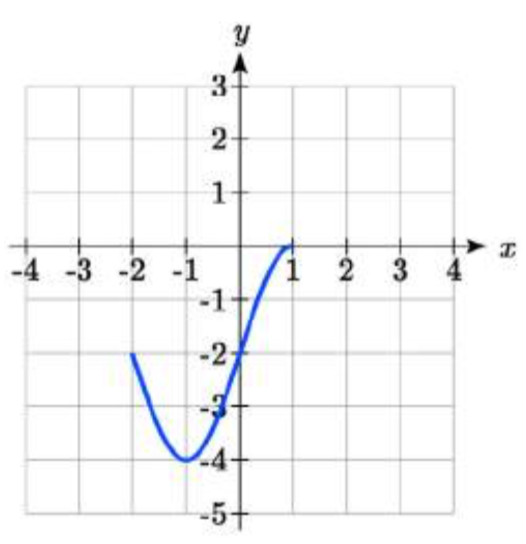
83. 
84. 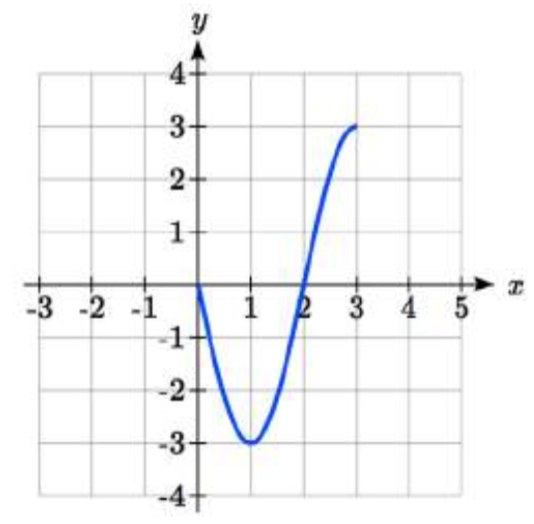 85.
85.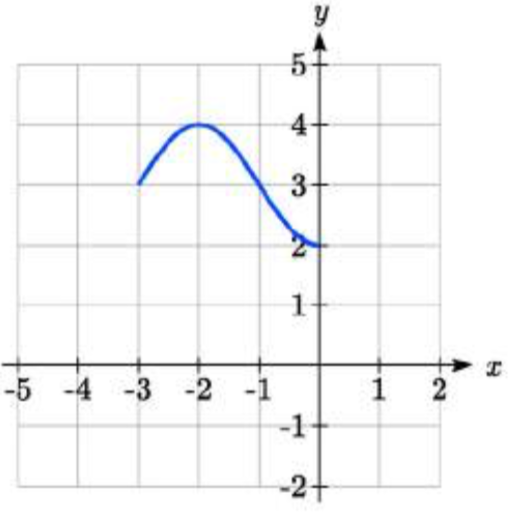 86.
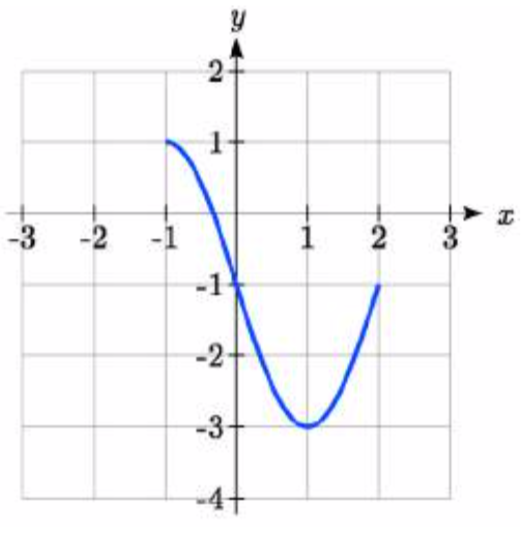
86.
Write an equation for each transformed toolkit function graphed below.
87.  88.
88.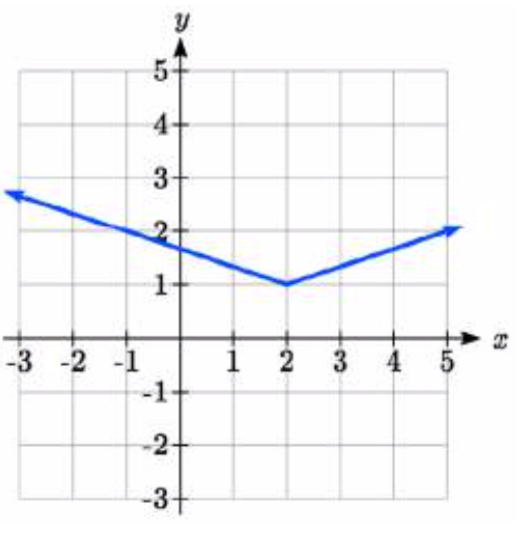 89.
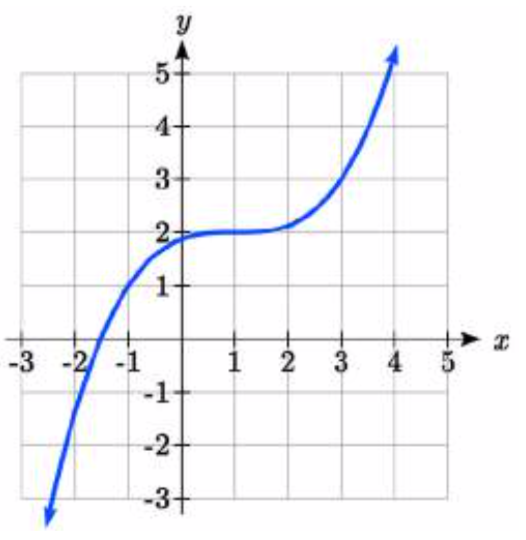
89. 
90.  91.
91.  92.
92.
93.  94.
94. 95.
95. 
96. 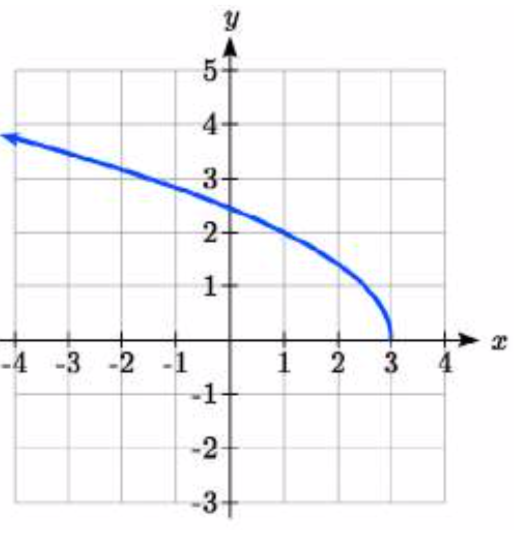 97.
97. 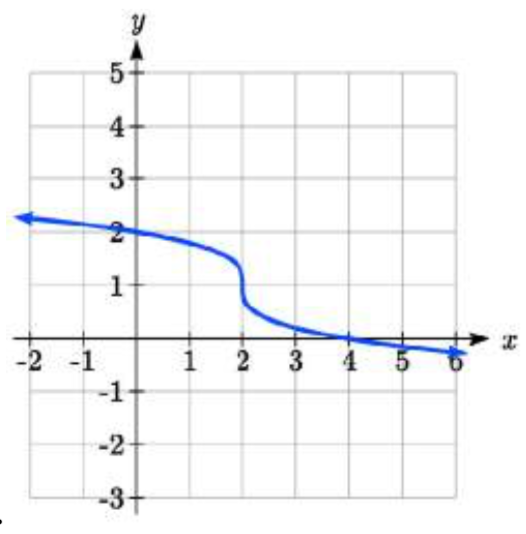 98.
98.
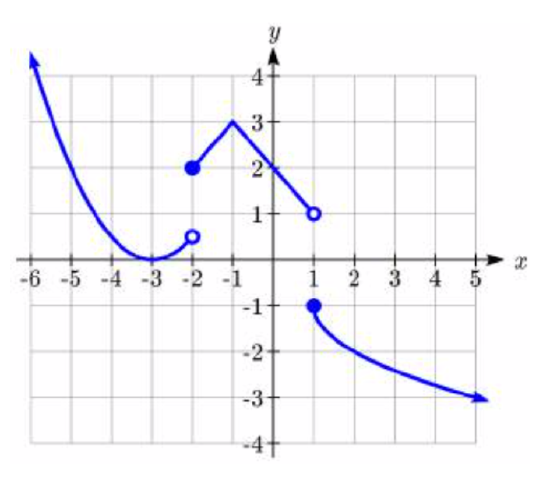
Write a formula for the piecewise function graphed below.
99. 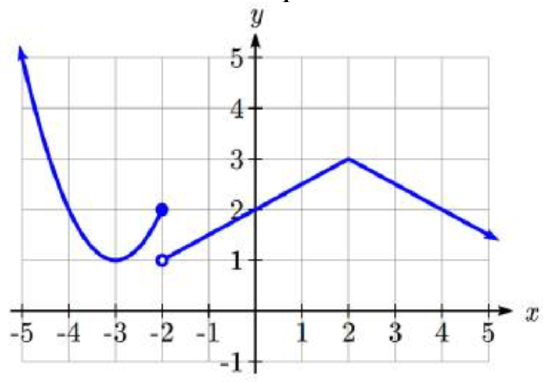 100.
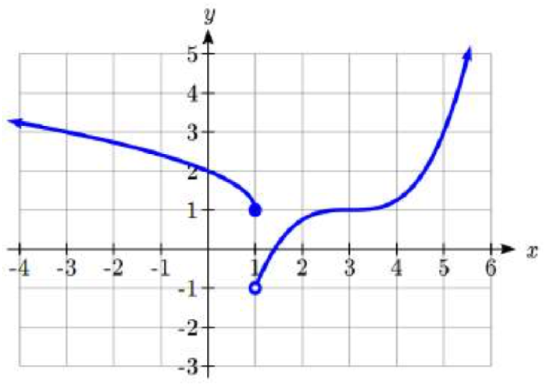
100. 
101. 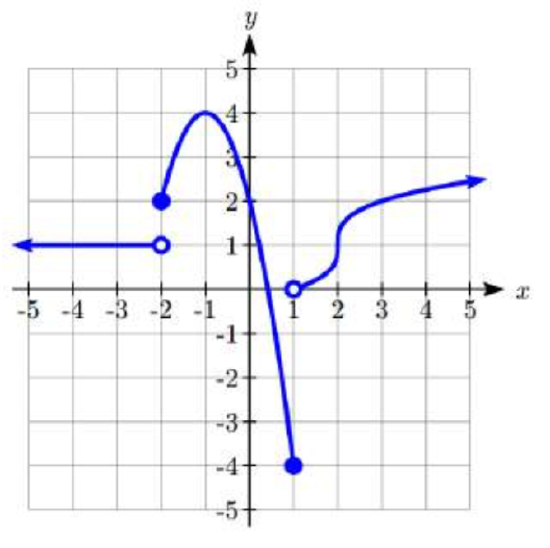 102.
102. 
103. Suppose you have a function y=f(x) such that the domain of f(x) is 1≤x≤6 and the range of f(x) is (-3 \le y \le 5\). [UW]
a. What is the domain of f(2(x−3))?
b. What is the range of f(2(x−3)) ?
c. What is the domain of 2f(x)−3 ?
d. What is the range of 2f(x)−3 ?
e. Can you find constants B and C so that the domain of f(B(x−C)) is 8≤x≤9?
f. Can you find constants A and D so that the range of Af(x)+D is 0 0≤y≤1?
- Answer
-
1. Horizontal shift right 49 units
3. Horizontal shift left 3 units
5. Vertical shift up 5 units
7. Vertical shift down 2 units
9. Horizontal shift right 2 units, Vertical shift up 3 units
11. f(x+2)+1=√x+2+1
13. f(x−3)−4=1x−3−4
15. g(x)=f(x−1), h(x)=f(x)+1
17.
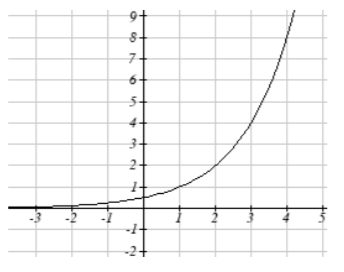
 19.
19. 
21.
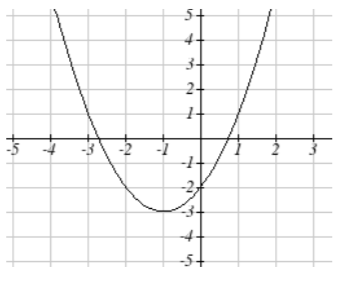 23.
23. 
25. y=|x−3|−2
27. y=√x+3−1
29. y=−√x
31.

33. a. −f(−x)=−6−x
b. −f(x+2)−3=−6x+2−335. y=−(x+1)2+2
37. y=√−x+1
39. a. Even
b. Neither
c. Odd41. Reflect f(x) about the x-axis
43. Vertically stretch y values by 4
45. Horizontally compress x values by 1/5
47. Horizontally stretch x values by 3
49. Reflect f(x) about the y-axis and vertically stretch y values by 3
51. f(−4x)=|−4x|
53. 13f(x+2)−3=13(x+2)2−3
55. f(2(x−5))+1=(2(x−5))2+1
57. Horizontal shift left 1 unit, vertical stretch y values by 4, vertical shift down 5 units
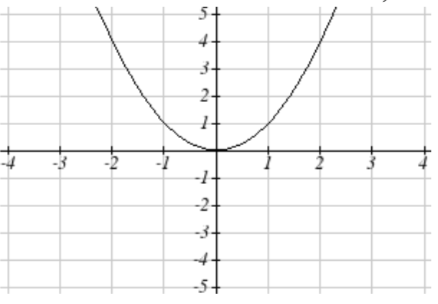 becomes
becomes 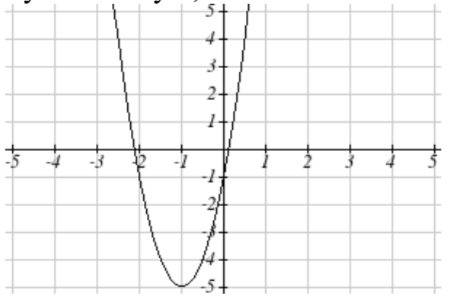
59. Horizontal shift right 4 units, vertical stretch y values by 2, reflect over x axis, vertically shift up 3 units.
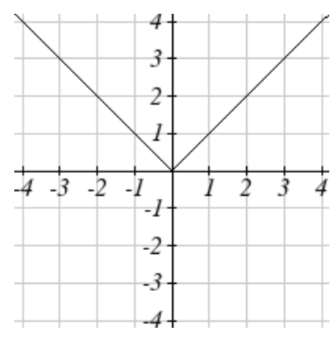 becomes
becomes 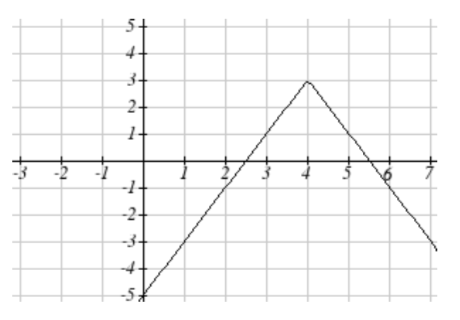
61. Vertically compress y values by 1/2
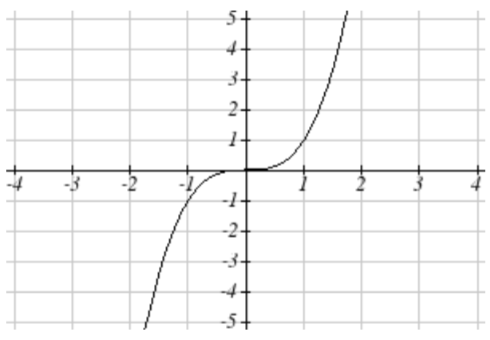 becomes
becomes 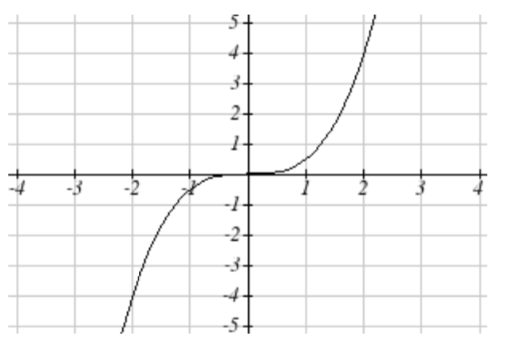
63. Horizontally stretch x values by 3, vertical shift down 3 units
 becomes
becomes 
65. Reflected over the y axis, horizontally shift right 4 units a(x)=√−(x−4)
 becomes
becomes 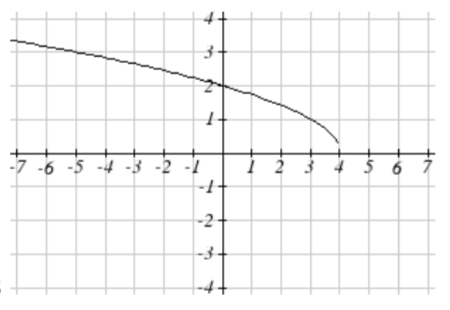
67. This function is increasing on (−1,∞) and decreasing on (−∞,−1)
69. This function is decreasing on (−∞,4)
71. This function is concave down on (−3,∞) and concave up on (−∞,−3)
73. This function is concave up everywhere
75. f(−x)
77. 3f(x)
79. 2f(−x)
81. 2f(12x)
83. 2f(x)−2
85. −f(x+1)+3
87. y=−2(x+2)2+3
89. y=(12(x−1))3+2
91. y=√2(x+2)+1
93. y=−1(x−2)2+3
95. y=−2|x+1|+3
97. y=3√−12(x−2)+1
99. f(x)={(x+3)2+1ifx≤−212|x−2|+3ifx>−2
101. f(x)={1ifx<−2−2(x+1)2+4if−2≤x≤13√x−2+1ifx>1
103a. Domain: 3.5≤x≤6
d. Range: −9≤y≤7

