4.2: The Law of Sines - The Ambiguous Case
- Page ID
- 37225
In all of the examples and problems in Section \(4.1,\) notice that we were always given two angles and one side, although we could use the Law of sines if we were given one angle and two sides (as long as one of the sides corresponded to the given angle). This is because when we use the Law of sines to find an angle, an ambiguity can arise due to the sine function being positive in Quadrant I and Quadrant II.
We saw in Chapter 3 that multiple answers arise when we use the inverse trigonometric functions. For problems in which we use the Law of sines given one angle and two sides, there may be one possible triangle, two possible triangles or no possible triangles. There are six different scenarios related to the ambiguous case of the Law of sines: three result in one triangle, one results in two triangles and two result in no triangle.
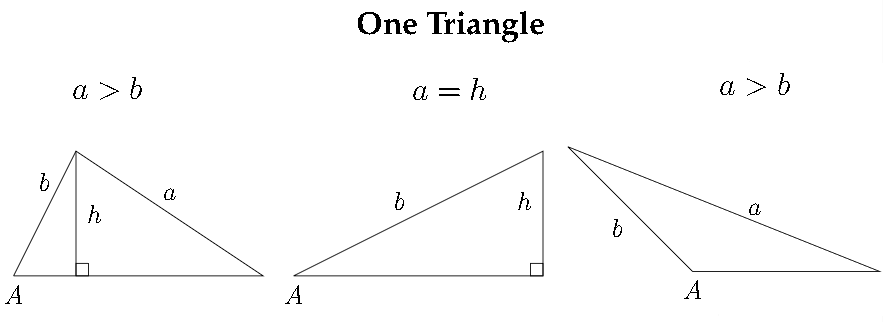
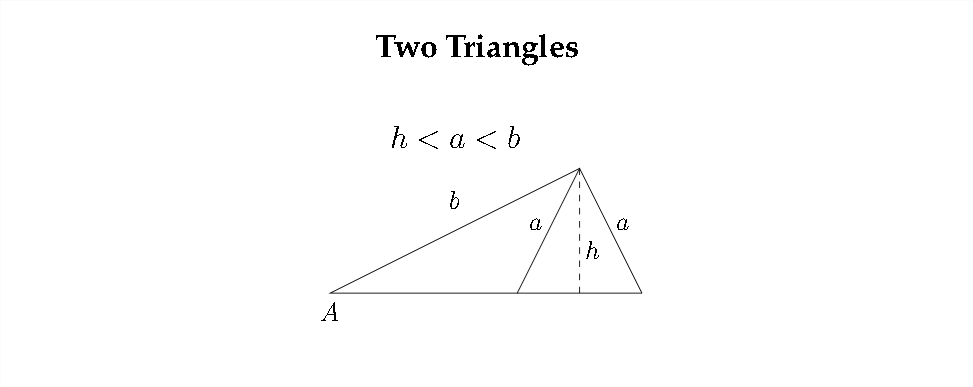
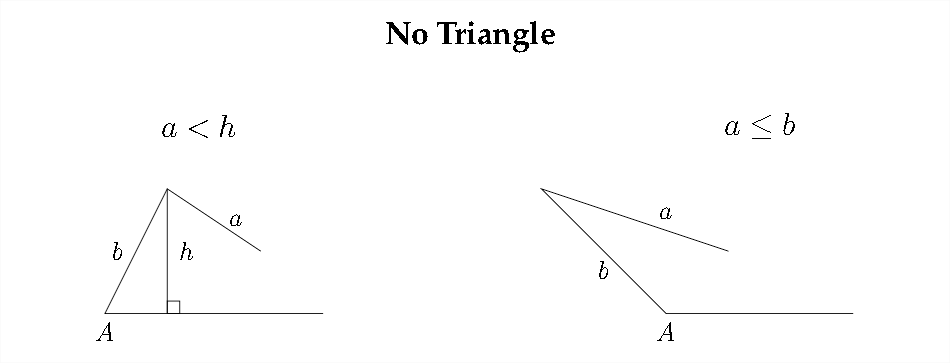
We'll look at three examples: one for one triangle, one for two triangles and one for no triangles.
Example \(\PageIndex{1}\)
Solve the triangle if: \(\angle A=112^{\circ}, \quad a=45, \quad b=24\)
Round the angles and side lengths to the nearest \(10^{t h}\)
Solution
Using the Law of sines, we can say that:
\[ \begin{array}{c}
\frac{\sin 112^{\circ}}{45}=\frac{\sin B}{24} \\
\frac{0.9272}{45} \approx \frac{\sin B}{24} \\
24 * \frac{0.9272}{45} \approx \sin B \\
0.4945 \approx \sin B
\end{array}
\]
Then, we find \(\sin ^{-1}(0.4945) \approx 29.6^{\circ} .\) Remember from Chapter 3 that there is a Quadrant II angle that has \(\sin \theta \approx 0.4945,\) with a reference angle of \(29.6^{\circ} . \mathrm{So}, \angle B\) could also be \(\approx 150.4^{\circ} .\) However, with \(\angle A=112^{\circ},\) there is no way that another angle of \(150.4^{\circ}\) would fit inside the same triangle. For this reason, we know then that \(\angle B\) must be \(29.6^{\circ}\)
\[ 29.6^{\circ} \approx B\]
So now
\[ \begin{array}{c}
\angle A=112^{\circ} \\
\angle B \approx 29.6^{\circ} \\
\operatorname{and} \angle C=180^{\circ}-\left(112^{\circ}+29.6^{\circ}\right)=180^{\circ}-141.6^{\circ} \approx 38.4^{\circ} \\
\angle C \approx 38.4^{\circ}
\end{array}\]
We already know that \(a=45\) and \(b=24 .\) To find side \(c,\) I would recommend using the most exact values possible in the Law of sines calculation. This will provide the most accurate result in finding the length of side \(c\)
\begin{array}{cc}
\frac{45}{\sin 112^{\circ}}=\frac{c}{\sin 38.4^{\circ}} \\
\frac{45}{0.9272} \approx \frac{c}{0.6211} \\
0.6211 * \frac{45}{0.9272} \approx c \\
30.1 \approx c \\
\angle A=112^{\circ} & a=45 \\
\angle B \approx 29.6^{\circ} & b=24 \\
\angle C \approx 38.4^{\circ} & c \approx 30.1
\end{array}
Example \(\PageIndex{2}\)
Solve the triangle if: \(\angle A=38^{\circ}, \quad a=40, \quad b=52\)
Round the angles and side lengths to the nearest \(10^{\text {th }}\).
Solution
Using the Law of sines, we can say that:
\[ \begin{array}{c}
\frac{\sin 38^{\circ}}{40}=\frac{\sin B}{52} \\
\frac{0.6157}{40} \approx \frac{\sin B}{52} \\
52 * \frac{0.6157}{40} \approx \sin B \\
0.8004 \approx \sin B
\end{array}
\]
Just as in the previous example, we can find \(\sin ^{-1}(0.8004) \approx 53.2^{\circ} .\) But again, there is a Quadrant II angle whose sine has the same value \(\approx 0.8004\). The angle \(126.8^{\circ}\) has a sine \(\approx 0.8004\) and a reference angle of \(53.2^{\circ} .\) With \(\angle A=38^{\circ},\) both of these angles \(\left(53.2^{\circ} \text { and } 126.8^{\circ}\right)\) could potentially fit in the triangle with angle \(A\)
If we go back to the diagrams we looked at earlier in this section, we can see how this would happen:

In the first possibility \(\angle C\) would be \(\approx 15.2^{\circ}\)
In the second possibility \(\angle C\) would be \(\approx 88.8^{\circ}\)
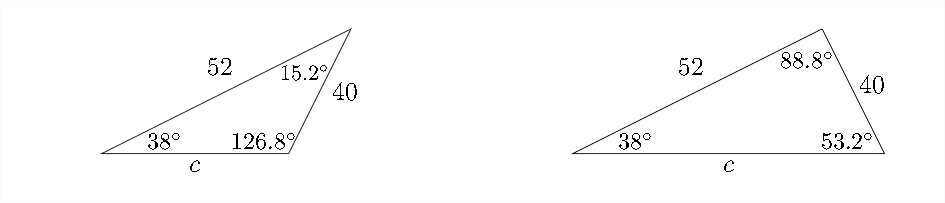
To find the two possible lengths for side \(c,\) we'll need to solve two Law of sines calculations, one with \(\angle C \approx 15.2^{\circ}\) and one with the \(\angle C \approx 88.8^{\circ}\)
\[ \begin{array}{c}
\frac{40}{\sin 38^{\circ}}=\frac{c}{\sin 15.2^{\circ}} \\
\frac{40}{0.6157} \approx \frac{c}{0.2622} \\
0.2622 * \frac{40}{0.6157} \approx c \\
17.0 \approx c
\end{array}
\]
With \(\angle C \approx 88.8^{\circ}\):
\[ \frac{40}{\sin 38^{\circ}}=\frac{c}{\sin 88.8^{\circ}} \\
\frac{40}{0.6157} \approx \frac{c}{0.9998} \\
0.9998 * \frac{40}{0.6157} \approx c \\
65.0 \approx c
\]
So, our two possible solutions would be:
\[ \begin{array}{lll}
\angle A=38^{\circ} & a=40 \\
\angle B \approx 126.8^{\circ} & b=52 \\
\angle C \approx 15.2^{\circ} & c \approx 17.0
\end{array}
\] \(\mathrm{OR}\)
\[ \angle A=38^{\circ} \quad a=40
\] \[ \begin{array}{lll}
\angle B \approx 53.2^{\circ} & b=52 \\
\angle C \approx 88.8^{\circ} & c \approx 65.0
\end{array}
\]
Example \(\PageIndex{3}\)
Solve the triangle if:
\[\angle B=73^{\circ}, \quad b=51, \quad a=92.\]
Round the angles and side lengths to the nearest \(10^{\text {th }}\).
Solution
Using the Law of sines, we can say that:
\[ \frac{\sin 73^{\circ}}{51}=\frac{\sin A}{92}\]
\[ \begin{array}{c}
\frac{0.9563}{51} \approx \frac{\sin A}{92} \\
92 * \frac{0.9563}{51} \approx \sin A \\
1.7251 \approx \sin A
\end{array}\]
As we saw previously, no real-valued angle has a sine greater than \(1 .\) Therefore, no triangle is possible.
Exercises
In each problem, solve the triangle. Round side lengths to the nearest \(100^{\text {th }}\) and angle measures to the nearest \(10^{\text {th }}\).
1. \(\quad \angle A=50^{\circ}, \quad b=20, \quad a=32\)
2. \(\quad \angle B=40^{\circ}, \quad b=4, \quad c=3\)
3. \(\quad \angle A=43^{\circ}, \quad a=23, \quad b=29\)
4. \(\quad \angle C=20^{\circ}, \quad c=43, \quad a=55\)
5. \(\quad \angle B=62^{\circ}, \quad b=4, \quad a=5\)
6. \(\quad \angle A=75^{\circ}, \quad b=8, \quad a=3\)
7. \(\quad \angle B=24^{\circ}, \quad a=17, \quad b=8\)
8. \(\quad \angle A=40^{\circ}, \quad a=4, \quad c=5\)
9. \(\quad \angle A=108^{\circ}, \quad a=12, \quad b=7\)
10. \(\quad \angle B=117^{\circ}, \quad b=19.6, \quad c=10.5\)
11. \(\quad \angle A=42^{\circ}, \quad a=18, \quad c=11\)
12. \(\quad \angle C=27^{\circ}, \quad a=42, \quad c=37\)
13. \(\quad \angle C=125^{\circ}, \quad c=2.7, \quad b=5.2\)
14. \(\quad \angle B=115^{\circ}, \quad b=68, \quad a=92\)
15. \(\quad \angle A=43^{\circ}, \quad a=31, \quad b=37\)
16. \(\quad \angle A=28^{\circ}, \quad b=3.5, \quad a=4.3\)
17. \(\quad \angle C=132^{\circ}, \quad c=22, \quad b=16\)
18. \(\quad \angle B=114.2^{\circ}, \quad b=87.2, \quad a=12.1\)
19. \(\quad \angle B=52^{\circ}, \quad c=82.7, \quad b=70\)
20. \(\quad \angle C=65^{\circ}, \quad b=7.6, \quad c=7.1\)


