5.3E: Exercises
( \newcommand{\kernel}{\mathrm{null}\,}\)
5.3: The Other Trigonometric Functions
Verbal
1) On an interval of [0,2π), can the sine and cosine values of a radian measure ever be equal? If so, where?
- Answer
-
Yes, when the reference angle is π4 and the terminal side of the angle is in quadrants I and III. Thus, at x=π4,5π4, the sine and cosine values are equal.
2) What would you estimate the cosine of π degrees to be? Explain your reasoning.
3) For any angle in quadrant II, if you knew the sine of the angle, how could you determine the cosine of the angle?
- Answer
-
Substitute the sine of the angle in for y in the Pythagorean Theorem x2+y2=1. Solve for x and take the negative solution.
4) Describe the secant function.
5) Tangent and cotangent have a period of π. What does this tell us about the output of these functions?
- Answer
-
The outputs of tangent and cotangent will repeat every π units.
Algebraic
For the exercises 6-17, find the exact value of each expression.
6) tanπ6
7) secπ6
- Answer
-
2√33
8) cscπ6
9) cotπ6
- Answer
-
√3
10) tanπ4
11) secπ4
- Answer
-
√2
12) cscπ4
13) cotπ4
- Answer
-
1
14) tanπ3
15) secπ3
- Answer
-
2
16) cscπ3
17) cotπ3
- Answer
-
√33
For the exercises 18-48, use reference angles to evaluate the expression.
18) tan5π6
19) sec7π6
- Answer
-
−2√33
20) csc11π6
21) cot13π6
- Answer
-
√3
22) tan7π4
23) sec3π4
- Answer
-
−√2
24) csc5π4
25) cot11π4
- Answer
-
−1
26) tan8π3
27) sec4π3
- Answer
-
−2
28) csc2π3
29) cot5π3
- Answer
-
−√33
30) \tan 225°
31) \sec 300°
- Answer
-
2
32) \csc 150°
33) \cot 240°
- Answer
-
\dfrac{\sqrt{3}}{3}
34) \tan 330°
35) \sec 120°
- Answer
-
−2
36) \csc 210°
37) \cot 315°
- Answer
-
−1
38) If \sin t= \dfrac{3}{4}, and t is in quadrant II, find \cos t, \sec t, \csc t, \tan t, \cot t .
39) If \cos t=−\dfrac{1}{3}, and t is in quadrant III, find \sin t, \sec t, \csc t, \tan t, \cot t.
- Answer
-
If \sin t=−\dfrac{2\sqrt{2}}{3}, \sec t=−3, \csc t=−\csc t=−\dfrac{3\sqrt{2}}{4},\tan t=2\sqrt{2}, \cot t= \dfrac{\sqrt{2}}{4}
40) If \tan t=\dfrac{12}{5}, and 0≤t< \dfrac{π}{2}, find \sin t, \cos t, \sec t, \csc t, and \cot t.
41) If \sin t= \dfrac{\sqrt{3}}{2} and \cos t=\dfrac{1}{2}, find \sec t, \csc t, \tan t, and \cot t.
- Answer
-
\sec t=2, \csc t=\csc t=\dfrac{2\sqrt{3}}{3}, \tan t= \sqrt{3}, \cot t= \dfrac{\sqrt{3}}{3}
42) If \sin 40°≈0.643 \; \cos 40°≈0.766 \; \sec 40°,\csc 40°,\tan 40°, \text{ and } \cot 40°.
43) If \sin t= \dfrac{\sqrt{2}}{2}, what is the \sin (−t)?
- Answer
-
−\dfrac{\sqrt{2}}{2}
44) If \cos t= \dfrac{1}{2}, what is the \cos (−t)?
45) If \sec t=3.1, what is the \sec (−t)?
- Answer
-
3.1
46) If \csc t=0.34, what is the \csc (−t)?
47) If \tan t=−1.4, what is the \tan (−t)?
- Answer
-
1.4
48) If \cot t=9.23, what is the \cot (−t)?
Graphical
For the exercises 49-51, use the angle in the unit circle to find the value of the each of the six trigonometric functions.
49)

- Answer
-
\sin t= \dfrac{\sqrt{2}}{2}, \cos t= \dfrac{\sqrt{2}}{2}, \tan t=1,\cot t=1,\sec t= \sqrt{2}, \csc t= \csc t= \sqrt{2}
50)
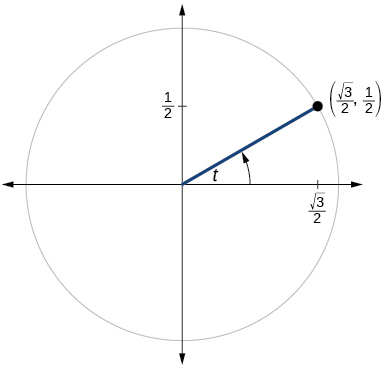
51)

- Answer
-
\sin t=−\dfrac{\sqrt{3}}{2}, \cos t=−\dfrac{1}{2}, \tan t=\sqrt{3}, \cot t= \dfrac{\sqrt{3}}{3}, \sec t=−2, \csc t=−\csc t=−\dfrac{2\sqrt{3}}{3}
Technology
For the exercises 52-61, use a graphing calculator to evaluate.
52) \csc \dfrac{5π}{9}
53) \cot \dfrac{4π}{7}
- Answer
-
–0.228
54) \sec \dfrac{π}{10}
55) \tan \dfrac{5π}{8}
- Answer
-
–2.414
56) \sec \dfrac{3π}{4}
57) \csc \dfrac{π}{4}
- Answer
-
1.414
58) \tan 98°
59) \cot 33°
- Answer
-
1.540
60) \cot 140°
61) \sec 310°
- Answer
-
1.556
Extensions
For the exercises 62-69, use identities to evaluate the expression.
62) If \tan (t)≈2.7, and \sin (t)≈0.94, find \cos (t).
63) If \tan (t)≈1.3, and \cos (t)≈0.61, find \sin (t).
- Answer
-
\sin (t)≈0.79
64) If \csc (t)≈3.2, and \csc (t)≈3.2, and \cos (t)≈0.95, find \tan (t).
65) If \cot (t)≈0.58, and \cos (t)≈0.5, find \csc (t).
- Answer
-
\csc (t)≈1.16
66) Determine whether the function f(x)=2 \sin x \cos x is even, odd, or neither.
67) Determine whether the function f(x)=3 \sin ^2 x \cos x + \sec x is even, odd, or neither.
- Answer
-
even
68) Determine whether the function f(x)= \sin x −2 \cos ^2 x is even, odd, or neither.
69) Determine whether the function f(x)= \csc ^2 x+ \sec x is even, odd, or neither.
- Answer
-
even
For the exercises 70-71, use identities to simplify the expression.
70) \csc t \tan t
71) \dfrac{\sec t}{ \csc t}
- Answer
-
\dfrac{ \sin t}{ \cos t}= \tan t
Real-World Applications
72) The amount of sunlight in a certain city can be modeled by the function h=15 \cos \left(\dfrac{1}{600}d\right), where h represents the hours of sunlight, and d is the day of the year. Use the equation to find how many hours of sunlight there are on February 10, the 42^{nd} day of the year. State the period of the function.
73) The amount of sunlight in a certain city can be modeled by the function h=16 \cos \left(\dfrac{1}{500}d\right), where h represents the hours of sunlight, and d is the day of the year. Use the equation to find how many hours of sunlight there are on September 24, the 267^{th} day of the year. State the period of the function.
- Answer
-
13.77 hours, period: 1000π
74) The equation P=20 \sin (2πt)+100 models the blood pressure, P, where t represents time in seconds.
- Find the blood pressure after 15 seconds.
- What are the maximum and minimum blood pressures?
75) The height of a piston, h, in inches, can be modeled by the equation y=2 \cos x+6, where x represents the crank angle. Find the height of the piston when the crank angle is 55°.
- Answer
-
7.73 inches
76) The height of a piston, h,in inches, can be modeled by the equation y=2 \cos x+5, where x represents the crank angle. Find the height of the piston when the crank angle is 55°.

