2.4: The Pythagorean Theorem

Learning Objectives

• Use the Pythagorean Theorem to find the unknown side of a right triangle.
• Solve application problems involving the Pythagorean Theorem.

Introduction

A long time ago, a Greek mathematician named Pythagoras discovered an interesting property about right triangles: the sum of the squares of the lengths of each of the triangle’s legs is the same as the square of the length of the triangle’s hypotenuse. This property, which has many applications in science, art, engineering, and architecture, is now called the Pythagorean Theorem.

Let’s take a look at how this theorem can help you learn more about the construction of triangles. And the best part is you don’t even have to speak Greek to apply Pythagoras’ discovery.

The Pythagorean Theorem

Pythagoras studied right triangles, and the relationships between the legs and the hypotenuse of a right triangle, before deriving his theory. The legs of a right triangle are the sides attached to the right angle and the hypotenuse is the side opposite the right angle. By convention the legs are refrred to with the letters a and b, and the hypotenuse is labeled c.

The Pythagorean Theorem

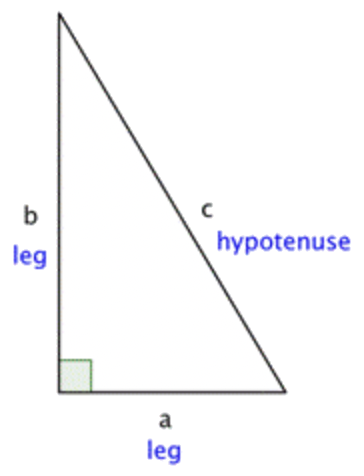
If \(\ a\) and \(\ b\) are the lengths of the legs of a right triangle and \(\ c\) is the length of the hypotenuse, then the sum of the squares of the lengths of the legs is equal to the square of the length of the hypotenuse.

This relationship is represented by the formula:

\[\ a^{2}+b^{2}=c^{2}\]

In the box above, you may have noticed the word “square,” as well as the small 2s to the top right of the letters in \(\ a^{2}+b^{2}=c^{2}\). To square a number means to multiply it by itself. So, for example, to square the number 5 you multiply \(\ 5 \cdot 5\), and to square the number 12, you multiply \(\ 12 \cdot 12\). Some common squares are shown in the table below.

Number	Number Times Itself	Square
1	\(\ 1^{2}=1 \cdot 1\)	1
2	\(\ 2^{2}=2 \cdot 2\)	4
3	\(\ 3^{2}=3 \cdot 3\)	9
4	\(\ 4^{2}=4 \cdot 4\)	16
5	\(\ 5^{2}=5 \cdot 5\)	25
10	\(\ 10^{2}=10 \cdot 10\)	100

When you see the equation \(\ a^{2}+b^{2}=c^{2}\), you can think of this as “the length of side \(\ a\) times itself, plus the length of side \(\ b\) times itself is the same as the length of side \(\ c\) times itself.”

Let’s try out the Pythagorean Theorem with an actual right triangle.

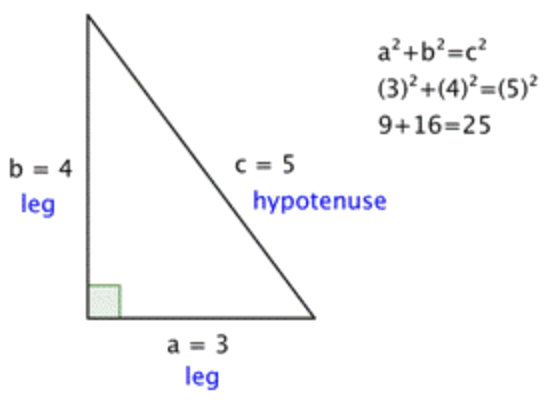
This theorem holds true for this right triangle: the sum of the squares of the lengths of both legs is the same as the square of the length of the hypotenuse. And, in fact, it holds true for all right triangles.

The Pythagorean Theorem can also be represented in terms of area. In any right triangle, the area of the square drawn from the hypotenuse is equal to the sum of the areas of the squares that are drawn from the two legs. You can see this illustrated below in the same 3-4-5 right triangle.

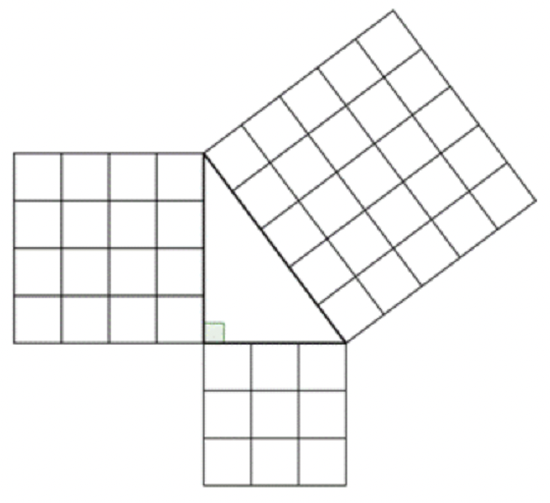
Note that the Pythagorean Theorem only works with right triangles.

Finding the Length of the Hypotenuse

You can use the Pythagorean Theorem to find the length of the hypotenuse of a right triangle if you know the length of the triangle’s other two sides, called the legs. Put another way, if you know the lengths of \(\ a\) and \(\ b\), you can find \(\ c\).

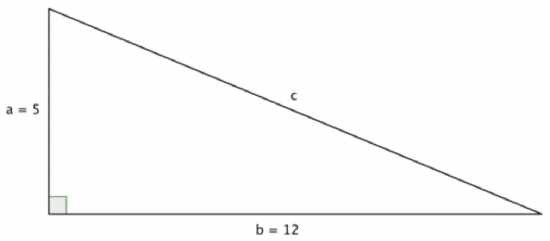
In the triangle above, you are given measures for legs \(\ a\) and \(\ b\): 5 and 12, respectively. You can use the Pythagorean Theorem to find a value for the length of \(\ c\), the hypotenuse.

\(\ a^{2}+b^{2}=c^{2}\)	The Pythagorean Theorem
\(\ (5)^{2}+(12)^{2}=c^{2}\)	Substitute known values for \(\ a\) and \(\ b\).
\(\ 25+144=c^{2}\)	Evaluate.
\(\ 169=c^{2}\)	Simplify. To find the value of \(\ c\), think about a number that, when multiplied by itself, equals 169. Does 10 work? How about 11? Does 12? 13? (You can use a calculator to multiply if the numbers are unfamiliar.)
\(\ 13=c\)	The square root of 169 is 13.

Using the formula, you find that the length of \(\ c\), the hypotenuse, is 13.

In this case, you did not know the value of \(\ c\), you were given the square of the length of the hypotenuse, and had to figure it out from there. When you are given an equation like \(\ 169=c^{2}\) and are asked to find the value of \(\ c\), this is called finding the square root of a number. (Notice you found a number, c, whose square was 169.)

Finding a square root takes some practice, but it also takes knowledge of multiplication, division, and a little bit of trial and error. Look at the table below.

Number \(\ x\)	Number \(\ y\) which, when multiplied by itself, equals number \(\ x\)	Square root \(\ y\)
1	\(\ 1 \cdot 1\)	1
4	\(\ 2 \cdot 2\)	2
9	\(\ 3 \cdot 3\)	3
16	\(\ 4 \cdot 4\)	4
25	\(\ 5 \cdot 5\)	5
100	\(\ 10 \cdot 10\)	10

It is a good habit to become familiar with the squares of the numbers from 0 through 10, as these arise frequently in mathematics. If you can remember those square numbers, or if you can use a calculator to find them, then finding many common square roots will be just a matter of recall.

Try It \(\PageIndex{1}\)

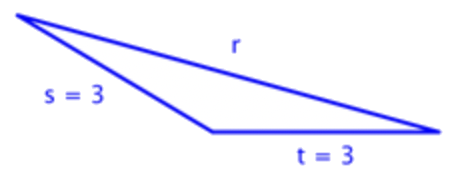
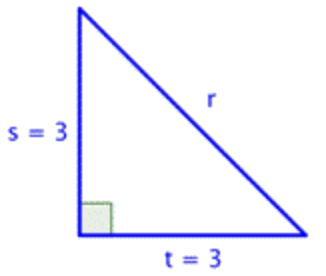
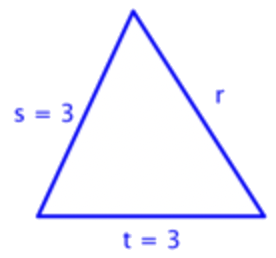
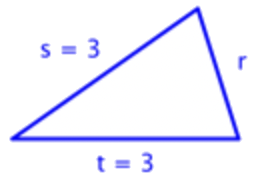
For which of these triangles is \(\ (3)^{2}+(3)^{2}=r^{2}\)?

1. 
2. 
3. 
4. 

Answer

B, since it is the only right triangle.

Finding the Length of a Leg

You can use the same formula to find the length of a right triangle’s leg if you are given measurements for the lengths of the hypotenuse and the other leg. Consider the example below.

Example \(\PageIndex{1}\)

Find the length of side a in the triangle below. Use a calculator to estimate the square root to one decimal place.

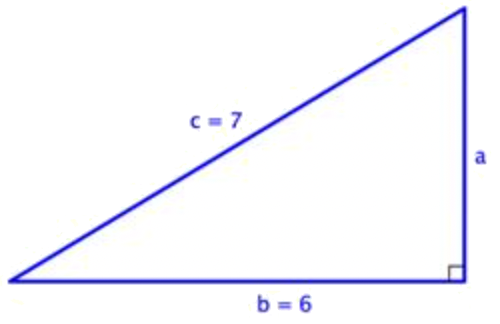
Solution

\(\ \begin{array}{l} a=? \\ b=6 \\ c=7 \end{array}\)	In this right triangle, you are given the measurements for the hypotenuse, \(\ c\), and one leg, \(\ b\). The hypotenuse is always opposite the right angle and it is always the longest side of the triangle.
\(\ \begin{array}{l} a^{2}+b^{2}=c^{2} \\ a^{2}+6^{2}=7^{2} \end{array}\)	To find the length of leg \(\ a\), substitute the known values into the Pythagorean Theorem.
\(\ a^{2}+36 =49 \)	Solve for \(\ a^{2}\) by subtracting 36 from both side of the equation
\(\ a^{2} =13 \)	Take a square root on both sides
\(\ a =\sqrt{13} \approx 3.6\)	Use a calculator to find the square root of 13. The calculator gives an answer of 3.6055..., which you can round to 3.6. (Since you are approximating, you use the symbol \(\ \approx\).)

Thus, \(\ a \approx 3.6\).

Try It \(\PageIndex{2}\)

Which of the following correctly uses the Pythagorean Theorem to find the missing side, \(\ x\)?

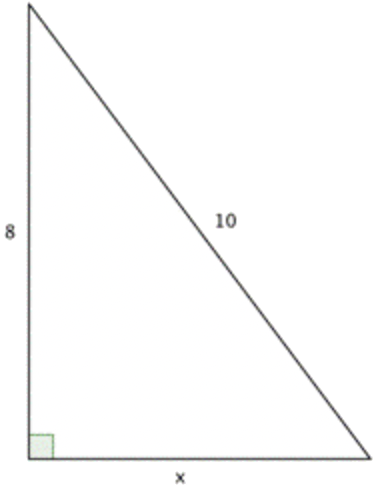
1. \(\ 8^{2}+10^{2}=x^{2}\)
2. \(\ x+8=10\)
3. \(\ x^{2}+8^{2}=10^{2}\)
4. \(\ x^{2}+10^{2}=8^{2}\)

Answer

1. Incorrect. In this triangle, you know the hypotenuse (the side opposite the right angle) has a length of 10. The lengths of the legs are 8 and \(\ x\).
2. Incorrect. The Pythagorean Theorem is a relationship between the lengths of the sides squared.
3. Correct. In this triangle, the hypotenuse has length 10, and the legs have length 8 and \(\ x\). Substituting into the Pythagorean Theorem you have: \(\ x^{2}+8^{2}=10^{2}\); this equation is the same as \(\ x^{2}+64=100\), or \(\ x^{2}=36\). What number, times itself, equals 36? That would make \(\ x=6\).
4. Incorrect. In this triangle, the hypotenuse has length 10 (always the longest side of the triangle and the side opposite the right angle) not 8.

Using the Theorem to Solve Real World Problems

The Pythagorean Theorem is perhaps one of the most useful formulas you will learn in mathematics because there are so many applications of it in real-world settings. Architects and engineers use this formula extensively when building ramps, bridges, and buildings. Look at the following examples.

Example \(\PageIndex{2}\)

The owners of a house want to convert a stairway leading from the ground to their back porch into a ramp. The porch is 3 feet off the ground and, due to building regulations, the ramp must start 12 feet away from the base of the porch. How long will the ramp be?

Use a calculator to find the square root, and round the answer to the nearest tenth.

Solution

To solve a problem like this one, it often makes sense to draw a simple diagram showing where the legs and hypotenuse of the triangle lie.

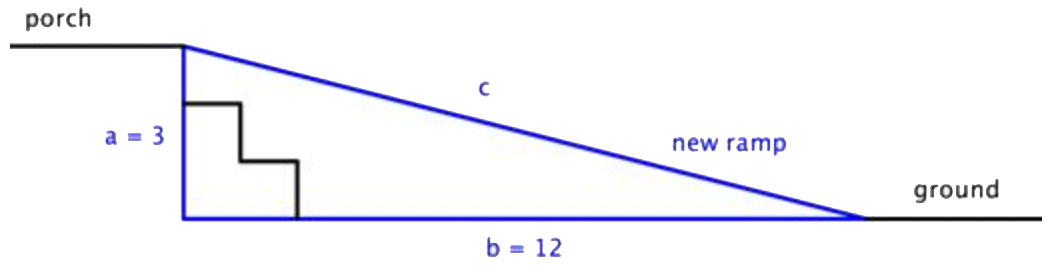
\(\ \begin{array}{r} a=3 \\ b=12 \\ c=? \end{array}\)	Identify the legs and the hypotenuse of the triangle. You know that the triangle is a right triangle since the ground and the raised portion of the porch are perpendicular. This means you can use the Pythagorean Theorem to solve this problem. Identify \(\ a\), \(\ b\), and \(\ c\).
\(\ \begin{aligned} a^{2}+b^{2} &=c^{2} \\ 3^{2}+12^{2} &=c^{2} \\ 9+144 &=c^{2} \\ 153 &=c^{2} \end{aligned}\)	Use the Pythagorean Theorem to find the length of \(\ c\).
\(\ c= \sqrt{153} \approx 12.4\)	Use your calculator to find \(\ c\). The square root of 153 is 12.369..., so you can round that to 12.4.

The ramp will be 12.4 feet long.

Example \(\PageIndex{3}\)

A sailboat has a large sail in the shape of a right triangle. The longest edge of the sail measures 17 yards and the bottom edge of the sail is 8 yards. How tall is the sail?

Solution

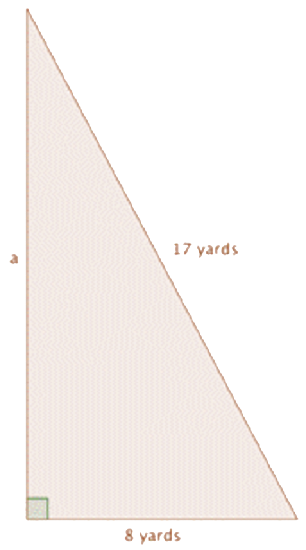	Draw an image to help you visualize the problem. In a right triangle, the hypotenuse will always be the longest side, so here it must be 17 yards. The problem also tells you that the bottom edge of the triangle is 8 yards.
\(\ \begin{array}{r} a^{2}+b^{2}=c^{2} \\ a^{2}+8^{2}=17^{2} \\ a^{2}+64=289 \\ a^{2}=225 \end{array}\)	Set up the Pythagorean Theorem.
\(\ a=15\)	\(\ 15 \cdot 15=225, \text { so } a=15\)

The height of the sail is 15 yards.

Summary

The Pythagorean Theorem states that in any right triangle, the sum of the squares of the lengths of the triangle’s legs is the same as the square of the length of the triangle’s hypotenuse. This theorem is represented by the formula \(\ a^{2}+b^{2}=c^{2}\). Put simply, if you know the lengths of two sides of a right triangle, you can apply the Pythagorean Theorem to find the length of the third side. Remember, this theorem only works for right triangles.