Skip to main content
Mathematics LibreTexts

10.1.4.1: Properties and Laws of Whole Numbers

  1. Last updated
  2. Save as PDF
  • Page ID
    113214

    • \( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

    Learning Objectives
    • Simplify by using the addition property of 0.
    • Simplify by using the multiplication property of 1.
    • Identify and use the commutative law of addition.
    • Identify and use the commutative law of multiplication.
    • Identify and use the associative law of addition.
    • Identify and use the associative law of multiplication.

    Introduction

    Mathematics often involves simplifying numerical expressions. When doing so, you can use laws and properties that apply to particular operations. The multiplication property of 1 states that any number multiplied by 1 equals the same number, and the addition property of zero states that any number added to zero is the same number.

    Two important laws are the commutative laws, which state that the order in which you add two numbers or multiply two numbers does not affect the answer. You can remember this because if you commute to work you go the same distance driving to work and driving home as you do driving home and driving to work. You can move numbers around in addition and multiplication expressions because the order in these expressions does not matter.

    You will also learn how to simplify addition and multiplication expressions using the associative laws. As with the commutative laws, there are associative laws for addition and multiplication. Just like people may associate with people in different groups, a number may associate with other numbers in one group or another. The associative laws allow you to place numbers in different groups using parentheses.

    Addition and Multiplication Properties of 0 and 1

    The addition property of 0 states that for any number being added to 0, the sum equals that number. Remember that you do not end up with zero as an answer; that only happens when you multiply. Your answer is simply the same as your original number.

    Example

    \(\ 62+0=?\)

    Solution

    \(\ 62+0=62\) Adding zero to 62 does not add any quantity to the sum, so the number remains 62.

    \(\ 62+0=62\)

    Exercise

    \(\ 112+0=?\)

    1. 112
    2. 0
    3. 1
    4. 1,120
    Answer
    1. Correct. Adding zero to a number does not change a number.
    2. Incorrect. Your answer would be zero if you multiply 112 by 0, but not if you add 112 to 0. The correct answer is 112.
    3. Incorrect. Adding 112 to 0 does not equal 1. The correct answer is 112.
    4. Incorrect. You do not put zero in the ones place and move other digits up by one place value. This only occurs if you are multiplying a number by ten. The correct answer is 112.

    According to the multiplication property of 1, the product of 1 and any number results in that number. The answer is simply identical to the original number.

    Example

    \(\ 2,500 \cdot 1=?\)

    Solution

    \(\ 2,500 \cdot 1=2,500\) Multiplying 2,500 by 1 yields the same number.

    \(\ 2,500 \cdot 1=2,500\)

    Exercise

    \(\ 72,540 \cdot 1=?\)

    1. 725,401
    2. 72,541
    3. 72,540
    4. 72,539
    Answer
    1. Incorrect. You do not put 1 in the ones place and move other digits up by one place value. The correct answer is 72,540.
    2. Incorrect. You do not add one to the number. The correct answer is 72,540.
    3. Correct. Multiplying any number by 1 yields the same number, which is in this case 72,540.
    4. Incorrect. You do not subtract one from the number. The correct answer is 72,540.

    The Commutative Law of Addition

    The commutative law of addition states that you can change the position of numbers in an addition expression without changing the sum. For example, 3+2 is the same as 2+3.

    3+2=5

    2+3=5

    You likely encounter daily routines in which the order can be switched. For example, when you get ready for work in the morning, putting on your left glove and right glove is commutative. You could put the right glove on before the left glove, or the left glove on before the right glove. Likewise, brushing your teeth and combing your hair is commutative, because it does not matter which one you do first.

    Remember that this law only applies to addition, and not subtraction. For example:

    8-2 is not the same as 2-8.

    Below, you will find examples of expressions that have been changed with the commutative law. Note that expressions involving subtraction cannot be changed.

    Original Expression Rewritten Expression
    4+5 5+4
    6+728 728+6
    9+4+1 9+1+4
    9-1 cannot be changed
    72-10 cannot be changed
    128-100 cannot be changed

    You also will likely encounter real life routines that are not commutative. When preparing to go to work, putting on our clothes has to occur before putting on a coat. Likewise, getting in the car has to occur before putting the key in the ignition. In a store, you would need to pick up the items you are buying before proceeding to the cash register for checkout.

    Example

    Write the expression 10+25 in a different way, using the commutative law of addition, and show that both expressions result in the same answer.

    Solution

    10+25=35 Solving the problem yields an answer of 35.
    25+10 Using the commutative property, you can switch the 10 and the 25 so that they are in different positions.
    25+10=35 Adding 25 to 10 in this new order also yields 35.

    10+25=35 and 25+10=35

    Exercise

    Rewrite 15+12=27 in a different way, using the commutative law of addition.

    1. 15=12+27
    2. 12=15+27
    3. 15+(12=27)
    4. 12+15=27
    Answer
    1. Incorrect. Only the order of the numbers to be added can change. The correct answer is 12+15=27.
    2. Incorrect. Only the order of the numbers to be added can change. The correct answer is 12+15=27.
    3. Incorrect. You would never put parentheses around an = sign (and parentheses are not used with the commutative laws). The correct answer is 12+15=27.
    4. Correct. The commutative law lets you change the order of the numbers being added.

    The Commutative Law of Multiplication

    Multiplication also has a commutative law. The commutative law of multiplication states that when two or more numbers are being multiplied, their order can be changed without affecting the answer. In the example below, note that 5 multiplied by 4 yields the same result as 4 multiplied by 5. In both cases, the answer is 20.

    \(\ 5 \cdot 4=20\)

    \(\ 4 \cdot 5=20\)

    This example shows how numbers can be switched in a multiplication expression.

    Example

    Write the expression \(\ 30 \cdot 50\) in a different way, using the commutative law of multiplication, and show that both expressions result in the same answer.

    Solution

    \(\ 30 \cdot 50=1,500\) Solving the problem yields an answer of 1,500.
    \(\ 50 \cdot 30\) Using the commutative law, you can switch the 30 and the 50 so that they are in different positions.
    \(\ 50 \cdot 30=1,500\) Multiplying 50 and 30 also yields 1,500.

    \(\ 50 \cdot 30\) and \(\ 30 \cdot 50=1,500\)

    Keep in mind that when you are using the commutative law, only the order is affected. The grouping remains unchanged.

    Exercise

    Rewrite \(\ 52 \cdot 46\) in a different way, using the commutative law of multiplication.

    1. \(\ 42 \cdot 56\)
    2. \(\ 5 \cdot 246\)
    3. \(\ 5 \cdot 24 \cdot 6\)
    4. \(\ 46 \cdot 52\)
    Answer
    1. Incorrect. You cannot switch one digit from each number. The correct answer is \(\ 46 \cdot 52\).
    2. Incorrect. The same two numbers should be multiplied. The numbers themselves should not be changed, only the order in which the numbers appear. The correct answer is \(\ 46 \cdot 52\).
    3. Incorrect. The same two numbers should be multiplied. The numbers themselves should not be changed, only the order in which the numbers appear. The correct answer is \(\ 46 \cdot 52\).
    4. Correct. The order of numbers is reversed, and the same two numbers are multiplied.

    The Associative Law of Addition

    Below are two ways of simplifying and solving an addition problem. Note that you can add numbers in any order. In the first example, 4 is added to 5 to make 9.

    4+5+6=9+6=15

    Here, the same problem is solved, but this time, 5 is added to 6 to make 11. Note that solving it this way yields the same answer.

    4+5+6=4+11=15

    The associative law of addition states that numbers in an addition expression can be regrouped using parentheses. You can remember the meaning of the associative law by remembering that when you associate with family members, friends, and co-workers, you end up forming groups with them. In the following expression, parentheses are used to group numbers together so that you know what to add first. Note that when parentheses are present, any numbers within parentheses are numbers you will add first. The expression can be re-written with different groups using the associative law.

    (4+5)+6=9+6=15

    4+(5+6)=4+11=15

    Here, it is clear that the parentheses do not affect the final answer. The answer is the same regardless of where the parentheses are.

    Example

    Rewrite (5+8)+3 using the associative law of addition. Show that the rewritten expression yields the same answer.

    Solution

    (5+8)+3=13+3=16 The original expression yields an answer of 16.
    5+(8+3)=5+11=16 Grouping 8 and 3 instead of 5 and 8 results in the same answer of 16.

    (5+8)+3=16 and 5+(8+3)=16

    When rewriting an expression using the associative law, remember that you are regrouping the numbers and not reversing the order, as in the commutative law.

    Exercise

    Rewrite 10+(5+6) using the associative property.

    1. (5+6)+10
    2. 10+(6+5)
    3. (10+5)+6
    4. (10+6)+5
    Answer
    1. Incorrect. The order of numbers is not changed when you are rewriting the expression using the associative law of addition. How the numbers are grouped should change. The correct answer is (10+5)+6.
    2. Incorrect. The order of numbers is not changed when you are rewriting the expression using the associative law of addition. How the numbers are grouped should change. The correct answer is (10+5)+6.
    3. Correct. Here, the numbers are regrouped. Now 10 and 5 are grouped in parentheses instead of 5 and 6.
    4. Incorrect. The order of numbers is not changed when you are rewriting the expression using the associative law of addition. Only how the numbers are grouped should change. The correct answer is (10+5)+6.

    The Associative Law of Multiplication

    Multiplication has an associative law that works exactly the same as the one for addition. The associative law of multiplication states that numbers in a multiplication expression can be regrouped using parentheses. The following expression can be rewritten in a different way using the associative law.

    \(\ (2 \cdot 3) \cdot 4=2 \cdot(3 \cdot 4)\)

    Here, it is clear that the parentheses do not affect the final answer. The answer is the same regardless of where the parentheses are.

    Example

    Rewrite \(\ (10 \cdot 200) \cdot 24\) using the associative law of multiplication, and show that the rewritten expression yields the same answer.

    Solution

    \(\ (10 \cdot 200) \cdot 24=2000 \cdot 24=48,000\) The original expression yields an answer of 48,000.
    \(\ 10 \cdot(200 \cdot 24)=10 \cdot 4800=48,000\) Grouping 200 and 24 instead of 10 and 200 results in the same answer of 48,000.

    \(\ (10 \cdot 200) \cdot 24=48,000\) and \(\ 10 \cdot(200 \cdot 24)=48,000\)

    When rewriting an expression using the associative law, remember that you are regrouping the numbers and not changing the order. Changing the order uses the commutative law.

    Exercise

    Rewrite \(\ 8 \cdot(7 \cdot 6)\) using the associative property.

    1. \(\ (8 \cdot 7) \cdot 6\)
    2. \(\ (7 \cdot 6) \cdot 8\)
    3. \(\ (7 \cdot 8) \cdot 6\)
    4. \(\ (8 \cdot 76)\)
    Answer
    1. Correct. Here, the numbers are regrouped. Now 8 and 7 are grouped in parentheses instead of 7 and 6.
    2. Incorrect. The order of numbers is not changed when you are rewriting the expression using the associative law of multiplication. How they are grouped should change. The correct answer is \(\ (8 \cdot 7) \cdot 6\).
    3. Incorrect. The order of numbers is not changed when you are rewriting the expression using the associative law of multiplication. Only how they are grouped should change. The correct answer is \(\ (8 \cdot 7) \cdot 6\).
    4. Incorrect. The digits of different numbers are not combined to create new numbers. How the numbers are grouped should change. The correct answer is \(\ (8 \cdot 7) \cdot 6\).

    Commutative or Associative?

    When an expression is being rewritten, you can tell whether it is being rewritten using the commutative or associative laws based on whether the order of the numbers change or the numbers are being regrouped using parentheses.

    If an expression is rewritten so that the order of the numbers is changed, the commutative law is being used.

    Example

    \(\ 10 \cdot 2=20\) is rewritten as 2 \(\ \cdot 10=20\). Was this expression rewritten using the commutative law or the associative law?

    Solution

    Screen Shot 2021-04-15 at 2.39.45 PM.png Rewriting the expression involves switching the order of the numbers. Therefore, the commutative law is being used.

    The commutative law is being used to rewrite the expression.

    Remember that when you associate with friends and family, typically you are grouping yourself with other people. So, if numbers in an expression are regrouped using parentheses and the order of numbers remains the same, then the associative law is being used.

    Example

    \(\ 2 \cdot(4 \cdot 6)=48\) is rewritten as \(\ (2 \cdot 4) \cdot 6=48\). Was this expression rewritten using the commutative law or the associative law?

    Solution

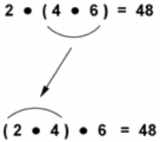    Screen Shot 2021-04-15 at 2.42.58 PM.png Regrouping using parentheses does not change the order of the numbers. Therefore, the associative law is being used.

    The associative law is being used to rewrite the expression.

    Exercise

    \(\ 12 \cdot(6 \cdot 2)=144\) is rewritten as \(\ 3 \cdot 17=51\). Was this expression rewritten using the commutative law or associative law?

    1. commutative law
    2. associative law
    Answer
    1. Correct. The order of numbers is being switched, which shows that the commutative law is being used.
    2. Incorrect. The associative law involves regrouping numbers using parentheses, which does not occur in this problem. Rather, the order of numbers is switched. The correct answer is commutative law.

    Using the Associative and Commutative Laws

    The associative and commutative laws are useful when you have an expression with only addition. Using the commutative law, the numbers can be reordered so that the numbers that are easiest to add are next to each other, and using the associative law, you can group them in any way.

    For example, here are some of the ways we can add 6+5+4 using the associative and commutative laws. Note that the answer is always the same.

    (6+5)+4=11+4=15 (grouping 6 and 5 to add first)

    (5+6)+4=11+4=15 (reordering 6 and 5)

    5+(6+4)=5+10=15 (grouping 6 and 4 to add first)

    6+(5+4)=6+9=15 (grouping 5 and 4 to add first)

    6+(4+5)=6+9=15 (reordering 4 and 5)

    (6+4)+5=10+5=15 (grouping 6 and 4 to add first)

    Example

    Write the expression 13+28+7 a different way to make it easier to simplify. Then simplify.

    Solution

    13+28+7

    13+7+28

    		 Using the commutative property, reorder the numbers 7 and 28 since 13+7 is easier to add than 13+28.
    20+28 Using the associative property, group the 13 and 7 together and add them first.
    48 Add 20 and 28.

    13+28+7=13+7+28=48

    Sometimes the commutative and associative laws can make the problem easy enough to do in your head.

    Example

    Jim is buying 8 pears, 7 apples, and 2 oranges. He decided the total number of fruits is 8+7+2. Use the commutative property to write this expression in a different way. Then find the total.

    Solution

    8+7+2

    8+2+7

    		 Using the commutative property, reorder 2 and 7.
    10+7 Using the associative property, group the 8 and 2 together and add them first.
    17 Add 10 and 7.

    8+7+2=8+2+7=17

    This also works when you are multiplying more than two numbers. You can use the commutative and associative laws freely if the expression involves only multiplication.

    Example

    There are 2 trucks in a garage, and each truck holds 60 boxes. There are 5 laptop computers in each box. Find the number of computers in the garage.

    Solution

    \(\ 2 \cdot 60 \cdot 5\) In order to find the answer, you need to multiply the number of trucks times the number of boxes in each truck, and, then by the number of computers in each box.
    \(\ 2 \cdot 5 \cdot 60\) Using the commutative property, reorder the 5 and the 60. Now you can multiply \(\ 2 \cdot 5\) first.
    \(\ 10 \cdot 60\) Using the associative property, multiply the 2 and the 5, 2 \cdot 5=10.
    600 Now it's easier to multiply 10 and 60 to get 600.

    There are 600 computers in the garage.

    Summary

    The addition property of 0 states that for any number being added to zero, the sum is the same number. The multiplication property of 1 states that for any number multiplied by one, that answer is that same number. Zero is called the additive identity, and one is called the multiplicative identity.

    When you rewrite an expression by a commutative law, you change the order of the numbers being added or multiplied. When you rewrite an expression using an associative law, you group a different pair of numbers together using parentheses.

    You can use the commutative and associative laws to regroup and reorder any number in an expression that involves only addition. You can also use the commutative and associative laws to regroup and reorder any number in an expression that involves only multiplication.

    This page titled 10.1.4.1: Properties and Laws of Whole Numbers is shared under a CC BY-SA 4.0 license and was authored, remixed, and/or curated by The NROC Project via source content that was edited to the style and standards of the LibreTexts platform; a detailed edit history is available upon request.