10.1.5.1: Understanding Exponents and Square Roots

Learning Objectives

• Evaluate expressions containing exponents.
• Write repeated factors using exponential notation.
• Find a square root of a perfect square.

Introduction

Exponents provide a special way of writing repeated multiplication. Numbers written in this way have a specific form, with each part providing important information about the number. Writing numbers using exponents can save a lot of space, too. The inverse operation of multiplication of a number by itself is called finding the square root of a number. This operation is helpful for problems about the area of a square.

Understanding Exponential Notation

Exponential notation is a special way of writing repeated factors, for example \(\ 7 \cdot 7\). Exponential notation has two parts. One part of the notation is called the base. The base is the number that is being multiplied by itself. The other part of the notation is the exponent, or power. This is the small number written up high to the right of the base. The exponent, or power, tells how many times to use the base as a factor in the multiplication. In the example, \(\ 7 \cdot 7\) can be written as \(\ 7^{2}\) (7 is the base and 2 is the exponent). The exponent 2 means there are two factors.

\(\ 7^{2}=7 \cdot 7=49\)

You can read 7² as “seven squared.” This is because multiplying a number by itself is called “squaring a number.” Similarly, raising a number to a power of 3 is called “cubing the number.” You can read 7³ as “seven cubed.”

You can read 2⁵ as “two to the fifth power” or “two to the power of five,” or “two raised to the power of five.” Read 8⁴ as “eight to the fourth power,” or “eight to the power of four,” or “eight raised to the power of four.” This format can be used to read any number written in exponential notation. In fact, while 6³ is most commonly read “six cubed,” it can also be read “six to the third power,” or “six to the power of three,” or "six raised to the power of three.”

To find the value of a number written in exponential form, rewrite the number as repeated multiplication and perform the multiplication. Two examples are shown below.

Example

Find the value of 4².

Solution

4 is the base. 2 is the exponent.	An exponent means repeated multiplication. The base is 4; 4 is the number being multiplied. The exponent is 2; This means to use two factors of 4 in the multiplication.
\(\ 4^{2}=4 \cdot 4\)	Rewrite as repeated multiplication.
\(\ 4 \cdot 4=16\)	Multiply.

\(\ 4^{2}=16\)

Example

Find the value of 2⁵.

Solution

\(\ 2 \cdot 2 \cdot 2 \cdot 2 \cdot 2\)	Rewrite 2^5 as repeated multiplication. The base is 2, the number being multiplied. The exponent is 5, the number of times to use 2 in the multiplication.
\(\ \begin{array}{r} 2 \cdot 2 \cdot 2 \cdot 2 \cdot 2 \\ 4 \cdot 2 \cdot 2 \cdot 2 \\ 8 \cdot 2 \cdot 2 \\ 16 \cdot 2 \\ 32 \end{array}\)	Perform multiplication.

\(\ 2^{5}=32\)

Exercise

Find the value of 4³.

1. 12
2. 64
3. 256
4. 43

Answer

1. Incorrect. Multiply \(\ 4 \cdot 4 \cdot 4\), not \(\ 4 \cdot 3\). The correct answer is 64.
2. Correct. 4 \(\ \cdot 4 \cdot 4=64\)
3. Incorrect. Use three factors of 4 in the multiplication. The correct answer is 64.
4. Incorrect. 4³ means \(\ 4 \cdot 4 \cdot 4\). The correct answer is 64.

Writing Repeated Multiplication Using Exponents

Writing repeated multiplication in exponential notation can save time and space. Consider the example \(\ 5 \cdot 5 \cdot 5 \cdot 5\). We can use exponential notation to write this repeated multiplication as 5⁴. Since 5 is being multiplied, it is written as the base. Since the base is used 4 times in the multiplication, the exponent is 4. The expression \(\ 5 \cdot 5 \cdot 5 \cdot 5\) can be rewritten in shorthand exponential notation as 5⁴ and is read, “five to the fourth power” or “five to the power of 4.”

To write repeated multiplication of the same number in exponential notation, first write the number being multiplied as the base. Then count how many times that number is used in the multiplication, and write that number as the exponent. Be sure to count the numbers, not the multiplication signs, to determine the exponent.

Example

Write \(\ 7 \cdot 7 \cdot 7\) in exponential notation.

Solution

7 is the base.	The base is the number being multiplied, 7.
Since 7 is used 3 times, 3 is the exponent.	The exponent tells the number of times the base is multiplied.

\(\ 7 \cdot 7 \cdot 7=7^{3}\) This is read “seven cubed.”

Exercise

Write \(\ 10 \cdot 10 \cdot 10 \cdot 10 \cdot 10 \cdot 10\). in exponential notation.

1. 1,000,000
2. 60
3. 10⁵
4. 10⁶

Answer

1. Incorrect. 1,000,000 is equivalent to \(\ 10 \cdot 10 \cdot 10 \cdot 10 \cdot 10 \cdot 10\), but it is not written in exponential notation. The correct answer is 10⁶.
2. Incorrect. This answer is way less than the correct value and is not in exponential notation. You may have thought that six 10s should be written \(\ 6 \cdot 10\) and then simplified that to 60. The correct answer is 10⁶ because the six 10s are multiplied together.

Understanding and Computing Square Roots

As you saw earlier, 5² is called “five squared.” “Five squared” means to multiply five by itself. In mathematics, we call multiplying a number by itself “squaring” the number. We call the result of squaring a whole number a square or a perfect square. A perfect square is any number that can be written as a whole number raised to the power of 2. For example, 9 is a perfect square because 3² is 9. A perfect square number can be represented as a square shape, as shown below. We see that 1, 4, 9, 16, 25, and 36 are examples of perfect squares.

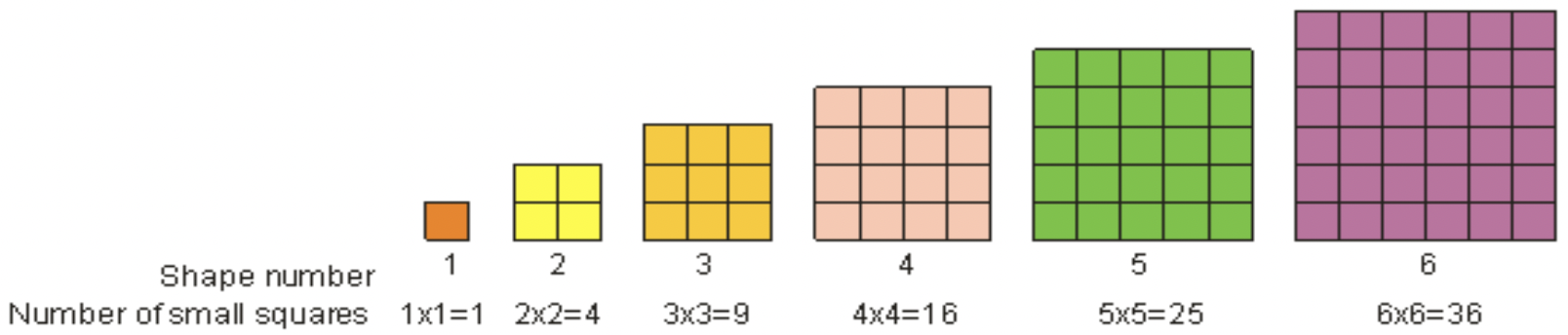
To square a number, multiply the number by itself. 3 squared \(\ =3^{2}=3 \cdot 3=9\).

Below are some more examples of perfect squares.

1 squared	1^2	\(\ 1 \cdot 1\)	1
2 squared	2^2	\(\ 2 \cdot 2\)	4
3 squared	3^2	\(\ 3 \cdot 3\)	9
4 squared	4^2	\(\ 4 \cdot 4\)	16
5 squared	5^2	\(\ 5 \cdot 5\)	25
6 squared	6^2	\(\ 6 \cdot 6\)	36
7 squared	7^2	\(\ 7 \cdot 7\)	49
8 squared	8^2	\(\ 8 \cdot 8\)	64
9 squared	9^2	\(\ 9 \cdot 9\)	81
10 squared	10^2	\(\ 10 \cdot 10\)	100

The inverse operation of squaring a number is called finding the square root of a number. Finding a square root is like asking, “what number multiplied by itself will give me this number?” The square root of 25 is 5, because 5 multiplied by itself is equal to 25. Square roots are written with the mathematical symbol, called a radical sign, which looks like this: \(\ \sqrt{ }\). The “square root of 25" is written \(\ \sqrt{25}\).

Example

Find \(\ \sqrt{81}\).

Solution

\(\ \sqrt{81}=9\)

Think, what number times itself gives 81? \(\ 9 \cdot 9=81\)

\(\ \sqrt{81}=9\)

Exercise

Find \(\ \sqrt{36}\).

1. 6
2. 18
3. 72
4. 7

Answer

1. Correct. Since \(\ 6 \cdot 6=36\), \(\ \sqrt{36}=6\).
2. Incorrect. The square root of 36 is the number that you can multiply by itself to get 36. The square root is not the number you multiply by 2 to get 36. The correct answer is 6, because \(\ 6 \cdot 6=36\).
3. Incorrect. You may have incorrectly added 36 to itself to get 72. The square root of 36 is the number that you can multiply by itself to get 36. The correct answer is 6, because \(\ 6 \cdot 6=36\).
4. Incorrect. \(\ 7 \cdot 7=49\), so \(\ \sqrt{49}=7\). The correct answer is 6, because \(\ 6 \cdot 6=36\). This means \(\ \sqrt{36}=6\).

Summary

Exponential notation is a shorthand way of writing repeated multiplication of the same number. A number written in exponential notation has a base and an exponent, and each of these parts provides information for finding the value of the expression. The base tells what number is being repeatedly multiplied, and the exponent tells how many times the base is used in the multiplication. Exponents 2 and 3 have special names. Raising a base to a power of 2 is called “squaring” a number. Raising a base to a power of 3 is called “cubing” a number. The inverse of squaring a number is finding the square root of a number. To find the square root of a number, ask yourself, “What number can I multiply by itself to get this number?”