3.1: Numbers and Numerals
- Last updated
- Save as PDF
- Page ID
- 82988
You will need: A-blocks (Material Cards 2A, 2B, 2C, 2D, 2E)
Exercise 1
Imagine you came across a person from a remote English-speaking colony where everyone had three fingers on each hand. Although the people of this colony were intelligent, they had never heard of counting and did not know what it was, what it meant or how to do it. They knew nothing about numbers, what they were, what they were called or what they looked like. Think about how you might teach this person about counting and numbers so they could go back and share this wonderful knowledge with their friends. What tools would you use to teach? What are some of the concepts you would have to get across? What difficulties might arise? Do you think this would be an easy or hard task? Write down some of your ideas, strategies and conclusions.
Exercise 2
What do you remember about learning about numbers or how to count? Have you ever helped to teach a young child how to count? If so, what was it like? How long do you think it takes for someone to learn how to count for the first time?
Most of us in the modern world pretty much take the concept of numbers and counting for granted. In fact, we take language for granted –but that topic is too involved to go into right now. Imagine what a breakthrough it was when people first thought to give names to objects. Eventually they used words, which we will call number names, to describe sets of objects (remember sets?!) in terms of their size. This was a considerable mathematical advancement. Although there are still some cultures that have not assigned names for numbers larger than three, most cultures have developed this concept. Some tribes even have different number names for different types of objects.
One difficulty with the concept of numbers as we use them today is that they are concepts and not necessarily used only for counting objects. The number four, for instance, is used in many ways –four years old, room 4, four feet high, 4 children, four hours, four minutes after eight, mail station 4, 4 fingers, a grade of 4 on a quiz, count to 4, four times bigger than something, 4th place, four wishes, fourth in line, call 444-4444, code 44, etc.
So, what is a number?
Well, a number is an abstract idea that represents a quantity. You can't see or touch a number. The symbols that we see and touch that are used to represent numbers are actually numerals. A numeral is made up of one or more symbols. In the Hindu-Arabic system we use, the numerals are made up of one or more of these ten symbols –0,1,2,3,4,5,6,7,8 and 9. For instance, in the Hindu-Arabic system, the number three is represented by writing the numeral three which we write like this: 3 and the number two million is represented by writing the numeral two million which we write like this: 2,000,000. Notice, it took one symbol to write the number for three and seven symbols to write the number for two million (we don't count the commas as symbols because they aren't essential to writing the numeral). Although it took seven symbols to write the numeral two million, note that there are only two different symbols used to write that numeral –a two (2) and a zero (0).
Exercise 3
In your own words, describe the word number.
Exercise 4
In your own words, describe the word numeral.
There are three common uses of numbers. Describing how many elements are in a finite set is the most common use of the counting numbers. When used this way, the number is referred to as a cardinal number. Another way numbers are used concerns order. For instance, someone might be the fifth place winner or you might be eighth in line. When used in this way, numbers are called ordinal numbers. There are also numbers used for identification such as your phone number, zip code, social security number or credit card number. These are called identification numbers and it is the actual order of the symbols that is important –not the value of the number
Exercise 5
When people recognized that a collection of four pebbles and a collection of four bananas had a common property, they were learning the concept of matching. Take out your A-blocks and divide them into subsets according to color. Take the subset of Red A-Blocks and the subset of Blue A-blocks. We will match each element in the Blue A-blocks with an element in the Red A-blocks. There are several ways to do this. I will show two ways to match them. Show a different way to match them under Matching #3 below.
| MATCHING #1 | MATCHING #2 | MATCHING #3 |
|---|---|---|
| SBT \(\leftrightarrow\) LRT | SBT \(\leftrightarrow\) SRQ | |
| LBT \(\leftrightarrow\) SRT | LBT \(\leftrightarrow\) LRQ | |
| SBQ \(\leftrightarrow\) LRQ | SBQ \(\leftrightarrow\) SRC | |
| LBQ \(\leftrightarrow\) SRQ | LBQ \(\leftrightarrow\) LRC | |
| SBC \(\leftrightarrow\) LRC | SBC \(\leftrightarrow\) SRT | |
| LBC \(\leftrightarrow\) SRC | LBC \(\leftrightarrow\) LRT |
Pictures may be used to represent a matching. Below is another way to show Matching #1:
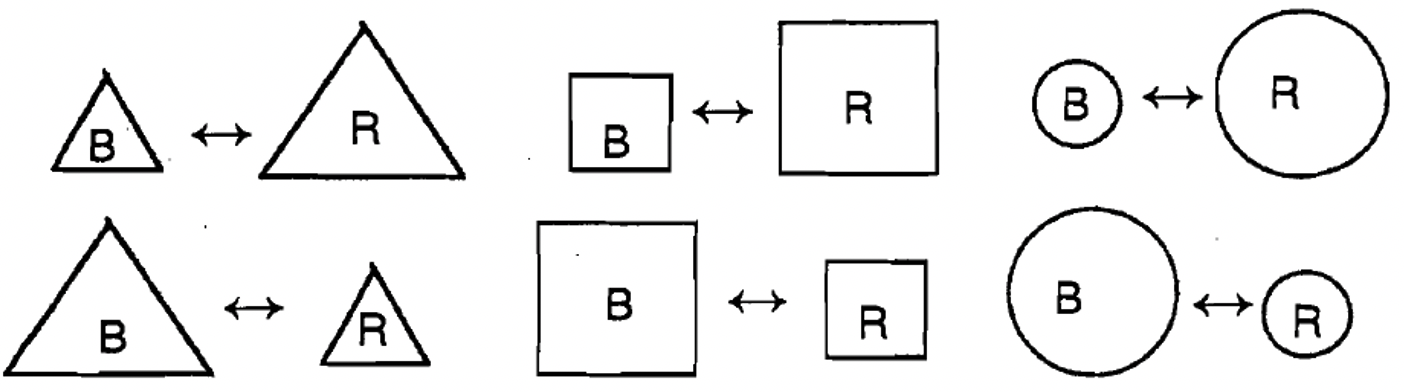
We say that the set of Blue A-blocks match the set of Red A-blocks because we can pair each element of the Blue A-blocks with exactly one element of the Red A-blocks.
Exercise 6
Using pictures, show a matching between the large triangles and the small circles. There is more than one possible way to match them up. Make sure each large triangle is "dancing" with one of the small circles.
NOTE: Two sets that match DO NOT have to match in a natural or pleasing way the way we might match the colors of our clothes together. It might be easier to think of the word pairing instead. Think of showing a matching between two sets up by taking one element in the first set and pairing it up to dance with one element in the second set. Now that they are "dancing" together, take another element from the first set and pick someone in the second set for it to dance with. Continue doing this until all elements are paired up and dancing! This is a matching. If your first set had 5 girls and your second set had 4 boys, they wouldn't match because one girl would be left without a partner. That is why it is necessary to have the same number of elements in each set in order to represent a matching.
Let's match up the subset of Circles with the subset of Squares now. Two different matchings are shown below. Note I've used different formats than in exercise 5.
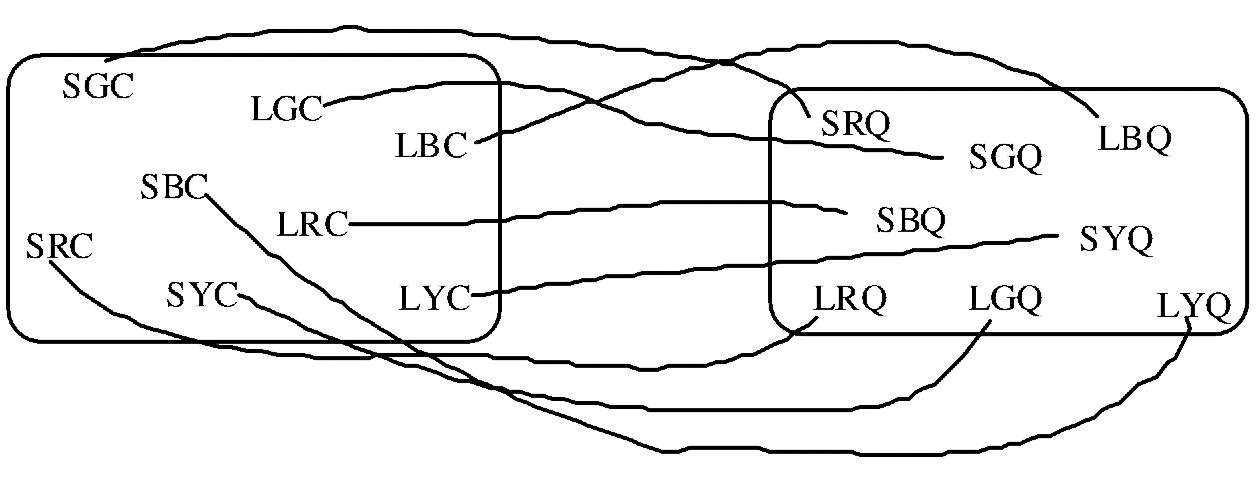
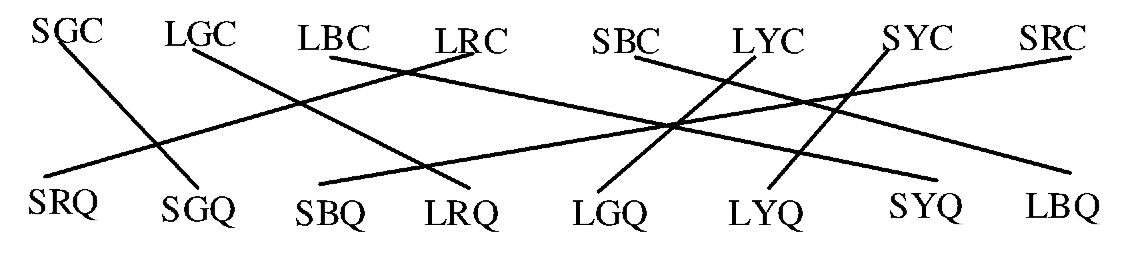
Exercise 7
Show two different ways to match the set of small triangles with the set of large squares.
Each of the pairings we have matched so far illustrates a one-to-one correspondence (1-1) between two sets. We say that two sets match if there is a one-to-one correspondence between their elements.
Exercise 8
If a 1-1 correspondence between two sets exists, what does that imply about the cardinality of the two sets?
Exercise 9
Draw a one-to-one correspondence between the elements of the sets below.
 |
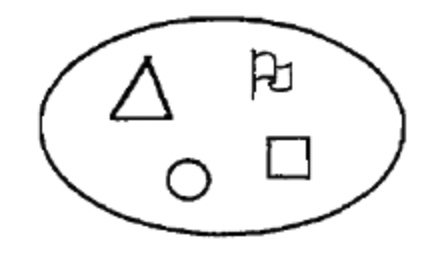 |
To show all of the 1-1 correspondences, or matchings between two sets, I think of all the ways two sets might partner up to do a single dance on the dance floor. Everyone from each set gets exactly one partner from the other set to dance with, and everyone gets to dance. For instance, think of a group of 4 boys–A, B, C and D and 4 girls–W, X, Y and Z. Each boy needs to be paired up with one girl for a dance, so there will be four couples dancing for one dance. One matching would be to show how they pair up for that one dance: maybe A with X, B with Z, C with W and D with Y –that is Matching 11, as shown below. That's only one matching. Note that you do not pair up an element of one set with an element of the same set. For this example, that means a girl only dances with a boy. To show ALL possibilities for this example, you'd have to list all the different ways they might end up as couples on the dance floor for one dance. Each matching shows the four couples, as they are paired up for one dance. To be a different matching, at least two couples would have changed partners. There are actually 24 possible 1-1 correspondences (or different matchings) for this example of four boys and four girls. Below is one way to list all of these possibilities:
|
Matching 1 A \(\leftrightarrow\) W B \(\leftrightarrow\) X C \(\leftrightarrow\) Y D \(\leftrightarrow\) Z |
Matching 2 A \(\leftrightarrow\) W B \(\leftrightarrow\) X C \(\leftrightarrow\) X D \(\leftrightarrow\) Y |
Matching 3 A \(\leftrightarrow\) W B \(\leftrightarrow\) Y C \(\leftrightarrow\) X D \(\leftrightarrow\) Z |
Matching 4 A \(\leftrightarrow\) W B \(\leftrightarrow\) Y C \(\leftrightarrow\) Z D \(\leftrightarrow\) X |
Matching 5 A \(\leftrightarrow\) W B \(\leftrightarrow\) Z C \(\leftrightarrow\) X D \(\leftrightarrow\) Y |
Matching 6 A \(\leftrightarrow\) W B \(\leftrightarrow\) Z C \(\leftrightarrow\) Y D \(\leftrightarrow\) X |
|
Matching 7 A \(\leftrightarrow\) X B \(\leftrightarrow\) W C \(\leftrightarrow\) Y D \(\leftrightarrow\) Z |
Matching 8 A \(\leftrightarrow\) X B \(\leftrightarrow\) W C \(\leftrightarrow\) Z D \(\leftrightarrow\) Y |
Matching 9 A \(\leftrightarrow\) X B \(\leftrightarrow\) Y C \(\leftrightarrow\) W D \(\leftrightarrow\) Z |
Matching 10 A \(\leftrightarrow\) B \(\leftrightarrow\) Y C \(\leftrightarrow\) W D \(\leftrightarrow\) Z |
Matching 11 A \(\leftrightarrow\) X B \(\leftrightarrow\) Z C \(\leftrightarrow\) W D \(\leftrightarrow\) Y |
Matching 12 A \(\leftrightarrow\) X B \(\leftrightarrow\) Z C \(\leftrightarrow\) Y D \(\leftrightarrow\) W |
|
Matching 13 A \(\leftrightarrow\) Y B \(\leftrightarrow\) W C \(\leftrightarrow\) X D \(\leftrightarrow\) Z |
Matching 14 A \(\leftrightarrow\) Y B \(\leftrightarrow\) W C \(\leftrightarrow\) Z D \(\leftrightarrow\) X |
Matching 15 A \(\leftrightarrow\) Y B \(\leftrightarrow\) X C \(\leftrightarrow\) W D \(\leftrightarrow\) Z |
Matching 16 A \(\leftrightarrow\) Y B \(\leftrightarrow\) X C \(\leftrightarrow\) Z D \(\leftrightarrow\) W |
Matching 17 A \(\leftrightarrow\) Y B \(\leftrightarrow\) Z C \(\leftrightarrow\) W D \(\leftrightarrow\) X |
Matching 18 A \(\leftrightarrow\) Y B \(\leftrightarrow\) Z C \(\leftrightarrow\) X D \(\leftrightarrow\) W |
|
Matching 19 A \(\leftrightarrow\) Z B \(\leftrightarrow\) W C \(\leftrightarrow\) Y D \(\leftrightarrow\) X |
Matching 20 A \(\leftrightarrow\) Z B \(\leftrightarrow\) W C \(\leftrightarrow\) X D \(\leftrightarrow\) Y |
Matching 21 A \(\leftrightarrow\) Z B \(\leftrightarrow\) X C \(\leftrightarrow\) W D \(\leftrightarrow\) Y |
Matching 22 A \(\leftrightarrow\) Z B \(\leftrightarrow\) X C \(\leftrightarrow\) Y D \(\leftrightarrow\) W |
Matching 23 A \(\leftrightarrow\) Z B \(\leftrightarrow\) Y C \(\leftrightarrow\) W D \(\leftrightarrow\) X |
Matching 24 A \(\leftrightarrow\) Z B \(\leftrightarrow\) Y C \(\leftrightarrow\) X D \(\leftrightarrow\) W |
If you were asked to list one matching between two sets {A, B, C, D} and {W, X, Y, Z}, any of the 24 matchings shown above would be fine. However, if you are asked to list ALL possibilities, you must be very clear what constitutes a matching, as I've illustrated above. In each matching, note the individual pairings (who is dancing with whom) must be shown. If the sets have one element each, then each matching has one pairing. If the sets have two elements each, then each matching has two pairings. If the sets have three elements each, then each matching has three pairings. And, if the sets have four elements each (as in the example above), then each matching has four pairings.
See if you notice any patterns above before going on. It is much easier to show the matchings when there are fewer elements in the sets. If there is one element in each set, then it's like being on a desert island, one man and one woman. There isn't much choice, is there?
Exercise 10
Show every possible 1-1 correspondence (or matching) between the sets.
| a. {1} and {A} | b. {2,3} and {B,C} | c. {4,5,6} and {D,E,F} |
It's important to realize the two sets that are being matched may contain one, some or all of the same elements. Study the following examples, where each set contains the exact same elements.
Examples: Show every possible 1-1 correspondence (or matching) between the sets listed.
Example 1
{3} and {3}
Solution
Matching 1
3 \(\leftrightarrow\) 3
Example 2
{6, 7} and {6, 7}
Solution
|
Matching 1 6 \(\leftrightarrow\) 6 7 \(\leftrightarrow\) 7 |
Matching 2 6 \(\leftrightarrow\) 7 7 \(\leftrightarrow\) 6 |
Example 3
{a, b, c} and {a, b, c}
Solution
|
Matching 1 a \(\leftrightarrow\) a b \(\leftrightarrow\) b c \(\leftrightarrow\) c |
Matching 2 a \(\leftrightarrow\) a b \(\leftrightarrow\) c c \(\leftrightarrow\) b |
Matching 1 a \(\leftrightarrow\) b b \(\leftrightarrow\) a c \(\leftrightarrow\) c |
Matching 1 a \(\leftrightarrow\) a b \(\leftrightarrow\) b c \(\leftrightarrow\) c |
Matching 1 a \(\leftrightarrow\) a b \(\leftrightarrow\) b c \(\leftrightarrow\) c |
Matching 1 a \(\leftrightarrow\) a b \(\leftrightarrow\) b c \(\leftrightarrow\) c |
Exercise 11
Show every possible 1-1 correspondence (or matching) between the sets listed. This exercise continues on the next page. Label the matchings, so it is clear how many matchings there are, and what pairs are in each matching.
| a. {M} and {M} | b. {x,y} and {x,z} | c. {1, 2} and {1, 2} | d. {1, 2, 3} and {1, 2, 3} | e. {1, 2, 3} and {3, 4, 5} |
Exercise 12
If you must list all the possible 1-1 correspondences between 2 sets, each having the cardinality listed below, how many possible matchings are there?
| a. 1 element each: ___ | b. 2 elements each: ___ | c. 3 elements each: ___ |
The formula for figuring out the number of 1-1 correspondences between sets having n elements is n! (this is read "n factorial"). To figure out what number n! is, multiply all the counting number together up to n. For instance, 1! = 1, 2! = 1 \(\cdot\) 2 = 2, 3! = 1 \(\cdot\) 2 \(\cdot\) 3 = 6, and 4! = 1 \(\cdot\) 2 \(\cdot\) 3 \(\cdot\) 4 = 24. In the very first example where I listed all the possible matchings for two sets, each having 4 elements, there were 24 possibilities. Did you get the right number of possibilities for all the ones you did?
It's time to point out some straightforward, yet important properties of matching.
Property 1
A set always matches itself.
Two sets that match do not have to be different from each other. For instance, you could match {X,Y,Z} with {X,Y,Z} by showing this natural one-to-one correspondence: X « X, Y « Y and Z « Z. (Or you can match them some other way. Here are two other ways to show a matching: X « Z, Y « X and Z « Y OR X « X, Y « Z and Z « Y.)
Property 2
For any two sets A and B, if A matches B, then B matches A.
This property emphasizes that the order in which the sets are mentioned is irrelevant.
Property 3
For any three sets, if A matches B and B matches C, then A matches C.
Exercise 13
Illustrate Property 3 by letting A be the set of small circles, B be the set of small triangles and C be the set of small squares. List the abbreviations (SRC, etc.) or draw the picture for each of the individual elements in each set! Three separate matchings, each having 4 pairings, should be shown. The first matching should show one matching between A and B, then there should be another matching shown between B and C. Last, there should be a matching shown between A and C.
|
Matching between A and B: |
Matching between B and C: |
Matching between A and C: |
Today, most but not all civilizations around the world use the Hindu-Arabic system of counting and numerals 1,2,3,4,5,6,7,8,9,10,11,12,... There are many advantages to this number system. While you might think this common, widely used system of counting the obvious choice, you might consider both the advantages and disadvantages of Hindu-Arabic as we learn about other numeration systems.
Exercise 14
Consider the STROKE system where there was only one symbol, a simple vertical stroke, to express a number. A vertical stroke like this, |, expressed one. To express the number seven, you would write seven strokes: | | | | | | |
a. Show how you would write the numeral eleven in STROKE:
b. Describe in words how to write the numerals five hundred twelve ; and two million in STROKE. Don't attempt to write the actual strokes!! We only have a semester!
Exercise 15
Name some advantages and disadvantages of the STROKE system, compared with the Hindu-Arabic system.
The tally system improved the STROKE system by introducing the concept of grouping. You are probably familiar with this system where the fifth stroke was placed across the previous four so it would be easier to read since you can count by fives. STROKE is a single symbol system, but Tally can be thought of as a two symbol system, although the second symbol is really just made up of five strokes. Note the difference in writing the numeral twenty-eight in the two systems.
|
STROKE system: ||||||||||||||||||||||||||||||| |
Tally system: \(\cancel{||||}\cancel{||||}\cancel{||||}\cancel{||||}\cancel{||||}|||\) |
Exercise 16
a. Write the numeral for 17 in STROKE.
b. Write the numeral for 17 in Tally.
Exercise 17
If you think of a single stroke (|) as one symbol and the 5-stroke tally (\(\cancel{||||}\)) as a separate symbol, then it takes eight symbols to express the numeral for 28 as shown above Exercise 13. In STROKE, it took 28 symbols to write the same number. How many symbols does it take to express the numeral for 172 in TALLY?
The Old Egyptian numeral system which was developed around 3400 B.C., employs an additive system of counting, where the symbols of the numeral do not have to be in any special order or even on a line going from left to right. The seven symbols in this ancient system are listed below, along with their Hindu-Arabic equivalents.
 |
 |
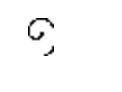 |
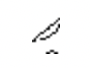 |
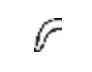 |
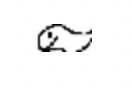 |
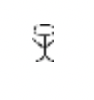 |
| Staff | Heel bone | Scroll | Lotus flower | Pointing finger | Polliwog | Astonished man |
| One | Ten | Hundred | Thousand | Ten thousand | Hundred thousand | Million |
| 1 | 10 | 100 | 1,000 | 10,000 | 100,000 | 1,000,000 |
Here are three different ways to write the numeral sixty five in the Old Egyptian system:
| \(\cap\cap\cap\cap\cap\cap\)|||||| | or | \(\cap\cap\||\cap|\cap\cap||\cap\) |
or as shown below with all the symbols enclosed in a visual set
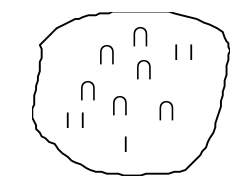
You simply add up the values of each of the symbols to get the answer.
Definition: additive principle
The additive principle states that the value of a set of symbols is the sum of the value of the symbols
In this additive system, the Egyptian numeral represents
1,000,000 + 10,000 + 1,000 + 1,000 + 1,000 + 100 + 100 + 1 + 1 + 1 + 1 = 1,013,204.
Remember: The order in which the symbols are written is irrelevant in an additive system.
In addition to being able to write the symbols of the numeral in any order, you may also use a different combination of symbols used to represent the same number. Here are two different ways to write the number for one hundred twenty three:
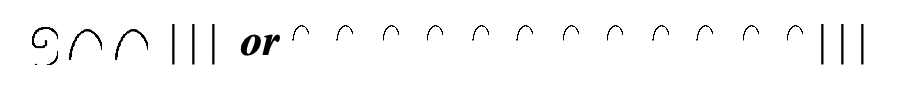
Exercise 18
Explain in words two more ways you could write the numeral one hundred twenty three by using a different combination of symbols than shown above.
Below is a reminder of what each symbol stands for in order to do the next few exercises.
 |
 |
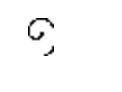 |
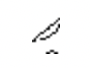 |
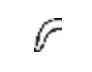 |
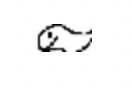 |
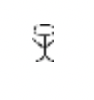 |
| Staff | Heel bone | Scroll | Lotus flower | Pointing finger | Polliwog | Astonished man |
| One | Ten | Hundred | Thousand | Ten thousand | Hundred thousand | Million |
| 1 | 10 | 100 | 1,000 | 10,000 | 100,000 | 1,000,000 |
Exercise 19
Express each Egyptian numeral below as a Hindu-Arabic numeral. Show work.
|
a.  |
|
b.  |
|
c. 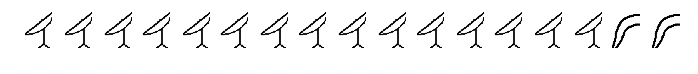 |
|
d.  |
Exercise 20
Express each Hindu-Arabic numeral below as an Egyptian numeral using the least number of symbols possible. Show work.
| a. 407 |
| b. 3,051,040 |
| c. 232,501 |
| d. 4,000,000 |
| e. 1,111,111 |
The Egyptian system employs the technique of grouping, where a certain number of the same symbols are grouped together and replaced with a new symbol. This is necessary in order to symbolize very large numbers efficiently. Systems that use this grouping principle are said to be of a certain base depending on how many symbols it takes to exchange to a new symbol. For instance, a system that groups six symbols together and then replaces them with a new symbol is said to be a Base Six system.
Exercise 21
In what base is the Egyptian system? What is the limitation of this system as far as grouping is concerned? (Think about how to write four trillion!)
Exercise 22
Name some advantages and disadvantages of the Old Egyptian system compared with the Hindu-Arabic sytem, the STROKE system and the Tally system.
Exercise 23
Here are examples of some Roman numerals: XVI, MCMLX, XIX, xii, iv, iii. Name some places you still see Roman numerals today.
Extra Credit: Post a picture on the Forum of Roman Numerals in the real world. It must be your own photo not one copied from the internet or another source. Make sure you are in the picture and include the value as a Hindu-Arabic numeral.
The Roman numeration system was in use by 300 B.C. The basic Roman numerals and their corresponding values are listed below. We'll be writing with upper case letters.
| I: 1 | V: 5 | X: 10 | L: 50 | C: 100 | D: 500 | M: 1000 |
Originally, the Roman Numeration System was a simple additive system –for example, DCCCLXXXVII = 500 + 100 + 100 + 100 + 50 + 10 + 10 + 10 + 5 + 1 + 1 = 887. It is actually a Base Ten system with additional symbols for 5, 50 and 500. These extra symbols significantly reduce the amount of writing one must do. For instance, writing the Roman numeral eight hundred eighty seven without the use of the additional symbols would look like this: CCCCCCCCXXXXXXXXIIIIIII. Writing this same numeral using the additional symbols would look like this: DCCCLXXXVII. Without the V, L and D, one would need to write out more than twice as many symbols for this particular numeral.
Exercise 24
Write each Hindu-Arabic numeral as a Roman numeral using the least amount of symbols:
| a. 32: | b. 561: |
| c. 708: | d. 2053: |
Exercise 25
Write each Roman numeral as a Hindu-Arabic numeral and show work:
| a. MMDCLXXXV11: ____ | b. MCCXXXII:_____ |
The Roman numeration system developed and incorporated changes over time. One limitation of a simple additive system is that you eventually run out of symbols for very large numbers. Did you already figure that out when answering exercise 19? The Romans devised a clever way to take care of this problem. If a bar was placed over a symbol or set of symbols, it indicated that number was to be multiplied by a thousand. More than one bar could be used on any given symbol or set of numerals. For example, two bars indicated the number was to be multiplied by 1000 1000 (or one million). Simply put, symbols with one bar over them are in the thousands, those with two bars over them are in the millions, those with three bars over them are in the billions and so on. Thus, the Roman numeration system is multiplicative as well as additive. Study this feature in the following examples:
Example 1
\(\bar{\text{CCLVI}}\)CCX = (100 + 100 + 50 + 5 + 1) 1000 + 100 + 100 + 10 = 256,210
Exercise 2
\(\bar{\bar{\text{V}}\text{LX}}\)DX = 5 1000000 + (50 + 10) 1000 + 500 + 10 = 5,060,510
Exercise 26
Write each Hindu-Arabic numeral as a Roman numeral using the least amount of symbols possible.
| a. 330,802 ____ | b. 70,001,651 ____ |
Exercise 27
Write each Roman numeral as a Hindu-Arabic numeral: Show work.
| a. \(\bar{\text{III}}\)CCXII | b. \(\bar{\text{LX}}\)DCL |
Over time, the Roman numeration system developed a subtractive principle. If a symbol representing a smaller number is to the left of a symbol representing a larger number, then the total value of those two symbols together represented the value of the larger symbol minus the value of the smaller symbol. There were specific conditions. Only symbols representing powers of ten (I,X,C,M) could be subtracted and each of these four could only be paired with the next two larger symbols. So, the following are the only possibilities for using the subtractive principle:
| IV: 4 | IX: 9 | XL: 40 | XC: 90 | CD: 400 | CM: 900 |
As you read a Roman numeral from left to right, use the additive principle. If a symbol denoting a smaller value precedes a symbol denoting a larger value, use the subtractive principle when reading those two symbols. Study the following examples:
Example 1
CMXXXIX = (1000-100) + 10 + 10 + 10 + (10 - 1) = 939
Example 2
\(\bar{\text{XCIV}}\)CCXCII = (100-10 + 5-1) 1000 + 100 + 100 + (100-10) + 1 + 1 = 94,292
Exercise 28
Rewrite each Roman numeral without using the subtractive principle:
| a. CMXLIV: ____ |
| b. CDXCIX: ____ |
Exercise 29
Rewrite each Roman numeral using the least number of symbols possible and the subtractive principle where applicable:
| a. CCCCCCCXXXXIIIIIIII ____ |
| b. MMMMMMMMMMMMMMCCCCXXXXXXXX ____ |
| c. CCCCCCCCCXXXXXXIIIIIIIII ____ |
| d. CCCCXXXXII ____ |
Exercise 30
Write each Hindu-Arabic numeral as a Roman numeral using the least number of symbols possible and the subtractive principle where applicable:
| a. 19,453 ____ |
| b. 2,849 ____ |
| c. 1,996 ____ |
From now on, because this is how they are used today, write all Roman numerals using the least number of symbols possible and the subtractive principle where applicable unless you are otherwise instructed.
My birthdate is July 15, 1958. If I were to write this date as a six digit numeral (the first two for the month, the second two for the day and the last two for the year), I would write 071558. This is how to write 71,558 as a Roman numeral: \(\bar{\text{LXXI}}\)DLVIII.
Exercise 31
Write your birthdate as a six digit numeral like I did for mine above. Then, write it in Roman numerals. If you prefer, you can lie or use the birthdate of someone you love!
Exercise 32
For numbers under a thousand, indicate the most times each symbol below can be repeated in a row when written as part of a Roman numeral. Assume the subtractive principle is used.
In this exercise set, you have learned some basic concepts of counting and have also learned about some numeration systems. In the next set, you'll learn about two more numeration systems. We'll end these exercises by comparing the systems we have worked with so far. First, we will consider the number of symbols one has to memorize in order to understand each individual system. We will then determine how many symbols one has to write down in order to represent various numerals in the different systems.
Exercise 33
For each of the numeration systems we've learned about so far, state how many different symbols a person has to memorize to understand the system. For Roman, do not consider symbols with bars over them as different symbols.
| a. Hindu-Arabic: _____ | b. STROKE: ____ | c. Tally: ____ | d. Egyptian: ____ | e. Roman: ____ |
Let's write the numeral 67 in the different systems and note how many total symbols (not necessarily different symbols) it takes to express the number 67 in each system.
| Hindu-Arabic: | We write: 67 | 2 symbols |
| STROKE: |
We write 67 strokes, shown below |||||||||||||||||||||||||||||||||||||||||||||||||||| |
67 symbols |
| Tally: |
WE write 13 tally groups and 2 strokes, shown below \(\cancel{||||}\cancel{||||}\cancel{||||}\cancel{||||}\cancel{||||}\cancel{||||}\cancel{||||}\cancel{||||}\cancel{||||}\cancel{||||}\cancel{||||}\cancel{||||}\cancel{||||} ||\) |
15 symbols |
| Egyptian: | We write: | 13 symbols |
| Roman: | We write: LXVII | 5 symbols |
Exercise 34
Write the numeral for 900 in each of the five systems below and state how many symbols it takes to write out the full numeral. Rather than writing the numerals in STROKE and Tally, explain in words how you would write them, still indicating the total number of symbols that would have to be written.
| a. Hindu-Arabic: _____ | b. STROKE: ____ | c. Tally: ____ | d. Egyptian: ____ | e. Roman: ____ |
Exercise 35
For each Hindu-Arabic number across the top row, state the least number of symbols it takes to write the numeral in each of the given numeration systems on the left. Some answers are filled in for you. You don't need to actually write the numeral –just determine how many symbols you would have to write down if you were going to write the numeral. Write your answer in Hindu-Arabic.
| 143 | 400 | 1,000,000 | 30,009 | 2,124 | |
| Hindu-Arabic | 3 | 7 | |||
| Stroke | 30,009 | ||||
| Tally | 31 | 200,000 | |||
| Egyptian | 9 | ||||
| Roman | 2 |


