5.7: Exponential and Logarithmic Equations
( \newcommand{\kernel}{\mathrm{null}\,}\)
- Use like bases to solve exponential equations.
- Use logarithms to solve exponential equations.
- Use the definition of a logarithm to solve logarithmic equations.
- Use the one-to-one property of logarithms to solve logarithmic equations.
- Solve applied problems involving exponential and logarithmic equations.
In 1859, an Australian landowner named Thomas Austin released 24 rabbits into the wild for hunting. Because Australia had few predators and ample food, the rabbit population exploded. In fewer than ten years, the rabbit population numbered in the millions.

Uncontrolled population growth, as in the wild rabbits in Australia, can be modeled with exponential functions. Equations resulting from those exponential functions can be solved to analyze and make predictions about exponential growth. In this section, we will learn techniques for solving exponential and logarithmic equations.
Exponential Equations
Exponential equations are equations that contain variables in the exponent.
Some examples of exponential equations: 2x=23x−78t−3+4=7
There are two main techniques to solving exponential equations. The first technique is for solving equations that involve like bases. The second technique is for solving equations that we are unable to write with the same base.
Using Like Bases to Solve Exponential Equations
Consider the equation 34x−7=32x. Notice that the bases are the same. To have equivalency, the exponents must be equal: 4x−7=2x. Solving this, we get x=72.
The one-to-one property of exponential functions tells us that, for any real numbers b, S, and T, where b>0, b≠1,
bS=bT if and only if S=T.
Solve 2x−1=22x−4
Solution
2x−1=22x−4The common base is 2x−1=2x−4By the one-to-one property the exponents must be equalx=3Solve for x
Solve 52x=53x+2
- Answer
-
x=−2
Sometimes the common base for an exponential equation is not explicitly shown. In these cases, we simply rewrite the terms in the equation as powers with a common base, and solve using the one-to-one property.
For example, consider the equation 256=4x−5. We can rewrite both sides of this equation as a power of 2. Then we apply the rules of exponents, along with the one-to-one property, to solve for x:
16=2x−524=2x−5Rewrite 16 as a power with base 24=x−5Apply the one-to-one property of exponents9=xAdd 5 to both sides
Solve 8x+2=16x+1
Solution
8x+2=16x+1(23)x+2=(24)x+1Write 8 and 16 as powers of 223x+6=24x+4To take a power of a power, multiply exponents3x+6=4x+4Use the one-to-one property to set the exponents equalx=2Solve for x
Solve 52x=253x+2
- Answer
-
x=−1
Solve 25x=√2
Solution
25x=212Write the square root of 2 as a power of 25x=12Use the one-to-one propertyx=110Solve for x
Solve 5x=√5
- Answer
-
x=12
Solving Exponential Equations Using Logarithms
Sometimes the terms of an exponential equation cannot be rewritten with a common base. The one-to-one property for logarithms tells us that, for real numbers a>0 and b>0, log(a)=log(b) is equivalent to a=b. This means that we may apply logarithms with the same base on both sides of an exponential equation. It is most common to apply the natural logarithm on both sides but any logarithms with the same base applied to both sides may be used.
Solve 5x+2=4x
Solution
5x+2=4xThere is no easy way to get the powers to have the same baseln5x+2=ln4xTake ln of both sides(x+2)ln5=xln4Use laws of logsxln5+2ln5=xln4Use the distributive lawxln5−xln4=−2ln5Get terms containing x on one side, terms without x on the otherx(ln5−ln4)=−2ln5On the left hand side, factor out an xxln(54)=ln(125)Use the laws of logsx=ln(125)ln(54)Divide by the coefficient of xx=−ln25ln5−ln4simplify using Laws of Logarithms
Solve 2x=3x
Solution
ln2x=ln3xTake ln of both sidesxln2=xln3move exponents to the frontxln2−xln3=0get like terms on one sidex(ln2−ln3)=0factor out xx=0ln2−ln3divide both sides byln2−ln3x=0
Solve 2x=3x+1
- Answer
-
x=ln3ln2−ln3(23) or x=ln3ln2−ln3
Solve 5x=11. Find the exact answer and then approximate it to three decimal places.
Solution:
5x=11
Since the exponential is isolated, take the logarithm of both sides.
log5x=log11
Use the Power Property to get the x as a factor, not an exponent.
xlog5=log11
Solve for x. Find the exact answer.
x=log11log5
Approximate the answer.
x≈1.490
Since 51=5 and 52=25, it makes sense that 51.490≈11.
Note in the previous example that y=5x is a basic exponential function. We know what the graph looks like and how the function values will start to become large quite quickly for larger x-values since this is an increasing exponential function of base 5, it seems reasonable that the function value of 11 would occur at a smaller x-value. Also, knowing that there is are points on the graph at (0,1) and at (1,5), this would indicate that the function would equal 11 for an x-value that is is just a bit bigger than 1.
Solve 7x=43. Find the exact answer and then approximate it to three decimal places.
- Answer
-
x=log43log7≈1.933
One common type of exponential equations are those with base e. This constant occurs again and again in nature, in mathematics, in science, in engineering, and in finance. When we have an equation with a base e on either side, we can use the natural logarithm to solve it.
Solve 100=20e2t
Solution
100=20e2t5=e2tDivide by the coefficient of the powerln5=2tTake ln of both sides. Use the fact that ln(x) and ex are inverse functionst=ln52Divide by the coefficient of t
Analysis
Using laws of logs, we can also write this answer in the form t=ln√5. If we want a decimal approximation of the answer, we use a calculator.
Solve 3e0.5t=11
- Answer
-
t=2ln(113) or ln(113)2
Solve 4e2x+5=12
Solution
4e2x+5=124e2x=7Combine like termse2x=74Divide by the coefficient of the power2x=ln(74)Take ln of both sidesx=12ln(74)Solve for x
Solve 3+e2t=7e2t
- Answer
-
t=ln(1√2)=−12ln(2)
Solve 3ex+2=24. Find the exact answer and then approximate it to three decimal places.
Solution:
3ex+2=24
Isolate the exponential by dividing both sides by 3.
ex+2=8
Take the natural logarithm of both sides.
lnex+2=ln8
Use the Power Property to get the x as a factor, not an exponent.
(x+2)lne=ln8
Use the property lne=1 to simplify.
x+2=ln8
Solve the equation. Find the exact answer.
x=ln8−2
Approximate the answer.
x≈0.079
Solve 5e2x=25. Find the exact answer and then approximate it to three decimal places.
- Answer
-
x=ln52≈0.805
You may be wondering if all exponential equations have solutions. The answer is not all exponential equations have solutions.
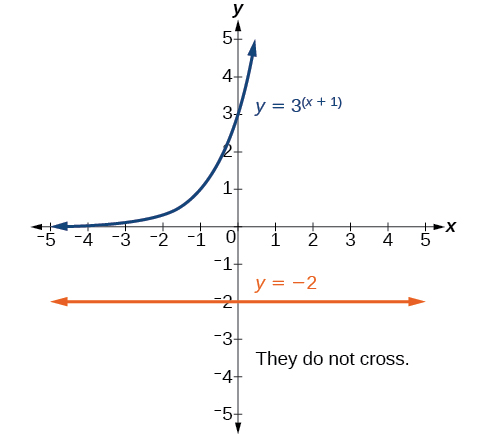
The equation 3x+1=−2 has no solution. There is no real value of x that will make the equation a true statement because any power of a positive number is positive.
Figure 5.7.2 shows that the two graphs do not cross so the left side is never equal to the right side. Thus the equation has no solution.
Another example of an equation that has no solution is 2=−3et.
Figure 5.7.3 shows that the two graphs do not cross so the left side is never equal to the right side. Thus the equation has no solution.

Checking for Extraneous Solutions When Solving Exponential Equations
Sometimes the methods used to solve an equation introduce an extraneous solution, which is a solution that is correct algebraically but does not satisfy the conditions of the original equation. One such situation arises in solving when the logarithm is taken on both sides of the equation. In such cases, remember that the argument of the logarithm must be positive. If the number we are evaluating in a logarithm function is negative, there is no output.
Solve e2x−ex=56
Solution
e2x−ex=56e2x−ex−56=0Get one side of the equation equal to zero since this equation is of quadratic form(ex+7)(ex−8)=0Factor by the FOIL method
Solving for each factor, ex+7=0orex−8=0ex=−7orex=8ex=−7 has no solution since x=ln(−7) is undefined. Solving ex=8,x=ln8
Solve e2x=ex+2
- Answer
-
x=ln2
Logarithmic Equations
Logarithmic equations are equations that contain logarithms with variables.
Some examples of logarithmic equations: log5x=8ln(t)−ln(t−2)=ln11
There are two main techniques to solving logarithmic equations. The first technique is for solving equations that contain only logarithmic terms with the same base. The second technique is for solving equations that contain logarithmic terms and constants.
Using the One-to-One Property of Logarithms to Solve Logarithmic Equations
As with exponential equations, we can use the one-to-one property to solve logarithmic equations. The one-to-one property of logarithmic functions tells us that, for any real numbers x>0, S>0, T>0 and any positive real number b, where b≠1,
logbS=logbT if and only if S=T.
For example,
If log2(x−1)=log2(8), then x−1=8.
So, if x−1=8, then we can solve for x,and we get x=9. To check, we can substitute x=9 into the original equation: log2(9−1)=log2(8)=3. In other words, when a logarithmic equation has the same base on each side, the arguments must be equal. This also applies when the arguments are algebraic expressions. Therefore, when given an equation with logs of the same base on each side, we can use rules of logarithms to rewrite each side as a single logarithm. Then we use the fact that logarithmic functions are one-to-one to set the arguments equal to one another and solve for the unknown.
For example, consider the equation log(3x−2)−log(2)=log(x+4). To solve this equation, we can use the rules of logarithms to rewrite the left side as a single logarithm, and then apply the one-to-one property to solve for x:
log(3x−2)−log(2)=log(x+4)log(3x−22)=log(x+4)Apply the quotient rule of logarithms3x−22=x+4Apply the one to one property of a logarithm3x−2=2x+8Multiply both sides of the equation by 2x=10Subtract 2x and add 2
To check the result, substitute x=10 into log(3x−2)−log(2)=log(x+4).
log(3(10)−2)−log(2)=log((10)+4)log(28)−log(2)=log(14)log(282)=log(14)The solution checks
Solve ln(x2)=ln(2x+3)
Solution
ln(x2)=ln(2x+3)x2=2x+3Use the one-to-one property of the logarithmx2−2x−3=0Get zero on one side before factoring(x−3)(x+1)=0Factor
Solving for each factor, x−3=0 or x+1=0 x=3 or x=−1
Analysis
There are two solutions: 3 or −1. The solution −1 is negative, but it checks when substituted into the original equation because the argument of the logarithm functions is still positive.
Solve ln(x2)=ln1
- Answer
-
x=1 or x=−1
Using the Definition of a Logarithm to Solve Logarithmic Equations
We have already seen that every logarithmic equation logb(x)=y is equivalent to the exponential equation by=x. We can use this fact, along with the rules of logarithms, to solve logarithmic equations where the argument is an algebraic expression.
For example, consider the equation log2(2)+log2(3x−5)=3. To solve this equation, we can use rules of logarithms to rewrite the left side in compact form and then apply the definition of logs to solve for x:
log2(2)+log2(3x−5)=3log2(2(3x−5))=3Apply the product rule of logarithmslog2(6x−10)=3Distribute23=6x−10Convert to exponential form8=6x−10Calculate 2318=6xAdd 10 to both sidesx=3Divide by 6
Solve 2lnx+3=7
Solution
2lnx+3=72lnx=4Subtract 3lnx=2Divide by 2x=e2Rewrite in exponential form
Solve 6+lnx=10
- Answer
-
x=e4
Solve 2ln(6x)=7
Solution
2ln(6x)=7ln(6x)=72Divide by 26x=e(72)convert to exponential formlnx=16e(72)Divide by 6
Solve 2ln(x+1)=10
- Answer
-
x=e5−1
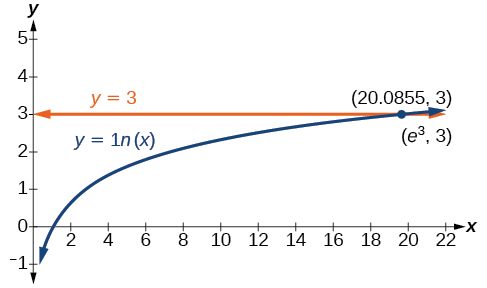
Solve lnx=3
Solution
lnx=3x=e3
Figure 5.7.4 represents the graph of the equation. On the graph, the x-coordinate of the point at which the two graphs intersect is close to 20. In other words e3≈20. A calculator gives a better approximation: e3≈20.0855.
Use a graphing calculator to estimate the approximate solution to the logarithmic equation 2x=1000 to 2 decimal places.
- Answer
-
x≈9.97
Checking for Extraneous Solutions When Solving Logarithmic Equations
It is possible to have extraneous solutions when solving logarithmic equations since the domain of a logarithm is (0,∞). Check your answers to see if they are extraneous or not.
Solve lnx+ln(2x−5)=ln3
Solution
lnx+ln(2x−5)=ln3ln(x(2x−5))=ln3ln(2x2−5x)=ln32x2−5x=32x2−5x−3=0(2x+1)(x−3)=02x+1=0x−3=0x=12x=3
Solve: log4(x+6)−log4(2x+5)=−log4x
Solution:
log4(x+6)−log4(2x+5)=−log4x
Use the Quotient Property on the left side and the Power Property on the right.
log4(x+62x+5)=log4x−1
Rewrite x−1=1x.
log4(x+62x+5)=log41x
Use the One-to-One Property, if logaM=logaN, then M=N.
x+62x+5=1x
Solve the rational equation.
x(x+6)=2x+5
Distribute.
x2+6x=2x+5
Write in standard form.
x2+4x−5=0
Factor.
(x+5)(x−1)=0
Use the Zero-Product-Property.
x+5=0,x−1=0
Solve each equation.
x=−5,x=1
Check.
We leave the check for you.
Solve: 2log5x=log581
Solution:
2log5x=log581
Use the Power Property.
log5x2=log581
Use the One-to-One Property, if logaM=logaN, then M=N.
x2=81
Solve using the Square Root Property.
x=±9
We eliminate x=−9 as we cannot take the logarithm of a negative number.
x=9,x=−9
Check. x=9
2log5x=log5812log59?=log581log592?=log581log581=log581
Solve: 2log3x=log336
- Answer
-
x=6
Solve: log(x+2)−log(4x+3)=−logx
- Answer
-
x=3
Solve: log(x−2)−log(4x+16)=log1x
- Answer
-
x=8
Solving Applied Problems
In previous sections, we learned the properties and rules for both exponential and logarithmic functions. We have seen that any exponential function can be written as a logarithmic function and vice versa. We have used exponents to solve logarithmic equations and logarithms to solve exponential equations. We are now ready to combine our skills to solve equations that model real-world situations, whether the unknown is in an exponent or in the argument of a logarithm.
Suppose $2000 is invested in an account which offers 7.125% compounded monthly.
- Express the amount A in the account as a function of the term of the investment t in years.
- How much is in the account after 5 years?
- How long will it take for the initial investment to double?
- Find and interpret the average rate of change of the amount in the account from the end of the fourth year to the end of the fifth year, and from the end of the thirty-fourth year to the end of the thirty-fifth year.
Solution
- Substituting P=2000, r=0.07125, and n=12 (since interest is compounded monthly into the compound interest formula yields A(t)=2000(1+0.0712512)12t=2000(1.0059375)12t
- Since t represents the length of the investment in years, we substitute t=5 into A(t) to find A(5)=2000(1.0059375)12(5)≈2852.92After 5 years, we have approximately $2852.92.
- Our initial investment is $2000, so to find the time it takes this to double, we need to find t when A(t)=4000. We get 2000(1.0059375)12t=4000, or (1.0059375)12t=2. Taking natural logs of both sides, we get t=ln(2)12ln(1.0059375)≈9.75. Hence, it takes approximately 9 years 9 months for the investment to double.
- To find the average rate of change of A from the end of the fourth year to the end of the fifth year, we compute A(5)−A(4)5−4≈195.63 Similarly, the average rate of change of A from the end of the thirty-fourth year to the end of the thirty-fifth year is A(35)−A(34)35−34≈1648.21 This means that the value of the investment is increasing at a rate of approximately $195.63 per year between the end of the fourth and fifth years, while that rate jumps to $1648.21 per year between the end of the thirty-fourth and thirty-fifth years. So, not only is it true that the longer you wait, the more money you have, but also the longer you wait, the faster the money increases. (in fact, the rate of increase of the amount in the account is exponential as well. This is the quality that really defines exponential functions and we refer the reader to a course in Calculus.


