1.5E: Exercises
- Last updated
- Sep 14, 2021
- Save as PDF
- Page ID
- 84726
( \newcommand{\kernel}{\mathrm{null}\,}\)
1) Given ⇀r(t)=(3t2−2)ˆi+(2t−sint)ˆj,
a. find the velocity of a particle moving along this curve.
b. find the acceleration of a particle moving along this curve.
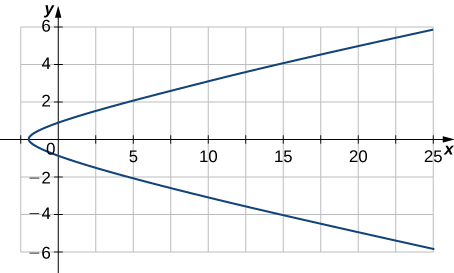
- Answer
- a. ⇀v(t)=6tˆi+(2−cost)ˆi
b. ⇀a(t)=6ˆi+sintˆi
In questions 2 - 5, given the position function, find the velocity, acceleration, and speed in terms of the parameter t.
2) ⇀r(t)=e−tˆi+t2ˆj+tantˆk
3) ⇀r(t)=⟨3cost,3sint,t2⟩
- Answer
- ⇀v(t)=−3sintˆi+3costˆj+2tˆk
⇀a(t)=−3costˆi−3sintˆj+2ˆk
Speed(t)=‖⇀v(t)‖=√9+4t2
4) ⇀r(t)=t5ˆi+(3t2+2t−5)ˆj+(3t−1)ˆk
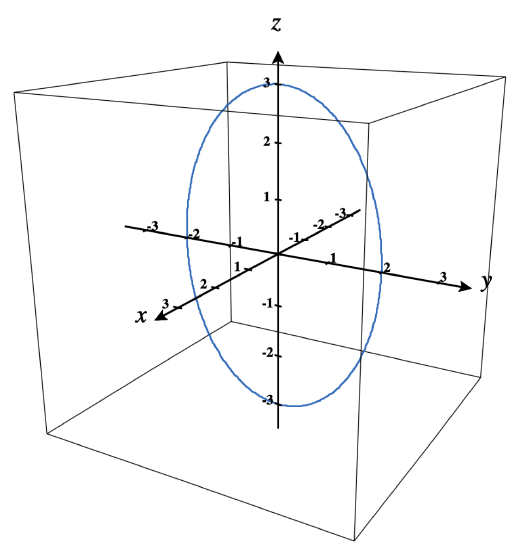
5) ⇀r(t)=2costˆj+3sintˆk. The graph is shown here:
- Answer
- ⇀v(t)=−2sintˆj+3costˆk
⇀a(t)=−2costˆj−3sintˆk
Speed(t)=‖⇀v(t)‖=√4sin2t+9cos2t=√4+5cos2t
In questions 6 - 8, find the velocity, acceleration, and speed of a particle with the given position function.
6) ⇀r(t)=⟨t2−1,t⟩
7) ⇀r(t)=⟨et,e−t⟩
- Answer
- ⇀v(t)=⟨et,−e−t⟩,
⇀a(t)=⟨et,e−t⟩,
‖⇀v(t)‖=√e2t+e−2t
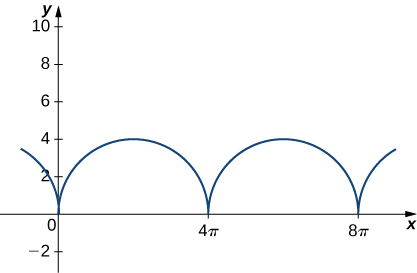
8) ⇀r(t)=⟨sint,t,cost⟩. The graph is shown here:

9) The position function of an object is given by ⇀r(t)=⟨t2,5t,t2−16t⟩. At what time is the speed a minimum?
- Answer
- t=4
10) Let \vecs r(t)=r\cosh(ωt)\,\hat{\mathbf{i}}+r\sinh(ωt)\,\hat{\mathbf{j}}. Find the velocity and acceleration vectors and show that the acceleration is proportional to \vecs r(t).
11) Consider the motion of a point on the circumference of a rolling circle. As the circle rolls, it generates the cycloid \vecs r(t)=(ωt−\sin(ωt))\,\hat{\mathbf{i}}+(1−\cos(ωt))\,\hat{\mathbf{j}}, where \omega is the angular velocity of the circle and b is the radius of the circle:
Find the equations for the velocity, acceleration, and speed of the particle at any time.
- Answer
- \vecs v(t)=(ω−ω\cos(ωt))\,\hat{\mathbf{i}}+(ω\sin(ωt))\,\hat{\mathbf{j}}
\vecs a(t)=(ω^2\sin(ωt))\,\hat{\mathbf{i}}+(ω^2\cos(ωt))\,\hat{\mathbf{j}}
\begin{align*} \text{speed}(t) &= \sqrt{(ω−ω\cos(ωt))^2 + (ω\sin(ωt))^2} \\ &= \sqrt{ω^2 - 2ω^2 \cos(ωt) + ω^2\cos^2(ωt) + ω^2\sin^2(ωt)} \\ &= \sqrt{2ω^2(1 - \cos(ωt))} \end{align*}
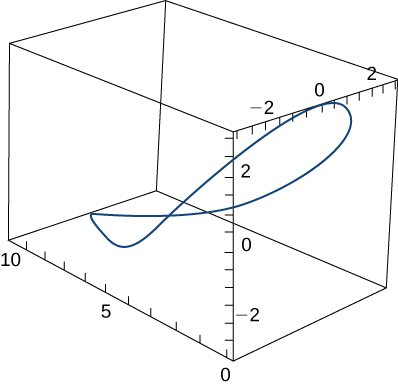
12) A person on a hang glider is spiraling upward as a result of the rapidly rising air on a path having position vector \vecs r(t)=(3\cos t)\,\hat{\mathbf{i}}+(3\sin t)\,\hat{\mathbf{j}}+t^2\,\hat{\mathbf{k}}. The path is similar to that of a helix, although it is not a helix. The graph is shown here:
Find the following quantities:
a. The velocity and acceleration vectors
b. The glider’s speed at any time
- Answer
- \|\vecs v(t)\|=\sqrt{9+4t^2}
c. The times, if any, at which the glider’s acceleration is orthogonal to its velocity
13) Given that \vecs r(t)=⟨e^{−5t}\sin t,\, e^{−5t}\cos t,\, 4e^{−5t}⟩ is the position vector of a moving particle, find the following quantities:
a. The velocity of the particle
- Answer
- \vecs v(t)=⟨e^{−5t}(\cos t−5\sin t),\, −e^{−5t}(\sin t+5\cos t),\, −20e^{−5t}⟩
b. The speed of the particle
c. The acceleration of the particle
- Answer
- \vecs a(t)=⟨e^{−5t}(−\sin t−5\cos t)−5e^{−5t}(\cos t−5\sin t), \; −e^{−5t}(\cos t−5\sin t)+5e^{−5t}(\sin t+5\cos t),\; 100e^{−5t}⟩
14) Find the maximum speed of a point on the circumference of an automobile tire of radius 1 ft when the automobile is traveling at 55 mph.
15) Find the position vector-valued function \vecs r(t), given that \vecs a(t)=\hat{\mathbf{i}}+e^t \,\hat{\mathbf{j}}, \quad \vecs v(0)=2\,\hat{\mathbf{j}}, and \vecs r(0)=2\,\hat{\mathbf{i}}.
16) Find \vecs r(t) given that \vecs a(t)=−32\,\hat{\mathbf{j}}, \vecs v(0)=600\sqrt{3} \,\hat{\mathbf{i}}+600\,\hat{\mathbf{j}}, and \vecs r(0)=\vecs 0.
17) The acceleration of an object is given by \vecs a(t)=t\,\hat{\mathbf{j}}+t\,\hat{\mathbf{k}}. The velocity at t=1 sec is \vecs v(1)=5\,\hat{\mathbf{j}} and the position of the object at t=1 sec is \vecs r(1)=0\,\hat{\mathbf{i}}+0\,\hat{\mathbf{j}}+0\,\hat{\mathbf{k}}. Find the object’s position at any time.
- Answer
- \vecs r(t)=0\,\hat{\mathbf{i}}+\left(\frac{1}{6}t^3+4.5t−\frac{14}{3}\right)\,\hat{\mathbf{j}}+\left(\frac{1}{6}t^3−\frac{1}{2}t+\frac{1}{3}\right)\,\hat{\mathbf{k}}
Projectile Motion
18) A projectile is shot in the air from ground level with an initial velocity of 500 m/sec at an angle of 60° with the horizontal.
a. At what time does the projectile reach maximum height?
- Answer
- 44.185 sec
b. What is the approximate maximum height of the projectile?
c. At what time is the maximum range of the projectile attained?
- Answer
- t=88.37 sec
d. What is the maximum range?
e. What is the total flight time of the projectile?
- Answer
- t=88.37 sec
19) A projectile is fired at a height of 1.5 m above the ground with an initial velocity of 100 m/sec and at an angle of 30° above the horizontal. Use this information to answer the following questions:
a. Determine the maximum height of the projectile.
b. Determine the range of the projectile.
- Answer
- The range is approximately 886.29 m.
20) A golf ball is hit in a horizontal direction off the top edge of a building that is 100 ft tall. How fast must the ball be launched to land 450 ft away?
21) A projectile is fired from ground level at an angle of 8° with the horizontal. The projectile is to have a range of 50 m. Find the minimum velocity (speed) necessary to achieve this range.
- Answer
- v=42.16 m/sec
22) Prove that an object moving in a straight line at a constant speed has an acceleration of zero.
Finding Components of Acceleration & Kepler's Laws
23) Find the tangential and normal components of acceleration for \vecs r(t)=t^2\,\hat{\mathbf{i}}+2t \,\hat{\mathbf{j}} when t=1.
- Answer
- a_\vecs{T}=\sqrt{2}, \quad a_\vecs{N}=\sqrt{2}
In questions 24 - 30, find the tangential and normal components of acceleration.
24) \vecs r(t)=⟨\cos(2t),\,\sin(2t),1⟩
25) \vecs r(t)=⟨e^t \cos t,\,e^t\sin t,\,e^t⟩. The graph is shown here:

- Answer
- a_\vecs{T}=\sqrt{3}e^t, \quad a_\vecs{N}=\sqrt{2}e^t
26) \vecs r(t)=⟨\frac{2}{3}(1+t)^{3/2}, \,\frac{2}{3}(1-t)^{3/2},\,\sqrt{2}t⟩
27) \vecs r(t)=\left\langle 2t,\,t^2,\,\dfrac{t^3}{3}\right\rangle
- Answer
- a_\vecs{T}=2t, \quad a_\vecs{N}=2
28) \vecs r(t)=t^2\,\hat{\mathbf{i}}+t^2\,\hat{\mathbf{j}}+t^3\,\hat{\mathbf{k}}
29) \vecs r(t)=⟨6t,\,3t^2,\,2t^3⟩
- Answer
- a_\vecs{T}=\dfrac{6t +12t^3}{\sqrt{1+t^2+t^4}}, \quad a_\vecs{N}=6\sqrt{\dfrac{1+4t^2+t^4}{1+t^2+t^4}}
30) \vecs r(t)=3\cos(2πt)\,\hat{\mathbf{i}}+3\sin(2πt)\,\hat{\mathbf{j}}
- Answer
- a_\vecs{T}=0, \quad a_\vecs{N}=12\pi^2
31) Find the tangential and normal components of acceleration for \vecs r(t)=a\cos(ωt)\,\hat{\mathbf{i}}+b\sin(ωt)\,\hat{\mathbf{j}} at t=0.
- Answer
- a_\vecs{T}=0, \quad a_\vecs{N}=aω^2
32) Suppose that the position function for an object in three dimensions is given by the equation \vecs r(t)=t\cos(t)\,\hat{\mathbf{i}}+t\sin(t)\,\hat{\mathbf{j}}+3t\,\hat{\mathbf{k}}.
a. Show that the particle moves on a circular cone.
b. Find the angle between the velocity and acceleration vectors when t=1.5.
c. Find the tangential and normal components of acceleration when t=1.5.
- Answer
- c. a_\vecs{T}=0.43\,\text{m/sec}^2, \quad a_\vecs{N}=2.46\,\text{m/sec}^2
33) The force on a particle is given by \vecs f(t)=(\cos t)\,\hat{\mathbf{i}}+(\sin t)\,\hat{\mathbf{j}}. The particle is located at point (c,0) at t=0. The initial velocity of the particle is given by \vecs v(0)=v_0\,\hat{\mathbf{j}}. Find the path of the particle of mass m. (Recall, \vecs F=m\vecs a.)
- Answer
- \vecs r(t)=\left(\dfrac{-\cos t}{m}+c+\frac{1}{m}\right)\,\hat{\mathbf{i}}+\left(\dfrac{−\sin t}{m}+\left(v_0+\frac{1}{m}\right)t\right)\,\hat{\mathbf{j}}
34) An automobile that weighs 2700 lb makes a turn on a flat road while traveling at 56 ft/sec. If the radius of the turn is 70 ft, what is the required frictional force to keep the car from skidding?
35) Using Kepler’s laws, it can be shown that v_0=\sqrt{\dfrac{2GM}{r_0}} is the minimum speed needed when \theta=0 so that an object will escape from the pull of a central force resulting from mass M. Use this result to find the minimum speed when \theta=0 for a space capsule to escape from the gravitational pull of Earth if the probe is at an altitude of 300 km above Earth’s surface.
- Answer
- 10.94 km/sec
36) Find the time in years it takes the dwarf planet Pluto to make one orbit about the Sun given that a=39.5 A.U.
Contributors
Gilbert Strang (MIT) and Edwin “Jed” Herman (Harvey Mudd) with many contributing authors. This content by OpenStax is licensed with a CC-BY-SA-NC 4.0 license. Download for free at http://cnx.org.