8.2E: Fourier Series I (Exercises)

Q8.2.1

1. Prove Theorem 8.1.5.

Q8.2.2

In Exercises 8.2.2-8.2.16 find the Fourier series of \(f\) on \([-L,L]\) and determine its sum for \(-L\leq x\leq L\). Graph \(f\) for Exercises 8.2.2, 8.2.6, 8.2.8, 8.2.15, and 8.2.16 and \[F_{m}(x)=a_{0}+\sum _{n=1}^{m}\left( a_{n}\cos\frac{n\pi x}{L}+b_{n}\sin\frac{n\pi x}{L} \right)\nonumber \] on the same axes for variable values of \(m\).

2. \(L=1\); \(f(x)=2-x\)

3. \(L=\pi\); \(f(x)=2x-3x^2\)

4. \(L=1\); \(f(x)=1-3x^2\)

5. \(L=\pi\); \(f(x)=|\sin x|\)

6. \(L=\pi\); \(f(x)=x\cos x\)

7. \(L=\pi\); \(f(x)=|x|\cos x\)

8. \(L=\pi\); \(f(x)=x\sin x\)

9. \(L=\pi\); \(f(x)=|x|\sin x\)

10. \(L=1\); \(f(x)= \left\{\begin{array}{cl} 0,&-1<x<-{1\over2},\\ \cos\pi x,&-{1\over2}<x<{1\over2},\\ 0,&\phantom{-}{1\over2}<x<1 \end{array}\right.\)

11. \(L=1\); \(f(x)= \left\{\begin{array}{cl} 0,&-1<x<-{1\over2},\\ x\cos\pi x,&-{1\over2}<x<{1\over2},\\ 0,&\phantom{-}{1\over2}<x<1 \end{array}\right.\)

12. \(L=1\); \(f(x)= \left\{\begin{array}{cl} 0,&-1<x<-{1\over2},\\ \sin\pi x,&-{1\over2}<x<{1\over2},\\ 0,&\phantom{-}{1\over2}<x<1 \end{array}\right.\)

13. \(L=1\); \(f(x)= \left\{\begin{array}{cl} 0,&-1<x<-{1\over2},\\ |\sin\pi x|,&-{1\over2}<x<{1\over2},\\ 0,&\phantom{-}{1\over2}<x<1 \end{array}\right.\)

14. \(L=1\); \(f(x)= \left\{\begin{array}{cl} 0,&-1<x<-{1\over2},\\ x\sin\pi x,&-{1\over2}<x<{1\over2},\\ 0,&\phantom{-}{1\over2}<x<1 \end{array}\right.\)

15. \(L=4\); \(f(x)= \left\{\begin{array}{cl} 0,&-4<x<0,\\x,&\phantom{-}0<x<4 \end{array}\right.\)

16. \(L=1\); \(f(x)= \left\{\begin{array}{cl} x^2,&-1< x<0, \\1-x^2,&\phantom{-}0<x<1 \end{array}\right.\)

Q8.2.3

17. Verify the Gibbs phenomenon for \(f(x)= \left\{\begin{array}{rl} 2,&-2< x< -1,\\1,&-1<x<1,\\-1,&\phantom{-}1< x<2. \end{array}\right.\)

18. Verify the Gibbs phenomenon for \(f(x)= \left\{\begin{array}{rl} 2,&-3< x< -2,\\3,&-2<x<2,\\1,&\phantom{-}2< x<3. \end{array}\right.\)

19. Deduce from Example 11.2.5 that

\[\sum_{n=0}^\infty{1\over(2n+1)^2}={\pi^2\over 8}.\nonumber \]

20.

1. Find the Fourier series of \(f(x)=e^x\) on \([-\pi,\pi]\).
2. Deduce from (a) that \[\sum_{n=0}^\infty{1\over n^2+1}={\pi\coth\pi-1\over2}.\nonumber \]

21. Find the Fourier series of \(f(x)=(x-\pi)\cos x\) on \([-\pi,\pi]\).

22. Find the Fourier series of \(f(x)=(x-\pi)\sin x\) on \([-\pi,\pi]\).

23. Find the Fourier series of \(f(x)=\sin kx\) (\(k\ne\) integer) on \([-\pi,\pi]\).

24. Find the Fourier series of \(f(x)=\cos kx\) (\(k\ne\) integer) on \([-\pi,\pi]\).

25.

1. Suppose \(f(-L)=f(L)\), \(f'(-L)=f'(L)\), \(f'\) is continuous, and \(f''\) is piecewise continuous on \([-L,L]\). Use Theorem 8.2.4 and integration by parts to show that \[f(x)=a_0+\sum_{n=1}^\infty\left(a_n\cos{n\pi x\over L}+b_n\sin{n\pi x\over L}\right),\quad -L\le x\le L,\nonumber \] with \[a_0={1\over 2L}\int_{-L}^L f(x)\,dx,\nonumber \] \[a_n= -{L\over n^2\pi^2}\int_{-L}^L f''(x)\cos{n\pi x\over L}\,dx,\quad \text{and} \quad b_n=-{L\over n^2\pi^2}\int_{-L}^L f''(x)\sin{n\pi x\over L}\,dx,\,n\ge1.\nonumber \]
2. Show that if, in addition to the assumptions in (a), \(f''\) is continuous and \(f'''\) is piecewise continuous on \([-L,L]\), then \[a_n={L^2\over n^3\pi^3}\int_{-L}^Lf'''(x)\sin{n\pi x\over L}\,dx.\nonumber \]

26. Show that if \(f\) is integrable on \([-L,L]\) and \[f(x+L)=f(x),\quad -L<x<0\nonumber \] (Figure 8.2.8), then the Fourier series of \(f\) on \([-L,L]\) has the form \[A_0+\sum_{n=1}^\infty\left(A_n\cos{2n\pi\over L}+B_n\sin{2n\pi\over L}\right)\nonumber \] where \[A_0={1\over L}\int_0^Lf(x)\,dx,\nonumber \] and \[A_n={2\over L}\int_0^Lf(x)\cos{2n\pi x\over L}\,dx, \quad B_n={2\over L}\int_0^Lf(x)\sin{2n\pi x\over L}\,dx,\quad n=1,2,3,\dots.\nonumber \]

Figure 8.2.8: \(y=f(x)\), where \(f(x+L)=f(x), -L<x<0\)

Figure 8.2.9: \(y=f(x)\), where \(f(x+L)=-f(x), -L<x<0\)

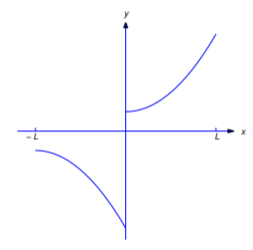
27. Show that if \(f\) is integrable on \([-L,L]\) and

\[f(x+L)=-f(x),\quad -L<x<0\nonumber \]

(Figure 8.2.9), then the Fourier series of \(f\) on \([-L,L]\) has the form

\[\sum_{n=1}^\infty\left(A_n\cos{(2n-1)\pi x\over L}+B_n\sin{(2n-1)\pi x\over L}\right),\nonumber \]

where

\[A_n={2\over L}\int_0^Lf(x)\cos{(2n-1)\pi x\over L}\,dx \quad \text{and} \quad B_n={2\over L}\int_0^Lf(x)\sin{(2n-1)\pi x\over L}\,dx,\quad n=1,2,3,\dots.\nonumber \]