7.2E: Exercises for Section 11.1
- Last updated
- May 24, 2023
- Save as PDF
- Page ID
- 130120
( \newcommand{\kernel}{\mathrm{null}\,}\)
In exercises 1 - 4, sketch the curves below by eliminating the parameter t. Give the orientation of the curve.
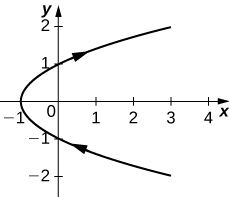
1) x=t2+2t,y=t+1
- Answer
-
Orientation: bottom to top
2) x=cos(t),y=sin(t),for (0,2π]
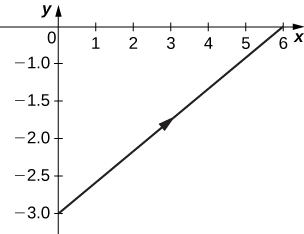
3) x=2t+4,y=t−1
- Answer
-
Orientation: left to right
4) x=3−t,y=2t−3,for 1.5≤t≤3
In exercise 5, eliminate the parameter and sketch the graph.
5) x=2t2,y=t4+1
- Answer
-
y=x24+1

In exercises 6 - 9, use technology (CAS or calculator) to sketch the parametric equations.
6) [T] x=t2+t,y=t2−1
7) [T] x=e−t,y=e2t−1
- Answer
-

8) [T] x=3cost,y=4sint
9) [T] x=sect,y=cost
- Answer
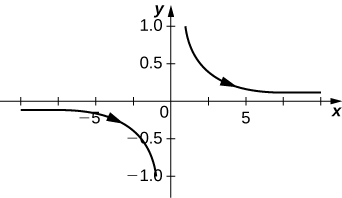
In exercises 10 - 20, sketch the parametric equations by eliminating the parameter. Indicate any asymptotes of the graph.
10) x=et,y=e2t+1
11) x=6sin(2θ),y=−4cos(2θ)
- Answer
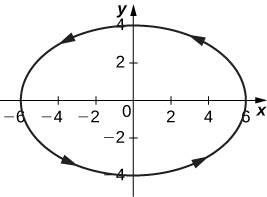
12) x=cosθ,y=2sin(2θ)
13) x=3−2cosθ,y=−5+3sinθ
- Answer

14) x=4+2cosθ,y=−1+sinθ
15) x=sect,y=tant
- Answer
-
Asymptotes are y=x and y=−x

16) x=ln(2t),y=t2
17) x=et,y=e2t
- Answer
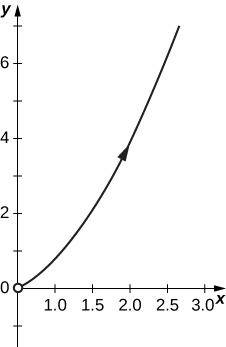
18) x=e−2t,y=e3t
19) x=t3,y=3lnt
- Answer
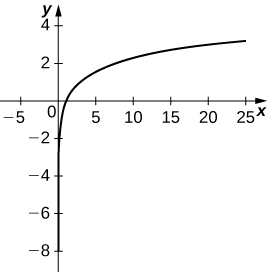
20) x=4secθ,y=3tanθ
In exercises 21 - 38, convert the parametric equations of a curve into rectangular form. No sketch is necessary. State the domain of the rectangular form.
21) x=t2−1,y=t2
- Answer
- x=4y2−1; domain: x∈[1,∞).
22) x=1√t+1,y=t1+t,for t>−1
23) x=4cosθ,y=3sinθ,for t∈(0,2π]
- Answer
- x216+y29=1; domain x∈[−4,4].
24) x=cosht,y=sinht
25) x=2t−3,y=6t−7
- Answer
- y=3x+2; domain: all real numbers.
26) x=t2,y=t3
27) x=1+cost,y=3−sint
- Answer
- (x−1)2+(y−3)2=1; domain: x∈[0,2].
28) x=√t,y=2t+4
29) x=sect,y=tant,for π≤t<3π2
- Answer
- y=√x2−1; domain: x∈(−∞,−1].
30) x=2cosht,y=4sinht
31) x=cos(2t),y=sint
- Answer
- y2=1−x2; domain: x∈[−1,1].
32) x=4t+3,y=16t2−9
33) x=t2,y=2lnt,for t≥1
- Answer
- y=lnx; domain: x∈[1,∞).
34) x=t3,y=3lnt,for t≥1
35) x=tn,y=nlnt,for t≥1, where n is a natural number
- Answer
- y=lnx; domain: x∈(0,∞).
36) x=ln(5t),y=ln(t2) where 1≤t≤e
37) x=2sin(8t),y=2cos(8t)
- Answer
- x2+y2=4; domain: x∈[−2,2].
38) x=tant,y=sec2t−1
In exercises 39 - 48, the pairs of parametric equations represent lines, parabolas, circles, ellipses, or hyperbolas. Name the type of basic curve that each pair of equations represents.
39) x=3t+4,y=5t−2
- Answer
- line
40) x−4=5t,y+2=t
41) x=2t+1,y=t2−3
- Answer
- parabola
42) x=3cost,y=3sint
43) x=2cos(3t),y=2sin(3t)
- Answer
- circle
44) x=cosht,y=sinht
45) x=3cost,y=4sint
- Answer
- ellipse
46) x=2cos(3t),y=5sin(3t)
47) x=3cosh(4t)y=4sinh(4t)
- Answer
- the right branch of a horizontally opening hyperbola
48) x=2cosht,y=2sinht
49) Show that x=h+rcosθ,y=k+rsinθ represents the equation of a circle.
50) Use the equations in the preceding problem to find a set of parametric equations for a circle whose radius is 5 and whose center is (−2,3).
In exercises 51 - 53, use a graphing utility to graph the curve represented by the parametric equations and identify the curve from its equation.
51) [T] x=θ+sinθ,y=1−cosθ
- Answer
-
The equations represent a cycloid.

52) [T] x=2t−2sint,y=2−2cost
53) [T] x=t−0.5sint,y=1−1.5cost
- Answer
54) An airplane traveling horizontally at 100 m/s over flat ground at an elevation of 4000 meters must drop an emergency package on a target on the ground. The trajectory of the package is given by x=100t,y=−4.9t2+4000,where t≥0 where the origin is the point on the ground directly beneath the plane at the moment of release. How many horizontal meters before the target should the package be released in order to hit the target?
55) The trajectory of a bullet is given by x=v0(cosα)t,y=v0(sinα)t−12gt2 where v0=500 m/s, g=9.8=9.8 m/s2, and α=30 degrees. When will the bullet hit the ground? How far from the gun will the bullet hit the ground?
- Answer
- 22,092 meters at approximately 51 seconds.
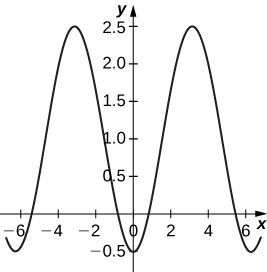
56) [T] Use technology to sketch the curve represented by x=sin(4t),y=sin(3t),for 0≤t≤2π.
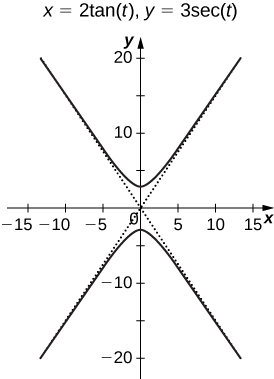
57) [T] Use technology to sketch x=2tan(t),y=3sec(t),for −π<t<π.
- Answer
58) Sketch the curve known as an epitrochoid, which gives the path of a point on a circle of radius b as it rolls on the outside of a circle of radius a. The equations are
x=(a+b)cost−c⋅cos[(a+b)tb],y=(a+b)sint−c⋅sin[(a+b)tb].
Let a=1,b=2,c=1.
59) [T] Use technology to sketch the spiral curve given by x=tcos(t),y=tsin(t) for −2π≤t≤2π.
- Answer
60) [T] Use technology to graph the curve given by the parametric equations x=2cot(t),y=1−cos(2t),for −π/2≤t≤π/2. This curve is known as the witch of Agnesi.
61) [T] Sketch the curve given by parametric equations x=cosh(t),y=sinh(t), for −2≤t≤2.
- Answer


