2.1E: Exercises

Practice Makes Perfect

Verify a Solution of an Equation

In the following exercises, determine whether the given value is a solution to the equation.

Exercise $\PageIndex{1}$

Is $y=\frac{5}{3}$ a solution of
$6 y+10=12 y ?$

Answer

Yes

Exercise $\PageIndex{2}$

Is $x=\frac{9}{4}$ a solution of
$4 x+9=8 x ?$

Exercise $\PageIndex{3}$

Is $u=-\frac{1}{2}$ a solution of
$8 u-1=6 u ?$

Answer

No

Exercise $\PageIndex{4}$

Is $v=-\frac{1}{3}$ a solution of
$9 v-2=3 v ?$

Solve Equations using the Subtraction and Addition Properties of Equality

In the following exercises, solve each equation using the Subtraction and Addition Properties of Equality.

Exercise $\PageIndex{5}$

$x+24=35$

Answer

x = 11

Exercise $\PageIndex{6}$

$x+17=22$

Exercise $\PageIndex{7}$

$y+45=-66$

Answer

y = -111

Exercise $\PageIndex{8}$

$y+39=-83$

Exercise $\PageIndex{9}$

$b+\frac{1}{4}=\frac{3}{4}$

Answer

$b = \frac{1}{2}$

Exercise $\PageIndex{10}$

$a+\frac{2}{5}=\frac{4}{5}$

Exercise $\PageIndex{11}$

$p+2.4=-9.3$

Answer

p = -11.7

Exercise $\PageIndex{12}$

$m+7.9=11.6$

Exercise $\PageIndex{13}$

$a-45=76$

Answer

a = 121

Exercise $\PageIndex{14}$

$a-30=57$

Exercise $\PageIndex{15}$

$m-18=-200$

Answer

m = -182

Exercise $\PageIndex{16}$

$m-12=-12$

Exercise $\PageIndex{17}$

$x-\frac{1}{3}=2$

Answer

$x=\frac{7}{3}$

Exercise $\PageIndex{18}$

$x-\frac{1}{5}=4$

Exercise $\PageIndex{19}$

$y-3.8=10$

Answer

y = 10.8

Exercise $\PageIndex{20}$

$y-7.2=5$

Exercise $\PageIndex{21}$

$x-165=-420$

Answer

$x=-255$

Exercise $\PageIndex{22}$

$z-101=-314$

Exercise $\PageIndex{23}$

$z+0.52=-8.5$

Answer

$z=-9.02$

Exercise $\PageIndex{24}$

$x+0.93=-4.1$

Exercise $\PageIndex{25}$

$q+\frac{3}{4}=\frac{1}{2}$

Answer

$q = -\frac{1}{4}$

Exercise $\PageIndex{26}$

$p+\frac{1}{3}=\frac{5}{6}$

Exercise $\PageIndex{27}$

$p-\frac{2}{5}=\frac{2}{3}$

Answer

$p=\frac{16}{15}$

Exercise $\PageIndex{28}$

$y-\frac{3}{4}=\frac{3}{5}$

Solve Equations that Require Simplification

In the following exercises, solve each equation.

Exercise $\PageIndex{29}$

$c+31-10=46$

Answer

c = 25

Exercise $\PageIndex{30}$

$m+16-28=5$

Exercise $\PageIndex{31}$

$9 x+5-8 x+14=20$

Answer

x = 1

Exercise $\PageIndex{32}$

$6 x+8-5 x+16=32$

Exercise $\PageIndex{33}$

$-6 x-11+7 x-5=-16$

Answer

x = 0

Exercise $\PageIndex{34}$

$-8 n-17+9 n-4=-41$

Exercise $\PageIndex{35}$

$5(y-6)-4 y=-6$

Answer

$y=24$

Exercise $\PageIndex{36}$

$9(y-2)-8 y=-16$

Exercise $\PageIndex{37}$

$8(u+1.5)-7 u=4.9$

Answer

$u=-7.1$

Exercise $\PageIndex{38}$

$5(w+2.2)-4 w=9.3$

Exercise $\PageIndex{39}$

$6 a-5(a-2)+9=-11$

Answer

$a=-30$

Exercise $\PageIndex{40}$

$8 c-7(c-3)+4=-16$

Exercise $\PageIndex{41}$

$6(y-2)-5y=4(y+3) -4(y-1)$

Answer

y =28

Exercise $\PageIndex{42}$

$9(x-1)-8 x=-3(x+5)+3(x-5)$

Exercise $\PageIndex{43}$

$3(5 n-1)-14 n+9=10(n-4)-6n-4(n+1)$

Answer

n = -50

Exercise $\PageIndex{44}$

$2(8m+3)-15m-4=9(m+6)-2(m-1)-7m$

Exercise $\PageIndex{45}$

$-(j+2)+2 j-1=5$

Answer

j = 8

Exercise $\PageIndex{46}$

$-(k+7)+2 k+8=7$

Exercise $\PageIndex{47}$

$-\left(\frac{1}{4} a-\frac{3}{4}\right)+\frac{5}{4} a=-2$

Answer

$a=-\frac{11}{4}$

Exercise $\PageIndex{48}$

$-\left(\frac{2}{3} d-\frac{1}{3}\right)+\frac{5}{3} d=-4$

Exercise $\PageIndex{49}$

$\begin{array}{l}{8(4 x+5)-5(6 x)-x} \\ {=53-6(x+1)+3(2 x+2)}\end{array}$

Answer

x=13

Exercise $\PageIndex{50}$

$\begin{array}{l}{6(9 y-1)-10(5 y)-3 y} \\ {=22-4(2 y-12)+8(y-6)}\end{array}$

Translate to an Equation and Solve

In the following exercises, translate to an equation and then solve it.

Exercise $\PageIndex{51}$

Nine more than $x$ is equal to $52 .$

Answer

$x+9=52 ; x=43$

Exercise $\PageIndex{52}$

The sum of $x$ and $-15$ is 23.

Exercise $\PageIndex{53}$

Ten less than $m$ is $-14$.

Answer

$m-10=-14 ; m=-4$

Exercise $\PageIndex{54}$

Three less than $y$ is $-19$.

Exercise $\PageIndex{55}$

The sum of $y$ and $-30$ is $40 .$

Answer

$y+(-30)=40 ; y=70$

Exercise $\PageIndex{56}$

Twelve more than $p$ is equal to $67 .$

Exercise $\PageIndex{57}$

The difference of 9$x$ and 8$x$ is 107.

Answer

$9 x-8 x=107 ; 107$

Exercise $\PageIndex{58}$

The difference of 5$c$ and 4$c$ is $602 .$

Exercise $\PageIndex{59}$

The difference of 5$c$ and 4$c$ is 602

Answer

$n-\frac{1}{6}=\frac{1}{2} ; \frac{2}{3}$

Exercise $\PageIndex{60}$

The difference of $f$ and $\frac{1}{3}$ is $\frac{1}{12}$.

Exercise $\PageIndex{61}$

The sum of $-4 n$ and 5$n$ is $-82$

Answer

$-4 n+5 n=-82 ;-82$

Exercise $\PageIndex{62}$

The sum of $-9 m$ and 10$m$ is $-95$

Translate and Solve Applications

In the following exercises, translate into an equation and solve.

Exercise $\PageIndex{63}$

Distance Avril rode her bike a total of 18 miles, from home to the library and then to the beach. The distance from Avril’s house to the library is 7 miles. What is the distance from the library to the beach?

Answer

11 miles

Exercise $\PageIndex{64}$

Reading Jeff read a total of 54 pages in his History and Sociology textbooks. He read 41 pages in his History textbook. How many pages did he read in his Sociology textbook?

Exercise $\PageIndex{65}$

Age Eva’s daughter is 15 years younger than her son. Eva’s son is 22 years old. How old is her daughter?

Answer

7 years old

Exercise $\PageIndex{66}$

Age Pablo’s father is 3 years older than his mother. Pablo’s mother is 42 years old. How old is his father?

Exercise $\PageIndex{67}$

Groceries For a family birthday dinner, Celeste bought a turkey that weighed 5 pounds less than the one she bought for Thanksgiving. The birthday turkey weighed 16 pounds. How much did the Thanksgiving turkey weigh?

Answer

21 pounds

Exercise $\PageIndex{68}$

Weight Allie weighs 8 pounds less than her twin sister Lorrie. Allie weighs 124 pounds. How much does Lorrie weigh?

Exercise $\PageIndex{69}$

Health Connor’s temperature was 0.7 degrees higher this morning than it had been last night. His temperature this morning was 101.2 degrees. What was his temperature last night?

Answer

100.5 degrees

Exercise $\PageIndex{70}$

Health The nurse reported that Tricia’s daughter had gained 4.2 pounds since her last checkup and now weighs 31.6 pounds. How much did Tricia’s daughter weigh at her last checkup?

Exercise $\PageIndex{71}$

Salary Ron’s paycheck this week was $17.43 less than his paycheck last week. His paycheck this week was $103.76. How much was Ron’s paycheck last week?

Answer

$121.19

Exercise $\PageIndex{72}$

Textbooks Melissa’s math book cost $22.85 less than her art book cost. Her math book cost $93.75. How much did her art book cost?

Everyday Math

Exercise $\PageIndex{73}$

Construction Miguel wants to drill a hole for a $\frac{5}{8}$ inch screw. The hole should be $\frac{1}{12}$ inch smaller than the screw. Let $d$ equal the size of the hole he should drill. Solve the equation $d=\frac{5}{8}-\frac{1}{12}$ to see what size the hole should be.

Answer

$d=\frac{13}{24}$ inch

Exercise $\PageIndex{74}$

Baking Kelsey needs $\frac{2}{3}$ cup of sugar for the cookie recipe she wants to make. She only has $\frac{3}{8}$ cup of sugar and will borrow
the rest from her ner neighbor. Let $s$ equal the amount of sugar she will borrow. Solve the equation $\frac{3}{8}+s=\frac{2}{3}$ to find the
amount of sugar she should ask to borrow.

Writing Exercises

Exercise $\PageIndex{75}$

Is $-8$ a solution to the equation $3 x=16-5 x ?$ How do you know?

Answer

No. Justifications will vary.

Exercise $\PageIndex{76}$

What is the first step in your solution to the equation $10 x+2=4 x+26 ?$

Self Check

ⓐ After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.

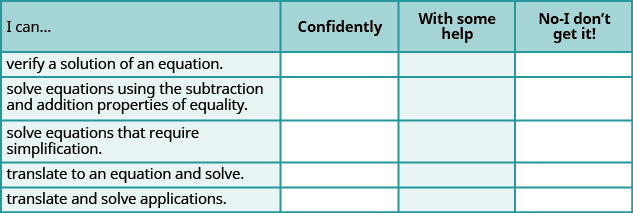
ⓑ If most of your checks were:

…confidently. Congratulations! You have achieved your goals in this section! Reflect on the study skills you used so that you can continue to use them. What did you do to become confident of your ability to do these things? Be specific!

…with some help. This must be addressed quickly as topics you do not master become potholes in your road to success. Math is sequential - every topic builds upon previous work. It is important to make sure you have a strong foundation before you move on. Who can you ask for help? Your fellow classmates and instructor are good resources. Is there a place on campus where math tutors are available? Can your study skills be improved?

…no - I don’t get it! This is critical and you must not ignore it. You need to get help immediately or you will quickly be overwhelmed. See your instructor as soon as possible to discuss your situation. Together you can come up with a plan to get you the help you need.