1.5E: Exercises

Last updated
Save as PDF

Page ID: 18543

This page is a draft and is under active development.

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$ \newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\id}{\mathrm{id}}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\kernel}{\mathrm{null}\,}$

$ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$

$ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$

$ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\AA}{\unicode[.8,0]{x212B}}$

$ \newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$ \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$ \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vectorC}[1]{\textbf{#1}} $

$ \newcommand{\vectorD}[1]{\overrightarrow{#1}} $

$ \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} $

$ \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} $

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$\newcommand{\avec}{\mathbf a}$ $\newcommand{\bvec}{\mathbf b}$ $\newcommand{\cvec}{\mathbf c}$ $\newcommand{\dvec}{\mathbf d}$ $\newcommand{\dtil}{\widetilde{\mathbf d}}$ $\newcommand{\evec}{\mathbf e}$ $\newcommand{\fvec}{\mathbf f}$ $\newcommand{\nvec}{\mathbf n}$ $\newcommand{\pvec}{\mathbf p}$ $\newcommand{\qvec}{\mathbf q}$ $\newcommand{\svec}{\mathbf s}$ $\newcommand{\tvec}{\mathbf t}$ $\newcommand{\uvec}{\mathbf u}$ $\newcommand{\vvec}{\mathbf v}$ $\newcommand{\wvec}{\mathbf w}$ $\newcommand{\xvec}{\mathbf x}$ $\newcommand{\yvec}{\mathbf y}$ $\newcommand{\zvec}{\mathbf z}$ $\newcommand{\rvec}{\mathbf r}$ $\newcommand{\mvec}{\mathbf m}$ $\newcommand{\zerovec}{\mathbf 0}$ $\newcommand{\onevec}{\mathbf 1}$ $\newcommand{\real}{\mathbb R}$ $\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$ $\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$ $\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$ $\newcommand{\laspan}[1]{\text{Span}\{#1\}}$ $\newcommand{\bcal}{\cal B}$ $\newcommand{\ccal}{\cal C}$ $\newcommand{\scal}{\cal S}$ $\newcommand{\wcal}{\cal W}$ $\newcommand{\ecal}{\cal E}$ $\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$ $\newcommand{\gray}[1]{\color{gray}{#1}}$ $\newcommand{\lgray}[1]{\color{lightgray}{#1}}$ $\newcommand{\rank}{\operatorname{rank}}$ $\newcommand{\row}{\text{Row}}$ $\newcommand{\col}{\text{Col}}$ $\renewcommand{\row}{\text{Row}}$ $\newcommand{\nul}{\text{Nul}}$ $\newcommand{\var}{\text{Var}}$ $\newcommand{\corr}{\text{corr}}$ $\newcommand{\len}[1]{\left|#1\right|}$ $\newcommand{\bbar}{\overline{\bvec}}$ $\newcommand{\bhat}{\widehat{\bvec}}$ $\newcommand{\bperp}{\bvec^\perp}$ $\newcommand{\xhat}{\widehat{\xvec}}$ $\newcommand{\vhat}{\widehat{\vvec}}$ $\newcommand{\uhat}{\widehat{\uvec}}$ $\newcommand{\what}{\widehat{\wvec}}$ $\newcommand{\Sighat}{\widehat{\Sigma}}$ $\newcommand{\lt}{<}$ $\newcommand{\gt}{>}$ $\newcommand{\amp}{&}$ $\definecolor{fillinmathshade}{gray}{0.9}$

Moments and Centers of Mass

For the following exercises, calculate the center of mass for the collection of masses given.

Exercise $\PageIndex{1}$

$\displaystyle m_1=2$ at $\displaystyle x_1=1$ and $\displaystyle m_2=4$ at $\displaystyle x_2=2$

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{2}$

$\displaystyle m_1=1$ at $\displaystyle x_1=−1$ and $\displaystyle m_2=3$ at $\displaystyle x_2=2$

Answer: $\displaystyle \frac{5}{4}$

Exercise $\PageIndex{3}$

$\displaystyle m=3$ at $\displaystyle x=0,1,2,6$

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{4}$

Unit masses at $\displaystyle (x,y)=(1,0),(0,1),(1,1)$

Answer: $\displaystyle (\frac{2}{3},\frac{2}{3})$

Exercise $\PageIndex{5}$

$\displaystyle m_1=1$ at $\displaystyle (1,0)$ and $\displaystyle m_2=4$ at $\displaystyle (0,1)$

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{6}$

$\displaystyle m_1=1$ at $\displaystyle (1,0)$ and $\displaystyle m_2=3$ at $\displaystyle (2,2)$

Answer: $\displaystyle (\frac{7}{4},\frac{3}{2})$

For the following exercises, compute the center of mass x–.

Exercise $\PageIndex{7}$

$\displaystyle ρ=1$ for $\displaystyle x∈(−1,3)$

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{8}$

$\displaystyle ρ=x^2$ for $\displaystyle x∈(0,L)$

Answer: $\displaystyle \frac{3L}{4}$

Exercise $\PageIndex{9}$

$\displaystyle ρ=1$ for $\displaystyle x∈(0,1)$ and $\displaystyle ρ=2$ for $\displaystyle x∈(1,2)$

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{10}$

$\displaystyle ρ=sinx$ for $\displaystyle x∈(0,π)$

Answer: $\displaystyle \frac{π}{2}$

Exercise $\PageIndex{11}$

$\displaystyle ρ=cosx$ for $\displaystyle x∈(0,\frac{π}{2})$

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{12}$

$\displaystyle ρ=e^x$ for $\displaystyle x∈(0,2)$

Answer: $\displaystyle \frac{e^2+1}{e^2−1}$

Exercise $\PageIndex{13}$

$\displaystyle ρ=x^3+xe^{−x}$ for $\displaystyle x∈(0,1)$

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{14}$

$\displaystyle ρ=xsinx$ for $\displaystyle x∈(0,π)$

Answer: $\displaystyle \frac{π^2−4}{π}$

Exercise $\PageIndex{15}$

$\displaystyle ρ=\sqrt{x}$ for $\displaystyle x∈(1,4)$

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{16}$

$\displaystyle ρ=lnx$ for $\displaystyle x∈(1,e)$

Answer: $\displaystyle \frac{1}{4}(1+e^2)$

For the following exercises, compute the center of mass $\displaystyle (\bar{x},\bar{y})$. Use symmetry to help locate the center of mass whenever possible.

Exercise $\PageIndex{17}$

$\displaystyle ρ=7$ in the square $\displaystyle 0≤x≤1, 0≤y≤1$

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{18}$

$\displaystyle ρ=3$ in the triangle with vertices $\displaystyle (0,0), (a,0)$, and $\displaystyle (0,b)$

Answer: $\displaystyle (\frac{a}{3},\frac{b}{3})$

Exercise $\PageIndex{19}$

$\displaystyle ρ=2$ for the region bounded by $\displaystyle y=cos(x), y=−cos(x), x=−\frac{π}{2}$, and $\displaystyle x=\frac{π}{2}$

Answer: Add texts here. Do not delete this text first.

For the following exercises, use a calculator to draw the region, then compute the center of mass $\displaystyle (\bar{x},\bar{y})$. Use symmetry to help locate the center of mass whenever possible.

Exercise $\PageIndex{20}$

The region bounded by $\displaystyle y=cos(2x), x=−\frac{π}{4}$, and $\displaystyle x=\frac{π}{4}$

Answer: $\displaystyle (0,\frac{π}{8})$

Exercise $\PageIndex{21}$

The region between $\displaystyle y=2x^2, y=0, x=0,$ and $\displaystyle x=1$

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{22}$

The region between $\displaystyle y=\frac{5}{4}x^2$ and $\displaystyle y=5$

Answer: $\displaystyle (0,3)$

Exercise $\PageIndex{23}$

Region between $\displaystyle y=\sqrt{x}, y=ln(x), x=1,$ and $\displaystyle x=4$

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{24}$

The region bounded by $\displaystyle y=0, \frac{x^2}{4}+\frac{y^2}{9}=1$

Answer: $\displaystyle (0,\frac{4}{π})$

Exercise $\PageIndex{25}$

The region bounded by $\displaystyle y=0, x=0,$ and $\displaystyle \frac{x^2}{4}+\frac{y^2}{9}=1$

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{26}$

The region bounded by $\displaystyle y=x^2$ and $\displaystyle y=x^4$ in the first quadrant

Answer: $\displaystyle (\frac{5}{8},\frac{1}{3})$

For the following exercises, use the theorem of Pappus to determine the volume of the shape.

Exercise $\PageIndex{27}$

Rotating $\displaystyle y=mx$ around the $\displaystyle x$-axis between $\displaystyle x=0$ and $\displaystyle x=1$

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{28}$

Rotating $\displaystyle y=mx$ around the $\displaystyle y$-axis between $\displaystyle x=0$ and $\displaystyle x=1$

Answer: $\displaystyle \frac{mπ}{3}$

Exercise $\PageIndex{29}$

A general cone created by rotating a triangle with vertices $\displaystyle (0,0), (a,0),$ and $\displaystyle (0,b)$ around the $\displaystyle y$-axis. Does your answer agree with the volume of a cone?

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{30}$

A general cylinder created by rotating a rectangle with vertices $\displaystyle (0,0), (a,0),(0,b),$ and $\displaystyle (a,b)$ around the $\displaystyle y$ -axis. Does your answer agree with the volume of a cylinder?

Answer: $\displaystyle πa^2b$

Exercise $\PageIndex{31}$

A sphere created by rotating a semicircle with radius $\displaystyle a$ around the $\displaystyle y$-axis. Does your answer agree with the volume of a sphere?

Answer: Add texts here. Do not delete this text first.

For the following exercises, use a calculator to draw the region enclosed by the curve. Find the area $\displaystyle M$ and the centroid $\displaystyle (\bar{x},\bar{y})$ for the given shapes. Use symmetry to help locate the center of mass whenever possible.

Exercise $\PageIndex{32}$

Quarter-circle: $\displaystyle y=\sqrt{1−x^2}, y=0$, and $\displaystyle x=0$

Answer: $\displaystyle (\frac{4}{3π},\frac{4}{3π})$

Exercise $\PageIndex{33}$

Triangle: $\displaystyle y=x, y=2−x$, and $\displaystyle y=0$

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{34}$

Lens: $\displaystyle y=x^2$ and $\displaystyle y=x$

Answer: $\displaystyle (\frac{1}{2},\frac{2}{5})$

Exercise $\PageIndex{35}$

Ring: $\displaystyle y^2+x^2=1$ and $\displaystyle y^2+x^2=4$

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{36}$

Half-ring: $\displaystyle y^2+x^2=1, y^2+x^2=4,$ and $\displaystyle y=0$

Answer: $\displaystyle (0,\frac{28}{9π})$

Exercise $\PageIndex{37}$

Find the generalized center of mass in the sliver between $\displaystyle y=x^a$ and $\displaystyle y=x^b$ with $\displaystyle a>b$. Then, use the Pappus theorem to find the volume of the solid generated when revolving around the y-axis.

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{38}$

Find the generalized center of mass between $\displaystyle y=a^2−x^2, x=0$, and $\displaystyle y=0$. Then, use the Pappus theorem to find the volume of the solid generated when revolving around the y-axis.

Answer: Center of mass: $\displaystyle (\frac{a}{6},\frac{4a^2}{5}),$ volume: $\displaystyle \frac{2πa^4}{9}$

Exercise $\PageIndex{39}$

Find the generalized center of mass between $\displaystyle y=bsin(ax), x=0,$ and $\displaystyle x=\frac{π}{a}.$ Then, use the Pappus theorem to find the volume of the solid generated when revolving around the y-axis.

Answer: Add texts here. Do not delete this text first.

Exercise $\PageIndex{40}$

Use the theorem of Pappus to find the volume of a torus (pictured here). Assume that a disk of radius $\displaystyle a$ is positioned with the left end of the circle at $\displaystyle x=b, b>0,$ and is rotated around the y-axis.

$alt$

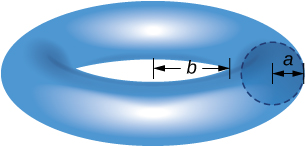
Answer: Find the center of mass $\displaystyle (\bar{x},\bar{y})$ for a thin wire along the semicircle $\displaystyle y=\sqrt{1−x^2}$ with unit mass. (Hint: Use the theorem of Pappus.)

Search

Text Color

Text Size

Margin Size

Font Type