9.1E: Exercises
( \newcommand{\kernel}{\mathrm{null}\,}\)
Exercise 9.1E.1
For the following exercises, determine whether the statement is true or false.
1. The domain of vector field ⇀F=⇀F(x,y) is a set of points (x,y) in a plane, and the range of F is a set of vectors in the plane.
- Answer
-
True.
2. Vector field ⇀F=⟨3x2,1⟩ is a gradient field for both ϕ1(x,y)=x3+y and ϕ2(x,y)=y+x3+100.
3. Vector field ⇀F=〈y,x〉√x2+y2 is constant in direction and magnitude on a unit circle
- Answer
-
False.
4. Vector field ⇀F=〈y,x〉√x2+y2 is neither a radial field nor a rotation.
Exercise 9.1E.2
For the following exercises, describe each vector field by drawing some of its vectors.
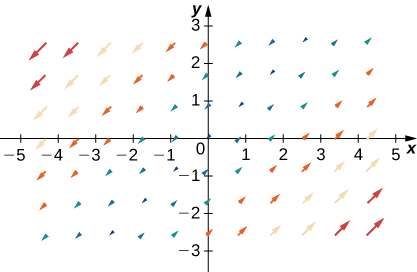
5. [T] ⇀F(x,y)=xˆi+yˆj
- Answer
-
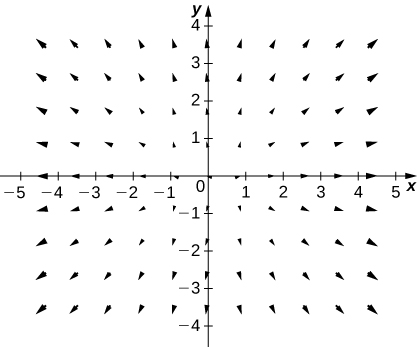
6. [T] ⇀F(x,y)=−yˆi+xˆj
7. [T] ⇀F(x,y)=xˆi−yˆj
- Answer
-
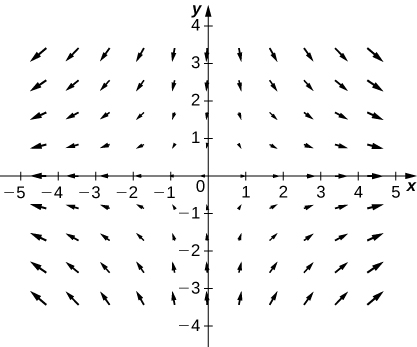
8. [T] ⇀F(x,y)=ˆi+ˆj
9. [T] ⇀F(x,y)=2xˆi+3yˆj
- Answer
-
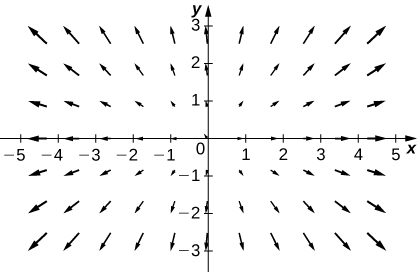
10. [T] ⇀F(x,y)=3ˆi+xˆj
11. [T] ⇀F(x,y)=yˆi+sinxˆj
- Answer
-
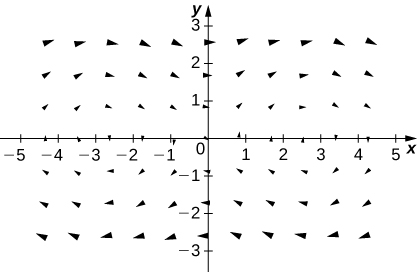
12. [T] ⇀F(x,y,z)=xˆi+yˆj+zˆk
13. [T] ⇀F(x,y,z)=2xˆi−2yˆj−2zˆk
- Answer
-

14. [T] ⇀F(x,y,z)=yzˆi−xzˆj
Exercise 9.1E.3
For the following exercises, find the gradient vector field ⇀F of each function f.
15. f(x,y)=xsin(y)+cos(y)
- Answer
-
⇀F(x,y)=sin(y)ˆi+(xcos(y)−sin(y))ˆj
16. f(x,y,z)=ze−xy
17. f(x,y,z)=x2y+xy+y2z
- Answer
-
⇀F(x,y,z)=(2xy+y)ˆi+(x2+x+2yz)ˆj+y2ˆk
18. f(x,y)=x2sin(5y)
19. f(x,y)=ln(1+x2+2y2)
- Answer
-
⇀F(x,y)=(2x1+x2+2y2)ˆi+(4y1+x2+2y2)ˆj
20. f(x,y,z)=xcos(yz)
21. What is vector field ⇀F(x,y) with a value at (x,y) that is of unit length and points toward (1,0)?
- Answer
-
⇀F(x,y)=(1−x)ˆi−yˆj√(1−x)2+y2
Exercise 9.1E.4
For the following exercises, write formulas for the vector fields with the given properties.
22. All vectors are parallel to the x-axis and all vectors on a vertical line have the same magnitude.
23. All vectors point toward the origin and have a constant length.
Solution: ⇀F(x,y)=(yˆi−xˆj)√x2+y2
24. All vectors are of unit length and are perpendicular to the position vector at that point.
25. Give a formula ⇀F(x,y)=M(x,y)ˆi+N(x,y)ˆj for the vector field in a plane that has the properties that ⇀F=0 at (0,0) and that at any other point (a,b),⇀F is tangent to circle x2+y2=a2+b2 and points in the clockwise direction with magnitude |⇀F|=√a2+b2.
Solution: ⇀F(x,y)=yˆi−xˆj
26. Is vector field ⇀F(x,y)=(P(x,y),Q(x,y))=(sinx+y)ˆi+(cosy+x)ˆj a gradient field?
27. Find a formula for vector field ⇀F(x,y)=M(x,y)ˆi+N(x,y)ˆj given the fact that for all points (x,y), ⇀F points toward the origin and |⇀F|=10x2+y2.
- Answer
-
⇀F(x,y)=−10(x2+y2)3/2(xˆi+yˆj)
Exercise 9.1E.5
For the following exercises, assume that an electric field in the xy-plane caused by an infinite line of charge along the x-axis is a gradient field with potential function V(x,y)=cln(r0√x2+y2), where c>0 is a constant and r0 is a reference distance at which the potential is assumed to be zero.
28. Find the components of the electric field in the x- and y-directions, where ⇀E(x,y)=−∇V(x,y).
29. Show that the electric field at a point in the xy-plane is directed outward from the origin and has magnitude |⇀E∣=cr, where r=√x2=y2.
- Answer
-
⇀E=c|r|2r=c|r|r|r|
Exercise 9.1E.6
A flow line (or streamline) of a vector field\vecs{F}is a curve r(t) such that \(dr/dt=\vecs{F}(r(t))\). If ⇀F represents the velocity field of a moving particle, then the flow lines are paths taken by the particle. Therefore, flow lines are tangent to the vector field. For the following exercises, show that the given curve \(c(t)\) is a flow line of the given velocity vector field ⇀F(x,y,z).
30. c(t)=(e2t,ln|t|,1t),t≠0;⇀F(x,y,z)=⟨2x,z,−z2⟩
31. c(t)=(sint,cost,et);⇀F(x,y,z)=〈y,−x,z〉
- Answer
-
\(c′(t)=(cost,−sint,e^{−t})=\vecs{F}(c(t))\)
Exercise 9.1E.7
For the following exercises, let ⇀F=xˆi+yˆj,⇀G=−yˆi+xˆj, and ⇀H=xˆi−yˆj. Match ⇀F,⇀G, and ⇀H with their graphs.
32.

33.
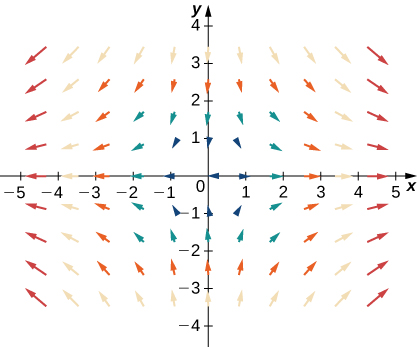
- Answer
-
⇀H
34.
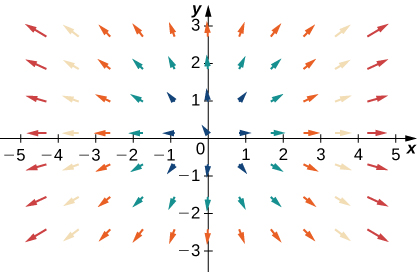
- Answer
-
Add texts here. Do not delete this text first.
Exercise 9.1E.8
For the following exercises, let ⇀F=xˆi+yˆj,⇀G=−yˆi+xˆj,and⇀H=−xˆi+yˆj. Match the vector fields with their graphs in (I)−(IV).
- ⇀F+⇀G
- ⇀F+⇀H
- ⇀H+⇀G
- ⇀−F+⇀G
35.
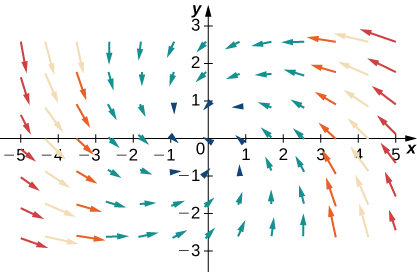
- Answer
-
⇀−F+⇀G
36.
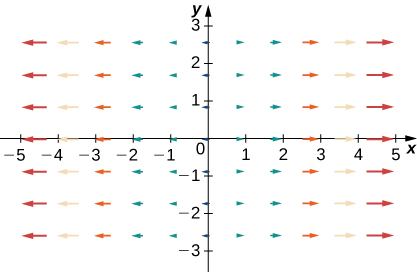
37.
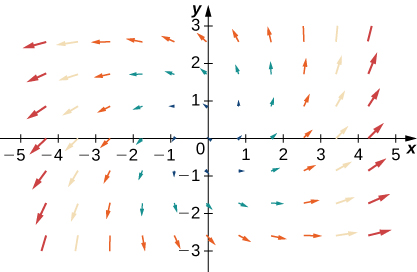
- Answer
-
⇀F+⇀G
38.