The flight path of a commercial airliner is carefully regulated by the Federal Aviation Administration. Each airplane must maintain a distance of five miles from another plane flying at the same altitude and 2,000 feet above and below another aircraft (1,000 feet if the altitude is less than 29,000 feet). So, each aircraft only has certain positions it is allowed to maintain while it flies. As we explore quantum mechanics, we see that electrons have similar restrictions on their locations.
Orbitals
We can apply our knowledge of quantum numbers to describe the arrangement of electrons for a given atom. We do this with something called electron configurations. They are effectively a map of the electrons for a given atom. We look at the four quantum numbers for a given electron and then assign that electron to a specific orbital below.
s Orbitals
For any value of \(n\), a value of \(l=0\) places that electron in an \(s\) orbital. This orbital is spherical in shape:
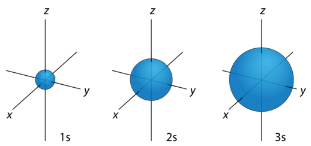
Figure \(\PageIndex{1}\): \(s\) orbitals have no orientational preference and resemble spheres.
p Orbitals
For the table below, we see that we can have three possible orbitals when \(l=1\). These are designated as \(p\) orbitals and have dumbbell shapes. Each of the \(p\) orbitals has a different orientation in three-dimensional space.
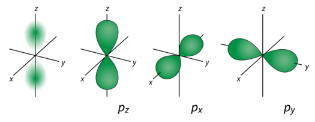
Figure \(\PageIndex{2}\): \(p\) orbitals have an orientational preference and resemble dumbbells.
d Orbitals
When \(l=2\), \(m_l\) values can be \(-2, \: -1, \: 0, \: +1, \: +2\) for a total of five \(d\) orbitals. Note that all five of the orbitals have specific three-dimensional orientations.
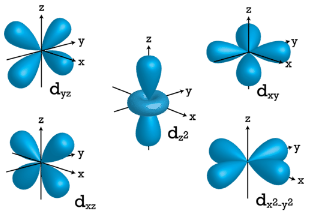
Figure \(\PageIndex{3}\): \(d\) orbitals have an orientational preference and exhibit complex structures.
f Orbitals
The most complex set of orbitals are the \(f\) orbitals. When \(l=3\), \(m_l\) values can be \(-3, \: -2, \: -1, \: 0, \: +1, \: +2, \: +3\) for a total of seven different orbital shapes. Again, note the specific orientations of the different \(f\) orbitals.

Figure \(\PageIndex{4}\): \(f\) orbitals have an orientational preference and exhibit quite complex structures.
Orbitals that have the same value of the principal quantum number form a shell. Orbitals within a shell are divided into subshells that have the same value of the angular quantum number. Some of the allowed combinations of quantum numbers are compared in Table \(\PageIndex{1}\).
Table \(\PageIndex{1}\): Electron Arrangement Within Energy Levels
| Principal Quantum Number \(\left( n \right)\) |
Allowable Sublevels |
Number of Orbitals per Sublevel |
Number of Orbitals per Principal Energy Level |
Number of Electrons per Sublevel |
Number of Electrons per Principal Energy Level |
| 1 |
\(s\) |
1 |
1 |
2 |
2 |
| 2 |
\(s\) |
1 |
4 |
2 |
8 |
| \(p\) |
3 |
6 |
| 3 |
\(s\) |
1 |
9 |
2 |
18 |
| \(p\) |
3 |
6 |
| \(d\) |
5 |
10 |
| 4 |
\(s\) |
1 |
16 |
2 |
32 |
| \(p\) |
3 |
6 |
| \(d\) |
5 |
10 |
| \(f\) |
7 |
14 |
Electron Configurations
Can you name one thing that easily distinguishes you from the rest of the world? And we're not talking about DNA—that's a little expensive to sequence. For many people, it is their email address. Your email address allows people all over the world to contact you. It does not belong to anyone else, but serves to identify you. Electrons also have a unique set of identifiers in the quantum numbers that describe their location and spin. Chemists use an electronic configuration to represent the organization of electrons in shells and subshells in an atom. An electron configuration simply lists the shell and subshell labels, with a right superscript giving the number of electrons in that subshell. The shells and subshells are listed in the order of filling. Electrons are typically organized around an atom by starting at the lowest possible quantum numbers first, which are the shells-subshells with lower energies.
For example, an H atom has a single electron in the 1s subshell. Its electron configuration is
\[\ce{H}:\, 1s^1 \nonumber \]
He has two electrons in the 1s subshell. Its electron configuration is
\[\ce{He}:\, 1s^2 \nonumber \]
The three electrons for Li are arranged in the 1s subshell (two electrons) and the 2s subshell (one electron). The electron configuration of Li is
\[\ce{Li}:\, 1s^22s^1 \nonumber \]
Be has four electrons, two in the 1s subshell and two in the 2s subshell. Its electron configuration is
\[\ce{Be}:\, 1s^22s^2 \nonumber \]
Now that the 2s subshell is filled, electrons in larger atoms must go into the 2p subshell, which can hold a maximum of six electrons. The next six elements progressively fill up the 2p subshell:
- B: 1s22s22p1
- C: 1s22s22p2
- N: 1s22s22p3
- O: 1s22s22p4
- F: 1s22s22p5
- Ne: 1s22s22p6
Now that the 2p subshell is filled (all possible subshells in the n = 2 shell), the next electron for the next-larger atom must go into the n = 3 shell, s subshell.
Second Period Elements
Periods refer to the horizontal rows of the periodic table. Looking at a periodic table you will see that the first period contains only the elements hydrogen and helium. This is because the first principal energy level consists of only the \(s\) sublevel and so only two electrons are required in order to fill the entire principal energy level. Each time a new principal energy level begins, as with the third element lithium, a new period is started on the periodic table. As one moves across the second period, electrons are successively added. With beryllium \(\left( Z=4 \right)\), the \(2s\) sublevel is complete and the \(2p\) sublevel begins with boron \(\left( Z=5 \right)\). Since there are three \(2p\) orbitals and each orbital holds two electrons, the \(2p\) sublevel is filled after six elements. Table \(\PageIndex{1}\) shows the electron configurations of the elements in the second period.
| Element Name |
Symbol |
Atomic Number |
Electron Configuration |
Table \(\PageIndex{2}\): Electron Configurations of Second-Period Elements
| Lithium |
\(\ce{Li}\) |
3 |
\(1s^2 2s^1\) |
| Beryllium |
\(\ce{Be}\) |
4 |
\(1s^2 2s^2\) |
| Boron |
\(\ce{B}\) |
5 |
\(1s^2 2s^2 2p^1\) |
| Carbon |
\(\ce{C}\) |
6 |
\(1s^2 2s^2 2p^2\) |
| Nitrogen |
\(\ce{N}\) |
7 |
\(1s^2 2s^2 2p^3\) |
| Oxygen |
\(\ce{O}\) |
8 |
\(1s^2 2s^2 2p^4\) |
| Fluorine |
\(\ce{F}\) |
9 |
\(1s^2 2s^2 2p^5\) |
| Neon |
\(\ce{Ne}\) |
10 |
\(1s^2 2s^2 2p^6\) |
Aufbau Principle
Construction of a building begins at the bottom. The foundation is laid and the building goes up step by step. You obviously cannot start with the roof since there is no place to hang it. The building goes from the lowest level to the highest level in a systematic way. In order to create ground state electron configurations for any element, it is necessary to know the way in which the atomic sublevels are organized in order of increasing energy. Figure \(\PageIndex{5}\) shows the order of increasing energy of the sublevels.
The lowest energy sublevel is always the \(1s\) sublevel, which consists of one orbital. The single electron of the hydrogen atom will occupy the \(1s\) orbital when the atom is in its ground state. As we proceed with atoms with multiple electrons, those electrons are added to the next lowest sublevel: \(2s\), \(2p\), \(3s\), and so on. The Aufbau principle states that an electron occupies orbitals in order from lowest energy to highest. The Aufbau (German: "building up, construction") principle is sometimes referred to as the "building up" principle. It is worth noting that in reality atoms are not built by adding protons and electrons one at a time, and that this method is merely an aid for us to understand the end result.
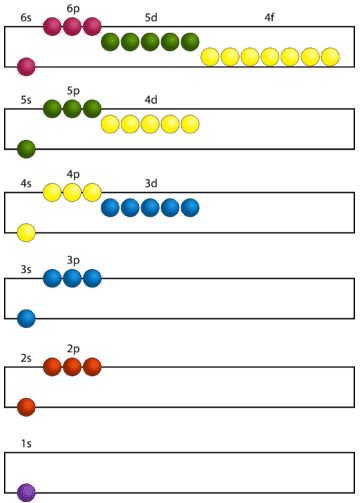
Figure \(\PageIndex{5}\): Electrons are added to atomic orbitals in order from low energy (bottom of the graph) to high (top of the graph) according to the Aufbau principle. Principle energy levels are color coded, while sublevels are grouped together and each circle represents an orbital capable of holding two electrons.
As seen in the figure above, the energies of the sublevels in different principal energy levels eventually begin to overlap. After the \(3p\) sublevel, it would seem logical that the \(3d\) sublevel should be the next lowest in energy. However, the \(4s\) sublevel is slightly lower in energy than the \(3d\) sublevel and thus fills first. Following the filling of the \(3d\) sublevel is the \(4p\), then the \(5s\) and the \(4d\). Note that the \(4f\) sublevel does not fill until just after the \(6s\) sublevel. Figure \(\PageIndex{6}\) is a useful and simple aid for keeping track of the order of fill of the atomic sublevels.
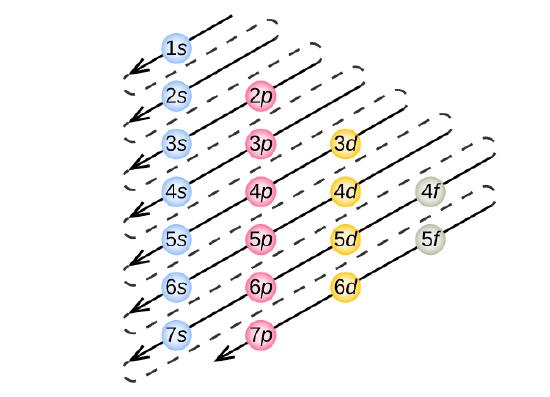
Figure \(\PageIndex{6}\): The arrow leads through each subshell in the appropriate filling order for electron configurations. This chart is straightforward to construct. Simply make a column for all the s orbitals with each n shell on a separate row. Repeat for p, d, and f. Be sure to only include orbitals allowed by the quantum numbers (no 1p or 2d, and so forth). Finally, draw diagonal lines from top to bottom as shown.
Video \(\PageIndex{1}\): Energy levels, sublevels and orbitals.
Example \(\PageIndex{1}\): Nitrogen Atoms
Nitrogen has 7 electrons. Write the electron configuration for nitrogen.
Solution:
Take a close look at Figure \(\PageIndex{5}\), and use it to figure out how many electrons go into each sublevel, and also the order in which the different sublevels get filled.
1. Begin by filling up the 1s sublevel. This gives 1s2. Now all of the orbitals in the red n = 1 block are filled.
- Since we used 2 electrons, there are 7 − 2 = 5 electrons left
2. Next, fill the 2s sublevel. This gives 1s22s2. Now all of the orbitals in the s sublevel of the orange n = 2 block are filled.
- Since we used another 2 electrons, there are 5 − 2 = 3 electrons left
3. Notice that we haven't filled the entire n = 2 block yet… there are still the p orbitals!
- The final 3 electrons go into the 2p sublevel. This gives 1s22s22p3
The overall electron configuration is: 1s22s22p3.
Example \(\PageIndex{2}\): Potassium Atoms
Potassium has 19 electrons. Write the electron configuration code for potassium.
Solution
This time, take a close look at Figure \(\PageIndex{5}\).
1. Begin by filling up the 1s sublevel. This gives 1s2. Now the n = 1 level is filled.
- Since we used 2 electrons, there are 19 − 2 = 17 electrons left
2. Next, fill the 2s sublevel. This gives 1s22s2
- Since we used another 2 electrons, there are 17 − 2 = 15 electrons left
3. Next, fill the 2p sublevel. This gives 1s22s22p6. Now the n = 2 level is filled.
- Since we used another 6 electrons, there are 15 − 6 = 9 electrons left
4. Next, fill the 3s sublevel. This gives 1s22s22p63s2
- Since we used another 2 electrons, there are 9 − 2 = 7 electrons left
5. Next, fill the 3p sublevel. This gives 1s22s22p63s23p6
- Since we used another 6 electrons, there are 7 − 6 = 1 electron left
Here's where we have to be careful – right after 3p6!
- Remember, 4s comes before 3d
6. The final electron goes into the 4s sublevel. This gives 1s22s22p63s23p64s1
The overall electron configuration is: 1s22s22p63s23p64s1
Exercise \(\PageIndex{1}\): Magnesium and Sodium Atoms
What is the electron configuration for Mg and Na?
- Answer Mg
- Mg: 1s22s22p63s2
- Answer Na
- Na: 1s22s22p63s1
Pauli Exclusion Principle
When we look at the orbital possibilities for a given atom, we see that there are different arrangements of electrons for each different type of atom. Since each electron must maintain its unique identity, we intuitively sense that the four quantum numbers for any given electron must not match up exactly with the four quantum numbers for any other electron in that atom.
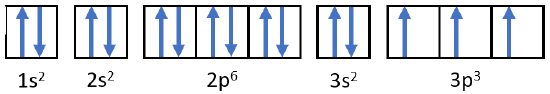
For the hydrogen atom, there is no problem since there is only one electron in the \(\ce{H}\) atom. However, when we get to helium we see that the first three quantum numbers for the two electrons are the same: same energy level, same spherical shape. What differentiates the two helium electrons is their spin. One of the electrons has a \(+\dfrac{1}{2}\) spin while the other electron has a \(-\dfrac{1}{2}\) spin. So the two electrons in the \(1s\) orbital are each unique and distinct from one another because their spins are different. This observation leads to the Pauli exclusion principle, which states that no two electrons in an atom can have the same set of four quantum numbers. The energy of the electron is specified by the principal, angular momentum, and magnetic quantum numbers. If those three numbers are identical for two electrons, the spin numbers must be different in order for the two electrons to be differentiated from one another. The two values of the spin quantum number allow each orbital to hold two electrons. Figure \(\PageIndex{7}\) shows how the electrons are indicated in a diagram.
Figure \(\PageIndex{7}\): In an orbital filling diagram, a square represents an orbital, while arrows represent electrons. An arrow pointing upward represents one spin direction, while an arrow pointing downward represents the other spin direction.
Hund's Rule
The last of the three rules for constructing electron arrangements requires electrons to be placed one at a time in a set of orbitals within the same sublevel. This minimizes the natural repulsive forces that one electron has for another. Hund's rule states that orbitals of equal energy are each occupied by one electron before any orbital is occupied by a second electron and that each of the single electrons must have the same spin. The figure below shows how a set of three \(p\) orbitals is filled with one, two, three, and four electrons.
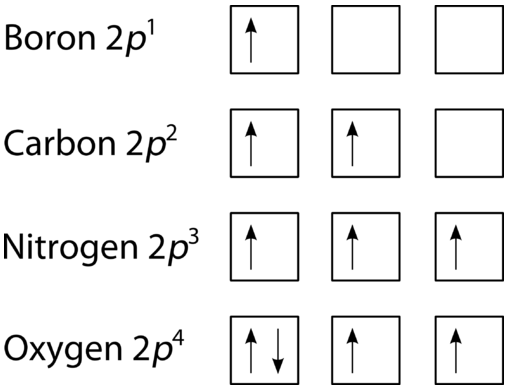
Figure \(\PageIndex{8}\): The \(2p\) sublevel, for the elements boron \(\left( Z=5 \right)\), carbon \(\left( Z=6 \right)\), nitrogen \(\left( Z=7 \right)\), and oxygen \(\left( Z=8 \right)\). According to Hund's rule, as electrons are added to a set of orbitals of equal energy, one electron enters each orbital before any orbital receives a second electron.
Orbital Filling Diagrams
An orbital filling diagram is the more visual way to represent the arrangement of all the electrons in a particular atom. In an orbital filling diagram, the individual orbitals are shown as circles (or squares) and orbitals within a sublevel are drawn next to each other horizontally. Each sublevel is labeled by its principal energy level and sublevel. Electrons are indicated by arrows inside of the circles. An arrow pointing upwards indicates one spin direction, while a downward pointing arrow indicates the other direction. The orbital filling diagrams for hydrogen, helium, and lithium are shown in the figure below.
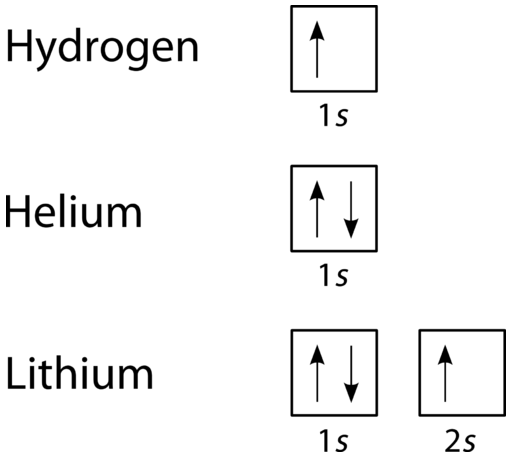
Figure \(\PageIndex{9}\): Orbital filling diagrams for hydrogen, helium, and lithium.
According to the Aufbau process, sublevels and orbitals are filled with electrons in order of increasing energy. Since the \(s\) sublevel consists of just one orbital, the second electron simply pairs up with the first electron as in helium. The next element is lithium and necessitates the use of the next available sublevel, the \(2s\).
The filling diagram for carbon is shown in Figure \(\PageIndex{10}\). There are two \(2p\) electrons for carbon and each occupies its own \(2p\) orbital.
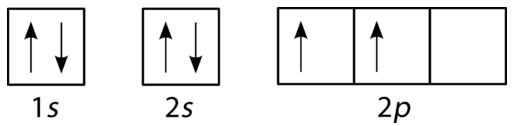
Figure \(\PageIndex{10}\): Orbital filling diagram for carbon.
Oxygen has four \(2p\) electrons. After each \(2p\) orbital has one electron in it, the fourth electron can be placed in the first \(2p\) orbital with a spin opposite that of the other electron in that orbital.
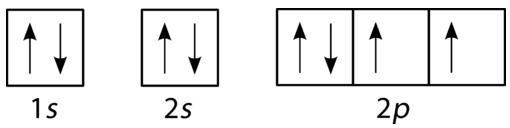
Figure \(\PageIndex{11}\): Orbital filling diagram for oxygen.
If you keep your papers in manila folders, you can pick up a folder and see how much it weighs. If you want to know how many different papers (articles, bank records, or whatever else you keep in a folder), you have to take everything out and count. A computer directory, on the other hand, tells you exactly how much you have in each file. We can get the same information on atoms. If we use an orbital filling diagram, we have to count arrows. When we look at electron configuration data, we simply add up the numbers.
Example \(\PageIndex{3}\): Carbon Atoms
Draw the orbital filling diagram for carbon and write its electron configuration.
Solution
Step 1: List the known quantities and plan the problem.
Known
- Atomic number of carbon, Z=6
Use the order of fill diagram to draw an orbital filling diagram with a total of six electrons. Follow Hund's rule. Write the electron configuration.
Step 2: Construct the diagram.
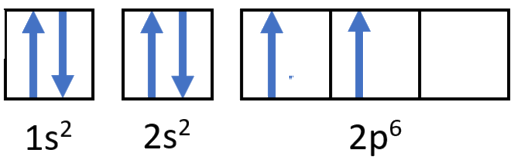
Orbital filling diagram for carbon.
Electron configuration 1s22s22p2
Step 3: Think about your result.
Following the 2s sublevel is the 2p, and p sublevels always consist of three orbitals. All three orbitals need to be drawn even if one or more is unoccupied. According to Hund's rule, the sixth electron enters the second of those p orbitals and has the same spin as the fifth electron.
Exercise \(\PageIndex{2}\): Electronic Configurations
Write the electron configurations and orbital diagrams for
- Potassium atom: \(\ce{K}\)
- Arsenic atom: \(\ce{As}\)
- Phosphorus atom: \(\ce{P}\)
- Answer a:
-
Potassium: \(1s^2 2s^2 2p^6 3s^2 3p^6 4s^1\)
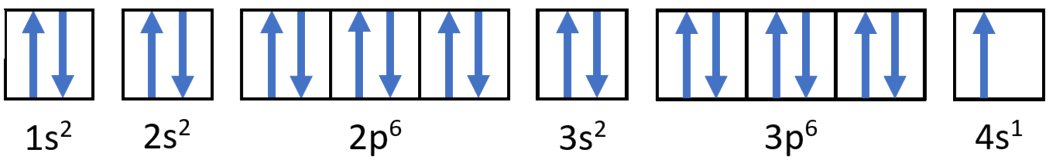
- Answer b:
-
Arsenic: \(1s^2 2s^2 2p^6 3s^2 3p^6 4s^2 3d^{10} 4p^3\)

- Answer c:
-
Phosphorus \(1s^2 2s^2 2p^6 3s^2 3p^3\)
The Atom Neighborhood
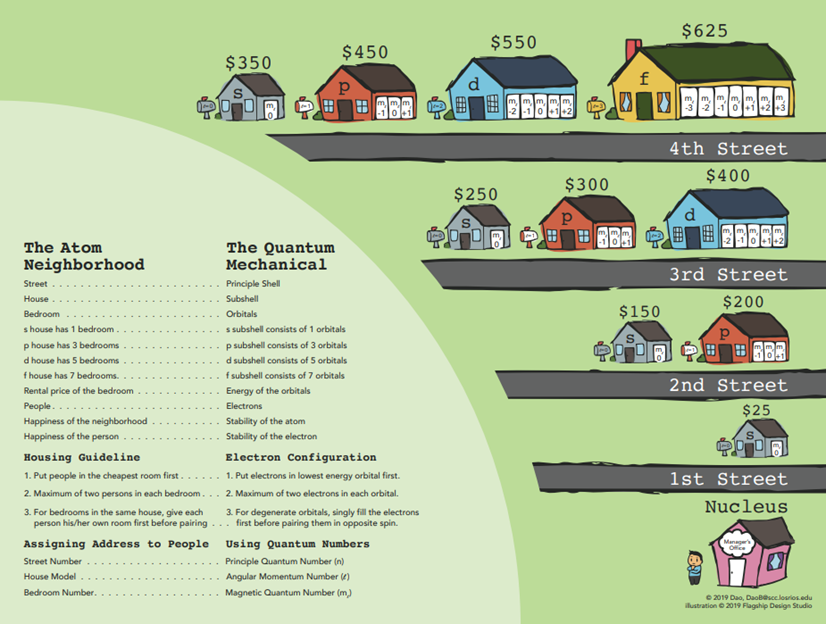
Figure \(\PageIndex{12}\): The atom neighborhood. Source: Dr. Binh Dao, Sacramento City College.


 Figure \(\PageIndex{1}\): \(s\) orbitals have no orientational preference and resemble spheres.
Figure \(\PageIndex{1}\): \(s\) orbitals have no orientational preference and resemble spheres.
 Figure \(\PageIndex{2}\): \(p\) orbitals have an orientational preference and resemble dumbbells.
Figure \(\PageIndex{2}\): \(p\) orbitals have an orientational preference and resemble dumbbells.
 Figure \(\PageIndex{3}\): \(d\) orbitals have an orientational preference and exhibit complex structures.
Figure \(\PageIndex{3}\): \(d\) orbitals have an orientational preference and exhibit complex structures.
 Figure \(\PageIndex{4}\): \(f\) orbitals have an orientational preference and exhibit quite complex structures.
Figure \(\PageIndex{4}\): \(f\) orbitals have an orientational preference and exhibit quite complex structures.
 Figure \(\PageIndex{5}\): Electrons are added to atomic orbitals in order from low energy (bottom of the graph) to high (top of the graph) according to the Aufbau principle. Principle energy levels are color coded, while sublevels are grouped together and each circle represents an orbital capable of holding two electrons.
Figure \(\PageIndex{5}\): Electrons are added to atomic orbitals in order from low energy (bottom of the graph) to high (top of the graph) according to the Aufbau principle. Principle energy levels are color coded, while sublevels are grouped together and each circle represents an orbital capable of holding two electrons.
 Figure \(\PageIndex{6}\): The arrow leads through each subshell in the appropriate filling order for electron configurations. This chart is straightforward to construct. Simply make a column for all the s orbitals with each n shell on a separate row. Repeat for p, d, and f. Be sure to only include orbitals allowed by the quantum numbers (no 1p or 2d, and so forth). Finally, draw diagonal lines from top to bottom as shown.
Figure \(\PageIndex{6}\): The arrow leads through each subshell in the appropriate filling order for electron configurations. This chart is straightforward to construct. Simply make a column for all the s orbitals with each n shell on a separate row. Repeat for p, d, and f. Be sure to only include orbitals allowed by the quantum numbers (no 1p or 2d, and so forth). Finally, draw diagonal lines from top to bottom as shown.
 Figure \(\PageIndex{7}\): In an orbital filling diagram, a square represents an orbital, while arrows represent electrons. An arrow pointing upward represents one spin direction, while an arrow pointing downward represents the other spin direction.
Figure \(\PageIndex{7}\): In an orbital filling diagram, a square represents an orbital, while arrows represent electrons. An arrow pointing upward represents one spin direction, while an arrow pointing downward represents the other spin direction.
 Figure \(\PageIndex{8}\): The \(2p\) sublevel, for the elements boron \(\left( Z=5 \right)\), carbon \(\left( Z=6 \right)\), nitrogen \(\left( Z=7 \right)\), and oxygen \(\left( Z=8 \right)\). According to Hund's rule, as electrons are added to a set of orbitals of equal energy, one electron enters each orbital before any orbital receives a second electron.
Figure \(\PageIndex{8}\): The \(2p\) sublevel, for the elements boron \(\left( Z=5 \right)\), carbon \(\left( Z=6 \right)\), nitrogen \(\left( Z=7 \right)\), and oxygen \(\left( Z=8 \right)\). According to Hund's rule, as electrons are added to a set of orbitals of equal energy, one electron enters each orbital before any orbital receives a second electron.
 Figure \(\PageIndex{9}\): Orbital filling diagrams for hydrogen, helium, and lithium.
Figure \(\PageIndex{9}\): Orbital filling diagrams for hydrogen, helium, and lithium.
 Figure \(\PageIndex{10}\): Orbital filling diagram for carbon.
Figure \(\PageIndex{10}\): Orbital filling diagram for carbon.
 Figure \(\PageIndex{11}\): Orbital filling diagram for oxygen.
Figure \(\PageIndex{11}\): Orbital filling diagram for oxygen.




 Figure \(\PageIndex{12}\): The atom neighborhood. Source: Dr. Binh Dao, Sacramento City College.
Figure \(\PageIndex{12}\): The atom neighborhood. Source: Dr. Binh Dao, Sacramento City College.