4.4: Absolute Value Inequalities
- Page ID
- 19702
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)In the last section, we solved absolute value equations. In this section, we turn our attention to inequalities involving absolute value.
Solving |x| < a
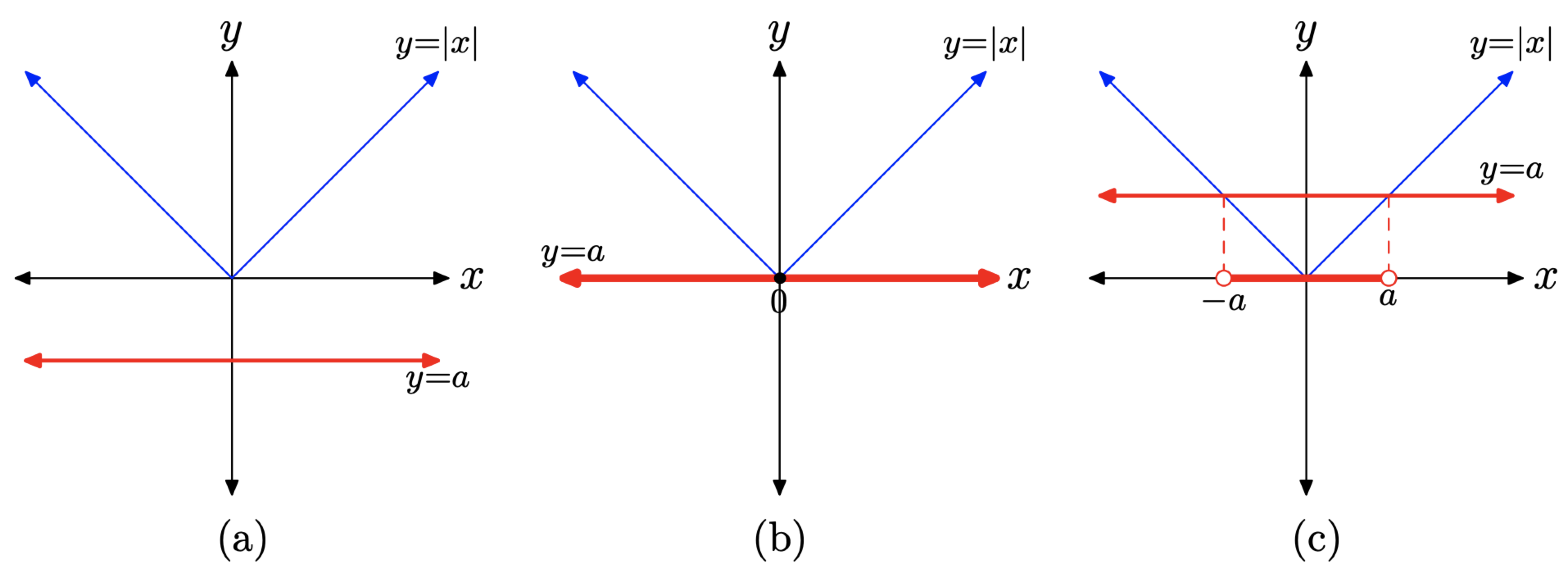
The solutions of \[|x|<a \nonumber \] again depend upon the value and sign of the number a. To solve |x| < a graphically, we must determine where the graph of the left-hand side lies below the graph of the right-hand side of the inequality |x| < a. There are three cases to consider.
- Case I: a < 0
In this case, the graph of y = a lies strictly below the x-axis. As you can see in Figure \(\PageIndex{1}\)(a), the graph of y = |x| never lies below the graph of y = a. Hence, the inequality |x| < a has no solutions.
- Case II: a = 0
In this case, the graph of y = 0 coincides with the x-axis. As you can see in Figure \(\PageIndex{1}\)(b), the graph of y = |x| never lies strictly below the x-axis. Hence, the inequality |x| < 0 has no solutions.
- Case III: a > 0
In this case, the graph of y = a lies strictly above the x-axis. In Figure \(\PageIndex{1}\)(c), the graph of y = |x| and y = a intersect at x = −a and x = a. In Figure \(\PageIndex{1}\)(c), we also see that the graph of y = |x| lies strictly below the graph of y = a when x is in-between −a and a; that is, when −a < x < a.
In Figure \(\PageIndex{1}\)(c), we’ve dropped dashed vertical lines from the points of intersection of the two graphs to the x-axis. On the x-axis, we’ve shaded the solution of |x| < a, that is, −a < x < a.
This discussion leads to the following key property.
The solution of |x| < a depends upon the value and sign of a.
- Case I: a < 0
The inequality |x| < a has no solution.
- Case II: a = 0
The inequality |x| < 0 has no solution.
- Case III: a > 0
The inequality |x| < a has solution set {x : −a < x < a}.
Let’s look at some examples.
Solve the inequality |x| < −5 for x.
Solution
The graph of the left-hand side of |x| < −5 is the “V” of Figure \(\PageIndex{1}\)(a). The graph of the right-hand side of |x| < −5 is a horizontal line located 5 units below the x-axis. This is the situation shown in Figure \(\PageIndex{1}\)(a). The graph of y = |x| is therefore never below the graph of y = −5. Thus, the inequality |x| < −5 has no solution.
An alternate approach is to consider the fact that the absolute value of x is always nonnegative and can never be less than −5. Thus, the inequality |x| < −5 has no solution.
Solve the inequality |x| < 0 for x.
Solution
This is the case shown in Figure \(\PageIndex{1}\)(b). The graph of y = |x| is never strictly below the x-axis. Thus, the inequality |x| < 0 has no solution.
Solve the inequality |x| < 8 for x.
Solution
The graph of the left-hand side of |x| < 8 is the “V” of Figure \(\PageIndex{1}\)(c). The graph of the right-hand side of |x| < 8 is a horizontal line located 8 units above the x-axis. This is the situation depicted in Figure \(\PageIndex{1}\)(c). The graphs intersect at (−8, 8) and (8, 8) and the graph of y = |x| lies strictly below the graph of y = 8 for values of x in-between −8 and 8. Thus, the solution of |x| < 8 is −8 < x < 8.
It helps the intuition if you check the results of the last example. Note that numbers between −8 and 8, such as −7.75, −3 and 6.8 satisfy the inequality,
\[|-7.75|<8 \qquad \text { and } \quad|-3|<8 \quad \text { and } \quad|6.8|<8 \nonumber \]
while values that do not lie between −8 and 8 do not satisfy the inequality. For example, none of the numbers −9.3, 8.2, and 11.7 lie between −8 and 8, and each of the following is a false statement.
\[|-9.3|<8 \quad \text { and } \qquad|8.2|<8 \qquad \text { and } \qquad|11.7|<8 \quad \text { (all are false) } \nonumber \]
If you reflect upon these results, they will help cement the notion that the solution of |x| < 8 is all values of x satisfying −8 < x < 8.
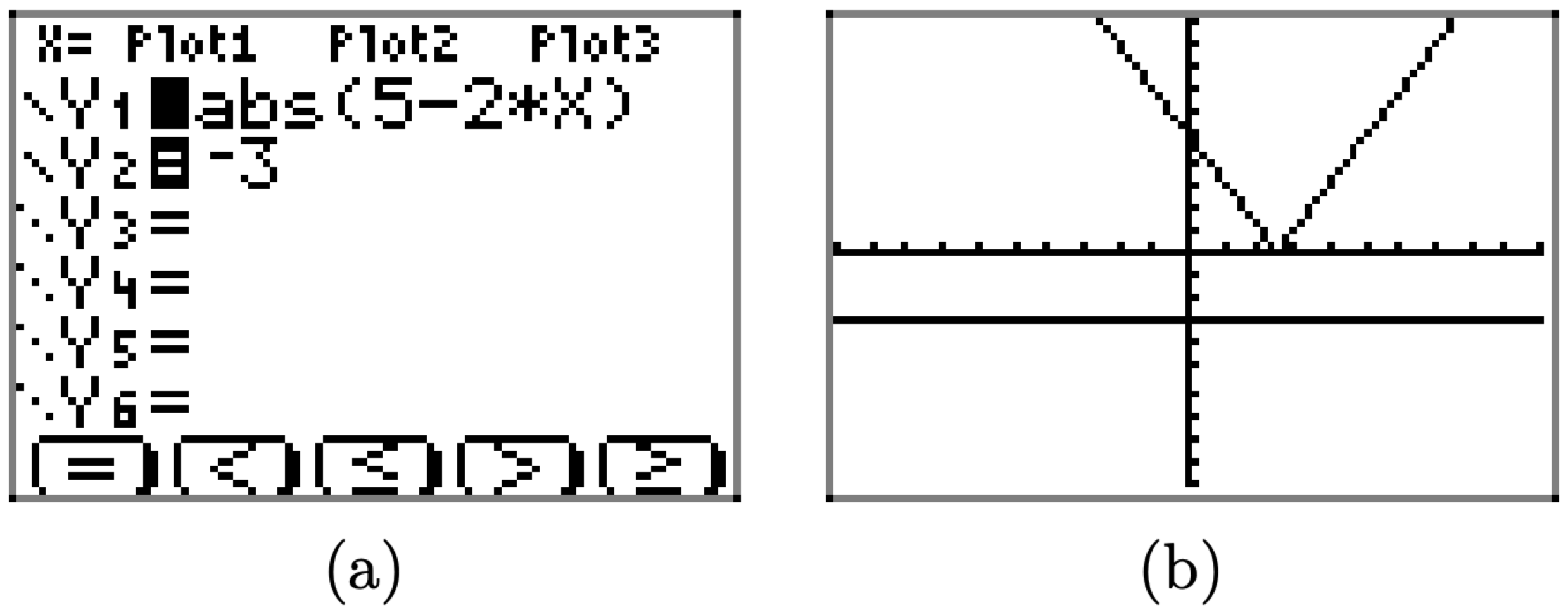
Solve the inequality |5 − 2x| < −3 for x.
Solution
If the inequality were |x| < −3, we would not hesitate. This is the situation depicted in Figure \(\PageIndex{1}\)(a) and the inequality |x| < −3 has no solutions. The reasoning applied to |x| < −3 works equally well for the inequality |5 − 2x| < −3. The left-hand side of this inequality must be nonnegative, so its graph must lie on or above the x-axis. The right-hand side of |5 − 2x| < −3 is a horizontal line located 3 units below the x-axis. Therefore, the graph of y = |5 − 2x| can never lie below the graph of y = −3 and the inequality |5 − 2x| < −3 has no solution.
We can verify this result with the graphing calculator. Load the left- and right-hand sides of |5 − 2x| < −3 into Y1 and Y2, respectively, as shown in Figure \(\PageIndex{2}\)(a). From the ZOOM menu, select 6:ZStandard to produce the image shown in Figure \(\PageIndex{2}\)(b).
As predicted, the graph of y = |5 − 2x| never lies below the graph of y = −3, so the inequality |5 − 2x| < −3 has no solution.
Solve the inequality |5 − 2x| < 0 for x.
Solution
We know that the left-hand side of the inequality |5 − 2x| < 0 has the “V” shape indicated in Figure \(\PageIndex{1}\)(b). The graph “touches” the x-axis when |5 − 2x| = 0, or when
\[\begin{aligned} 5-2 x &=0 \\-2 x &=-5 \\ x &=\frac{5}{2} \end{aligned} \nonumber \]
However, the graph of y = |5 − 2x| never falls below the x-axis, so the inequality |5 − 2x| < 0 has no solution.
Intuitively, it should be clear that the inequality |5−2x| < 0 has no solution. Indeed, the left-hand side of this inequality is always nonnegative, and can never be strictly less than zero.
Solve the inequality |5 − 2x| < 3 for x.
Solution
In this example, the graph of the right-hand side of the inequality |5 − 2x| < 3 is a horizontal line located 3 units above the x-axis. The graph of the left-hand side of the inequality has the “V” shape shown in Figure \(\PageIndex{3}\)(b) and (c). You can use the intersect utility on the graphing calculator to find the points of intersection of the graphs of y = |5 − 2x| and y = 3, as we have done in Figures \(\PageIndex{3}\)(b) and (c). Note that the calculator indicates two points of intersection, one at x = 1 and a second at x = 4.
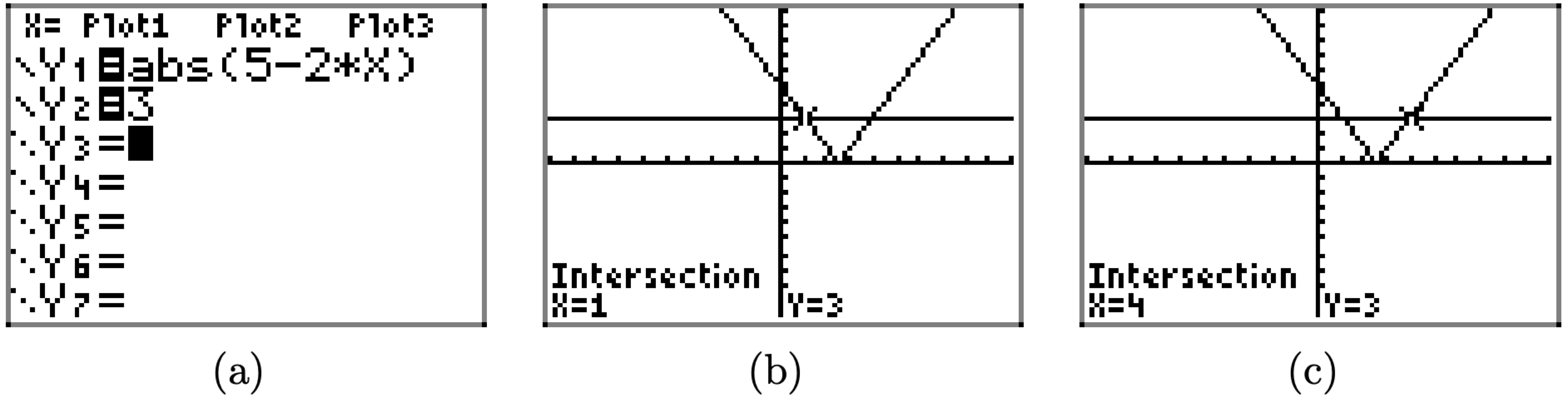
The graph of y = |5 − 2x| falls below the graph of y = 3 for all values of x between 1 and 4. Hence, the solution of the inequality |5 − 2x| < 3 is the set of all x satisfying 1 < x < 4; i.e. {x : 1 < x < 4}.
Expectations:
We need a way of summarizing this graphing calculator approach on our homework paper. First, draw a reasonable facsimile of your calculator’s viewing window on your homework paper. Use a ruler to draw all lines. Complete the following checklist.
- Label each axis, in this case with x and y.
- Scale each axis. To do this, press the WINDOW button on your calculator, then report the values of xmin, xmax, ymin, and ymax on the appropriate axis.
- Label each graph with its equation.
- Drop dashed vertical lines from the points of intersection to the x-axis. Shade and label the solution set of the inequality on the x-axis.
Following the guidelines in the above checklist, we obtain the image in Figure \(\PageIndex{4}\).
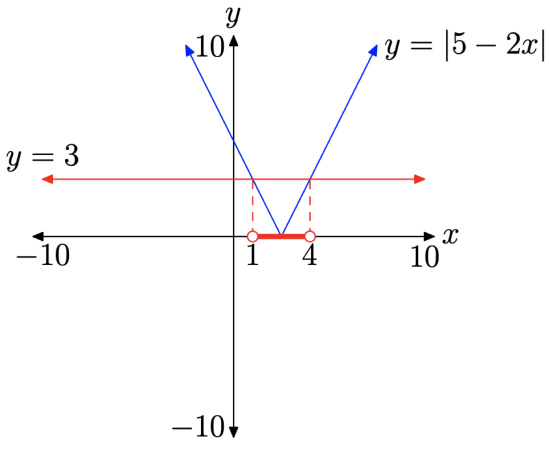
Algebraic Approach. Let’s now explore an algebraic solution of the inequality |5 − 2x| < 3. Much as |x| < 3 implies that −3 < x < 3, the inequality
\[|5-2 x|<3 \nonumber \]
requires that
\[-3<5-2 x<3 \nonumber \]
We can subtract 5 from all three members of this last inequality, then simplify.
\[\begin{aligned}-3-5 &<5-2 x-5<3-5 \\ &-8<-2 x<-2 \end{aligned} \nonumber \]
Divide all three members of this last inequality by −2, reversing the inequality symbols as you go.
\[4>x>1 \nonumber \]
We prefer that our inequalities read from “small-to-large,” so we write
\[1<x<4 \nonumber \]
This form matches the order of the shaded solution on the number line in Figure \(\PageIndex{4}\), which we found using the graphing calculator.
The algebraic technique of this last example leads us to the following property.
If a > 0, then the inequality |x| < a is equivalent to the inequality −a < x < a.
This property provides a simple method for solving inequalities of the form |x| < a. Let’s apply this algebraic technique in the next example.
Solve the inequality |4x + 5| < 7 for x.
Solution
The first step is to use Property 8 to write that \[|4 x+5|<7 \nonumber \]
is equivalent to the inequality
\[-7<4 x+5<7 \nonumber \]
From here, we can solve for x by first subtracting 5 from all three members, then dividing through by 4.
\[\begin{array}{l}{-12<4 x<2} \\ {-3<x<\frac{1}{2}}\end{array} \nonumber \]
We can sketch the solution on a number line.

And we can describe the solution in both interval and set-builder notation as follows.
\[\left(-3, \frac{1}{2}\right)=\left\{x :-3<x<\frac{1}{2}\right\} \nonumber \]
Assuming that a > 0, the inequality \(|x| \leq a\) requires that we find where the absolute value of x is either “less than” a or “equal to” a. We know that |x| < a when −a < x < a and we know that |x| = a when x = −a or x = a. Thus, the solution of \(|x| \leq a\) is the “union” of these two solutions.
This argument leads to the following property.
If \(a > 0\), then the inequality \(|x| \leq a\) is equivalent to the inequality \(−a \leq x \leq a\).
Solve the inequality \(5 − 3|x − 4| \geq −4\) for x.
Solution
At first glance, the inequality \[5-3|x-4| \geq-4 \nonumber \] has a form quite dissimilar from what we’ve done thus far. However, let’s subtract 5 from both sides of the inequality.
\[-3|x-4| \geq-9 \nonumber \]
Now, let’s divide both sides of this last inequality by −3, reversing the inequality sign.
\[|x-4| \leq 3 \nonumber \]
Aha! Familiar ground. Using Property 10, this last inequality is equivalent to
\[-3 \leq x-4 \leq 3 \nonumber \]
and when we add 4 to all three members, we have the solution.
\[1 \leq x \leq 7 \nonumber \]
We can sketch the solution on a number line

And we can describe the solution with interval and set-builder notation.
\[[1,7]=\{x : 1 \leq x \leq 7\} \nonumber \]
Solving |x| > a
The solutions of |x| > a again depend upon the value and sign of a. To solve |x| > a graphically, we must determine where the graph of y = |x| lies above the graph of y = a. Again, we consider three cases.
- Case I: a < 0
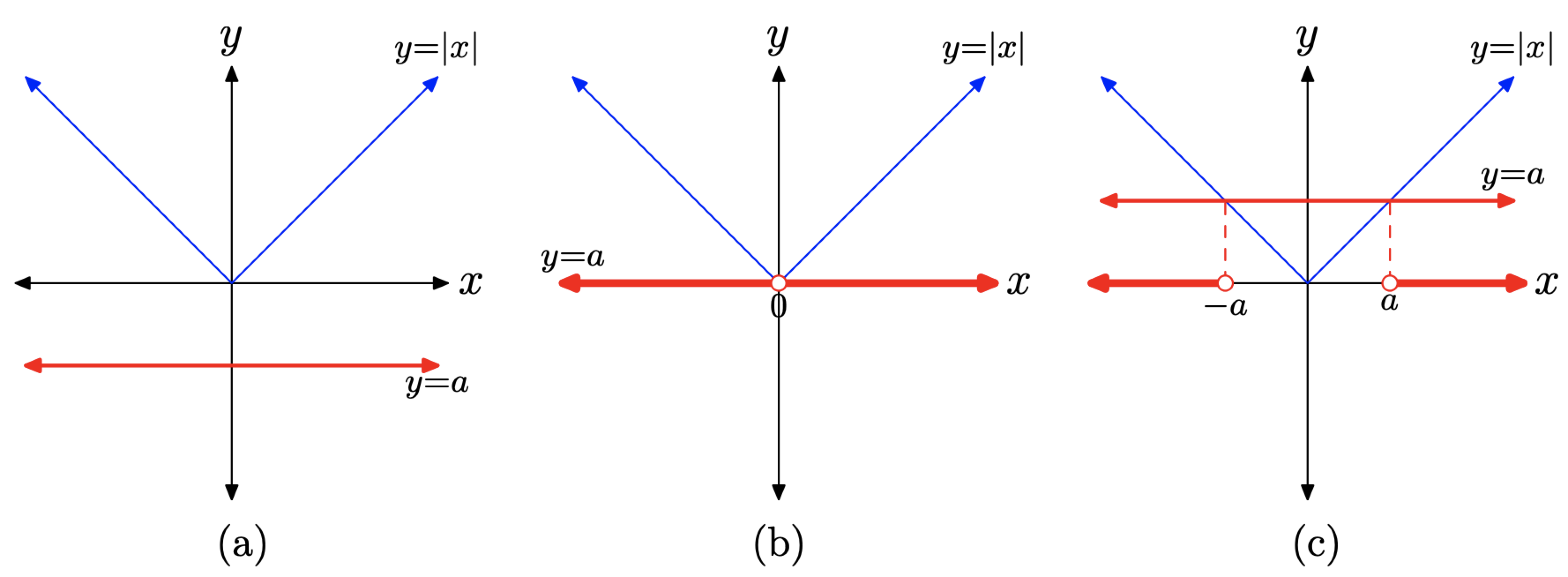
In this case, the graph of y = a lies strictly below the x-axis. Therefore, the graph of y = |x| in Figure \(\PageIndex{5}\)(a) always lies above the graph of y = a. Hence, all real numbers are solutions of the inequality |x| > a.
- Case II: a = 0
In this case, the graph of y = 0 coincides with the x-axis. As shown in Figure \(\PageIndex{5}\)(b), the graph of y = |x| will lie strictly above the graph of y = 0 for all values of x with one exception, namely, x cannot equal zero. Hence, every real number except x = 0 is a solution of |x| > 0. In Figure \(\PageIndex{5}\)(b), we’ve shaded the solution of |x| > 0, namely the set of all real numbers except x = 0.
- Case III: a > 0
In this case, the graph of y = a lies strictly above the x-axis. In Figure \(\PageIndex{5}\)(c), the graph of y = |x| intersects the graph of y = a at x = −a and x = a. In Figure \(\PageIndex{5}\)(c), we see that the graph of y = |x| lies strictly above the graph of y = a if x is less than −a or greater than a.
In Figure \(\PageIndex{5}\)(c), we’ve dropped dashed vertical lines from the points of intersection to the x-axis. On the x-axis, we’ve shaded the solution of |x| > a, namely the set of all real numbers x such that x < −a or x > a.
This discussion leads to the following property.
The solution of |x| > a depends upon the value and sign of a.
- Case I: a < 0
All real numbers are solutions of the inequality |x| > a.
- Case II: a = 0
All real numbers, with the exception of x = 0, are solutions of |x| > 0.
- Case III: a > 0
The inequality |x| > a has solution set {x : x < −a or x > a}.
State the solution of each of the following inequalities.
\[\text { a. }|x|>-5 \qquad \text { b. }|x|>0 \qquad \text { c. }|x|>4 \nonumber \]
Solution
a. The solution of |x| > −5 is all real numbers.
b. The solution of |x| > 0 is all real numbers except zero.
c. The solution of |x| > 4 is the set of all real numbers less than −4 or greater than 4.
Solve the inequality |4 − x| > −5 for x.
Solution
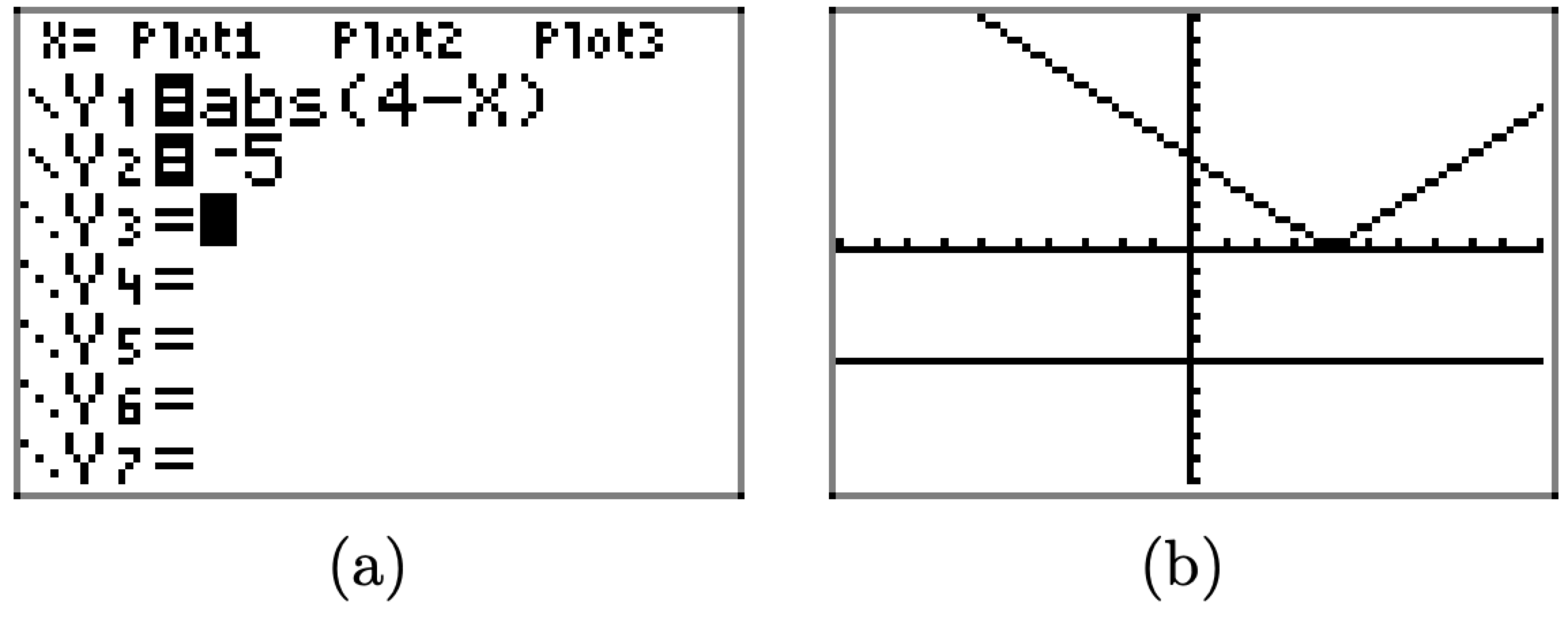
The left-hand side of the inequality |4 − x| > −5 is nonnegative, so the graph of y = |4 − x| must lie above or on the x-axis. The graph of the right-hand side of |4 − x| > −5 is a horizontal line located 5 units below the x-axis. Therefore, the graph of y = |4 − x| always lies above the graph of y = −5. Thus, all real numbers are solutions of the inequality |4 − x| > −5.
We can verify our thinking with the graphing calculator. Load the left- and righthand sides of the inequality |4 − x| > −5 into Y1 and Y2, respectively, as shown in Figure \(\PageIndex{6}\)(a). From the ZOOM menu, select 6:ZStandard to produce the image shown in Figure \(\PageIndex{6}\)(b).
As predicted, the graph of y = |4 − x| lies above the graph of y = −5 for all real numbers.
Intuitively, the absolute value of any number is always nonnegative, so |4−x| > −5 for all real values of x.
Solve the inequality |4 − x| > 0 for x.
Solution
As we saw in Figure \(\PageIndex{6}\)(b), the graph of y = |4 − x| lies on or above the x-axis for all real numbers. It “touches” the x-axis at the “vertex” of the “V,” where \[|4-x|=0 \nonumber \]
This can occur only if
\[\begin{aligned} 4-x &=0 \\-x &=-4 \\ x &=4 \end{aligned} \nonumber \]
Thus, the graph of y = |4 − x| is strictly above the x-axis for all real numbers except x = 4. That is, the solution of |4 − x| > 0 is {x : x 6= 4}.
Solve the inequality |4 − x| > 5 for x.
Solution
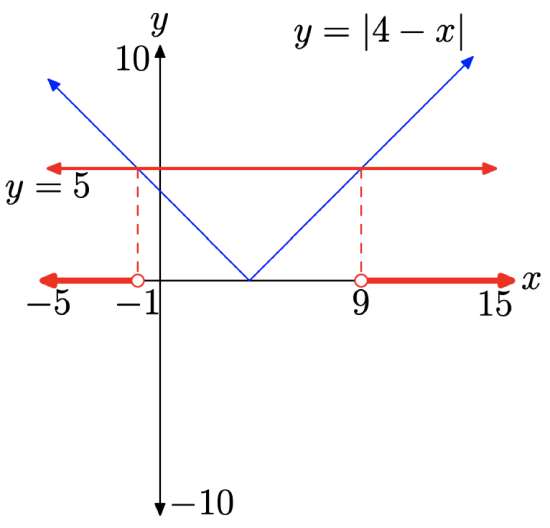
In this example, the graph of the right-hand side of |4 − x| > 5 is a horizontal line located 5 units above the x-axis. The graph of y = |4 − x| has the “V” shape shown in Figure \(\PageIndex{6}\)(c). You can use the intersect utility on the graphing calculator to approximate the points of intersection of the graphs of y = |4 − x| and y = 5, as we have done in Figure \(\PageIndex{7}\)(c) and (d). The calculator indicates two points of intersection, one at x = −1 and a second at x = 9.
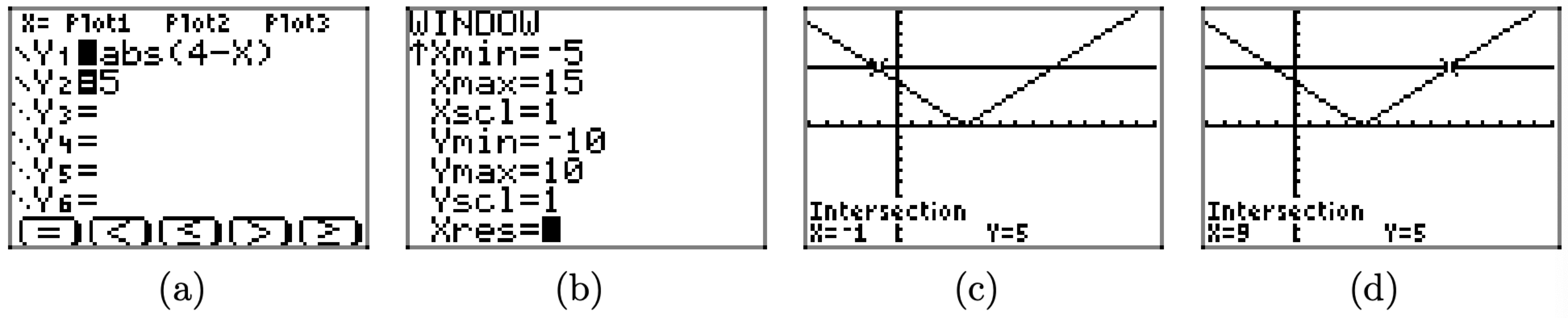
The graph of y = |4 − x| lies above the graph of y = 5 for all values of x that lie either to the left of −1 or to the right of 9. Hence, the solution of |4 − x| > 5 is the set {x : x < −1 or x > 9}.
Following the guidelines established in Example \(\PageIndex{6}\), we create the image shown in Figure \(\PageIndex{8}\) on our homework paper. Note that we’ve labeled each axis, scaled each axis with xmin, xmax, ymin, and ymax, labeled each graph with its equation, and shaded and labeled the solution on x-axis.
Algebraic Approach. Let’s explore an algebraic solution of |4 − x| > 5. In much the same manner that |x| > 5 leads to the conditions x < −5 or x > 5, the inequality
\[|4-x|>5 \nonumber \]
requires that
\[4-x<-5 \qquad \text { or } \qquad 4-x>5 \nonumber \]
We can solve each of these independently by first subtracting 4 from each side of the inequality, then multiplying both sides of each inequality by −1, reversing each inequality as we do so.
\[\begin{array}{rllrrl}{4-x} & {<} & {-5} & {\text { or }} & {4-x} & {>} & {5} \\ {-x} & {<} & {-9} && {-x} & {>} & {1} \\ {x} & {>} & {9} && {x} & {<} & {-1}\end{array} \nonumber \]
We prefer to write this solution in the order
\[x<-1 \qquad \text { or } \qquad x>9 \nonumber \]
as it then matches the order of the graphical solution shaded in Figure \(\PageIndex{8}\). That is, the solution set is {x : x < −1 or x > 9}.
The algebraic technique of this last example leads to the following property.
If a > 0, then the inequality |x| > a is equivalent to the compound inequality x < −a or x > a.
This property provides a simple algebraic technique for solving inequalities of the form |x| > a, when a > 0. Let’s concentrate on this technique in the examples that follow.
Solve the inequality |4x − 3| > 1 for x.
Solution
The first step is to use Property 17 to write that \[|4 x-3|>1 \nonumber \] is equivalent to
\[4 x-3<-1 \qquad \text { or } \qquad 4 x-3>1 \nonumber \]
We can now solve each inequality independently. We begin by adding 3 to both sides of each inequality, then we divide both sides of the resulting inequalities by 4.
\[\begin{array}{rrlrrl}{4 x-3} & {<} & {-1} & {\text { or }} & {4 x-3} & {>} & {1} \\ {4 x} & {<} & {2} && {4 x} & {>} & {4} \\ {x} & {<} & {\frac{1}{2}} & &{x} & {>} & {1}\end{array} \nonumber \]
We can sketch the solutions on a number line.

And we can describe the solution using interval and set-builder notation.
\[(-\infty, 1 / 2) \cup(1, \infty)=\{x : x<1 / 2 \text { or } x>1\} \nonumber \]
Again, let a > 0. As we did with \(|x| \leq a\), we can take the union of the solutions of |x| = a and |x| > a to find the solution of \(|x| \geq a\). This leads to the following property.
If a > 0, then the inequality \(|x| \geq a\) is equivalent to the inequality \(x \leq −a\) or \(x \geq a\).
Solve the inequality \(3|1 − x| − 4 \geq |1 − x|\) for x.
Solution
Again, at first glance, the inequality \[3|1-x|-4 \geq|1-x| \nonumber \]
looks unlike any inequality we’ve attempted to this point. However, if we subtract |1 − x| from both sides of the inequality, then add 4 to both sides of the inequality, we get
\[3|1-x|-|1-x| \geq 4 \nonumber \]
On the left, we have like terms. Note that 3|1−x|−|1−x| = 3|1−x|−1|1−x| = 2|1−x|. Thus,
\[2|1-x| \geq 4 \nonumber \]
Divide both sides of the last inequality by 2.
\[|1-x| \geq 2 \nonumber \]
We can now use Property 19 to write
\[1-x \leq-2 \quad \text { or } \qquad 1-x \geq 2 \nonumber \]
We can solve each of these inequalities independently. First, subtract 1 from both sides of each inequality, then multiply both sides of each resulting inequality by −1, reversing each inequality as you go.
\[\begin{array}{rllrrl}{1-x } & {\leq} & {-2} & {\text { or }} & {1-x } & {\geq} & { 2} \\ {-x } & {\leq} & {-3} && {-x } & {\geq } & {1} \\ {x} & { \geq } & {3} & &{x} & { \leq} & {-1}\end{array} \nonumber \]
We prefer to write this in the order
\[x \leq-1 \qquad \text { or } \qquad x \geq 3 \nonumber \]
We can sketch the solutions on a number line.

And we can describe the solutions using interval and set-builder notation.
\[(-\infty,-1] \cup[3, \infty)=\{x : x \leq-1 \text { or } x \geq 3\} \nonumber \]
Revisiting Distance
If a and b are any numbers on the real line, then the distance between a and b is found by taking the absolute value of their difference. That is, the distance d between a and b is calculated with d = |a − b|. More importantly, we’ve learned to pronounce the symbolism |a − b| as “the distance between a and b.” This pronunciation is far more useful than saying “the absolute value of a minus b.”
Solve the inequality |x − 3| < 8 for x.
Solution
This inequality is pronounced “the distance between x and 3 is less than 8.” Draw a number line, locate 3 on the line, then note two points that are 8 units away from 3.
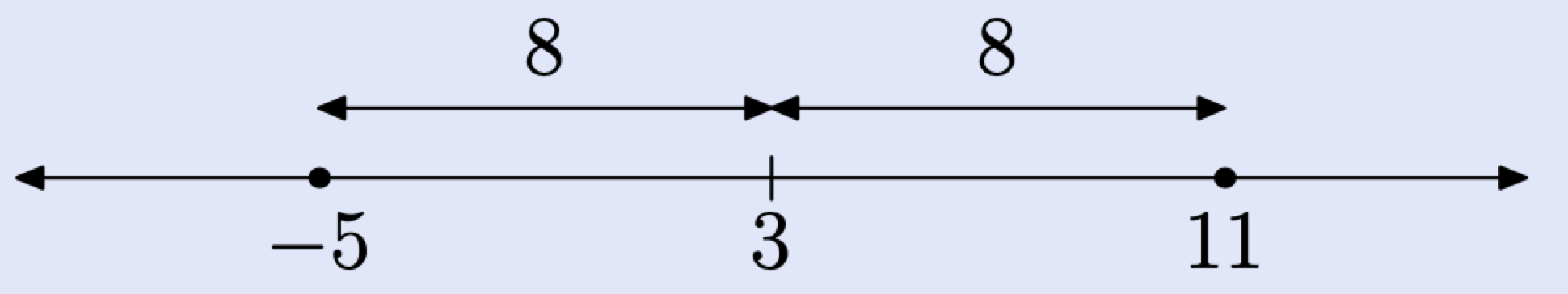
Now, we need to shade the points that are less than 8 units from 3.
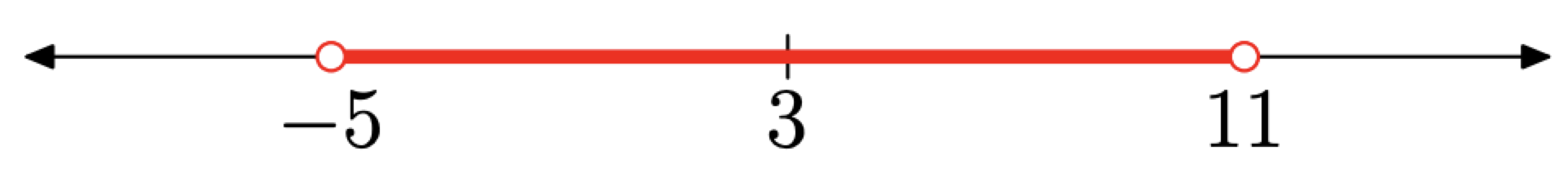
Hence, the solution of the inequality |x − 3| < 8 is \[(-5,11)=\{x :-5<x<11\} \nonumber \]
Solve the inequality |x + 5| > 2 for x.
Solution
First, write the inequality as a difference.
\[|x-(-5)|>2 \nonumber \]
This last inequality is pronounced “the distance between x and −5 is greater than 2.” Draw a number line, locate −5 on the number line, then note two points that are 2 units from −5.

Now, we need to shade the points that are greater than 2 units from −5.

Hence, the solution of the inequality |x + 5| > 2 is
\[(-\infty,-7) \cup(-3, \infty)=\{x : x<-7 \quad \text { or } \quad x>-3\} \nonumber \]
Exercise
For each of the inequalities in Exercises 1-10, perform each of the following tasks.
- Set up a coordinate system on a sheet of graph paper. Label and scale each axis.
- Sketch the graph of each side of the inequality without the aid of a calculator. Label each graph with its equation.
- Shade the solution of the inequality on the x-axis (if any) in the manner shown in Figures 4 and 8 in the narrative. That is, drop dashed lines from the points of intersection to the axis, then shade and label the solution set on the x-axis. Use set-builder and interval notation (when possible) to describe your solution set.
|x| > −2
- Answer
-

Solution: \(\mathbb{R} = (−\infty, \infty)\)
|x| > 0
|x| < 3
- Answer
-

Solution: (−3, 3) = {x : −3 < x < 3}
|x| > 2
|x| > 1
- Answer
-
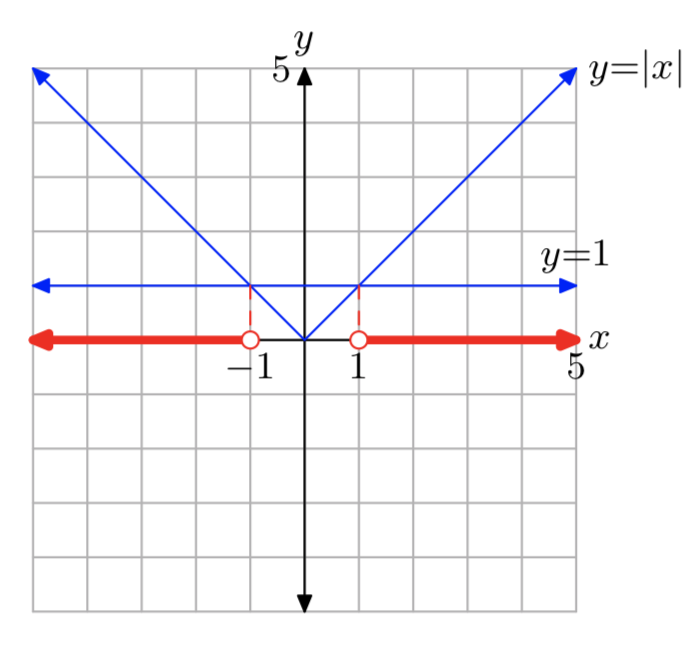
Solution: \((−\infty,−1) \cup (1,\infty)\) = {x : x <−1 or x > 1}.
|x| < 4
|x| ≤ 0
- Answer
-
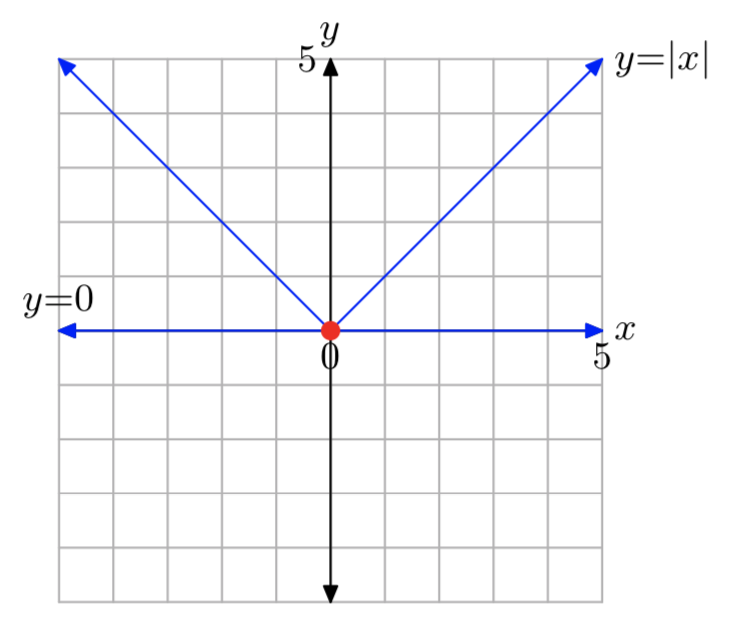
Solution: {x : x = 0}
|x| ≤ −2
|x| ≤ 2
- Answer
-
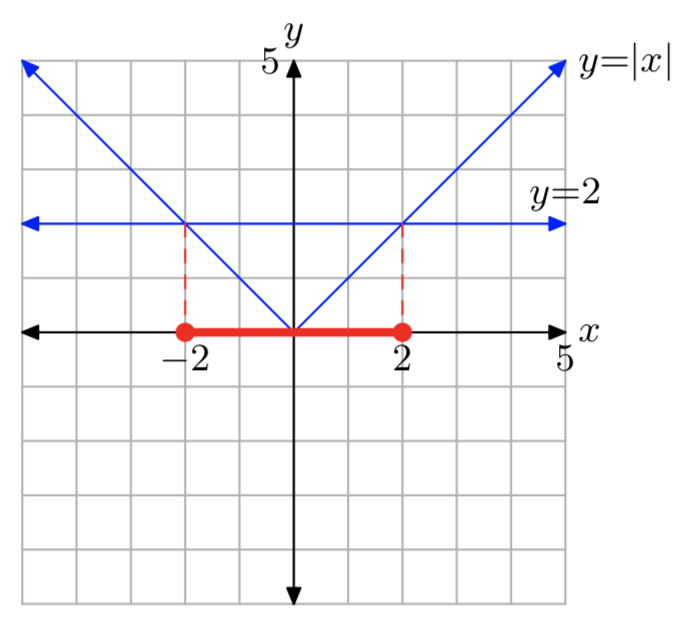
Solution: [−2, 2] = {x: \(−2 \le x \le 2\)}.
|x| ≥ 1
For each of the inequalities in Exercises 11-22, perform each of the following tasks.
- Load each side of the inequality into the Y= menu of your calculator. Adjust the viewing window so that all points of intersection of the two graphs are visible in the viewing window.
- Copy the image in your viewing screen onto your homework paper. Label each axis and scale each axis with xmin, xmax, ymin, and ymax. Label each graph with its equation.
- Use the intersect utility in the CALC menu to determine the points of intersection. Shade the solution of the inequality on the x-axis (if any) in the manner shown in Figures 4 and 8 in the narrative. That is, drop dashed lines from the points of intersection to the axis, then shade and label the solution set on the x-axis. Use set- builder and interval notation (when appropriate) to describe your solution set.
|3−2x| > 5
- Answer
-
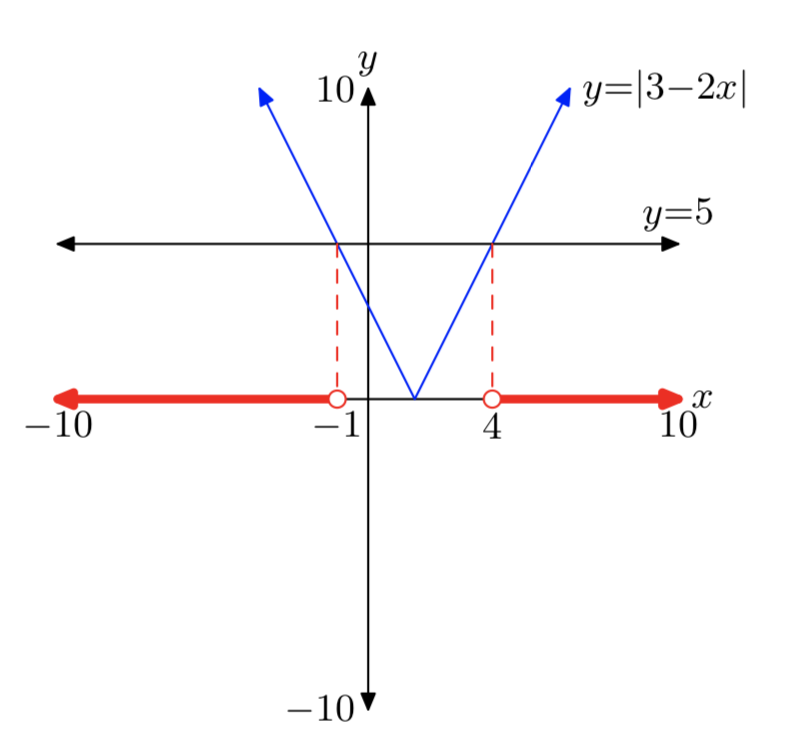
Solution: \((−\infty, −1) \cup (4, \infty)\) = {x : x <−1 or x > 4}.
|2x+7| < 4
|4x+5| < 7
- Answer
-
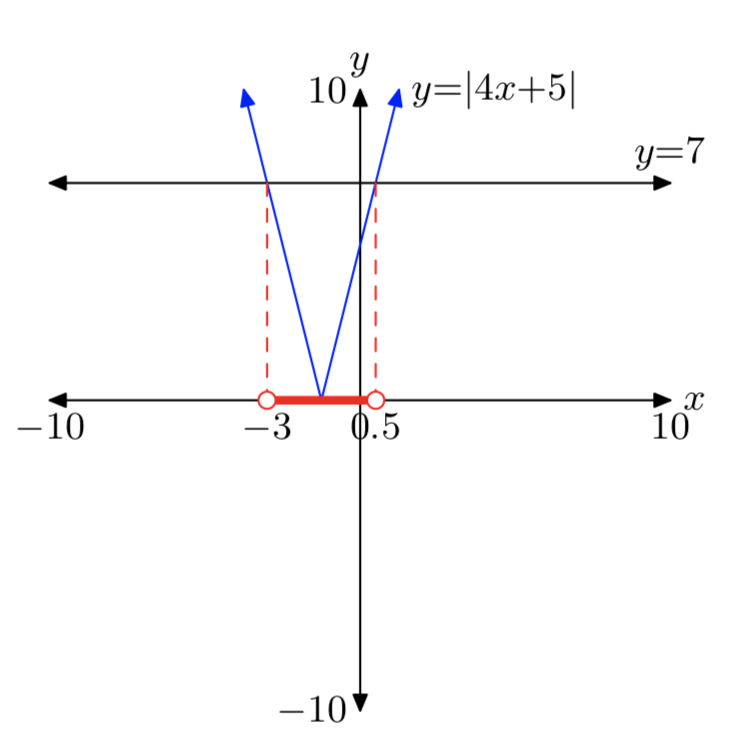
Solution: (−3, 0.5) = {x : −3 < x < 0.5}.
|5x−7| > 8
|4x+5| > −2
- Answer
-
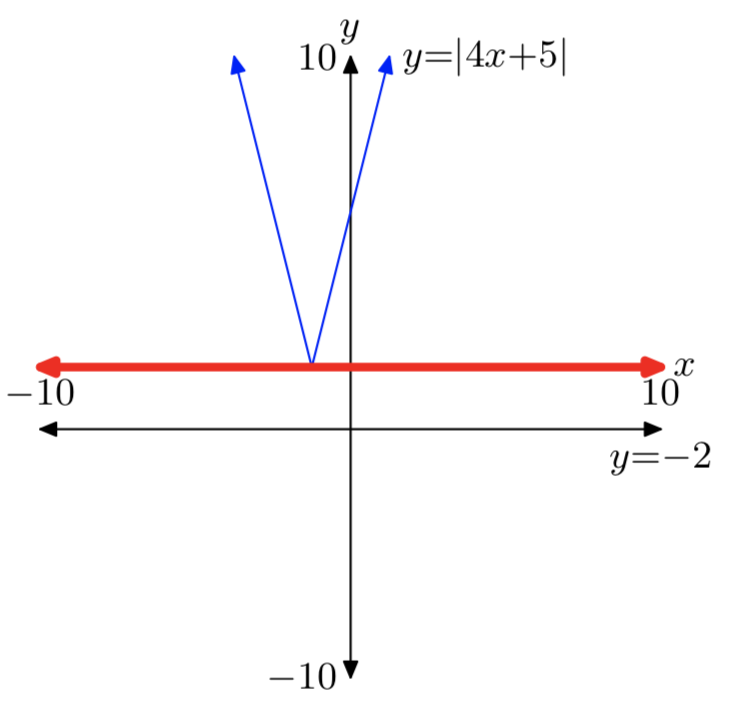
Solution: \(\mathbb{R} = (−\infty, \infty)\)
|3x−5| < −3
\(|2x−9| \ge 6\)
- Answer
-
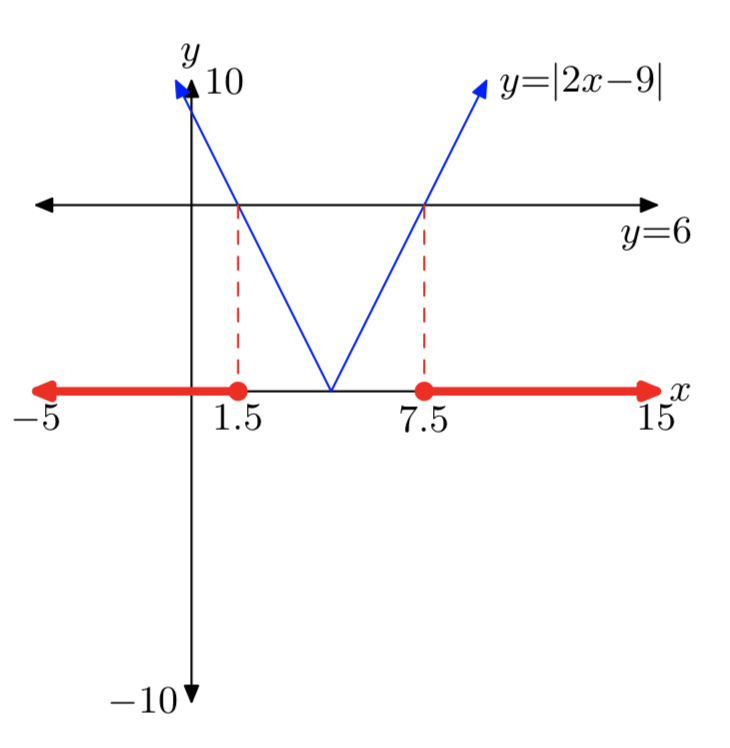
Solution: \((−\infty, 1.5] \cup [7.5, \infty)\) = {x : \(x \ le 1.5 or x \ge 7.5\)}.
\(|3x+25| \ge 8\)
\(|13−2x| \le 7\)
- Answer
-
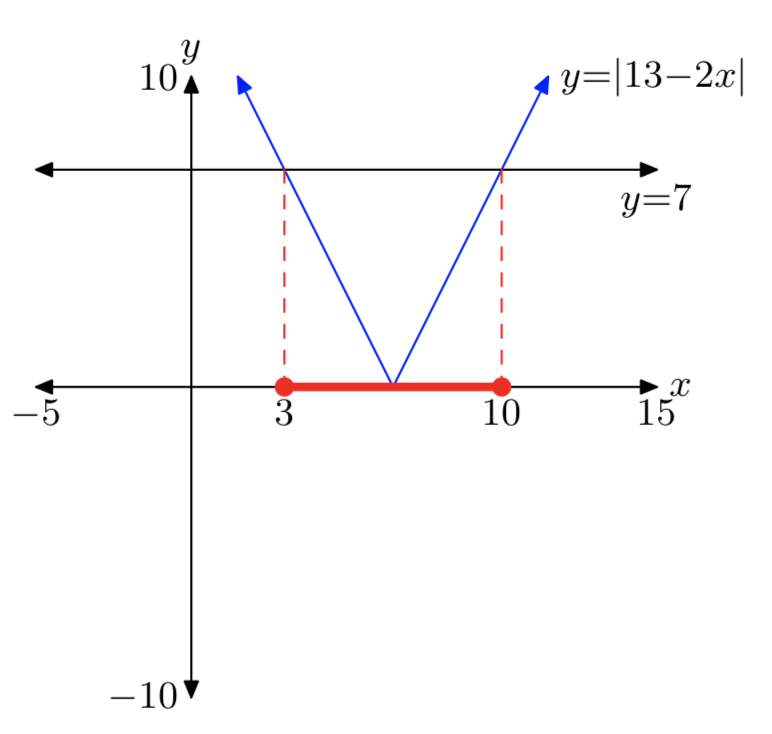
Solution: [3, 10] = {x: \(3 \le x \le 10\)}.
\(|2x+15| \le 7\)
\(|3x−11| > 0\)
- Answer
-
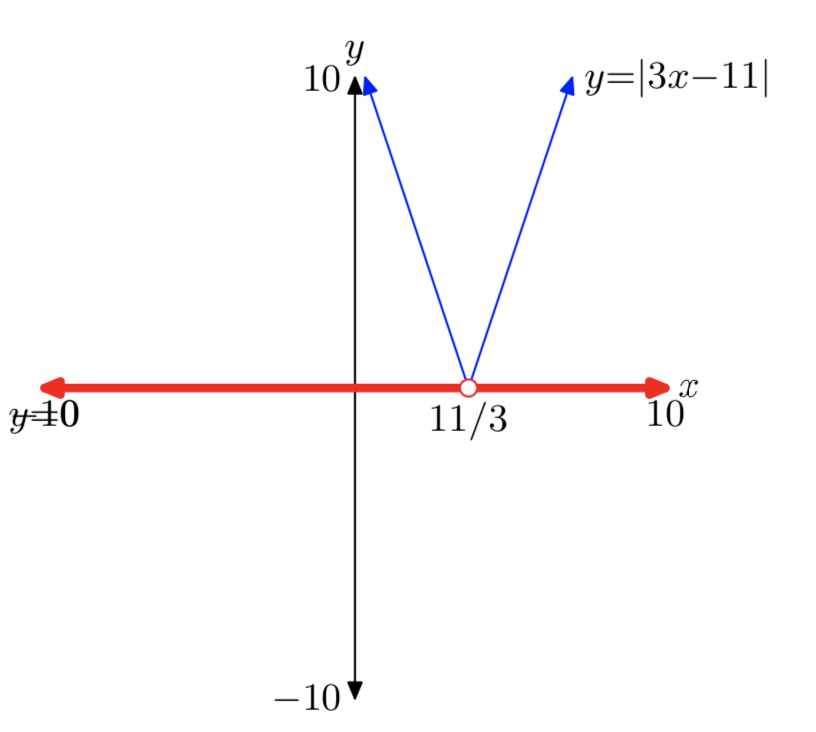
Solution: {x : \(x \ne \frac{11}{3}\)}
\(|4x+19| \le 0\)
For each of the inequalities in Exercises 23-32, provide a purely algebraic solution without the use of a calculator. Show all of your work that leads to the solution, shade your solution set on a number line, then use set-builder and interval notation (if possible) to describe your solution set.
|4x+3| < 8
- Answer
-

(\(−\frac{11}{4}, \frac{5}{4}\)) = {x : \(−\frac{11}{4} < x < \frac{5}{4}\)}
|3x−5| > 11
\(|2x−3| \le 10\)
- Answer
-

[\(−\frac{7}{2}, \frac{13}{2}\)] = {x: \(−\frac{7}{2} \le x \le \frac{13}{2}\)}
\(|3−5x| \ge 15\)
|3x−4| < 7
- Answer
-

(−1, \(\frac{11}{3}\)) = {x: \(−1 < x < \frac{11}{3}\)}
|5−2x| > 10
\(|3−7x| \ge 5\)
- Answer
-

\((−\infty,−\frac{2}{7}] \cup [\frac{8}{7}, \infty)\) = {x : \(x \le −\frac{2}{7}\) or \(x \ge \frac{8}{7}\)}
\(|2−11x| \le 6\)
\(|x+2| \ge −3\)
- Answer
-

\(\mathbb{R} = (−\infty, \infty)\)
|x+5| < −4
For each of the inequalities in Exercises 33-38, perform each of the following tasks.
- Arrange each of the following parts on your homework paper in the same location. Do not do place the algebraic work on one page and the graphical work on another.
- Follow each of the directions given for Exercises 11-22 to find and record a solution with your graphing calculator.
- Provide a purely algebraic solution, showing all the steps of your work. Sketch your solution on a number line, then use set-builder and interval notation to describe your solution set. Do these solutions compare favorably with those found using your graphing calculator in part (2)? If not, look for a mistake in your work.
|x−8| < 7
- Answer
-
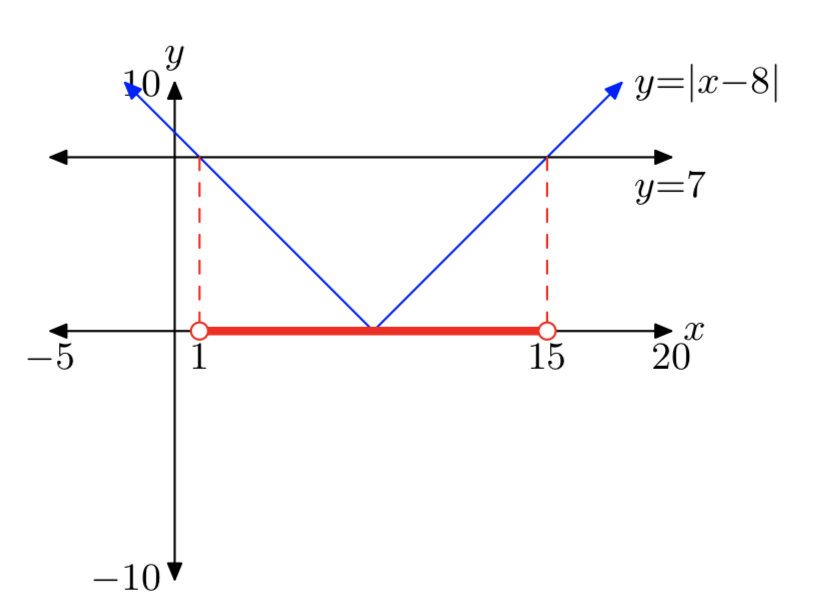
(1, 15) = {x: 1 < x < 15}
|2x−15| > 5
\(|2x+11| \ge 6\)
- Answer
-
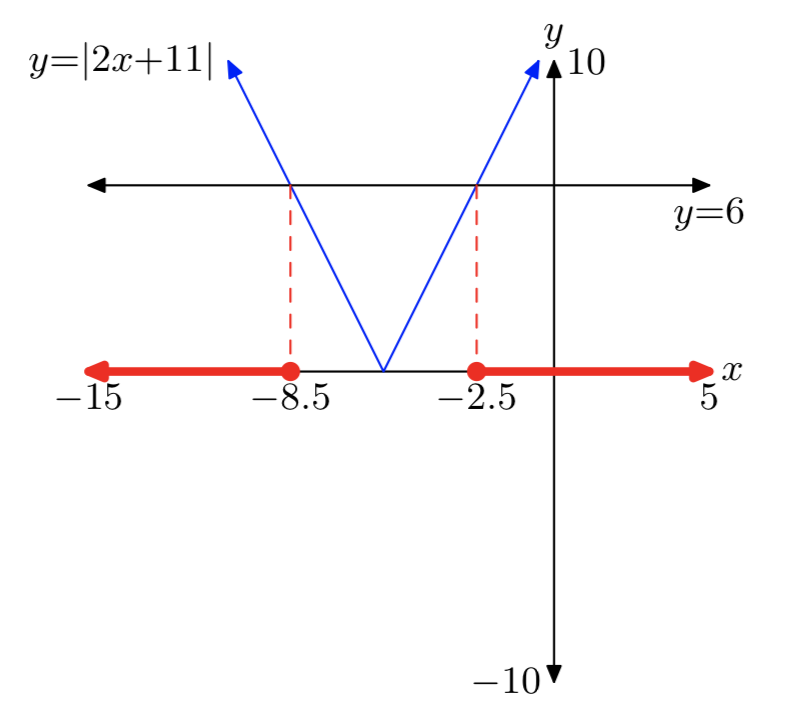
\((−\infty, −8.5] \cup [−2.5, \infty)\) = {x : \(x \le −8.5\) or \(x \ge −2.5\)}
\(|5x−21| \le 7\)
|x−12| > 6
- Answer
-
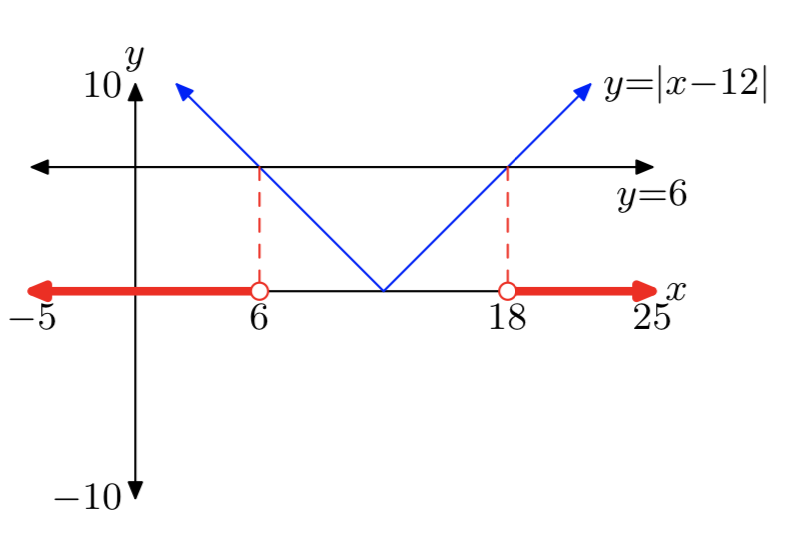
\((−\infty, 6) \cup (18, \infty)\) = {x: x < 6 or x > 18}
|x+11| < 5
Use a strictly algebraic technique to solve each of the equations in Exercises 39-46. Do not use a calculator. Shade the solution set on a number line and describe the solution set using both set-builder and interval notation.
|x+2|−3 > 4
- Answer
-

\((−\infty, −9) \cup (5, \infty)\) = {x: x < −9 or x > 5}
3|x+5| < 6
\(−2|3−2x| \le −6\)
- Answer
-

\((−\infty, 0] \cup [3, \infty)\) = {x: \(x \le 0\) or \(x \ge 3\)}
\(|4−x|+5 \ge 12\)
3|x+2|−5 > |x+2|+7
- Answer
-

\((−\infty, −8) \cup (4, \infty)\) = {x: x < −8 or x > 4}
4−3|4−x| > 2|4−x|−1
\(|\frac{x}{3}−\frac{1}{4}| \le \frac{1}{12}\)
- Answer
-

[\(\frac{1}{2}\), 1] = {x: \(\frac{1}{2} \le x \le 1\)}
\(|\frac{x}{4}−\frac{1}{2}| \ge \frac{2}{3}\)
Use the technique of distance on the number line demonstrated in Examples 21 and 22 to solve each of the inequalities in Exercises 47-50. Provide number line sketches as in Example 17 in the narrative. Describe the solution set using both set-builder and interval notation.
|x−5| < 8
- Answer
-

(−3, 13) = {x : −3 < x < 13}
|x−2| > 4
\(|x+4| \ge 3\)
- Answer
-
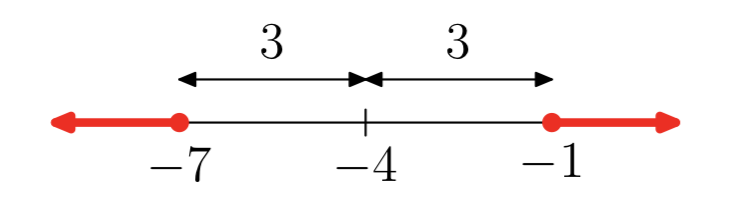
\((−\infty, −7] \cup [−1, \infty)\) = {x: \(x \le −7\) or \(x \ge −1\)}
\(|x+2| \le 11\)
Use the instructions provided in Exercises 11-22 to solve the inequalities in Exercises 51-52. Describe the solution set using both set-builder and interval notation.
\(|x+2| < \frac{1}{3}x+5\)
- Answer
-
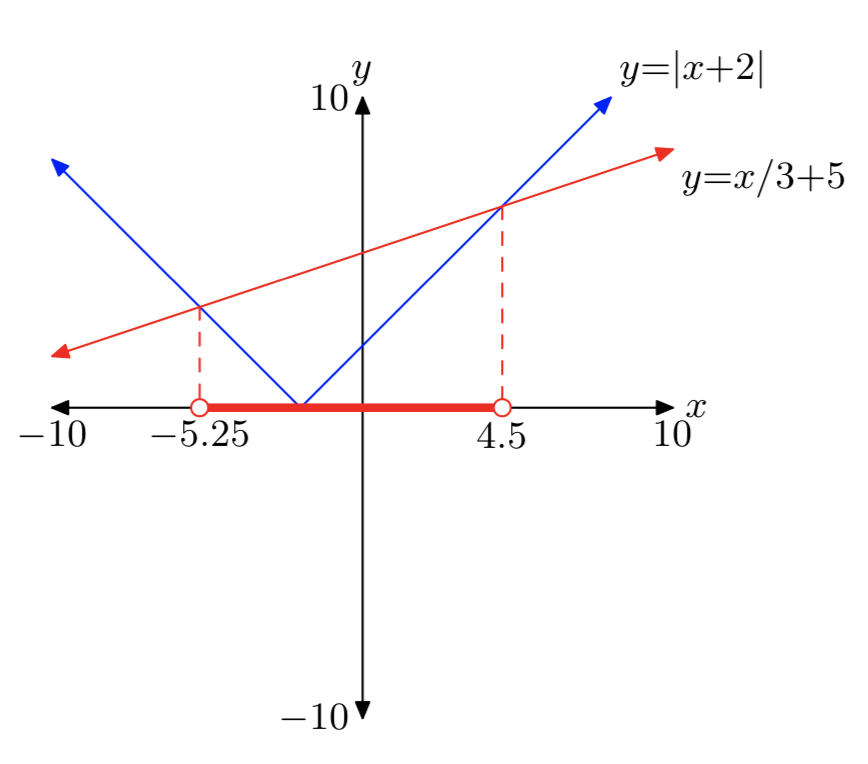
(−5.25, 4.5) = {x : −5.25 < x < 4.5}
\(|x−3| > 5−\frac{1}{2}x\)
In Exercises 53-54, perform each of the following tasks.
- Set up a coordinate system on graph paper. Label and scale each axis.
- Without the use of a calculator,sketch the graphs of the left- and right-hand sides of the given inequality. Label each graph with its equation.
- Shade the solution of the inequality on the x-axis (if any) in the manner shown in Figures 4 and 8 in the narrative. That is, drop dashed lines from the points of intersection to the axis, then shade and label the solution set on the x-axis (you will have to approximate). Describe the solution set using both set-builder and interval notation.
\(|x−2| > \frac{1}{3}x+2\)
- Answer
-
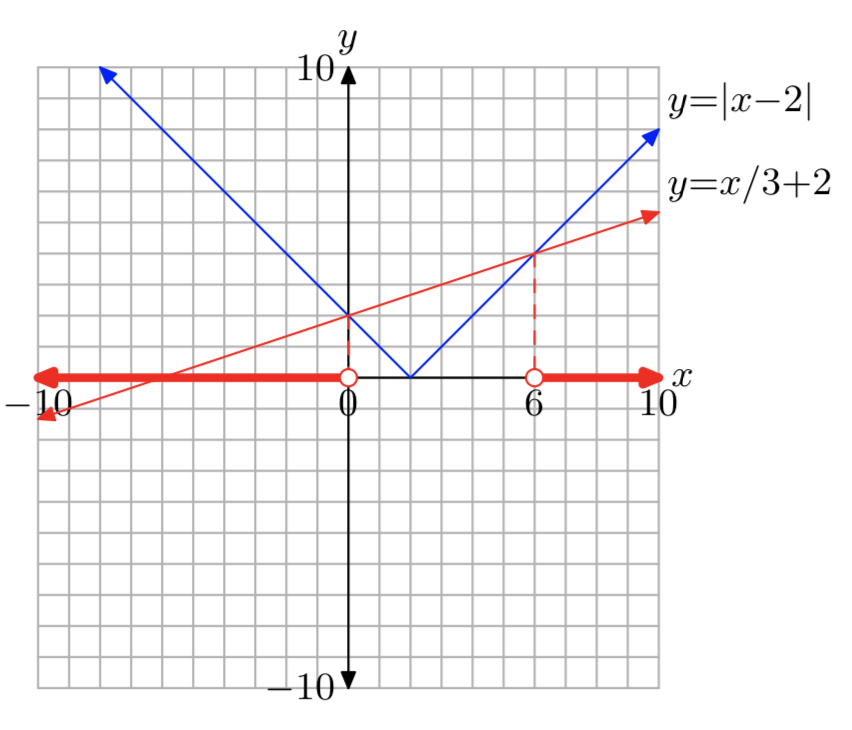
\((−\infty, 0) \cup (6, \infty)\) = {x: x < 0 or x > 6}
\(|x+4| < \frac{1}{3}x+4\)


