7.6: Complex Fractions

In this section we learn how to simplify what are called complex fractions, an example of which follows.

$$\dfrac{\dfrac{1}{2}+\dfrac{1}{3}}{\dfrac{1}{4}+\dfrac{2}{3}} \nonumber$$

Note that both the numerator and denominator are fraction problems in their own right, lending credence to why we refer to such a structure as a “complex fraction.”

There are two very different techniques we can use to simplify the complex fraction (1). The first technique is a “natural” choice.

Simplifying Complex Fractions — First Technique

To simplify a complex fraction, proceed as follows:

1. Simplify the numerator.
2. Simplify the denominator.
3. Simplify the division problem that remains.

Let’s follow this outline to simplify the complex fraction (1). First, add the fractions in the numerator as follows.

$$\dfrac{1}{2}+\dfrac{1}{3}=\dfrac{3}{6}+\dfrac{2}{6}=\dfrac{5}{6} \nonumber$$

Secondly, add the fractions in the denominator as follows.

$$\dfrac{1}{4}+\dfrac{2}{3}=\dfrac{3}{12}+\dfrac{8}{12}=\dfrac{11}{12} \nonumber$$

Substitute the results from (2) and (3) into the numerator and denominator of (1), respectively.

$$\dfrac{\dfrac{1}{2}+\dfrac{1}{3}}{\dfrac{1}{4}+\dfrac{2}{3}}=\dfrac{\dfrac{5}{6}}{\dfrac{11}{12}} \nonumber$$

The right-hand side of (4) is equivalent to

$$\dfrac{5}{6} \div \dfrac{11}{12} \nonumber$$

This is a division problem, so invert and multiply, factor, then cancel common factors.

$$\begin{aligned} \dfrac{\dfrac{1}{2}+\dfrac{1}{3}}{\dfrac{1}{4}+\dfrac{2}{3}} &=\dfrac{5}{6} \cdot \dfrac{12}{11} \\ &=\dfrac{5}{2 \cdot 3} \cdot \dfrac{2 \cdot 2 \cdot 3}{11} \\ &=\dfrac{5}{\not{2} \cdot \not{3}} \cdot \dfrac{\not{2} \cdot 2 \cdot \not{3}}{11} \\ &=\dfrac{10}{11} \end{aligned} \nonumber$$

Here is an arrangement of the work, from start to finish, presented without comment. This is a good template to emulate when doing your homework.

$$\begin{aligned} \dfrac{\dfrac{1}{2}+\dfrac{1}{3}}{\dfrac{1}{4}+\dfrac{2}{3}} &=\dfrac{\dfrac{3}{6}+\dfrac{2}{6}}{\dfrac{3}{12}+\dfrac{8}{12}} \\ &=\dfrac{\dfrac{5}{6}}{\dfrac{11}{12}} \\ &=\dfrac{5}{6} \cdot \dfrac{12}{11} \\ &=\dfrac{5}{2 \cdot 3} \cdot \dfrac{2 \cdot 2 \cdot 3}{11}\\ &=\dfrac{5}{\not{2} \cdot \not{3}} \cdot \dfrac{\not{2} \cdot 2 \cdot \not{3}}{11} \\ &=\dfrac{10}{11} \end{aligned} \nonumber$$

Now, let’s look at a second approach to the problem. We saw that simplifying the numerator in (2) required a common denominator of 6. Simplifying the denominator in (3) required a common denominator of 12. So, let’s choose another common denominator, this one a common denominator for both numerator and denominator, namely, 12. Now, multiply top and bottom (numerator and denominator) of the complex fraction (1) by 12, as follows.

$$\dfrac{\dfrac{1}{2}+\dfrac{1}{3}}{\dfrac{1}{4}+\dfrac{2}{3}}=\dfrac{\left(\dfrac{1}{2}+\dfrac{1}{3}\right) \color{blue}{12}}{\left(\dfrac{1}{4}+\dfrac{2}{3}\right) \color{blue}{12}} \nonumber$$

Distribute the 12 in both numerator and denominator and simplify.

$$\dfrac{\left(\dfrac{1}{2}+\dfrac{1}{3}\right) \color{blue}{12}}{\left(\dfrac{1}{4}+\dfrac{2}{3}\right) \color{blue}{12}}=\dfrac{\left(\dfrac{1}{2}\right) \color{blue}{12}+\left(\dfrac{1}{3}\right) \color{blue}{12}}{\left(\dfrac{1}{4}\right) \color{blue}{12}+\left(\dfrac{2}{3}\right) \color{blue}{12}}=\dfrac{6+4}{3+8}=\dfrac{10}{11} \nonumber$$

Let’s summarize this second technique.

Simplifying Complex Fractions — Second Technique

To simplify a complex fraction, proceed as follows:

1. Find a common denominator for both numerator and denominator.
2. Clear fractions from the numerator and denominator by multiplying each by the common denominator found in the first step.

Note that for this particular problem, the second method is much more efficient. It saves both space and time and is more aesthetically pleasing. It is the technique that we will favor in the rest of this section.

Let’s look at another example.

Example $\PageIndex{1}$

Use both the First and Second Techniques to simplify the expression $$\dfrac{\dfrac{1}{x}-1}{1-\dfrac{1}{x^{2}}} \nonumber$$ State all restrictions.

Solution

Let’s use the first technique, simplifying numerator and denominator separately before dividing. First, make equivalent fractions with a common denominator for the subtraction problem in the numerator of (7) and simplify. Do the same for the denominator.

$$\dfrac{\dfrac{1}{x}-1}{1-\dfrac{1}{x^{2}}}=\dfrac{\dfrac{1}{x}-\dfrac{x}{x}}{\dfrac{x^{2}}{x^{2}}-\dfrac{1}{x^{2}}}=\dfrac{\dfrac{1-x}{x}}{\dfrac{x^{2}-1}{x^{2}}} \nonumber$$

Next, invert and multiply, then factor.

$$\dfrac{\dfrac{1}{x}-1}{1-\dfrac{1}{x^{2}}}=\dfrac{1-x}{x} \cdot \dfrac{x^{2}}{x^{2}-1}=\dfrac{1-x}{x} \cdot \dfrac{x^{2}}{(x+1)(x-1)} \nonumber$$

Let’s invoke the sign change rule and negate two parts of the fraction (1 − x)/x, numerator and fraction bar, then cancel the common factors.

\[\dfrac{\dfrac{1}{x}-1}{1-\dfrac{1}{x^{2}}}=-\dfrac{x-1}{x} \cdot \dfrac{x^{2}}{(x+1)(x-1)}=-\dfrac{x-1}{\not{x}} \cdot \dfrac{x \not{x}}{(x+1)(x-1)} \nonumber \]

Hence,

$$\dfrac{\dfrac{1}{x}-1}{1-\dfrac{1}{x^{2}}}=-\dfrac{x}{x+1} \nonumber$$

Now, let’s try the problem a second time, multiplying numerator and denominator by $x^2$ to clear fractions from both the numerator and denominator.

$$\dfrac{\dfrac{1}{x}-1}{1-\dfrac{1}{x^{2}}}=\dfrac{\left(\dfrac{1}{x}-1\right) \color{blue}{x^{2}}}{\left(1-\dfrac{1}{x^{2}}\right)\color{blue}{x^{2}}}=\dfrac{\left(\dfrac{1}{x}\right) \color{blue}{x^{2}}-(1) \color{blue}{x^{2}}}{(1) \color{blue}{x^{2}}-\left(\dfrac{1}{x^{2}}\right) \color{blue}{x^{2}}}=\dfrac{x-x^{2}}{x^{2}-1} \nonumber$$

The order in the numerator of the last fraction intimates that a sign change would be helpful. Negate the numerator and fraction bar, factor, then cancel common factors.

\[\dfrac{\dfrac{1}{x}-1}{1-\dfrac{1}{x^{2}}}=-\dfrac{x^{2}-x}{x^{2}-1}=-\dfrac{x(x-1)}{(x+1)(x-1)}=-\dfrac{x(x-1)}{(x+1)(x-1)}=-\dfrac{x}{x+1} \nonumber \]

This is precisely the same answer found with the first technique. To list the restrictions, we must make sure that no values of x make any denominator equal to zero, at the beginning of the problem, in the body of our work, or in the final answer.

In the original problem, if x = 0, then both 1/x and $1/x^{2}$ are undefined, so x = 0 is a restriction. In the body of our work, the factors x + 1 and x − 1 found in various denominators make x = −1 and x = 1 restrictions. No other denominators supply restrictions that have not already been listed. Hence, for all x other than −1, 0, and 1, the left-hand side of

$$\dfrac{\dfrac{1}{x}-1}{1-\dfrac{1}{x^{2}}}=-\dfrac{x}{x+1} \nonumber$$

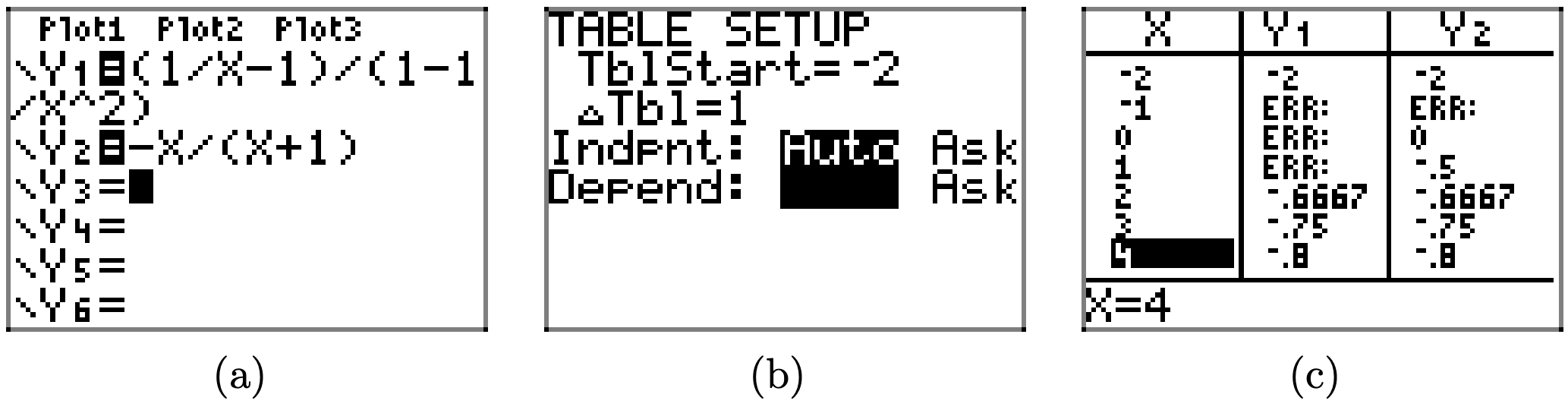
is identical to the right-hand side. Again, the calculator’s table utility provides ample evidence of this fact in the screenshots shown in Figure $\PageIndex{1}$.

Note the ERR (error) messages at each of the restricted values of x, but also note the perfect agreement of Y1 and Y2 at all other values of x.

Let’s look at another example, an important example involving function notation.

Figure $\PageIndex{1}$. Using the table feature of the graphing calculator to check the identity in (8).

Example $\PageIndex{2}$

Given that $$f(x)=\dfrac{1}{x} \nonumber$$ , simplify the expression $$\dfrac{f(x)-f(2)}{x-2} \nonumber$$ . List all restrictions.

Solution

Remember, f(2) means substitute 2 for x. Because f(x) = 1/x, we know that f(2) = 1/2, so

$$\dfrac{f(x)-f(2)}{x-2}=\dfrac{\dfrac{1}{x}-\dfrac{1}{2}}{x-2} \nonumber$$

To clear the fractions from the numerator, we’d use a common denominator of 2x. There are no fractions in the denominator that need clearing, so the common denominator for numerator and denominator is 2x. Multiply numerator and denominator by 2x.

$$\dfrac{f(x)-f(2)}{x-2}=\dfrac{\left(\dfrac{1}{x}-\dfrac{1}{2}\right) \color{blue}{2x}}{(x-2) \color{blue}{2x}}=\dfrac{\left(\dfrac{1}{x}\right) \color{blue}{2x}-\left(\dfrac{1}{2}\right) \color{blue}{2x}}{(x-2) \color{blue}{2x}}=\dfrac{2-x}{2 x(x-2)} \nonumber$$

Negate the numerator and fraction bar, then cancel common factors.

\[\dfrac{f(x)-f(2)}{x-2}=-\dfrac{x-2}{2 x(x-2)}=-\dfrac{x-2}{2 x(x-2)}=-\dfrac{1}{2 x} \nonumber \]

In the original problem, we have a denominator of x − 2, so x = 2 is a restriction. If the body of our work, there is a fraction 1/x, which is undefined when x = 0, so x = 0 is also a restriction. The remaining denominators provide no other restrictions. Hence, for all values of x except 0 and 2, the left-hand side of

$$\dfrac{f(x)-f(2)}{x-2}=-\dfrac{1}{2 x} \nonumber$$

is identical to the right-hand side.

Let’s look at another example involving function notation.

Example $\PageIndex{3}$

Given $$f(x)=\dfrac{1}{x^{2}} \nonumber$$ , simplify the expression $$\dfrac{f(x+h)-f(x)}{h} \nonumber$$ List all restrictions.

Solution

The function notation f(x + h) is asking us to replace each instance of x in the formula 1$/ x^{2}$ with x + h. Thus, $f(x+h)=1 /(x+h)^{2}$.

Here is another way to think of this substitution. Suppose that we remove the x from

$$f(x)=\dfrac{1}{x^{2}} \nonumber$$

so that it reads

$$f( \space)=\dfrac{1}{( \space)^{2}} \nonumber$$

Now, if you want to compute f(2), simply insert a 2 in the blank area between parentheses. In our case, we want to compute f(x + h), so we insert an x + h in the blank space between parentheses in (12) to get

$$f(x+h)=\dfrac{1}{(x+h)^{2}} \nonumber$$

With these preliminary remarks in mind, let’s return to the problem. First, we interpret the function notation as in our preliminary remarks and write

$$\dfrac{f(x+h)-f(x)}{h}=\dfrac{\\(\begin{aligned} \dfrac{f(x+h)-f(x)}{h} &=\dfrac{x^{2}-\left(x^{2}+2 x h+h^{2}\right)}{h x^{2}(x+h)^{2}} \\ &=\dfrac{x^{2}-x^{2}-2 x h-h^{2}}{h x^{2}(x+h)^{2}} \\ &=\dfrac{-2 x h-h^{2}}{h x^{2}(x+h)^{2}} \end{aligned}\)frac{1}{(x+h)^{2}}-\dfrac{1}{x^{2}}}{h} \nonumber$$

The common denominator for the numerator is found by listing each factor to the highest power that it occurs. Hence, the common denominator is $x^{2}(x+h)^{2}$. The denominator has no fractions to be cleared, so it suffices to multiply both numerator and denominator by $x^{2}(x+h)^{2}$.

$$\begin{aligned} \dfrac{f(x+h)-f(x)}{h} &=\dfrac{\left(\dfrac{1}{(x+h)^{2}}-\dfrac{1}{x^{2}}\right) \color{blue}{x^{2}(x+h)^{2}}}{h \color{blue}{x^{2}(x+h)^{2}}} \\ &=\dfrac{\left(\dfrac{1}{(x+h)^{2}}\right) \color{blue}{x^{2}(x+h)^{2}}-\left(\dfrac{1}{x^{2}}\right) \color{blue}{x^{2}(x+h)^{2}}}{h \color{blue}{x^{2}(x+h)^{2}}} \\ &=\dfrac{x^{2}-(x+h)^{2}}{h x^{2}(x+h)^{2}} \end{aligned} \nonumber$$

We will now expand the numerator. Don’t forget to use parentheses and distribute that minus sign.

$$\begin{aligned} \dfrac{f(x+h)-f(x)}{h} &=\dfrac{x^{2}-\left(x^{2}+2 x h+h^{2}\right)}{h x^{2}(x+h)^{2}} \\ &=\dfrac{x^{2}-x^{2}-2 x h-h^{2}}{h x^{2}(x+h)^{2}} \\ &=\dfrac{-2 x h-h^{2}}{h x^{2}(x+h)^{2}} \end{aligned} \nonumber$$

Finally, factor a −h out of the numerator in hopes of finding a common factor to cancel.

$$\begin{aligned} \dfrac{f(x+h)-f(x)}{h} &=\dfrac{-h(2 x+h)}{h x^{2}(x+h)^{2}} \\ &=\dfrac{-\not{h}(2 x+h)}{\not{h} x^{2}(x+h)^{2}} \\ &=\dfrac{-(2 x+h)}{x^{2}(x+h)^{2}} \end{aligned} \nonumber$$

We must now discuss the restrictions. In the original question (11), the h in the denominator must not equal zero. Hence, h = 0 is a restriction. In the final simplified form, the factor of $x^{2}$ in the denominator is undefined if x = 0. Hence, x = 0 is a restriction. Finally, the factor of $(x+h)^{2}$ in the final denominator is undefined if x+h = 0, so x = −h is a restriction. The remaining denominators provide no additional restrictions. Hence, provided $h \neq 0, x \neq 0,$ and $x \neq-h$, for all other combinations of x and h, the left-hand side of

$$\dfrac{f(x+h)-f(x)}{h}=\dfrac{-(2 x+h)}{x^{2}(x+h)^{2}} \nonumber$$

is identical to the right-hand side.

Let’s look at one final example using function notation.

Example $\PageIndex{4}$

If $$f(x)=\dfrac{x}{x+1} \nonumber$$ simplify f(f(x)).

Solution

We first evaluate f at x, then evaluate f at the result of the first computation. Thus, we work the inner function first to obtain

$$f(f(x))=f\left(\dfrac{x}{x+1}\right) \nonumber$$

The notation f(x/(x + 1)) is asking us to replace each occurrence of x in the formula x/(x + 1) with the expression x/(x + 1). Confusing? Here is an easy way to think of this substitution. Suppose that we remove x from

$$f(x)=\dfrac{x}{x+1} \nonumber$$

replacing each occurrence of x with empty parentheses, which will produce the template

$$f(\space )=\dfrac{( \space)}{( \space)+1} \nonumber$$

Now, if asked to compute f(3), simply insert 3 into the blank areas between parentheses. In this case, we want to compute f(x/(x+ 1)), so we insert x/(x+ 1) in the blank space between each set of parentheses in (15) to obtain

$$f\left(\dfrac{x}{x+1}\right)=\dfrac{\dfrac{x}{x+1}}{\dfrac{x}{x+1}+1} \nonumber$$

We now have a complex fraction. The common denominator for both top and bottom of this complex fraction is x + 1. Thus, we multiply both numerator and denominator of our complex fraction by x + 1 and use the distributive property as follows.

$$\dfrac{\dfrac{x}{x+1}}{\dfrac{x}{x+1}+1}=\dfrac{\left(\dfrac{x}{x+1}\right)\color{blue}{(x+1)}}{\left(\dfrac{x}{x+1}+1\right)\color{blue}{(x+1)}}=\dfrac{\left(\dfrac{x}{x+1}\right)\color{blue}{(x+1)}}{\left(\dfrac{x}{x+1}\right)\color{blue}{(x+1)}+(1)\color{blue}{(x+1)}} \nonumber$$

Cancel and simplify.

$$\dfrac{\left(\dfrac{x}{x+1}\right)\color{blue}{(x+1)}}{\left(\dfrac{x}{x+1}\right)\color{blue}{(x+1)}+(1)\color{blue}{(x+1)}}=\dfrac{x}{x+(x+1)}=\dfrac{x}{2 x+1} \nonumber$$

In the final denominator, the value x = −1/2 makes the denominator 2x + 1 equal to zero. Hence, x = −1/2 is a restriction. In the body of our work, several fractions have denominators of x + 1 and are therefore undefined at x = −1. Thus, x = −1 is a restriction. No other denominators add additional restrictions.

Hence, for all values of x, except x = −1/2 and x = −1, the left-hand side of

$$f(f(x))=\dfrac{x}{2 x+1} \nonumber$$

is identical to the right-hand side.

Exercise

In Exercises 1-6, evaluate the function at the given rational number. Then use the first or second technique for simplifying complex fractions explained in the narrative to simplify your answer.

Exercise $\PageIndex{1}$

Given

$f(x) = \frac{x+1}{2−x}$,

evaluate and simplify $f(\frac{1}{2})$.

Answer

1

Exercise $\PageIndex{2}$

Given

$f(x) = \frac{2−x}{x+5}$,

evaluate and simplify $f(\frac{3}{2})$.

Exercise $\PageIndex{3}$

Given

$f(x) = \frac{2x+3}{4−x}$,

evaluate and simplify $f(\frac{1}{3})$.

Answer

1

Exercise $\PageIndex{4}$

Given

$f(x) = \frac{3−2x}{x+5}$

evaluate and simplify $f(\frac{2}{5})$

Exercise $\PageIndex{5}$

Given

$f(x) = \frac{5−2x}{x+4}$,

evaluate and simplify $f(\frac{3}{5})$.

Answer

$\frac{19}{23}$

Exercise $\PageIndex{6}$

Given

$f(x) = \frac{2x−9}{11−x}$,

evaluate and simplify $f(\frac{4}{3})$.

In Exercises 7-46, simplify the given complex rational expression. State all restrictions.

Exercise $\PageIndex{7}$

$\frac{5+\frac{6}{x}}{\frac{25}{x}−\frac{36}{x^3}}$

Answer

Provided $x \ne 0, −\frac{6}{5}, or \frac{6}{5}$,

$\frac{x^2}{5x−6}$.

Exercise $\PageIndex{8}$

$\frac{7+\frac{9}{x}}{\frac{49}{x}−\frac{81}{x^3}}$

Exercise $\PageIndex{9}$

$\frac{\frac{7}{x−2}−\frac{5}{x−7}}{\frac{8}{x−7}+\frac{3}{x+8}}$

Answer

Provided $x \ne 2, 7, −8, or −\frac{43}{11}$,

$\frac{(2x−39)(x+8)}{(11x+43)(x−2)}$

Exercise $\PageIndex{10}$

$\frac{\frac{9}{x+4}−\frac{7}{x−9}}{\frac{9}{x−9}+\frac{5}{x−4}}$

Exercise $\PageIndex{11}$

$\frac{3+\frac{7}{x}}{\frac{9}{x^2}−\frac{49}{x^4}}$

Answer

Provided $x \ne 0, −\frac{7}{3}, or \frac{7}{3}$,

$\frac{x^3}{3x−7}$.

Exercise $\PageIndex{12}$

$\frac{2−\frac{5}{x}}{\frac{4}{x^2}−\frac{25}{x^4}}$

Exercise $\PageIndex{13}$

$\frac{\frac{9}{x+4}+\frac{7}{x+9}}{\frac{9}{x+9}+\frac{2}{x−8}}$

Answer

Provided $x \ne −4, −9, 8, or \frac{54}{11}$,

$\frac{(16x+109)(x−8)}{(11x−54)(x+4)}$

Exercise $\PageIndex{14}$

$\frac{\frac{4}{x−6}+\frac{9}{x−9}}{\frac{9}{x−6}+\frac{8}{x−9}}$

Exercise $\PageIndex{15}$

$\frac{\frac{5}{x−7}−\frac{4}{x−4}}{\frac{10}{x−4}−\frac{5}{x+2}}$

Answer

Provided $x \ne 7, 4, −2, or −8$,

$\frac{x+2}{5(x−7)}$

Exercise $\PageIndex{16}$

$\frac{\frac{3}{x+6}+\frac{7}{x+9}}{\frac{9}{x+6}−\frac{4}{x+9}}$

Exercise $\PageIndex{17}$

$\frac{\frac{6}{x−3}+\frac{5}{x−8}}{\frac{9}{x−3}+\frac{7}{x−8}}$

Answer

Provided $x \ne 3, 8, or \frac{93}{16}$

$\frac{11x−63}{16x−93}$

Exercise $\PageIndex{18}$

$\frac{\frac{7}{x−7}−\frac{4}{x−2}}{\frac{7}{x−7}−\frac{6}{x−2}}$

Exercise $\PageIndex{19}$

$\frac{\frac{4}{x−2}+\frac{7}{x−7}}{\frac{5}{x−2}+\frac{2}{x−6}}$

Answer

Provided $x \ne 2, 7, or \frac{39}{7}$,

$\frac{11x−42}{7x−39}$

Exercise $\PageIndex{20}$

$\frac{\frac{9}{x+2}−\frac{7}{x+5}}{\frac{4}{x+2}+\frac{3}{x+5}}$

Exercise $\PageIndex{21}$

$\frac{5+\frac{4}{x}}{\frac{25}{x}−\frac{16}{x^3}}$

Answer

Provided $x \ne 0, −\frac{4}{5}, or \frac{4}{5}$,

$\frac{x^2}{5x−4}$.

Exercise $\PageIndex{22}$

$\frac{\frac{6}{x+5}+\frac{5}{x+4}}{\frac{8}{x+5}−\frac{3}{x+4}}$

Exercise $\PageIndex{23}$

$\frac{\frac{9}{x−5}+\frac{8}{x+4}}{\frac{5}{x−5}−\frac{4}{x+4}}$

Answer

Provided $x \ne 5, −4, or −40$,

$\frac{17x−4}{x+40}$.

Exercise $\PageIndex{24}$

$\frac{\frac{4}{x−6}+\frac{4}{x−9}}{\frac{6}{x−6}+\frac{6}{x−9}}$

Exercise $\PageIndex{25}$

$\frac{\frac{6}{x+8}+\frac{5}{x−2}}{\frac{5}{x−2}−\frac{2}{x+2}}$

Answer

Provided $x \ne −8, 2, −2, or −\frac{14}{3}$,

$\frac{(11x+28)(x+2)}{(3x+14)(x+8)}$.

Exercise $\PageIndex{26}$

$\frac{\frac{7}{x+9}+\frac{9}{x−2}}{\frac{4}{x−2}+\frac{7}{x+1}}$

Exercise $\PageIndex{27}$

$\frac{\frac{7}{x+7}−\frac{5}{x+4}}{\frac{8}{x+7}−\frac{3}{x+4}}$

Answer

Provided $x \ne −7, −4, or −\frac{11}{5}$,

$\frac{2x−7}{5x+11}$.

Exercise $\PageIndex{28}$

$\frac{25−\frac{16}{x^2}}{5+\frac{4}{x}}$

Exercise $\PageIndex{29}$

$\frac{\frac{64}{x}−\frac{25}{x^3}}{8−\frac{5}{x}}$

Answer

Provided $x \ne 0 or \frac{5}{8}$,

$\frac{8x+5}{x^2}$.

Exercise $\PageIndex{30}$

$\frac{\frac{4}{x+2}+\frac{5}{x−6}}{\frac{7}{x−6}−\frac{5}{x+7}}$

Exercise $\PageIndex{31}$

$\frac{\frac{2}{x−6}−\frac{4}{x+9}}{\frac{3}{x−6​​​​​​​}−\frac{6}{x+9}}$

Answer

Provided $x \ne 6, −9, or 21$,

$\frac{2}{3}$.

Exercise $\PageIndex{32}$

$\frac{\frac{3}{x+6​​​​​​​}−\frac{4}{x+4}}{\frac{6}{x+6}−\frac{8}{x+4}}$

Exercise $\PageIndex{33}$

$\frac{\frac{9}{x^2}−\frac{64}{x^4}}{3−\frac{8}{x}}$

Answer

Provided $x \ne 0 or \frac{8}{3}$,

$\frac{3x+8}{x^3}$.

Exercise $\PageIndex{34}$

$\frac{\frac{9}{x^2}−\frac{25}{x^4}}{3−\frac{5}{x}}$

Exercise $\PageIndex{35}$

$\frac{\frac{4}{x−4​​​​​​​}−\frac{8}{x−7}}{\frac{4}{x−7}+\frac{2}{x+2}}$

Answer

Provided $x \ne 4, 7, −2, or 1$,

$\frac{−2(x+2)}{3(x−4)}$.

Exercise $\PageIndex{36}$

$\frac{2−\frac{7}{x}}{4−\frac{49}{x^2}}$

Exercise $\PageIndex{37}$

$\frac{\frac{3}{x^2+8x−9}+\frac{3}{x^2−81}}{\frac{9}{x^2−81}+\frac{9}{x^2−8x−9}}$

Answer

Provided $x \ne 1, −9, 9, −1, −5$,

$\frac{(x−5)(x+1)}{3(x+5)(x−1)}$

Exercise $\PageIndex{38}$

$\frac{\frac{7}{x^2−5x−14}+\frac{2}{x^2−7x−18}}{\frac{5}{x^2−7x−18}+\frac{8}{x^2−6x−27}}$

Exercise $\PageIndex{39}$

$\frac{\frac{2}{x^2+8x+7}+\frac{5}{x^2+13x+42}}{\frac{7}{x^2+13x+42}+\frac{6}{x^2+3x−18}}$

Answer

Provided $x \ne −1, −7, −6, 3, −\frac{21}{13}$,

$\frac{(7x+17)(x−3)}{(13x+21)(x+1)}$

Exercise $\PageIndex{40}$

$\frac{\frac{3}{x^2+5x−14}+\frac{3}{x^2−7x−98}}{\frac{3}{x^2−7x−98}+\frac{3}{x^2−15x+14}}$

Exercise $\PageIndex{41}$

\(\frac{\frac{6}{x^2+11x+24}−\frac{6}{x^2+13+40}}{\frac{9}{x^2+13x+40}−{\frac{9}{x^2−3x−40}}\)

Answer

Provided $x \ne −3, −8, −5, 8$,

$\frac{−1(x−8)}{12(x+3)}$

Exercise $\PageIndex{42}$

$\frac{\frac{7}{x^2+19x+90}+\frac{7}{x^2+19x+90}}{\frac{9}{x^2+19x+90}+\frac{9}{x^2+7x−18}}$

Exercise $\PageIndex{43}$

$\frac{\frac{7}{x^2−6x+5}+\frac{7}{x^2+2x−35}}{\frac{8}{x^2+2x−35}+\frac{8}{x^2+8x+7}}$

Answer

Provided $x \ne 1, 5, −7, −1, 2$,

$\frac{7(x+3)(x+1)}{8(x−2)(x−1)}$

Exercise $\PageIndex{44}$

$\frac{\frac{2}{x^2−4x−12​​​​​​​}−\frac{2}{x^2−x−30}}{\frac{2}{x^2−x−30}−\frac{2}{x^2−4x−45}}$

Exercise $\PageIndex{45}$

$\frac{\frac{4}{x^2 +6x−7​​​​​​​}−\frac{4}{x^2+2x−3}}{\frac{4}{x^2+2x−3}​−\frac{4}{x^2+5x+6}}$

Answer

Provided $x \ne −7, 1, −3, −2$,

$\frac{−4(x+2)}{3(x+7)}$

Exercise $\PageIndex{46}$

$\frac{\frac{9}{x^2+3x−4}+\frac{8}{x^2−7x+6}}{\frac{4}{x^2−7x+6}+\frac{9}{x^2−10x+24}}$

Exercise $\PageIndex{47}$

Given $f(x) = \frac{2}{x}$, simplify​​​​​​​

$\frac{f(x)−f(3)}{x−3}$.

State all restrictions.

Answer

Provided $x \ne 0, 3$,

$\frac{−2}{3x}$

Exercise $\PageIndex{48}$

Given $f(x) = \frac{5}{x}$, simplify​​​​​​​

$\frac{f(x)−f(2)}{x−2}$.

State all restrictions.

Exercise $\PageIndex{49}$

Given $f(x) = \frac{3}{x^2}$, simplify​​​​​​​

$\frac{f(x)−f(1)}{x−1}$.

State all restrictions.

Answer

Provided $x \ne 0, 1$,

$−\frac{3(x+1)}{x^2}$

Exercise $\PageIndex{50}$

Given $f(x) = \frac{5}{x^2}$, simplify​​​​​​​

$\frac{f(x)−f(2)}{x−2}$.

State all restrictions.

Exercise $\PageIndex{51}$

Given $f(x) = \frac{7}{x}$, simplify​​​​​​​

$\frac{f(x+h)−f(x)}{h}$.

State all restrictions.

Answer

Provided $x \ne 0, −h$, and $h \ne 0$,

$−\frac{7}{h(x+h)}$

Exercise $\PageIndex{52}$

Given $f(x) = \frac{4}{x}$, simplify​​​​​​​

$\frac{f(x+h)−f(x)}{h}$.

State all restrictions.

Exercise $\PageIndex{53}$

Given

$f(x) = \frac{x+1}{3−x}$,

find and simplify $f(\frac{1}{x})$. State all restrictions.

Answer

Provided $x \ne 0, \frac{1}{3}$,

$\frac{x+1}{3x−1}$

Exercise $\PageIndex{54}$

Given

$f(x) = \frac{2−x}{3x+4}$

find and simplify $f(\frac{2}{x})$. State all restriction.​​​​​​​

Exercise $\PageIndex{55}$

Given

$f(x) = \frac{x+1}{2−5x}$,

find and simplify $f(\frac{5}{x})$. State all restrictions.

Answer

Provided $x \ne 0, \frac{25}{2}$,

$\frac{x+5}{2x−25}$

Exercise $\PageIndex{56}$

Given

$f(x) = \frac{2x−3}{4+x}$,

find and simplify $f(\frac{1}{x})$. State all restrictions.

Exercise $\PageIndex{57}$

Given

$f(x) = \frac{x}{x+2}$,

find and simplify f(f(x)). State all restrictions.

Answer

Provided $x \ne −2, −\frac{4}{3}$,

$\frac{x}{3x+4}$

Exercise $\PageIndex{58}$

Given

$f(x) = \frac{2x}{x+5}$

find and simplify f(f(x)). State all restrictions.

​​​​​​​