You do not have permission to view this page - please try signing in.

5.5E: Exercises

Last updated

Feb 14, 2022
Save as PDF
- 5.5: Dividing Polynomials
- Chapter 5 Review Exercises

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\id}{\mathrm{id}}$ $\newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$ $\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$ $\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\id}{\mathrm{id}}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\kernel}{\mathrm{null}\,}$

$\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$

$\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$

$\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$ $\newcommand{\AA}{\unicode[.8,0]{x212B}}$

$\newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$\newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$\newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vectorC}[1]{\textbf{#1}}$

$\newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$\newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$\newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}}$

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\avec}{\mathbf a}$

$\newcommand{\bvec}{\mathbf b}$

$\newcommand{\cvec}{\mathbf c}$

$\newcommand{\dvec}{\mathbf d}$

$\newcommand{\dtil}{\widetilde{\mathbf d}}$

$\newcommand{\evec}{\mathbf e}$

$\newcommand{\fvec}{\mathbf f}$

$\newcommand{\nvec}{\mathbf n}$

$\newcommand{\pvec}{\mathbf p}$

$\newcommand{\qvec}{\mathbf q}$

$\newcommand{\svec}{\mathbf s}$

$\newcommand{\tvec}{\mathbf t}$

$\newcommand{\uvec}{\mathbf u}$

$\newcommand{\vvec}{\mathbf v}$

$\newcommand{\wvec}{\mathbf w}$

$\newcommand{\xvec}{\mathbf x}$

$\newcommand{\yvec}{\mathbf y}$

$\newcommand{\zvec}{\mathbf z}$

$\newcommand{\rvec}{\mathbf r}$

$\newcommand{\mvec}{\mathbf m}$

$\newcommand{\zerovec}{\mathbf 0}$

$\newcommand{\onevec}{\mathbf 1}$

$\newcommand{\real}{\mathbb R}$

$\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$

$\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$

$\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$

$\newcommand{\laspan}[1]{\text{Span}\{#1\}}$

$\newcommand{\bcal}{\cal B}$

$\newcommand{\ccal}{\cal C}$

$\newcommand{\scal}{\cal S}$

$\newcommand{\wcal}{\cal W}$

$\newcommand{\ecal}{\cal E}$

$\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$

$\newcommand{\gray}[1]{\color{gray}{#1}}$

$\newcommand{\lgray}[1]{\color{lightgray}{#1}}$

$\newcommand{\rank}{\operatorname{rank}}$

$\newcommand{\row}{\text{Row}}$

$\newcommand{\col}{\text{Col}}$

$\renewcommand{\row}{\text{Row}}$

$\newcommand{\nul}{\text{Nul}}$

$\newcommand{\var}{\text{Var}}$

$\newcommand{\corr}{\text{corr}}$

$\newcommand{\len}[1]{\left|#1\right|}$

$\newcommand{\bbar}{\overline{\bvec}}$

$\newcommand{\bhat}{\widehat{\bvec}}$

$\newcommand{\bperp}{\bvec^\perp}$

$\newcommand{\xhat}{\widehat{\xvec}}$

$\newcommand{\vhat}{\widehat{\vvec}}$

$\newcommand{\uhat}{\widehat{\uvec}}$

$\newcommand{\what}{\widehat{\wvec}}$

$\newcommand{\Sighat}{\widehat{\Sigma}}$

$\newcommand{\lt}{<}$

$\newcommand{\gt}{>}$

$\newcommand{\amp}{&}$

$\definecolor{fillinmathshade}{gray}{0.9}$

Practice Makes Perfect

Divide Monomials

In the following exercises, divide the monomials.

1. $15r^4s^9÷(15r^4s^9)$

2. $20m^8n^4÷(30m^5n^9)$

Answer: $\dfrac{2m^3}{3n^5}$

3. $\dfrac{18a^4b^8}{−27a^9b^5}$

4. $\dfrac{45x^5y^9}{−60x^8y^6}$

Answer: $\dfrac{−3y^3}{4x^3}$

5. $\dfrac{(10m^5n^4)(5m^3n^6)}{25m^7n^5}$

6. $\dfrac{(−18p^4q^7)(−6p^3q^8)}{−36p^{12}q^{10}}$

Answer: $\dfrac{−3q^5}{p^5}$

7. $\dfrac{(6a^4b^3)(4ab^5)}{(12a^2b)(a^3b)}$

8. $\dfrac{(4u^2v^5)(15u^3v)}{(12u^3v)(u^4v)}$

Answer: $\dfrac{5v^4}{u^2}$

Divide a Polynomial by a Monomial

In the following exercises, divide each polynomial by the monomial.

9. $(9n^4+6n^3)÷3n$

10. $(8x^3+6x^2)÷2x$

Answer: $4x^2+3x$

11. $(63m^4−42m^3)÷(−7m^2)$

12. $(48y^4−24y^3)÷(−8y^2)$

Answer: $−6y^2+3y$

13. $\dfrac{66x^3y^2−110x^2y^3−44x^4y^3}{11x^2y^2}$

14. $\dfrac{72r^5s^2+132r^4s^3−96r^3s^5}{12r^2s^2}$

Answer: $6r^3+11r^2s−8rs^3$

15. $10x^2+5x−4−5x$

16. $20y^2+12y−1−4y$

Answer: $−5y−3+\dfrac{1}{4y}$

Divide Polynomials using Long Division

In the following exercises, divide each polynomial by the binomial.

17. $(y^2+7y+12)÷(y+3)$

18. $(a^2−2a−35)÷(a+5)$

Answer: $a−7$

19. $(6m^2−19m−20)÷(m−4)$

20. $(4x^2−17x−15)÷(x−5)$

Answer: $4x+3$

21. $(q^2+2q+20)÷(q+6)$

22. $(p^2+11p+16)÷(p+8)$

Answer: $p+3−\dfrac{8}{p+8}$

23. $(3b^3+b^2+4)÷(b+1)$

24. $(2n^3−10n+28)÷(n+3)$

Answer: $\dfrac{2n^2−6n+8+4}{n+3}$

25. $(z^3+1)÷(z+1)$

26. $(m^3+1000)÷(m+10)$

Answer: $m^2−10m+100$

27. $(64x^3−27)÷(4x−3)$

28. $(125y^3−64)÷(5y−4)$

Answer: $25y^2+20x+16$

Divide Polynomials using Synthetic Division

In the following exercises, use synthetic Division to find the quotient and remainder.

29. $x^3−6x^2+5x+14$ is divided by $x+1$

30. $x^3−3x^2−4x+12$ is divided by $x+2$

Answer: $x^2−5x+6; \space 0$

31. $2x^3−11x^2+11x+12$ is divided by $x−3$

32. $2x^3−11x^2+16x−12$ is divided by $x−4$

Answer: $2x^2−3x+4; \space 4$

33. $x^4-5x^2+2+13x+3$ is divided by $x+3$

34. $x^4+x^2+6x−10$ is divided by $x+2$

Answer: $x^3−2x^2+5x−4; \space −2$

35. $2x^4−9x^3+5x^2−3x−6$ is divided by $x−4$

36. $3x^4−11x^3+2x^2+10x+6$ is divided by $x−3$

Answer: $3x^3−2x^2−4x−2;\space 0$

Divide Polynomial Functions

In the following exercises, divide.

37. For functions $f(x)=x^2−13x+36$ and $g(x)=x−4$ , find ⓐ $\left(\dfrac{f}{g}\right)(x)$ ⓑ $\left(\dfrac{f}{g}\right)(−1)$

38. For functions $f(x)=x^2−15x+54$ and $g(x)=x−9$ , find ⓐ $\left(\dfrac{f}{g}\right)(x)$ ⓑ $\left(\dfrac{f}{g}\right)(−5)$

Answer: ⓐ $\left(\dfrac{f}{g}\right)(x)=x−6$
ⓑ $\left(\dfrac{f}{g}\right)(−5)=−11$

39. For functions $f(x)=x^3+x^2−7x+2$ and $g(x)=x−2$ , find ⓐ $\left(\dfrac{f}{g}\right)(x)$ ⓑ $\left(\dfrac{f}{g}\right)(2)$

40. For functions $f(x)=x^3+2x^2−19x+12$ and $g(x)=x−3$ , find ⓐ $\left(\dfrac{f}{g}\right)(x)$ ⓑ $\left(\dfrac{f}{g}\right)(0)$

Answer: ⓐ $\left(\dfrac{f}{g}\right)(x)=x^2+5x−4$
ⓑ $\left(\dfrac{f}{g}\right)(0)=−4$

41. For functions $f(x)=x^2−5x+2$ and $g(x)=x^2−3x−1$ , find ⓐ $(f·g)(x)$ ⓑ $(f·g)(−1)$

42. For functions $f(x)=x^2+4x−3$ and $g(x)=x^2+2x+4$ , find ⓐ $(f·g)(x)$ ⓑ $(f·g)(1)$

Answer: ⓐ $(f·g)(x)=x^4+6x^3+9x^2+10x−12$ ; ⓑ $(f·g)(1)=14$

Use the Remainder and Factor Theorem

In the following exercises, use the Remainder Theorem to find the remainder.

43. $f(x)=x^3−8x+7$ is divided by $x+3$

44. $f(x)=x^3−4x−9$ is divided by $x+2$

Answer: $−9$

45. $f(x)=2x^3−6x−24$ divided by $x−3$

46. $f(x)=7x^2−5x−8$ divided by $x−1$

Answer: $−6$

In the following exercises, use the Factor Theorem to determine if x−cx−c is a factor of the polynomial function.

47. Determine whether $x+3$ a factor of $x^3+8x^2+21x+18$

48. Determine whether $x+4$ a factor of $x^3+x^2−14x+8$

Answer: no

49. Determine whether $x−2$ a factor of $x^3−7x^2+7x−6$

50. Determine whether $x−3$ a factor of $x^3−7x^2+11x+3$

Answer: yes

Writing Exercises

51. James divides $48y+6$ by $6$ this way: $\dfrac{48y+6}{6}=48y$ . What is wrong with his reasoning?

52. Divide $\dfrac{10x^2+x−12}{2x}$ and explain with words how you get each term of the quotient.

Answer: Answer will vary

53. Explain when you can use synthetic division.

54. In your own words, write the steps for synthetic division for $x^2+5x+6$ divided by $x−2$ .

Answer: Answers will vary.

Self Check

a. After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section

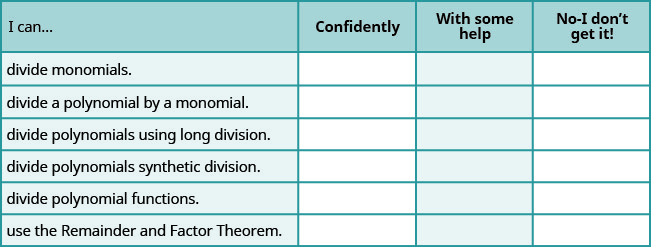
$The figure shows a table with seven rows and four columns. The first row is a header row and it labels each column. The first column header is “I can…”, the second is "confidently", the third is “with some help”, “no minus I don’t get it!”. Under the first column are the phrases “divide monomials”, “divide a polynomial by using a monomial”, “divide polynomials using long division”, “divide polynomials using synthetic division”, “divide polynomial functions”, and “use the Remainder and Factor Theorem”. Under the second, third, fourth columns are blank spaces where the learner can check what level of mastery they have achieved.$

b. On a scale of 1-10, how would you rate your mastery of this section in light of your responses on the checklist? How can you improve this?

Search

Text Color

Text Size

Margin Size

Font Type

Practice Makes Perfect

Writing Exercises

Self Check

Support Center

How can we help?