10.2E: Exercises
- Page ID
- 30568
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Practice Makes Perfect
In the following exercises, find
- \((f \circ g)(x)\)
- \((g \circ f)(x)\)
- \((f \cdot g)(x)\)
- \(f(x)=4 x+3\) and \(g(x)=2 x+5\)
- \(f(x)=3 x-1\) and \(g(x)=5 x-3\)
- \(f(x)=6 x-5\) and \(g(x)=4 x+1\)
- \(f(x)=2 x+7\) and \(g(x)=3 x-4\)
- \(f(x)=3 x\) and \(g(x)=2 x^{2}-3 x\)
- \(f(x)=2 x\) and \(g(x)=3 x^{2}-1\)
- \(f(x)=2 x-1\) and \(g(x)=x^{2}+2\)
- \(f(x)=4 x+3\) and \(g(x)=x^{2}-4\)
- Answer
-
1.
- \(8x+23\)
- \(8x+11\)
- \(8 x^{2}+26 x+15\)
3.
- \(24x+1\)
- \(24x-19\)
- \(24x^{2}+19x-5\)
5.
- \(6 x^{2}-9 x\)
- \(18 x^{2}-9 x\)
- \(6 x^{3}-9 x^{2}\)
7.
- \(2 x^{2}+3\)
- \(4 x^{2}-4 x+3\)
- \(2 x^{3}-x^{2}+4 x-2\)
In the following exercises, find the values described.
- For functions \(f(x)=2 x^{2}+3\) and \(g(x)=5x-1\), find
- \((f \circ g)(-2)\)
- \((g \circ f)(-3)\)
- \((f \circ f)(-1)\)
- For functions \(f(x)=5 x^{2}-1\) and \(g(x)=4x−1\), find
- \((f \circ g)(1)\)
- \((g \circ f)(-1)\)
- \((f \circ f)(2)\)
- For functions \(f(x)=2x^{3}\) and \(g(x)=3x^{2}+2\), find
- \((f \circ g)(-1)\)
- \((g \circ f)(1)\)
- \((g \circ g)(1)\)
- For functions \(f(x)=3 x^{3}+1\) and \(g(x)=2 x^{2}=3\), find
- \((f \circ g)(-2)\)
- \((g \circ f)(-1)\)
- \((g \circ g)(1)\)
- Answer
-
1.
- \(245\)
- \(104\)
- \(53\)
3.
- \(250\)
- \(14\)
- \(77\)
In the following exercises, determine if the set of ordered pairs represents a function and if so, is the function one-to-one.
- \(\begin{array}{l}{\{(-3,9),(-2,4),(-1,1),(0,0)}, {(1,1),(2,4),(3,9) \}}\end{array}\)
- \(\begin{array}{l}{\{(9,-3),(4,-2),(1,-1),(0,0)}, {(1,1),(4,2),(9,3) \}}\end{array}\)
- \(\begin{array}{l}{\{(-3,-5),(-2,-3),(-1,-1)}, {(0,1),(1,3),(2,5),(3,7) \}}\end{array}\)
- \(\begin{array}{l}{\{(5,3),(4,2),(3,1),(2,0)}, {(1,-1),(0,-2),(-1,-3) \}}\end{array}\)
- Answer
-
1. Function; not one-to-one
3. One-to-one function
In the following exercises, determine whether each graph is the graph of a function and if so, is it one-to-one.
1.
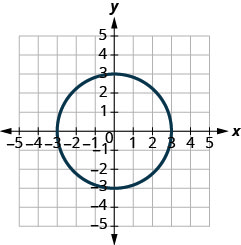
Figure 10.1.65
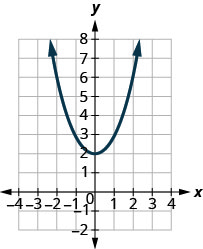
Figure 10.1.66
2.
Figure 10.1.67
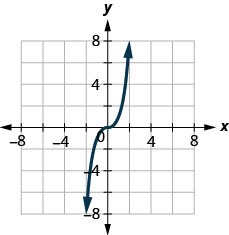
Figure 10.1.68
3.
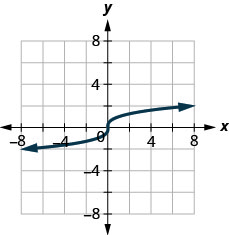
Figure 10.1.69
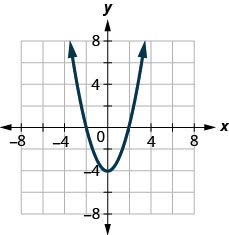
Figure 10.1.70
4.
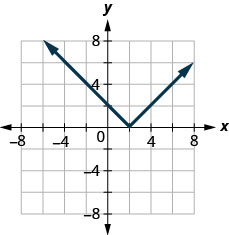
Figure 10.1.71
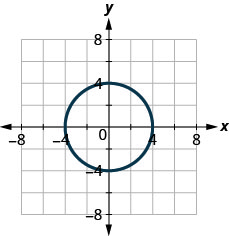
Figure 10.1.72
- Answer
-
1.
- Not a function
- Function; not one-to-one
3.
- One-to-one function
- Function; not one-to-one
In the following exercises, find the inverse of each function. Determine the domain and range of the inverse function.
- \(\{(2,1),(4,2),(6,3),(8,4)\}\)
- \(\{(6,2),(9,5),(12,8),(15,11)\}\)
- \(\{(0,-2),(1,3),(2,7),(3,12)\}\)
- \(\{(0,0),(1,1),(2,4),(3,9)\}\)
- \(\{(-2,-3),(-1,-1),(0,1),(1,3)\}\)
- \(\{(5,3),(4,2),(3,1),(2,0)\}\)
- Answer
-
1. \(\begin{array}{l}{\text { Inverse function: }\{(1,2),(2,4),(3,6),(4,8)\} . \text { Domain: }\{1,2,3,4\} . \text { Range: }} {\{2,4,6,8\} .}\end{array}\)
3. \(\begin{array}{l}{\text { Inverse function: }\{(-2,0),(3,1),(7,2),(12,3)\} . \text { Domain: }\{-2,3,7,12\} \text { . }} {\text { Range: }\{0,1,2,3\}}\end{array}\)
5. \(\begin{array}{l}{\text { Inverse function: }\{(-3,-2),(-1,-1),(1,0),(3,1)\} . \text { Domain: }} {\{-3,-1,1,3\} . \text { Range: }\{-2,-1,0,1\}}\end{array}\)
In the following exercises, graph, on the same coordinate system, the inverse of the one-to-one function shown.
Figure 10.1.73
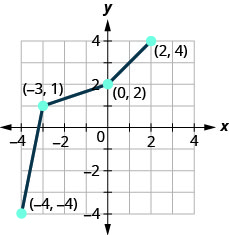
Figure 10.1.74
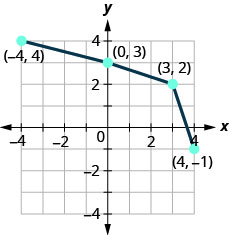
Figure 10.1.75
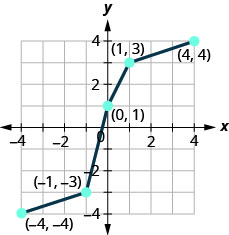
Figure 10.1.76
- Answer
-
1.
Figure 10.1.77 3.
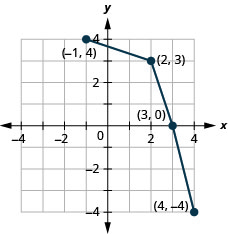
Figure 10.1.78
In the following exercises, determine whether or not the given functions are inverses.
- \(f(x)=x+8\) and \(g(x)=x-8\)
- \(f(x)=x-9\) and \(g(x)=x+9\)
- \(f(x)=7 x\) and \(g(x)=\frac{x}{7}\)
- \(f(x)=\frac{x}{11}\) and \(g(x)=11 x\)
- \(f(x)=7 x+3\) and \(g(x)=\frac{x-3}{7}\)
- \(f(x)=5 x-4\) and \(g(x)=\frac{x-4}{5}\)
- \(f(x)=\sqrt{x+2}\) and \(g(x)=x^{2}-2\)
- \(f(x)=\sqrt[3]{x-4}\) and \(g(x)=x^{3}+4\)
- Answer
-
1. \(g(f(x))=x,\) and \(f(g(x))=x,\) so they are inverses.
3. \(g(f(x))=x,\) and \(f(g(x))=x,\) so they are inverses.
5. \(g(f(x))=x,\) and \(f(g(x))=x,\) so they are inverses.
7. \(g(f(x))=x,\) and \(f(g(x))=x,\) so they are inverses (for nonnegative \(x )\)
In the following exercises, find the inverse of each function.
- \(f(x)=x-12\)
- \(f(x)=x+17\)
- \(f(x)=9 x\)
- \(f(x)=8 x\)
- \(f(x)=\frac{x}{6}\)
- \(f(x)=\frac{x}{4}\)
- \(f(x)=6 x-7\)
- \(f(x)=7 x-1\)
- \(f(x)=-2 x+5\)
- \(f(x)=-5 x-4\)
- \(f(x)=x^{2}+6, x \geq 0\)
- \(f(x)=x^{2}-9, x \geq 0\)
- \(f(x)=x^{3}-4\)
- \(f(x)=x^{3}+6\)
- \(f(x)=\frac{1}{x+2}\)
- \(f(x)=\frac{1}{x-6}\)
- \(f(x)=\sqrt{x-2}, x \geq 2\)
- \(f(x)=\sqrt{x+8}, x \geq-8\)
- \(f(x)=\sqrt[3]{x-3}\)
- \(f(x)=\sqrt[3]{x+5}\)
- \(f(x)=\sqrt[4]{9 x-5}, x \geq \frac{5}{9}\)
- \(f(x)=\sqrt[4]{8 x-3}, x \geq \frac{3}{8}\)
- \(f(x)=\sqrt[5]{-3 x+5}\)
- \(f(x)=\sqrt[5]{-4 x-3}\)
- Answer
-
1. \(f^{-1}(x)=x+12\)
3. \(f^{-1}(x)=\frac{x}{9}\)
5. \(f^{-1}(x)=6 x\)
7. \(f^{-1}(x)=\frac{x+7}{6}\)
9. \(f^{-1}(x)=\frac{x-5}{-2}\)
11. \(f^{-1}(x)=\sqrt{x-6}\)
13. \(f^{-1}(x)=\sqrt[3]{x+4}\)
15. \(f^{-1}(x)=\frac{1}{x}-2\)
17. \(f^{-1}(x)=x^{2}+2, x \geq 0\)
19. \(f^{-1}(x)=x^{3}+3\)
21. \(f^{-1}(x)=\frac{x^{4}+5}{9}, x \geq 0\)
23. \(f^{-1}(x)=\frac{x^{5}-5}{-3}\)
- Explain how the graph of the inverse of a function is related to the graph of the function.
- Explain how to find the inverse of a function from its equation. Use an example to demonstrate the steps.
- Answer
-
1. Answers will vary.
Self Check
a. After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.
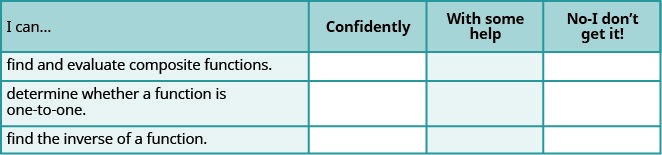
b. If most of your checks were:
…confidently. Congratulations! You have achieved the objectives in this section. Reflect on the study skills you used so that you can continue to use them. What did you do to become confident of your ability to do these things? Be specific.
…with some help. This must be addressed quickly because topics you do not master become potholes in your road to success. In math every topic builds upon previous work. It is important to make sure you have a strong foundation before you move on. Who can you ask for help? Your fellow classmates and instructor are good resources. Is there a place on campus where math tutors are available? Can your study skills be improved?
…no—I don’t get it! This is a warning sign and you must not ignore it. You should get help right away or you will quickly be overwhelmed. See your instructor as soon as you can to discuss your situation. Together you can come up with a plan to get you the help you need.