4.2.1: Case n=3
- Page ID
- 2177
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
The Euler-Poisson-Darboux equation in this case is
$$(rM)_{rr}=c^{-2}(rM)_{tt}.\]
Thus \(rM\) is the solution of the one-dimensional wave equation with initial data
\begin{equation}
\label{initialn3}\tag{4.2.1.1}
(rM)(r,0)=rF(r)\ \ \ (rM)_t(r,0)=rG(r).
\end{equation}
From the d'Alembert formula we get formally
\begin{eqnarray}
\label{meansol1}
M(r,t)&=&\dfrac{(r+ct)F(r+ct)+(r-ct)F(r-ct)}{2r}\\ \tag{4.2.1.2}
&&+\dfrac{1}{2cr}\int_{r-ct}^{r+ct}\ \xi G(\xi)\ d\xi.
\end{eqnarray}
The right hand side of the previous formula is well defined if the domain of dependence \([x-ct,x+ct]\) is a subset of \((0,\infty)\). We can extend \(F\) and \(G\) to \(F_0\) and \(G_0\) which are defined on \((-\infty,\infty)\) such that \(rF_0\) and \(rG_0\) are \(C^2(\mathbb{R}^1)\)-functions as follows.
Set
$$
F_0(r)=\left\{\begin{array}{r@{\quad:\quad}l}
F(r)&r>0\\
f(x)&r=0\\
F(-r)&r<0
\end{array}\right.\
\]
The function \(G_0(r)\) is given by the same definition where \(F\) and \(f\) are replaced by \(G\) and \(g\), respectively.
Lemma. \(rF_0(r),\ rG_0(r)\in C^2(\mathbb{R}^2)\).
Proof. From definition of \(F(r)\) and \(G(r)\), \(r>0\), it follows from the mean value theorem
$$\lim_{r\to+0} F(r)=f(x),\ \ \ \lim_{r\to+0} G(r)=g(x).\]
Thus \(rF_0(r)\) and \(rG_0(r)\) are \(C(\mathbb{R}^1)\)-functions. These functions are also in \(C^1(\mathbb{R}^1)\). This follows since \(F_0\) and \(G_0\) are in \(C^1(\mathbb{R}^1)\). We have, for example,
\begin{eqnarray*}
F'(r)&=&\dfrac{1}{\omega_n}\int_{\partial B_1(0)}\ \sum_{j=1}^n f_{y_j}(x+r\xi)\xi_j\ dS_\xi\\
F'(+0)&=&\dfrac{1}{\omega_n}\int_{\partial B_1(0)}\ \sum_{j=1}^n f_{y_j}(x)\xi_j\ dS_\xi\\
&=&\dfrac{1}{\omega_n}\sum_{j=1}^n f_{y_j}(x)\int_{\partial B_1(0)}\ n_j\ dS_\xi\\
&=&0.
\end{eqnarray*}
Then, \(rF_0(r)\) and \(rG_0(r)\) are in \(C^2(\mathbb{R}^1)\), provided \(F''\) and \(G''\) are bounded as \(r\to+0\). This property follows from
$$F''(r)=\dfrac{1}{\omega_n}\int_{\partial B_1(0)}\ \sum_{i,j=1}^n f_{y_iy_j}(x+r\xi)\xi_i\xi_j\ dS_\xi.\]
Thus
$$F''(+0)=\dfrac{1}{\omega_n}\sum_{i,j=1}^n f_{y_iy_j}(x)\int_{\partial B_1(0)}\ n_in_j\ dS_\xi.\]
We recall that \(f,g\in C^2(\mathbb{R}^2)\) by assumption.
\(\Box\)
The solution of the above initial value problem, where \(F\) and \(G\) are replaced by \(F_0\) and \(G_0\), respectively, is
\begin{eqnarray*}
M_0(r,t)&=&\dfrac{(r+ct)F_0(r+ct)+(r-ct)F_0(r-ct)}{2r}\\
&&+\dfrac{1}{2cr}\int_{r-ct}^{r+ct}\ \xi G_0(\xi)\ d\xi.
\end{eqnarray*}
Since \(F_0\) and \(G_0\) are even functions, we have
$$\int_{r-ct}^{ct-r}\ \xi G_0(\xi)\ d\xi=0.\]
Thus
\begin{eqnarray}
M_0(r,t)&=&\dfrac{(r+ct)F_0(r+ct)-(ct-r)F_0(ct-r)}{2r}\nonumber\\
\label{meansol2} \tag{4.2.1.3}
&&+\dfrac{1}{2cr}\int_{ct-r}^{ct+r}\ \xi G_0(\xi)\ d\xi,
\end{eqnarray}
see Figure 4.2.1.1.
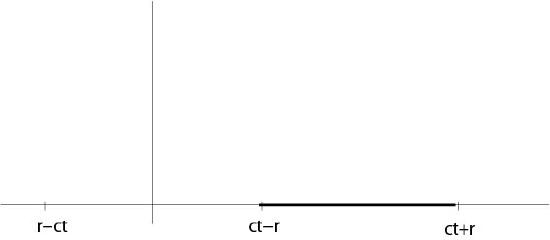
Figure 4.2.1.1: Changed domain of integration
For fixed \(t>0\) and \(0<r<ct\) it follows that \(M_0(r,t)\) is the solution of the initial value problem with given initially data (\ref{initialn3}) since \(F_0(s)=F(s)\), \(G_0(s)=G(s)\) if \(s>0\).
Since for fixed \(t>0\)
$$u(x,t)=\lim_{r\to 0} M_0(r,t),\]
it follows from d'Hospital's rule that
\begin{eqnarray*}
u(x,t)&=&ctF'(ct)+F(ct)+tG(ct)\\
&=&\dfrac{d}{dt}\left(tF(ct)\right)+tG(ct).
\end{eqnarray*}
Theorem 4.2. Assume \(f\in C^3(\mathbb{R}^3)\) and \(g\in C^2(\mathbb{R}^3)\) are given. Then there exists a unique solution \(u\in C^2(\mathbb{R}^3\times [0,\infty))\) of the initial value problem (4.2.2)-(4.2.3), where \(n=3\), and the solution is given by the Poisson's formula
\begin{eqnarray}
u(x,t)&=&\dfrac{1}{4\pi c^2}\dfrac{\partial}{\partial t}\left(\dfrac{1}{t}\int_{\partial B_{ct}(x)}\ f(y)\ dS_y\right)\\ \tag{4.2.1.4}
&&+\dfrac{1}{4\pi c^2 t}\int_{\partial B_{ct}(x)}\ g(y)\ dS_y.
\end{eqnarray}
Proof. Above we have shown that a \(C^2\)-solution is given by Poisson's formula. Under the additional assumption \(f\in C^3\) it follows from Poisson's formula that this formula defines a solution which is in \(C^2\), see F. John [10], p. 129.
\(\Box\)
Corollary. From Poisson's formula we see that the domain of dependence for \(u(x,t_0)\) is the intersection of the cone defined by \(|y-x|=c|t-t_0|\) with the hyperplane defined by \(t=0\), see Figure 4.2.1.2.
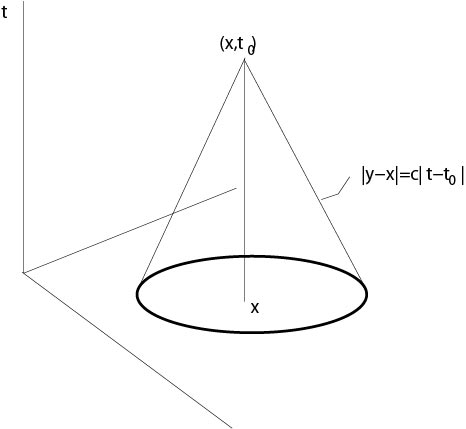
Figure 4.2.1.2: Domain of dependence, case \(n=3\).
Contributors and Attributions
Integrated by Justin Marshall.


