4.2.2: Case n=2

Consider the initial value problem

$$\begin{eqnarray} \label{n2dgl}\tag{4.2.2.1} v_{xx}+v_{yy}&=&c^{-2}v_{tt}\\ \label{initial1} \tag{4.2.2.2} v(x,y,0)&=&f(x,y)\\ \label{initial2} \tag{4.2.2.3} v_t(x,y,0)&=&g(x,y), \end{eqnarray}$$

where $f\in C^3,\ g\in C^2$.

Using the formula for the solution of the three-dimensional initial value problem we will derive a formula for the two-dimensional case. The following consideration is called Hadamard's method of decent.

Let $v(x,y,t)$ be a solution of ($\ref{n2dgl}$)-($\ref{initial2}$), then

$$u(x,y,z,t):=v(x,y,t)\]

is a solution of the three-dimensional initial value problem with initial data $f(x,y)$, $g(x,y)$, independent of $z$, since $u$ satisfies ($\ref{n2dgl}$)-($\ref{initial2}$). Hence, since $u(x,y,z,t)=u(x,y,0,t)+u_z(x,y,\delta z,t)z$, $0<\delta<1$, and $u_z=0$, we have

$$v(x,y,t)=u(x,y,0,t).\]

Poisson's formula in the three-dimensional case implies

$$\begin{eqnarray} v(x,y,t)&=&\frac{1}{4\pi c^2}\frac{\partial}{\partial t}\left(\frac{1}{t}\int_{\partial B_{ct}(x,y,0)}\ f(\xi,\eta)\ dS\right)\nonumber\\ \label{poissonhilf1} \tag{4.2.2.4} &&+\frac{1}{4\pi c^2 t} \int_{\partial B_{ct}(x,y,0)}\ g(\xi,\eta)\ dS. \end{eqnarray}$$

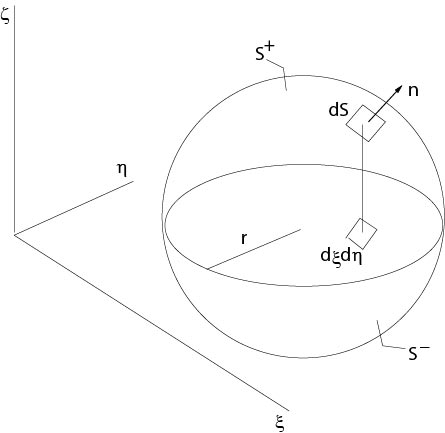
Figure 4.2.2.1: Domains of integration

The integrands are independent on $\zeta$. The surface $S$ is defined by $\chi(\xi,\eta,\zeta):=(\xi-x)^2+(\eta-y)^2+\zeta^2-c^2 t^2=0$. Then the exterior normal $n$ at $S$ is $n=\nabla\chi/|\nabla\chi|$ and the surface element is given by $dS=(1/|n_3|)d\xi d\eta$, where the third coordinate of $n$ is

$$n_3=\pm\frac{\sqrt{c^2 t^2-(\xi-x)^2-(\eta-y)^2}}{ct}.\]

The positive sign applies on $S^+$, where $\zeta>0$ and the sign is negative on $S^-$ where $\zeta<0$, see Figure 4.2.2.1. We have $S=S^+\cup\overline{S^-}$.

Set $\rho=\sqrt{(\xi-x)^2+(\eta-y)^2}$. Then it follows from ($\ref{poissonhilf1}$)

Theorem 4.3. The solution of the Cauchy initial value problem ($\ref{n2dgl}$)-($\ref{initial2}$) is given by

$$\begin{eqnarray*} v(x,y,t)&=&\frac{1}{2\pi c}\frac{\partial}{\partial t}\int_{B_{ct}(x,y)}\ \frac{f(\xi,\eta)}{\sqrt{c^2 t^2-\rho^2}}\ d\xi d\eta\\ &&+\frac{1}{2\pi c}\int_{B_{ct}(x,y)}\ \frac{g(\xi,\eta)}{\sqrt{c^2 t^2-\rho^2}}\ d\xi d\eta. \end{eqnarray*}$$

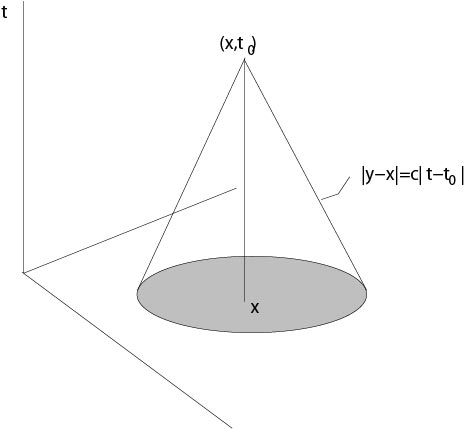
Figure 4.2.2.2: Interval of dependence, case $n=2$

Corollary. In contrast to the three dimensional case, the domain of dependence is here the disk $B_{ct_o}(x_0,y_0)$ and not the boundary only. Therefore, see formula of Theorem 4.3, if $f,\ g$ have supports in a compact domain $D\subset\mathbb{R}^2$, then these functions have influence on the value $v(x,y,t)$ for all time $t>T$, $T$ sufficiently large.

Contributors and Attributions

• Prof. Dr. Erich Miersemann (Universität Leipzig)

• Integrated by Justin Marshall.