Search

Text Color

Margin Size

Font Type

Enable Dyslexic Font

A.3.3: Section 3.3 Answers

Last updated

Apr 25, 2022
Save as PDF
- A.3.2: Section 3.2 Answers
- A.4.1: Section 4.1 Answers

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\id}{\mathrm{id}}$ $\newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$ $\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$ $\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\id}{\mathrm{id}}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\kernel}{\mathrm{null}\,}$

$\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$

$\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$

$\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$ $\newcommand{\AA}{\unicode[.8,0]{x212B}}$

$\newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$\newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$\newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vectorC}[1]{\textbf{#1}}$

$\newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$\newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$\newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}}$

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\avec}{\mathbf a}$

$\newcommand{\bvec}{\mathbf b}$

$\newcommand{\cvec}{\mathbf c}$

$\newcommand{\dvec}{\mathbf d}$

$\newcommand{\dtil}{\widetilde{\mathbf d}}$

$\newcommand{\evec}{\mathbf e}$

$\newcommand{\fvec}{\mathbf f}$

$\newcommand{\nvec}{\mathbf n}$

$\newcommand{\pvec}{\mathbf p}$

$\newcommand{\qvec}{\mathbf q}$

$\newcommand{\svec}{\mathbf s}$

$\newcommand{\tvec}{\mathbf t}$

$\newcommand{\uvec}{\mathbf u}$

$\newcommand{\vvec}{\mathbf v}$

$\newcommand{\wvec}{\mathbf w}$

$\newcommand{\xvec}{\mathbf x}$

$\newcommand{\yvec}{\mathbf y}$

$\newcommand{\zvec}{\mathbf z}$

$\newcommand{\rvec}{\mathbf r}$

$\newcommand{\mvec}{\mathbf m}$

$\newcommand{\zerovec}{\mathbf 0}$

$\newcommand{\onevec}{\mathbf 1}$

$\newcommand{\real}{\mathbb R}$

$\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$

$\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$

$\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$

$\newcommand{\laspan}[1]{\text{Span}\{#1\}}$

$\newcommand{\bcal}{\cal B}$

$\newcommand{\ccal}{\cal C}$

$\newcommand{\scal}{\cal S}$

$\newcommand{\wcal}{\cal W}$

$\newcommand{\ecal}{\cal E}$

$\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$

$\newcommand{\gray}[1]{\color{gray}{#1}}$

$\newcommand{\lgray}[1]{\color{lightgray}{#1}}$

$\newcommand{\rank}{\operatorname{rank}}$

$\newcommand{\row}{\text{Row}}$

$\newcommand{\col}{\text{Col}}$

$\renewcommand{\row}{\text{Row}}$

$\newcommand{\nul}{\text{Nul}}$

$\newcommand{\var}{\text{Var}}$

$\newcommand{\corr}{\text{corr}}$

$\newcommand{\len}[1]{\left|#1\right|}$

$\newcommand{\bbar}{\overline{\bvec}}$

$\newcommand{\bhat}{\widehat{\bvec}}$

$\newcommand{\bperp}{\bvec^\perp}$

$\newcommand{\xhat}{\widehat{\xvec}}$

$\newcommand{\vhat}{\widehat{\vvec}}$

$\newcommand{\uhat}{\widehat{\uvec}}$

$\newcommand{\what}{\widehat{\wvec}}$

$\newcommand{\Sighat}{\widehat{\Sigma}}$

$\newcommand{\lt}{<}$

$\newcommand{\gt}{>}$

$\newcommand{\amp}{&}$

$\definecolor{fillinmathshade}{gray}{0.9}$

1. $y_{1}=1.550598190, \: y_{2} = 2.469649729$

2. $y_{1} = 1.221551366,\: y_{2} = 1.492920208$

3. $y_{1} = 1.890339767,\: y_{2} = 1.763094323$

4. $y_{1} = 2.961316248,\: y_{2} = 2.920128958$

5. $y_{1} = 2.475605264,\: y_{2} = 1.825992433$

$x$	$h=0.1$	$h=0.05$	$h=0.025$	Exact
$1.0$	$54.654509699$	$54.648344019$	$54.647962328$	$54.647937102$

$x$	$h=0.1$	$h=0.05$	$h=0.025$	Exact
$2.0$	$1.353191745$	$1.353193606$	$1.353193712$	$1.353193719$

$x$	$h=0.05$	$h=0.025$	$h=0.0125$	Exact
$1.50$	$10.498658198$	$10.499906266$	$10.499993820$	$10.500000000$

$x$	$h=0.1$	$h=0.05$	$h=0.025$	$h=0.1$	$h=0.05$	$h=0.025$
$3.0$	$1.456023907$	$1.456023403$	$1.456023379$	$0.0000124$	$0.000000611$	$0.0000000333$
	Approximate Solutions			Residuals

10.

$x$	$h=0.1$	$h=0.05$	$h=0.025$	$h=0.1$	$h=0.05$	$h=0.025$
$2.0$	$0.492663789$	$0.492663738$	$0.492663736$	$0.000000902$	$0.0000000508$	$0.00000000302$
	Approximate Solutions			Residuals

11.

$x$	$h=0.1$	$h=0.05$	$h=0.025$	"Exact"
$1.0$	$0.659957046$	$0.659957646$	$0.659957686$	$0.659957689$

12.

$x$	$h=0.1$	$h=0.05$	$h=0.025$	"Exact"
$2.0$	$-0.750911103$	$-0.750912294$	$-0.750912367$	$-0.750912371$

13. Applying variation of parameters to the given initial value problem yields $y = ue^{−3x}$ , where $(A) u' = 1 − 4x + 3x^{2} − 4x^{3}, u(0) = −3$ . Since $u^{(5)} = 0$ , the Runge-Kutta method yields the exact solution of (A). Therefore the Euler semilinear method produces the exact solution of the given problem.

$clipboard_e9e428930ffce428ad2d7df8d39937a25.png$

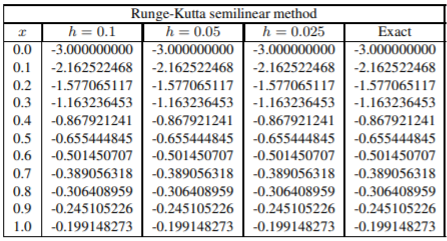
$clipboard_e075f364cb890bde142bc6294dc1ba24e.png$

14.

Runge-Kutta method
$x$	$h=0.1$	$h=0.05$	$h=0.025$	"Exact"
$3.0$	$15.281660036$	$15.281981407$	$15.282003300$	$15.282004826$

Runge-Kutta semilinear method
$x$	$h=0.1$	$h=0.05$	$h=0.025$	"Exact"
$3.0$	$15.282005990$	$15.282004899$	$15.282004831$	$15.282004826$

15.

Runge-Kutta method
$x$	$h=0.2$	$h=0.1$	$h=0.05$	"Exact"
$2.0$	$0.904678156$	$0.904295772$	$0.904277759$	$0.904276722$

Runge-Kutta semilinear method
$x$	$h=0.2$	$h=0.1$	$h=0.05$	"Exact"
$2.0$	$0.904592215$	$0.904297062$	$0.904278004$	$0.904276722$

16.

Runge-Kutta method
$x$	$h=0.2$	$h=0.1$	$h=0.05$	"Exact"
$3.0$	$0.967523147$	$0.967523152$	$0.967523153$	$0.967523153$

Runge-Kutta semilinear method
$x$	$h=0.2$	$h=0.1$	$h=0.05$	"Exact"
$3.0$	$0.967523147$	$0.967523152$	$0.967523153$	$0.967523153$

17.

Runge-Kutta method
$x$	$h=0.0500$	$h=0.0250$	$h=0.0125$	"Exact"
$1.50$	$0.343839158$	$0.343784814$	$0.343780796$	$0.343780513$

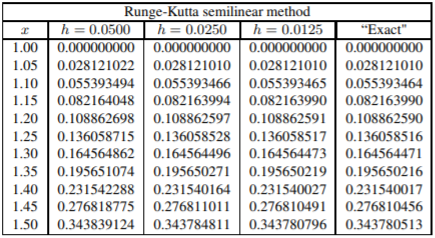
$clipboard_e7cd069c28f0e49fea809ca150442f812.png$

18.

Runge-Kutta method
$x$	$h=0.2$	$h=0.1$	$h=0.05$	"Exact"
$2.0$	$0.732633229$	$0.732638318$	$0.732638609$	$0.732638628$

Runge-Kutta semilinear method
$x$	$h=0.2$	$h=0.1$	$h=0.05$	"Exact"
$2.0$	$0.732639212$	$0.732638663$	$0.732638630$	$0.732638628$

19.

Runge-Kutta method
$x$	$h=0.0500$	$h=0.0250$	$h=0.0125$	"Exact"
$1.50$	$2.244025683$	$2.244024088$	$2.244023989$	$2.244023982$

Runge-Kutta semilinear method
$x$	$h=0.0500$	$h=0.0250$	$h=0.0125$	"Exact"
$1.50$	$2.244025081$	$2.244024051$	$2.244023987$	$2.244023982$

20.

Runge-Kutta method
$x$	$h=0.1$	$h=0.05$	$h=0.025$	"Exact"
$1.0$	$0.056426886$	$0.056416137$	$0.056415552$	$0.056415515$

Runge-Kutta semilinear method
$x$	$h=0.1$	$h=0.05$	$h=0.025$	"Exact"
$1.0$	$0.056415185$	$0.056415495$	$0.056415514$	$0.056415515$

21.

Runge-Kutta method
$x$	$h=0.1$	$h=0.05$	$h=0.025$	"Exact"
$1.0$	$54.695901186$	$54.727111858$	$54.729426250$	$54.729594761$

Runge-Kutta semilinear method
$x$	$h=0.1$	$h=0.05$	$h=0.025$	"Exact"
$1.0$	$54.729099966$	$54.729561720$	$54.729592658$	$54.729594761$

22.

Runge-Kutta method
$x$	$h=0.1$	$h=0.05$	$h=0.025$	"Exact"
$3.0$	$1.361384082$	$1.361383812$	$1.361383809$	$1.361383810$

Runge-Kutta semilinear method
$x$	$h=0.1$	$h=0.05$	$h=0.025$	"Exact"
$3.0$	$1.361456502$	$1.361388196$	$1.361384079$	$1.361383810$

24.

$x$	$h=.1$	$h=.05$	$h=.025$	Exact
$2.00$	$-1.000000000$	$-1.000000000$	$-1.000000000$	$-1.000000000$

25.

$x$	$h=.1$	$h=.05$	$h=.025$	"Exact"
$1.00$	$1.000000000$	$1.000000000$	$1.000000000$	$1.000000000$

26.

$x$	$h=.1$	$h=.05$	$h=.025$	"Exact"
$1.50$	$4.142171279$	$4.142170553$	$4.142170508$	$4.142170505$

27.

$x$	$h=.1$	$h=.05$	$h=.025$	"Exact"
$3.0$	$16.666666988$	$16.666666687$	$16.666666668$	$16.666666667$

Support Center

How can we help?