2.3.3: Using Equations for Lines
- Page ID
- 35704
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Lesson
Let's write equations for lines.
Exercise \(\PageIndex{1}\): Missing center
A dilation with scale factor 2 sends \(A\) to \(B\). Where is the center of the dilation?
Exercise \(\PageIndex{2}\): Writing Relationships from Two Points
Here is a line.
- Using what you know about similar triangles, find an equation for the line in the diagram.
- What is the slope of this line? Does it appear in your equation?
- Is \((9,11)\) also on the line? How do you know?
- Is \((100,93)\) also on the line?
Are you ready for more?
There are many different ways to write down an equation for a line like the one in the problem. Does \(\frac{y-3}{x-6}=2\) represent the line? What about \(\frac{y-6}{x-4}=5\)? What about \(\frac{y+5}{x-1}=2\)? Explain your reasoning.
Exercise \(\PageIndex{3}\): Dilations and Slope Triangles
Here is triangle \(ABC\).
- Draw the dilation of triangle \(ABC\) with center \((0,1)\) and scale factor 2.
- Draw the dilation of triangle \(ABC\) with center \((0,1)\) and scale factor 2.5.
- Where is \(C\) mapped by the dilation with center \((0,1)\) and scale factor ?
- For which scale factor does the dilation with center \((0,1)\) send \(C\) to \((9,5.5)\)? Explain how you know.
Summary
We can use what we know about slope to decide if a point lies on a line. Here is a line with a few points labeled.
The slope triangle with vertices \((0,1)\) and \((2,5)\) gives a slope of \(\frac{5-1}{2-0}=2\). The slope triangle with vertices \((0,1)\) and \((x,y)\) gives a slope of \(\frac{y-1}{x}\). Since these slopes are the same, \(\frac{y-1}{x}=2\) is an equation for the line. So, if we want to check whether or not the point \((11,23)\) lies on this line, we can check that \(\frac{23-1}{11}=2\). Since \((11,23)\) is a solution to the equation, it is on the line!
Glossary Entries
Definition: Similar
Two figures are similar if one can fit exactly over the other after rigid transformations and dilations.
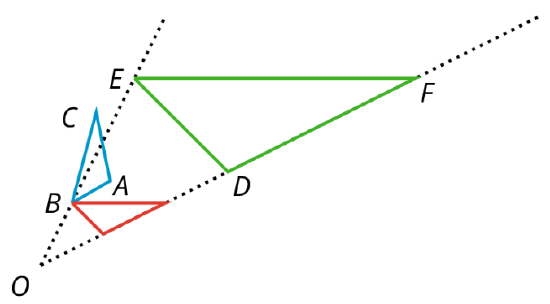
In this figure, triangle \(ABC\) is similar to triangle \(DEF\).
If \(ABC\) is rotated around point \(B\) and then dilated with center point \(O\), then it will fit exactly over \(DEF\). This means that they are similar.
Definition: Slope
The slope of a line is a number we can calculate using any two points on the line. To find the slope, divide the vertical distance between the points by the horizontal distance.
The slope of this line is 2 divided by 3 or \(\frac{2}{3}\).
Practice
Exercise \(\PageIndex{4}\)
Select all the points that are on the line through \((0,5)\) and \((2,8)\).
- \((4,11)\)
- \((5,10)\)
- \((6,14)\)
- \((30,50)\)
- \((40,60)\)
Exercise \(\PageIndex{5}\)
All three points displayed are on the line. Find an equation relating \(x\) and \(y\).
Exercise \(\PageIndex{6}\)
Here is triangle \(ABC\).
- Draw the dilation of triangle \(ABC\) with center \((2,0)\) and scale factor 2.
- Draw the dilation of triangle \(ABC\) with center \((2,0)\) and scale factor 3.
- Draw the dilation of triangle \(ABC\) with center \((2,0)\) and scale factor \(\frac{1}{2}\).
- What are the coordinates of the image of point \(C\) when triangle \(ABC\) is dilated with center \((2,0)\) and scale factor \(s\)?
- Write an equation for the line containing all possible images of point \(C\).
Exercise \(\PageIndex{7}\)
Here are some line segments.
- Which segment is a dilation of \(\overline{BC}\) using \(A\) as the center of dilation and a scale factor of \(\frac{2}{3}\)?
- Which segment is a dilation of \(\overline{BC}\) using \(A\) as the center of dilation and a scale factor of \(\frac{3}{2}\)?
- Which segment is not a dilation of \(\overline{BC}\), and how do you know?
(From Unit 2.1.4)