9.S: Math Models and Geometry (Summary)
- Page ID
- 7238
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Key Terms
| angle | An angle is formed by two rays that share a common endpoint. Each ray is called a side of the angle. |
| area | The area is a measure of the surface covered by a figure. |
| complementary angles | If the sum of the measures of two angles is 90°, then they are called complementary angles. |
| cone | A cone is a solid figure with one circular base and a vertex. |
| cube | A cube is a rectangular solid whose length, width, and height are equal. |
| cylinder | A cylinder is a solid figure with two parallel circles of the same size at the top and bottom. |
| equilateral triangle | A triangle with all three sides of equal length is called an equilateral triangle. |
| hypotenuse | The side of the triangle opposite the 90° angle is called the hypotenuse. |
| irregular figure | A figure that is not a standard geometric shape. Its area cannot be calculated using any of the standard area formulas. |
| isosceles triangle | A triangle with two sides of equal length is called an isosceles triangle. |
| legs of a right triangle | The sides of a right triangle adjacent to the right angle |
| perimeter | The perimeter is a measure of the distance around a figure. |
| rectangle | A geometric figure that has four sides and four right angles. |
| right triangle | A triangle that has one 90° angle. |
| similar figures | In geometry, if two figures have exactly the same shape but different sizes, we say they are similar figures. |
| supplementary angles | If the sum of the measures of two angles is 180°, then they are called supplementary angles. |
| trapezoid | A four-sided figure, a quadrilateral, with two sides that are parallel and two sides that are not. |
| triangle | A geometric figure with three sides and three angles. |
| vertex of an angle | When two rays meet to form an angle, the common endpoint is called the vertex of the angle. |
Key Concepts
9.1 - Use a Problem Solving Strategy
- Problem Solving Strategy
- Read the word problem. Make sure you understand all the words and ideas. You may need to read the problem two or more times. If there are words you don't understand, look them up in a dictionary or on the internet.
- Identify what you are looking for.
- Name what you are looking for. Choose a variable to represent that quantity.
- Translate into an equation. It may be helpful to first restate the problem in one sentence before translating.
- Solve the equation using good algebra techniques.
- Check the answer in the problem. Make sure it makes sense.
- Answer the question with a complete sentence.
9.2 - Solve Money Applications
- Finding the Total Value for Coins of the Same Type
- For coins of the same type, the total value can be found as follows:$$number \cdot value = total\; value$$where number is the number of coins, value is the value of each coin, and total value is the total value of all the coins.
- Solve a Coin Word Problem
- Read the problem. Make sure you understand all the words and ideas, and create a table to organize the information.
- Identify what you are looking for.
- Name what you are looking for. Choose a variable to represent that quantity.
- Use variable expressions to represent the number of each type of coin and write them in the table.
- Multiply the number times the value to get the total value of each type of coin.
- Translate into an equation. Write the equation by adding the total values of all the types of coins.
- Solve the equation using good algebra techniques.
- Check the answer in the problem and make sure it makes sense.
- Answer the question with a complete sentence.
Table 9.16
| Type | Number | Value ($) | Total Value ($) |
|---|---|---|---|
9.3 - Use Properties of Angles, Triangles, and the Pythagorean Theorem
- Supplementary and Complementary Angles
- If the sum of the measures of two angles is 180°, then the angles are supplementary.
- If ∠A and ∠B are supplementary, then m∠A + m∠B = 180 .
- If the sum of the measures of two angles is 90°, then the angles are complementary.
- If ∠A and ∠B are complementary, then m∠A + m∠B = 90.
- Solve Geometry Applications
- Read the problem and make sure you understand all the words and ideas. Draw a figure and label it with the given information.
- Identify what you are looking for.
- Name what you are looking for and choose a variable to represent it.
- Translate into an equation by writing the appropriate formula or model for the situation. Substitute in the given information.
- Solve the equation using good algebra techniques.
- Check the answer in the problem and make sure it makes sense.
- Answer the question with a complete sentence.
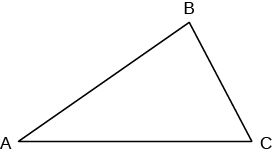
- Sum of the Measures of the Angles of a Triangle
- For any ΔABC, the sum of the measures is 180°
- m∠A + m∠B = 180
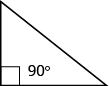
- Right Triangle
- A right triangle is a triangle that has one 90° angle, which is often marked with a ⦜ symbol.
- Properties of Similar Triangles
- If two triangles are similar, then their corresponding angle measures are equal and their corresponding side lengths have the same ratio.
9.4 - Use Properties of Rectangles, Triangles, and Trapezoids
- Properties of Rectangles
- Rectangles have four sides and four right (90°) angles.
- The lengths of opposite sides are equal.
- The perimeter, P , of a rectangle is the sum of twice the length and twice the width.$$P = 2L + 2W$$
- The area, A, of a rectangle is the length times the width.$$A = L \cdot W$
- Triangle Properties
- For any triangle ΔABC , the sum of the measures of the angles is 180°.$$m \angle A + m \angle B + m \angle C = 180°$$
- The perimeter of a triangle is the sum of the lengths of the sides.$$P = a + b + c$$
- The area of a triangle is one-half the base, b, times the height, h.$$A = \dfrac{1}{2} bh$$
9.5 - Solve Geometry Applications: Circles and Irregular Figures
- Problem Solving Strategy for Geometry Applications
- Read the problem and make sure you understand all the words and ideas. Draw the figure and label it with the given information.
- Identify what you are looking for.
- Name what you are looking for. Choose a variable to represent that quantity.
- Translate into an equation by writing the appropriate formula or model for the situation. Substitute in the given information.
- Solve the equation using good algebra techniques.
- Check the answer in the problem and make sure it makes sense.
- Answer the question with a complete sentence.
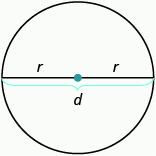
- Properties of Circles
- d = 2r
- Circumference: C = 2\(\pi\)r or C = \(\pi\)d
- Area: A = \(\pi\)r2
9.6 - Solve Geometry Applications: Volume and Surface Area
- Volume and Surface Area of a Rectangular Solid
- V = LWH
- S = 2LH + 2LW + 2WH
- Volume and Surface Area of a Cube
- V = s3
- S = 6s2
- Volume and Surface Area of a Sphere
- V = \(\dfrac{4}{3} \pi\)r3
- S = 4\(\pi\)r2
- Volume and Surface Area of a Cylinder
- V = \(\pi\)r2h
- S = 2\(\pi\)r2 + 2\(\pi\)rh
- Volume of a Cone
- For a cone with radius r and height h: Volume: V = \(\dfrac{1}{3} \pi\)r2h
9.7 - Solve a Formula for a Specific Variable
- Distance, Rate, and Time
- d = rt
Contributors and Attributions
Lynn Marecek (Santa Ana College) and MaryAnne Anthony-Smith (Formerly of Santa Ana College). This content is licensed under Creative Commons Attribution License v4.0 "Download for free at http://cnx.org/contents/fd53eae1-fa2...49835c3c@5.191."


