2.1: Iterations of real quadratic functions
- Page ID
- 101321
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
Iteration diagram
We can trace real maps xn+1 = f( xn ) dynamics on 2D the "iteration diagram" to the left below. Dependence xn on n is plotted to the right.
Controls: Drag the blue curve to change C and xo value. Press <Enter> to set new parameter values from text fields.

Here the blue curve is the map f oN(x) = f(f(...f(x))). -2 ≤ x, y ≤ 2. For N = 1 we get y(0) = f(0) = C and C value coincides with Y coordinate. Iterations begin from the starting point xo .

To plot the iteration diagram we draw the vertical red line from xo toward the blue curve y = f(x) = x2 + c, where y1 = f(xo ). To get the second iteration we draw the red horizontal line to the green y = x one, where x1 = y1 = f(xo ). Then draw again the vertical line to the blue curve to get y2 = f(x1 ) and so on.
Points fc: xo → x1 → x2 → ... for some value c and xo form the orbit of xo.
Fixed points, attracting cycles and bifurcations

Fixed points of a map x∗ = f(x∗ ) correspond to intersections of the y = x and y = f(x) (green and blue) curves. E.g. for C = -1/2 iterations go away unstable fixed point (the right intersection) and converge to attracting fixed point to the left.

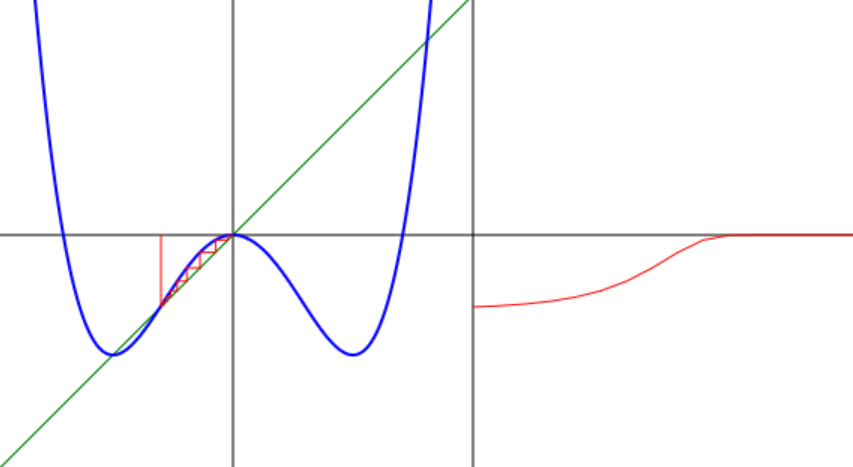
For c < -0.75 the left attracting fixed point becomes repelling and iterations converge to attracting period-2 orbit x1 → x2 → x1 ... The map f o2 has two attracting fixed points x1, x2 (on the right image). Such qualitative change in map dynamics is called bifurcation. Drag the blue curve to watch the bifurcation.

