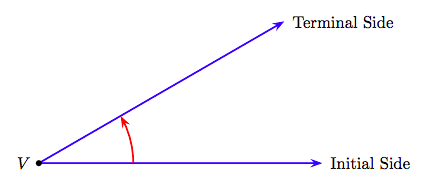
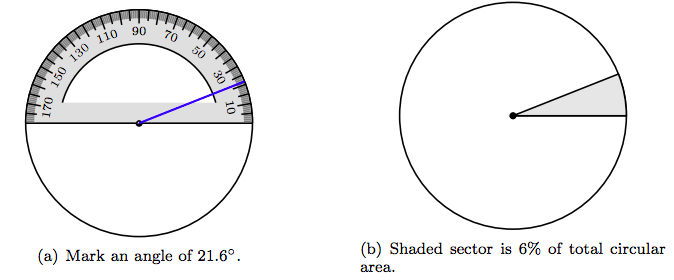
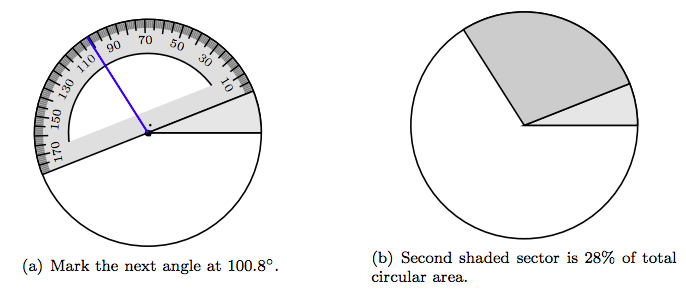
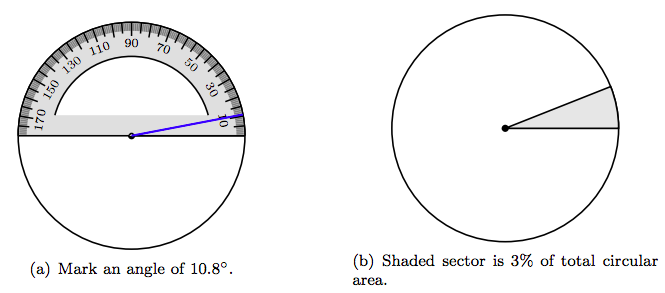
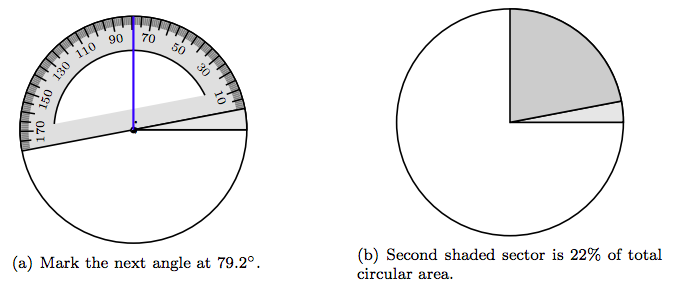
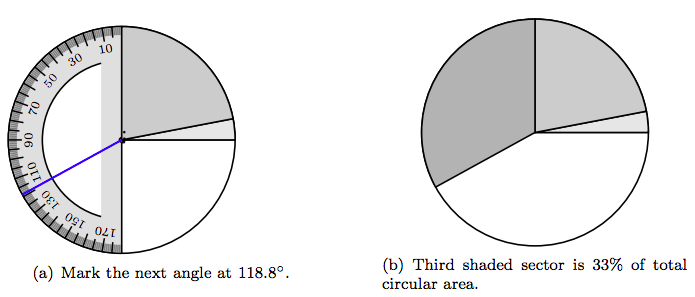
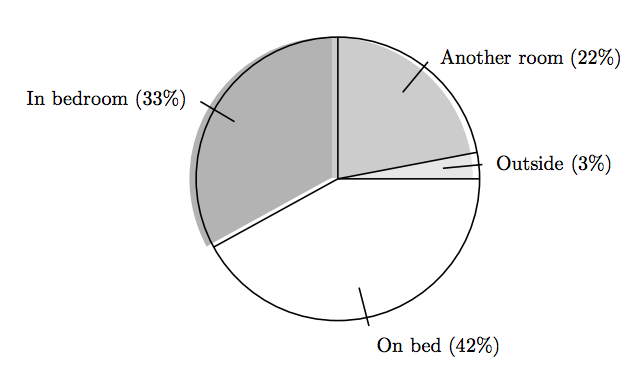
In this section we turn our attention to pie charts, but before we do, we need to establish some fundamentals regarding measurement of angles.
If you take a circle and divide it into 360 equal increments, then each increment is called one degree (1◦). See Figure 7.2.
Exercises
1. In an election for class president, Raven received 21% of the votes, Anita received 27%, Jamal received 24% of the votes, and 28% of the votes were received by other candidates. If a pie chart is created for this data, what will be the degree measure of the central angle of the sector representing Raven’s share of the vote? Round your answer to the nearest degree.
2. In an election for class president, Fernando received 26% of the votes, Luisa received 26%, Ali received 26% of the votes, and 22% of the votes were received by other candidates. If a pie chart is created for this data, what will be the degree measure of the central angle of the sector representing Fernando’s share of the vote? Round your answer to the nearest degree.
3. In an election for class president, Akbar received 23% of the votes, Ali received 27%, Juanita received 30% of the votes, and 20% of the votes were received by other candidates. If a pie chart is created for this data, what will be the degree measure of the central angle of the sector representing Akbar’s share of the vote? Round your answer to the nearest degree.
4. In an election for class president, Kamili received 21% of the votes, Bernardo received 22%, Fernando received 30% of the votes, and 27% of the votes were received by other candidates. If a pie chart is created for this data, what will be the degree measure of the central angle of the sector representing Kamili’s share of the vote? Round your answer to the nearest degree.
5. In an election for class president, Jamal received 30% of the votes, Luisa received 20%, Kamili received 28% of the votes, and 22% of the votes were received by other candidates. If a pie chart is created for this data, what will be the degree measure of the central angle of the sector representing Jamal’s share of the vote? Round your answer to the nearest degree.
6. In an election for class president, Juanita received 30% of the votes, Ali received 24%, Estevan received 24% of the votes, and 22% of the votes were received by other candidates. If a pie chart is created for this data, what will be the degree measure of the central angle of the sector representing Juanita’s share of the vote? Round your answer to the nearest degree.
7. In an election for class president, Chin received 5 votes, Mabel received 13 votes, and Juanita received the remaining 32 votes cast. If a pie chart is created for this voting data, what will be the degree measure of the central angle of the sector representing Chin’s share of the vote? Round your answer to the nearest degree.
8. In an election for class president, Anita received 11 votes, Jose received 9 votes, and Bernardo received the remaining 30 votes cast. If a pie chart is created for this voting data, what will be the degree measure of the central angle of the sector representing Anita’s share of the vote? Round your answer to the nearest degree.
9. In an election for class president, Kamili received 14 votes, Jamal received 9 votes, and Jose received the remaining 27 votes cast. If a pie chart is created for this voting data, what will be the degree measure of the central angle of the sector representing Kamili’s share of the vote? Round your answer to the nearest degree.
10. In an election for class president, Jun received 13 votes, Abdul received 15 votes, and Raven received the remaining 22 votes cast. If a pie chart is created for this voting data, what will be the degree measure of the central angle of the sector representing Jun’s share of the vote? Round your answer to the nearest degree.
11. In an election for class president, Hue received 13 votes, Ali received 6 votes, and Henry received the remaining 31 votes cast. If a pie chart is created for this voting data, what will be the degree measure of the central angle of the sector representing Hue’s share of the vote? Round your answer to the nearest degree.
12. In an election for class president, Mercy received 9 votes, Bernardo received 7 votes, and Hans received the remaining 34 votes cast. If a pie chart is created for this voting data, what will be the degree measure of the central angle of the sector representing Mercy’s share of the vote? Round your answer to the nearest degree.
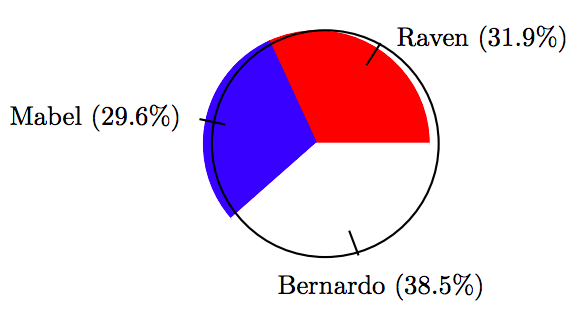
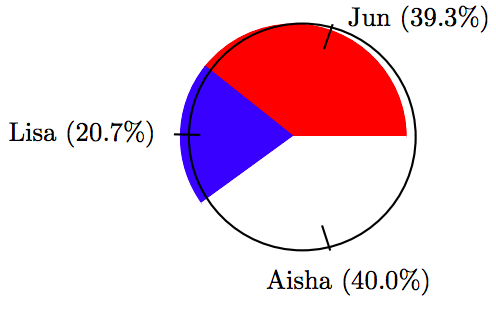
13. In an election for class president, the vote distribution among three candidates is shown in the following pie chart.
If there were a total of 95 votes cast in the election, find the number of votes that Raven received, correct to the nearest vote.
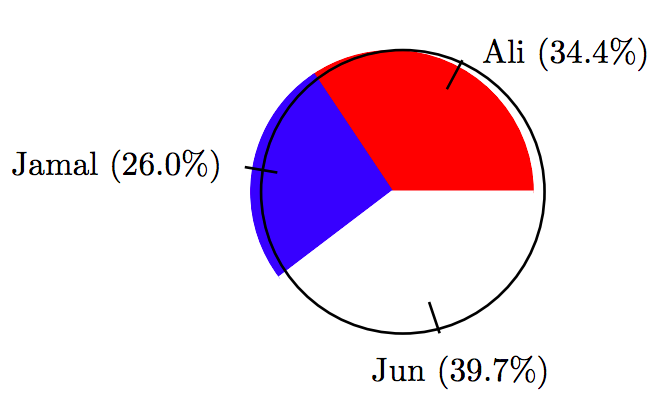
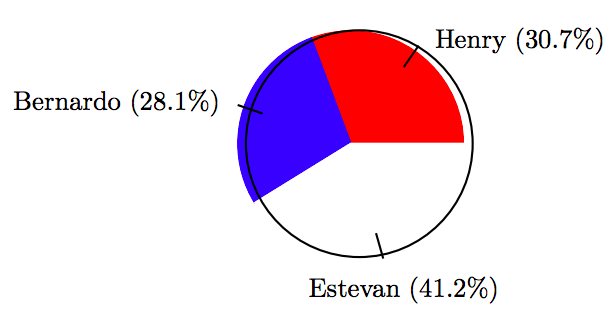
14. In an election for class president, the vote distribution among three candidates is shown in the following pie chart.
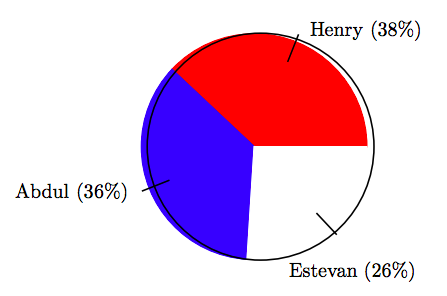
If there were a total of 79 votes cast in the election, find the number of votes that Henry received, correct to the nearest vote.
15. In an election for class president, the vote distribution among three candidates is shown in the following pie chart.

If there were a total of 58 votes cast in the election, find the number of votes that Ali received, correct to the nearest vote.
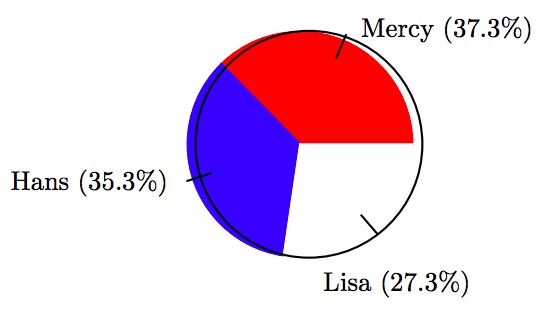
16. In an election for class president, the vote distribution among three candidates is shown in the following pie chart.
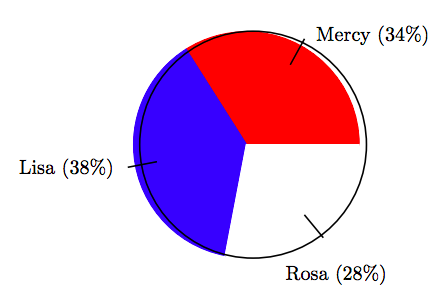
If there were a total of 65 votes cast in the election, find the number of votes that Mercy received, correct to the nearest vote.
17. In an election for class president, the vote distribution among three candidates is shown in the following pie chart.
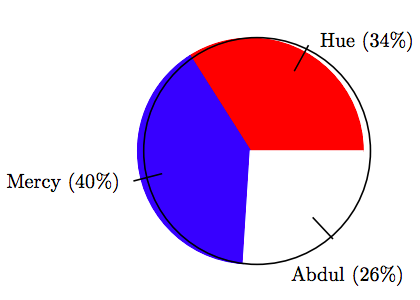
If there were a total of 95 votes cast in the election, find the number of votes that Hue received, correct to the nearest vote.
18. In an election for class president, the vote distribution among three candidates is shown in the following pie chart.
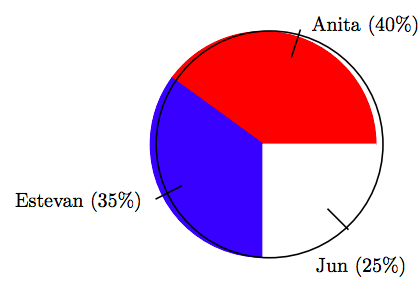
If there were a total of 75 votes cast in the election, find the number of votes that Anita received, correct to the nearest vote.
19. In an election for class president, the vote distribution among three candidates is shown in the following table.
\[ \begin{array}{c|c|c|c} \text{Candidate } & \text{ Votes } \\ \hline \text{Ali } & 45 \\ \text{Jamal } & 34 \\ \text{Jun } & 52 \\ \hline \end{array}\nonumber \]
Use a protractor to help create a pie chart showing the distribution of votes.
20. In an election for class president, the vote distribution among three candidates is shown in the following table.
\[ \begin{array}{c|c|c|c} \text{Candidate } & \text{ Votes } \\ \hline \text{Aisha } & 39 \\ \text{Akbar } & 31 \\ \text{Fernando } & 36 \\ \hline \end{array}\nonumber \]
Use a protractor to help create a pie chart showing the distribution of votes.
21. In an election for class president, the vote distribution among three candidates is shown in the following table.
\[ \begin{array}{c|c|c|c} \text{Candidate } & \text{ Votes } \\ \hline \text{Bernardo } & 44 \\ \text{Rosa } & 40 \\ \text{Abdul } & 58 \\ \hline \end{array}\nonumber \]
Use a protractor to help create a pie chart showing the distribution of votes.
22. In an election for class president, the vote distribution among three candidates is shown in the following table.
\[ \begin{array}{c|c|c|c} \text{Candidate } & \text{ Votes } \\ \hline \text{Estevan } & 46 \\ \text{Ali } & 58 \\ \text{Henry } & 49 \\ \hline \end{array}\nonumber \]
Use a protractor to help create a pie chart showing the distribution of votes.
23. In an election for class president, the vote distribution among three candidates is shown in the following table.
\[ \begin{array}{c|c|c|c} \text{Candidate } & \text{ Votes } \\ \hline \text{Mercy } & 56 \\ \text{Hans } & 53 \\ \text{Lisa } & 41 \\ \hline \end{array}\nonumber \]
Use a protractor to help create a pie chart showing the distribution of votes.
24. In an election for class president, the vote distribution among three candidates is shown in the following table.
\[ \begin{array}{c|c|c|c} \text{Candidate } & \text{ Votes } \\ \hline \text{Estevan } & 60 \\ \text{Hue } & 33 \\ \text{Aisha } & 31 \\ \hline \end{array}\nonumber \]
Use a protractor to help create a pie chart showing the distribution of votes.
25. In an election for class president, the vote distribution among three candidates is shown in the following table.
\[ \begin{array}{c|c|c|c} \text{Candidate } & \text{ Votes } \\ \hline \text{Raven } & 43 \\ \text{Mabel } & 40 \\ \text{Bernardo } & 52 \\ \hline \end{array}\nonumber \]
Use a protractor to help create a pie chart showing the distribution of votes.
26. In an election for class president, the vote distribution among three candidates is shown in the following table.
\[ \begin{array}{c|c|c|c} \text{Candidate } & \text{ Votes } \\ \hline \text{Hue } & 48 \\ \text{Lisa } & 48 \\ \text{Akbar } & 47 \end{array}\nonumber \]
Use a protractor to help create a pie chart showing the distribution of votes.
27. In an election for class president, the vote distribution among three candidates is shown in the following table.
\[ \begin{array}{c|c|c|c} \text{Candidate } & \text{ Votes } \\ \hline \text{Jun } & 57 \\ \text{Lisa } & 30 \\ \text{Aisha } & 58 \\ \hline \end{array}\nonumber \]
Use a protractor to help create a pie chart showing the distribution of votes.
28. In an election for class president, the vote distribution among three candidates is shown in the following table.
\[ \begin{array}{c|c|c|c} \text{Candidate } & \text{ Votes } \\ \hline \text{Bernardo } & 54 \\ \text{Mabel } & 38 \\ \text{Henry } & 49 \\ \hline \end{array}\nonumber \]
Use a protractor to help create a pie chart showing the distribution of votes.
29. In an election for class president, the vote distribution among three candidates is shown in the following table.
\[ \begin{array}{c|c|c|c} \text{Candidate } & \text{ Votes } \\ \hline \text{Henry } & 35 \\ \text{Bernardo } & 32 \\ \text{Estevan } & 47 \\ \hline \end{array}\nonumber \]
Use a protractor to help create a pie chart showing the distribution of votes.
30. In an election for class president, the vote distribution among three candidates is shown in the following table.
\[ \begin{array}{c|c|c|c} \text{Candidate } & \text{ Votes } \\ \hline \text{Bernardo } & 38 \\ \text{Fernando } & 49 \\ \text{Aisha } & 44 \\ \hline \end{array}\nonumber \]
Use a protractor to help create a pie chart showing the distribution of votes.
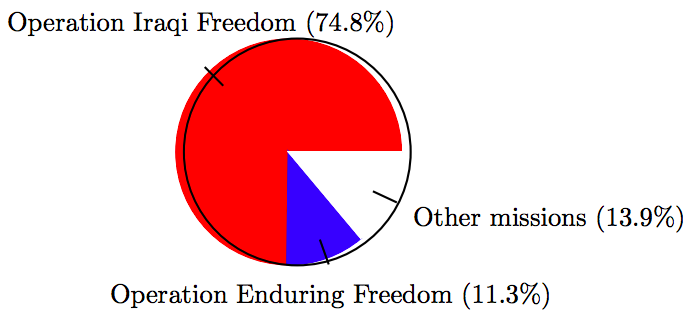
31. Guard deployment. The table shows the number of guard troop services since Sept. 11, 2001 (as of Dec. 2008; some troops have activated multiple times). Associated Press-Times-Standard 02/18/10 Guard troops wait for promised pay.
\[ \begin{array}{c|c|c|c} \text{Mission } & \text{ Troops } \\ \hline \text{Operation Iraqi Freedom } & 193,598 \\ \text{Operation Enduring Freedom } & 29,212 \\ \text{Other missions } & 35,849 \\ \hline \end{array}\nonumber \]
Use a protractor to help create a pie chart showing the distribution of National Guard troops.