4.8: Rational Functions
- Page ID
- 33309
Skills to Develop
- Use arrow notation.
- Solve applied problems involving rational functions.
- Find the domains of rational functions.
- Identify vertical asymptotes.
- Identify horizontal asymptotes.
- Graph rational functions.
Suppose we know that the cost of making a product is dependent on the number of items, \(x\), produced, given by the equation \(C (x)=15,000x−0.1x^2+1000.\) If we want to know the average cost for producing \(x\) items, we would divide the cost function by the number of items, \(x\).
The average cost function, which yields the average cost per item for \(x\) items produced, is
Many other application problems require finding an average value in a similar way, giving us variables in the denominator. Written without a variable in the denominator, this function will contain a negative integer power.
In the last few sections, we have worked with polynomial functions, which are functions with non-negative integers for exponents. In this section, we explore rational functions, which have variables in the denominator.
Using Arrow Notation
We have seen the graphs of the basic reciprocal function and the squared reciprocal function from our study of toolkit functions. Examine these graphs, as shown in Figure \(\PageIndex{1}\), and notice some of their features.
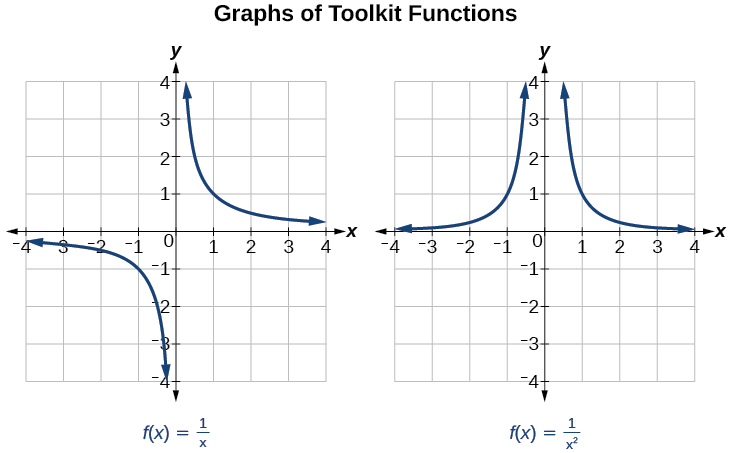
Figure \(\PageIndex{1}\).
Several things are apparent if we examine the graph of \(f(x)=\frac{1}{x}\).
- On the left branch of the graph, the curve approaches the \(x\)-axis \((y=0)\) as \(x\rightarrow -\infty\).
- As the graph approaches \(x = 0\) from the left, the curve drops, but as we approach zero from the right, the curve rises.
- Finally, on the right branch of the graph, the curves approaches the \(x\)-axis \((y=0) \) as \(x\rightarrow \infty\).
To summarize, we use arrow notation to show that \(x\) or \(f (x)\) is approaching a particular value. See Table \(\PageIndex{1}\).
Table \(\PageIndex{1}\).
| Symbol | Meaning |
|---|---|
| \(x\rightarrow a^-\) | \(x\) approaches \(a\) from the left (\(x<a\) but close to \(a\) ) |
| \(x\rightarrow a^+\) | \(x\) approaches \(a\) from the right (\(x>a\) but close to \(a\) ) |
| \(x\rightarrow a\) | \(x\) approaches \(a\) from both left and right (\(x \neq a\) but close to \(a\) ) |
| \(x\rightarrow \infty\) | \(x\) approaches infinity (\(x\) increases without bound) |
| \(x\rightarrow −\infty\) | \(x\) approaches negative infinity (\(x\) decreases without bound) |
| \(f(x)\rightarrow \infty\) | the output approaches infinity (the output increases without bound) |
| \(f(x)\rightarrow −\infty\) | the output approaches negative infinity (the output decreases without bound) |
| \(f(x)\rightarrow b\) | the output approaches \(b\) |
Local Behavior of \(f(x)=\frac{1}{x}\)
Let’s begin by looking at the reciprocal function, \(f(x)=\frac{1}{x}\). We cannot divide by zero, which means the function is undefined at \(x=0\); so zero is not in the domain. As the input values approach zero from the left side (negative values that are very small in magnitude), the function values decrease without bound (in other words, they approach negative infinity). We can see this behavior in Table \(\PageIndex{2}\).
| \(x\) | –0.1 | –0.01 | –0.001 | –0.0001 |
|---|---|---|---|---|
| \(f(x)=\frac{1}{x}\) | –10 | –100 | –1000 | –10,000 |
We write in arrow notation
As the input values approach zero from the right side (positive values that are very small in magnitude), the function values increase without bound (approaching infinity). We can see this behavior in Table \(\PageIndex{3}\).
| \(x\) | 0.1 | 0.01 | 0.001 | 0.0001 |
|---|---|---|---|---|
| \(f(x)=\frac{1}{x}\) | 10 | 100 | 1000 | 10,000 |
We write in arrow notation
See Figure \(\PageIndex{2}\).
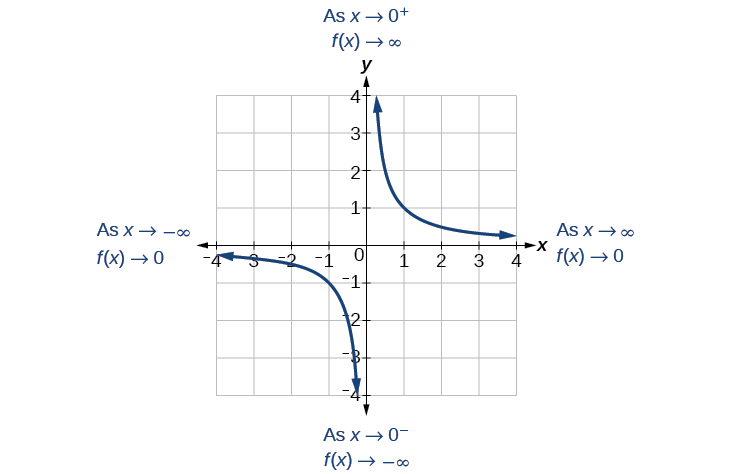
Figure \(\PageIndex{2}\).
This behavior creates a vertical asymptote, which is a vertical line that the graph approaches but never crosses. In this case, the graph is approaching the vertical line \(x=0\) as the input becomes close to zero. See Figure \(\PageIndex{3}\).
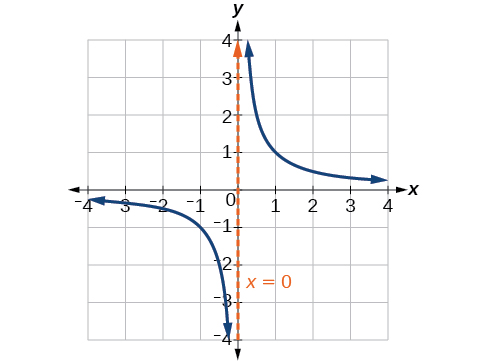
Figure \(\PageIndex{3}\).
VERTICAL ASYMPTOTE
A vertical asymptote of a graph is a vertical line \(x=a\) where the graph tends toward positive or negative infinity as the inputs approach \(a\) from either or both sides of \(a\). In the case when the graph heads in the same direction from both sides, we write
End Behavior of \(f(x)=\frac{1}{x}\)
As the values of \(x\) approach infinity, the function values approach \(0\). As the values of \(x\) approach negative infinity, the function values approach \(0\). See Figure \(\PageIndex{4}\). Symbolically, using arrow notation
As \(x\rightarrow \infty\), \(f(x)\rightarrow 0\), and as \(x\rightarrow −\infty\), \(f(x)\rightarrow 0\).
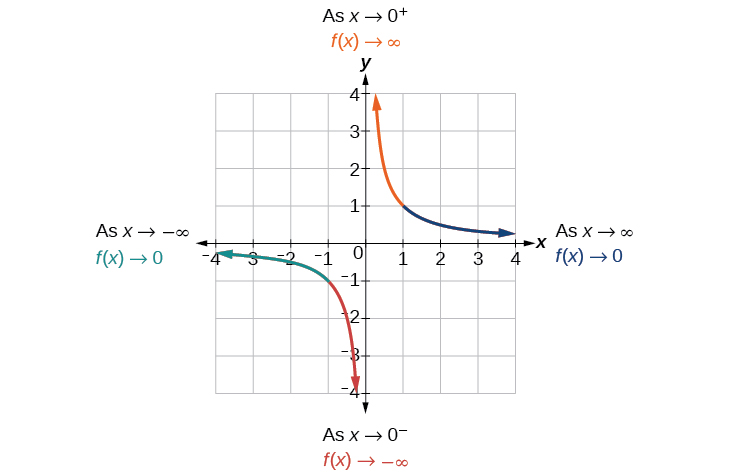
Figure \(\PageIndex{4}\).
Based on this overall behavior and the graph, we can see that the function approaches 0 but never actually reaches 0; it seems to level off as the inputs become large. This behavior creates a horizontal asymptote, a horizontal line that the graph approaches as the input increases or decreases without bound. In this case, the graph is approaching the horizontal line \(y=0\). See Figure \(\PageIndex{5}\).
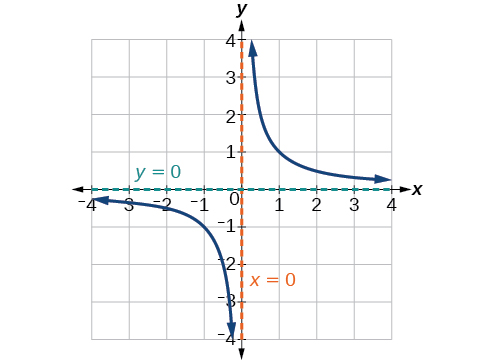
Figure \(\PageIndex{5}\).
HORIZONTAL ASYMPTOTE
A horizontal asymptote of a graph is a horizontal line \(y=b\) where the graph approaches the line as the inputs increase or decrease without bound. We write
Example \(\PageIndex{1}\): Using Arrow Notation.
Use arrow notation to describe the end behavior and local behavior of the function graphed in Figure \(\PageIndex{6}\).
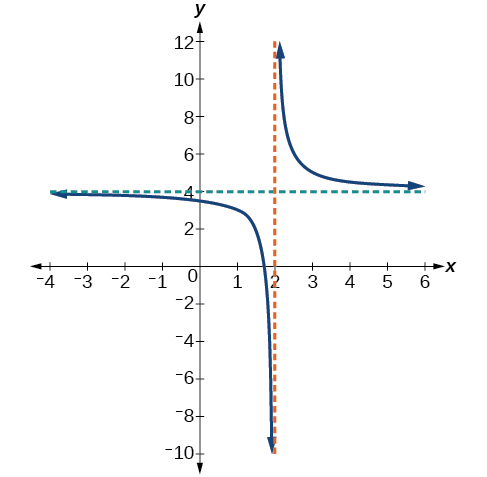
Figure \(\PageIndex{6}\).
Solution:
Notice that the graph is showing a vertical asymptote at \(x=2\), which tells us that the function is undefined at \(x=2\).
And as the inputs decrease without bound, the graph appears to be leveling off at output values of \(4\), indicating a horizontal asymptote at \(y=4\). As the inputs increase without bound, the graph also levels off at \(4\).
Note that the lines representing the asymptotes do not represent points on the graph; however, it is essential to indicate any asymptote that belongs to a graph.
There is an \(x\)-intercept at about \((1.7,0)\) and a \(y\)-intercept at about \((0,3.7)\). There are no turning points.
![]() \(\PageIndex{1}\)
\(\PageIndex{1}\)
Use arrow notation to describe the end behavior and local behavior for the reciprocal squared function.
- Answer
-
End behavior: as \(x\rightarrow \pm \infty\), \(f(x)\rightarrow 0\); Local behavior: as \(x\rightarrow 0\), \(f(x)\rightarrow \infty\) (There are no \(x\)- or \(y\)-intercepts, and no turning points.)
Example \(\PageIndex{2}\): Using Transformations to Graph a Rational Function.
Sketch a graph of the reciprocal function shifted two units to the left and up three units. Identify the horizontal and vertical asymptotes of the graph, if any.
Solution:
Shifting the graph left 2 and up 3 would result in the function
or equivalently, by giving the terms a common denominator,
The graph of the shifted function is displayed in Figure \(\PageIndex{7}\).
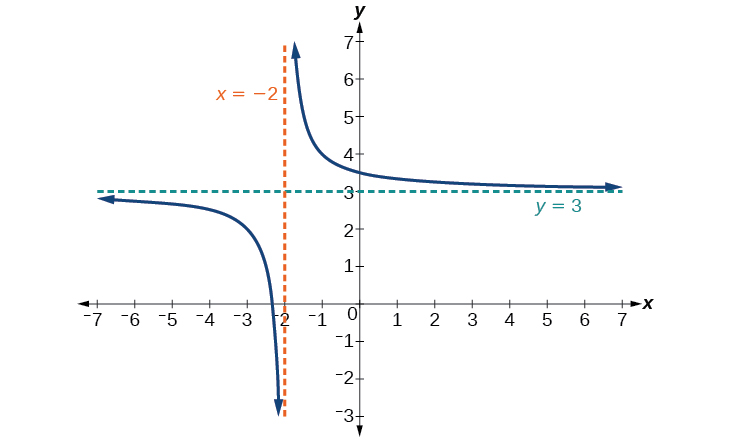
Figure \(\PageIndex{7}\).
Notice that this function is undefined at \(x=−2\), and the graph also is showing a vertical asymptote at \(x=−2\).
As the inputs increase and decrease without bound, the graph appears to be leveling off at output values of 3, indicating a horizontal asymptote at \(y=3\).
Notice that horizontal and vertical asymptotes are shifted left 2 and up 3 along with the function.
![]() \(\PageIndex{2}\)
\(\PageIndex{2}\)
Sketch the graph, and find the horizontal and vertical asymptotes of the reciprocal squared function that has been shifted right 3 units and down 4 units.
- Answer
-
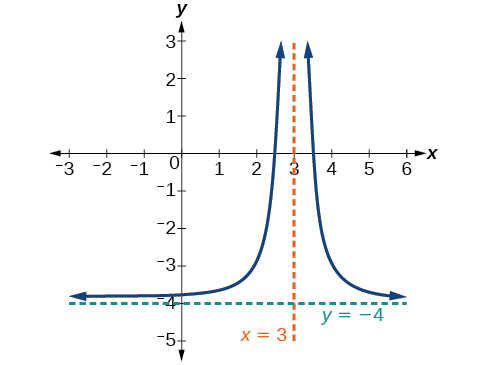
Figure \(\PageIndex{8}\).
The function and the asymptotes are shifted 3 units right and 4 units down. As \(x\rightarrow 3\), \(f(x)\rightarrow \infty\), and as \(x\rightarrow \pm \infty\), \(f(x)\rightarrow −4\).
The function is \(f(x)=\frac{1}{{(x−3)}^2}−4\).
Solving Applied Problems Involving Rational Functions
In Example \(\PageIndex{2}\), we shifted a toolkit function in a way that resulted in the function \(f(x)=\frac{3x+7}{x+2}\). This is an example of a rational function. A rational function is a function that can be written as the quotient of two polynomial functions. Many real-world problems require us to find the ratio of two polynomial functions. Problems involving rates and concentrations often involve rational functions.
RATIONAL FUNCTION
A rational function is a function that can be written as the quotient of two polynomial functions \(P(x)\) and \(Q(x)\).
Example \(\PageIndex{3}\): Solving an Applied Problem Involving a Rational Function
A large mixing tank currently contains 100 gallons of water into which 5 pounds of sugar have been mixed. A tap will open pouring 10 gallons per minute of water into the tank at the same time sugar is poured into the tank at a rate of 1 pound per minute. Find the concentration (pounds per gallon) of sugar in the tank after 12 minutes. Is that a greater concentration than at the beginning?
Solution:
Let \(t\) be the number of minutes since the tap opened. Since the water increases at 10 gallons per minute, and the sugar increases at 1 pound per minute, these are constant rates of change. This tells us the amount of water in the tank is changing linearly, as is the amount of sugar in the tank. We can write an equation independently for each:
The concentration, \(C\), will be the ratio of pounds of sugar to gallons of water
The concentration after 12 minutes is given by evaluating \(C(t)\) at \(t= 12\).
This means the concentration is 17 pounds of sugar to 220 gallons of water.
At the beginning, the concentration is
Since \(\frac{17}{220}≈0.08>\frac{1}{20}=0.05\), the concentration is greater after 12 minutes than at the beginning.
Analysis
To find the horizontal asymptote, divide the leading coefficient in the numerator by the leading coefficient in the denominator:
\[\dfrac{1}{10}=0.1 \nonumber\]
In this problem, the horizontal asymptote is \(y= 0.1.\) This means the concentration \(C,\) the ratio of pounds of sugar to gallons of water, will approach 0.1 in the long term.
![]() \(\PageIndex{3}\)
\(\PageIndex{3}\)
There are 1,200 freshmen and 1,500 sophomores at a prep rally at noon. After 12 p.m., 20 freshmen arrive at the rally every five minutes while 15 sophomores leave the rally. Find the ratio of freshmen to sophomores at 1 p.m.
- Answer
-
\(\frac{12}{11}\)
Finding the Domains of Rational Functions
A vertical asymptote indicates a value at which a rational function is undefined, so that value is not in the domain of the function. A reciprocal function cannot have values in its domain that cause the denominator to equal zero. In general, to find the domain of a rational function, we need to determine which inputs would cause division by zero.
DOMAIN OF A RATIONAL FUNCTION
The domain of a rational function includes all real numbers except those that cause the denominator to equal zero.
![]() Given a rational function, find the domain.
Given a rational function, find the domain.
- Set the denominator equal to zero.
- Solve to find the \(x\)-values that cause the denominator to equal zero.
- The domain is all real numbers except those found in Step 2.
Example \(\PageIndex{4}\): Finding the Domain of a Rational Function
Find the domain of \(f(x)=\dfrac{x+3}{x^2−9}\).
Solution:
Begin by setting the denominator equal to zero and solving.
The denominator is equal to zero when \(x=\pm 3\). The domain of the function is all real numbers except \(x=\pm 3\).
A graph of this function, as shown in Figure \(\PageIndex{9}\), confirms that the function is not defined when \(x=\pm 3\).

Figure \(\PageIndex{9}\).
There is a vertical asymptote at \(x=3\) and a hole in the graph at \(x=−3\). We will discuss these types of holes in greater detail later in this section.
![]() \(\PageIndex{4}\)
\(\PageIndex{4}\)
Find the domain of \(f(x)=\frac{4x}{5(x−1)(x−5)}\).
- Answer
-
The domain is all real numbers except \(x=1\) and \(x=5\).
Identifying Vertical Asymptotes of Rational Functions
By looking at the graph of a rational function, we can investigate its local behavior and easily see whether there are asymptotes. We may even be able to approximate their location. Even without the graph, however, we can still determine whether a given rational function has any asymptotes, and calculate their location.
Vertical Asymptotes
The vertical asymptotes of a rational function may be found by examining the factors of the denominator that are not common to the factors in the numerator. Vertical asymptotes occur at the zeros of such factors.
![]() Given a rational function, identify any vertical asymptotes of its graph
Given a rational function, identify any vertical asymptotes of its graph
- Factor the numerator and denominator.
- Note any restrictions in the domain of the function.
- Reduce the expression by canceling common factors in the numerator and the denominator.
- Note any values that cause the denominator to be zero in this simplified version. These are where the vertical asymptotes occur.
- Note any restrictions in the domain where asymptotes do not occur. These are removable discontinuities, or “holes.”
Example \(\PageIndex{5}\): Identifying Vertical Asymptotes
Find the vertical asymptotes of the graph of \(k(x)=\frac{5+2x^2}{2−x−x^2}\).
Solution:
First, factor the numerator and denominator.
To find the vertical asymptotes, we determine where this function will be undefined by setting the denominator equal to zero:
\[(2+x)(1−x)=0 \nonumber\]
\[x=−2, \mbox{ or }1 \nonumber\]

Removable Discontinuities
Occasionally, a graph will contain a hole: a single point where the graph is not defined, indicated by an open circle. We call such a hole a removable discontinuity. We have already seen a removable discontinuity, in Figure 4.8.9, at \(x=-3\).
For example, the function \(f(x)=\frac{x^2−1}{x^2−2x−3}\) may be re-written by factoring the numerator and the denominator.
Notice that \(x+1\) is a common factor to the numerator and the denominator. The zero of this factor, \(x=−1\), is the location of the removable discontinuity. Notice also that \(x–3\) is not a factor in both the numerator and denominator. The zero of this factor, \(x=3\), is the vertical asymptote. See Figure \(\PageIndex{11}\). (Note that removable discontinuities may not be visible when we use a graphing calculator, depending upon the window selected.)
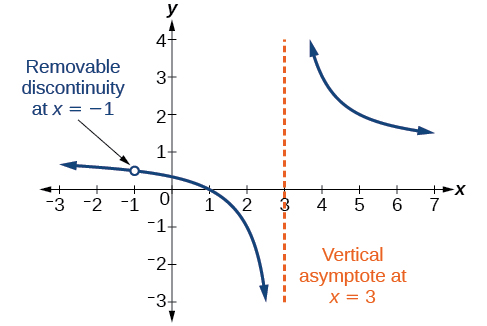
Figure \(\PageIndex{11}\).
REMOVABLE DISCONTINUITIES OF RATIONAL FUNCTIONS
A removable discontinuity may occur in the graph of a rational function at \(x=a\) if \(a\) is a zero for a factor in the denominator that is common with a factor in the numerator. Factor the numerator and denominator and check for common factors. If there are any, set the common factor equal to 0 and solve. If the multiplicity of this factor is greater than or equal to that in the denominator, this is the location of a removable discontinuity. If the multiplicity of the factor is greater in the denominator than in the numerator, then there is still an asymptote at \(x=a\).
Example \(\PageIndex{6}\): Identifying Vertical Asymptotes and Removable Discontinuities for a Graph
Find the vertical asymptotes and removable discontinuities of the graph of \(k(x)=\frac{x−2}{x^2−4}\).
Solution:
Factor the numerator and the denominator.
\((x–2)\) is a common factor in the numerator and the denominator. The zero for this factor is \(x=2\). Since the multiplicities of \((x-2)\) in both the numerator and denominator are equal, this is the location of a removable discontinuity.
The factor \((x+2)\) is in the denominator but is not in the numerator. The zero for this factor is \(x=−2\). The vertical asymptote is \(x=−2\). See Figure \(\PageIndex{12}\).
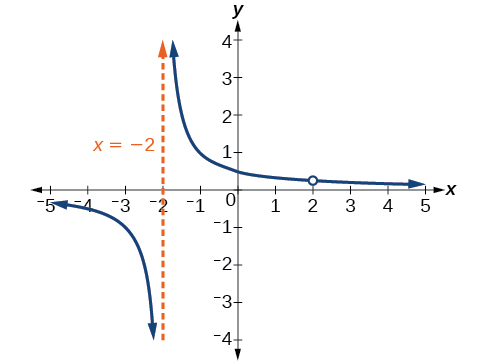
Figure \(\PageIndex{12}\).
The graph of this function has a vertical asymptote at \(x=−2\), but at \(x=2\) the graph has a hole.
![]() \(\PageIndex{5}\)
\(\PageIndex{5}\)
Find the vertical asymptotes and removable discontinuities of the graph of \(f(x)=\frac{x^2−25}{x^3−6x^2+5x}\).
- Answer
-
Removable discontinuity at \(x=5\). Vertical asymptotes: \(x=0\), \(x=1\).
Identifying Horizontal Asymptotes of Rational Functions
While vertical asymptotes describe the behavior of a graph as the output increases or decreases without bound, horizontal asymptotes help describe the behavior of a graph as the input approaches infinity or negative infinity. Recall that a polynomial’s end behavior will mirror that of the leading term. Likewise, a rational function’s end behavior will mirror that of the ratio of the numerator and the denominator of the leading terms.
There are four distinct outcomes when checking for horizontal asymptotes:
Case 1: If the degree of the denominator is greater than the degree of the numerator, there is a horizontal asymptote at \(y=0\).
In this case, the end behavior is \(f(x)≈\frac{4x}{x^2}=\frac{4}{x}\) (looking at the ratio of the leading term of the numerator to the leading term of the denominator). This tells us that, as the inputs increase or decrease without bound, this function will behave similarly to the function \(g(x)=\frac{4}{x}\), and the outputs will approach zero, resulting in a horizontal asymptote at \(y=0\). See Figure \(\PageIndex{13}\). Note that this graph crosses the horizontal asymptote.
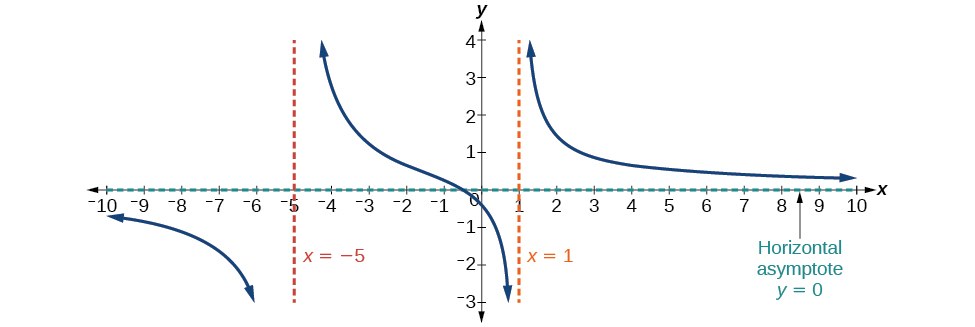
Figure \(\PageIndex{13}\): Horizontal asymptote \(y=0\) when \(f(x)=\dfrac{p(x)}{q(x)}\), where degree of \(p\) < degree of \(q\).
Case 2: If the degree of the denominator equals the degree of the numerator, there is a horizontal asymptote at \(y=\dfrac{a_n}{b_n}\), where \(a_n\) and \(b_n\) are respectively the leading coefficients of \(p(x)\) and \(q(x)\) for \(f(x)=\dfrac{p(x)}{q(x)}\).
In this case, the end behavior is \(f(x)≈\dfrac{3x^2}{x^2}=3\). This tells us that as the inputs grow large in magnitude, this function will behave like the function \(g(x)=3\), which is a horizontal line. As \(x\rightarrow \pm \infty\), \(f(x)\rightarrow 3\), resulting in a horizontal asymptote at \(y=3\). See Figure \(\PageIndex{14}\).
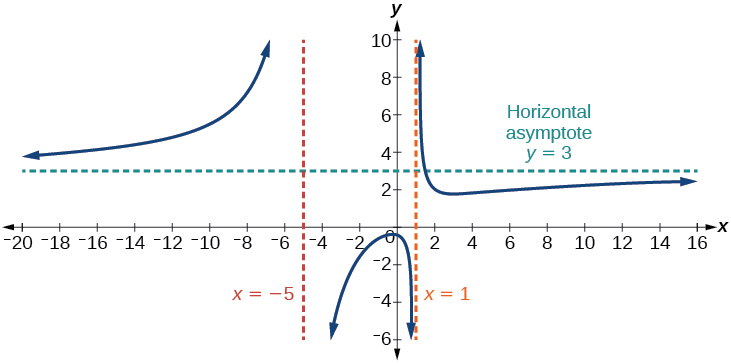
Figure \(\PageIndex{14}\): Horizontal asymptote when \(f(x)=\frac{p(x)}{q(x)}\), where degree of \(p\) =degree of \(q\).
Notice that this graph crosses the horizontal asymptote. While the graph of a rational function will never cross a vertical asymptote, the graph may or may not cross a horizontal asymptote. Also, although the graph of a rational function may have many vertical asymptotes, the graph will have at most one horizontal asymptote.
Case 3: If the degree of the denominator is less than the degree of the numerator by exactly one, we get a slant asymptote.
In this case, the end behavior is \(f(x)≈\frac{3x^2}{x}=3x\). This tells us that as the inputs increase or decrease without bound, this function will behave similarly to the function \(g(x)=3x\). As the inputs grow large, the outputs will grow and not level off, so this graph has no horizontal asymptote. However, the graph of \(g(x)=3x\) is a line with a slope of 3, and since \(f\) will behave similarly to \(g\), it will approach a line whose slope is 3. This line is a slant asymptote.
To find the equation of the slant asymptote, divide \(\frac{3x^2−2x+1}{x−1}\). The quotient is \(3x+1\), with a remainder of 2. The slant asymptote is the graph of the line \(g(x)=3x+1\). Note also that there is a vertical asymptote at \(x=1.\) See Figure \(\PageIndex{15}\).

Figure \(\PageIndex{15}\): Slant asymptote when \(f(x)=\frac{p(x)}{q(x)}\), where degree of \(p\) >degree of \(q\) by 1.
Case 4: If the degree of the numerator is larger than the degree of the denominator by more than one, the graph does not have a horizontal asymptote. The end behavior of the graph will mimic the behavior of the reduced end behavior fraction. For instance, if we had the function
with end behavior
the end behavior of the graph would look similar to that of an even polynomial with a positive leading coefficient.
HORIZONTAL ASYMPTOTES OF RATIONAL FUNCTIONS
The horizontal asymptote of a rational function can be determined by looking at the degrees of the numerator and denominator.
- Degree of numerator is less than degree of denominator: horizontal asymptote at \(y=0\).
- Degree of numerator is equal to degree of denominator: horizontal asymptote at ratio of leading coefficients, \(y=\dfrac{a_n}{b_n}\).
- Degree of numerator is greater than degree of denominator by one: no horizontal asymptote; slant asymptote.
- Degree of numerator is larger than degree of the denominator by more than one: no horizontal asymptote.
Example \(\PageIndex{7}\): Identifying Horizontal and Slant Asymptotes
For the functions listed, identify the horizontal or slant asymptote.
- \(g(x)=\dfrac{6x^3−10x}{2x^3+5x^2}\)
- \(h(x)=\dfrac{x^2−4x+1}{x+2}\)
- \(k(x)=\dfrac{x^2+4x}{x^3−8}\)
Solution:
For these solutions, we will refer to the polynomial in the numerator as \(p(x)\) and the polynomial in the denominator as \(q(x)\).
- \(g(x)=\frac{6x^3−10x}{2x^3+5x^2}\): The degree of \(p= 3 =\)degree of \(q\), so we can find the horizontal asymptote by taking the ratio of the leading terms. There is a horizontal asymptote at \(y =\frac{6}{2}\) or \(y=3\).
- \(h(x)=\frac{x^2−4x+1}{x+2}\): The degree of \(p=2\) and degree of \(q=1\). Since \(p>q\) by 1, there is a slant asymptote \(y=x-6\).
- \(k(x)=\frac{x^2+4x}{x^3−8}\) : The degree of \(p=2\) < degree of \(q=3\), so there is a horizontal asymptote \(y=0\).
Example \(\PageIndex{8}\): Identifying Horizontal Asymptotes
Find the horizontal asymptote and interpret it in the context of the problem.
Solution:
Both the numerator and denominator are linear (degree 1). Because the degrees are equal, there will be a horizontal asymptote at the ratio of the leading coefficients. In the numerator, the leading term is \(t\), with coefficient 1. In the denominator, the leading term is \(10t\), with coefficient 10. The horizontal asymptote will be at the ratio of these values:
This function will have a horizontal asymptote at \(y=\frac{1}{10}\).
This tells us that as the values of \(t\) increase, the values of \(C\) will approach \(\frac{1}{10}\). In context, this means that, as more time goes by, the concentration of sugar in the tank will approach one-tenth of a pound of sugar per gallon of water or \(\frac{1}{10}\) pounds per gallon.
Example \(\PageIndex{9}\): Identifying Horizontal and Vertical Asymptotes
Find the horizontal and vertical asymptotes of the function \[f(x)=\dfrac{(x−2)(x+3)}{(x−1)(x+2)(x−5)} \nonumber\]
Solution:
First, note that this function has no common factors, so there are no potential removable discontinuities.
The function will have vertical asymptotes when the denominator is zero, causing the function to be undefined. The denominator will be zero at \(x=1, -2,\) and \(5\), indicating vertical asymptotes at these values.
The numerator has degree \(2\), while the denominator has degree 3. Since the degree of the denominator is greater than the degree of the numerator, the denominator will grow faster than the numerator, causing the outputs to tend towards zero as the inputs get large, and so as \(x\rightarrow \pm \infty\), \(f(x)\rightarrow 0\). This function will have a horizontal asymptote at \(y =0.\) Figure \(\PageIndex{16}\) shows a graph of the function, created using technology.
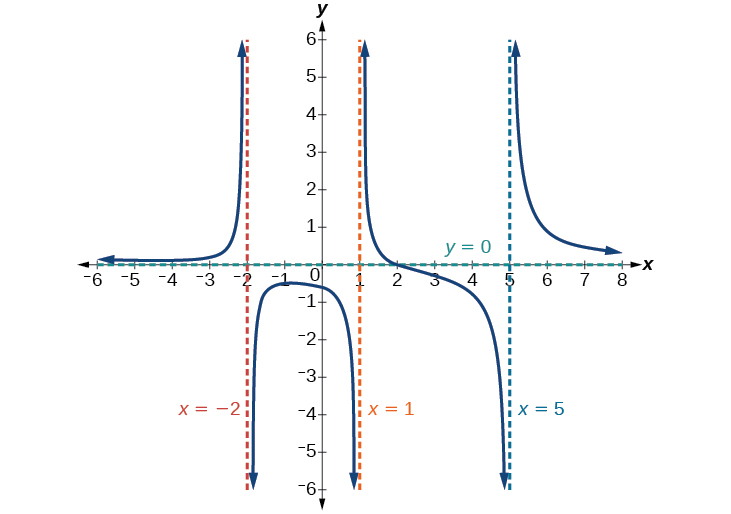
Figure \(\PageIndex{16}\).
![]() \(\PageIndex{6}\)
\(\PageIndex{6}\)
Find the vertical and horizontal asymptotes of the function:
\(f(x)=\dfrac{(2x−1)(2x+1)}{(x−2)(x+3)}\)
- Answer
-
Vertical asymptotes at \(x=2\) and \(x=-3\); horizontal asymptote at \(y =4\).
INTERCEPTS OF RATIONAL FUNCTIONS
A rational function will have a \(y\)-intercept at \(f(0),\) if the function is defined at zero. A rational function will not have a \(y\)-intercept if the function is not defined at zero.
Likewise, a rational function will have \(x\)-intercepts at the inputs that cause the output to be zero. A fraction is only equal to zero when the numerator is zero; thus, \(x\)-intercepts can only occur when the numerator of the rational function is equal to zero.
Example \(\PageIndex{10}\): Finding the Intercepts of a Rational Function
Find the intercepts of \(f(x)=\dfrac{(x−2)(x+3)}{(x−1)(x+2)(x−5)}\) (a continued analysis of the same function we saw in Example 4.8.9).
Solution:
We can find the \(y\)-intercept by evaluating the function at zero.
The \(x\)-intercepts will occur when the function is equal to zero:
The \(y\)-intercept is \((0,–0.6)\), the \(x\)-intercepts are \((2,0)\) and \((–3,0)\). See Figure \(\PageIndex{17}\).
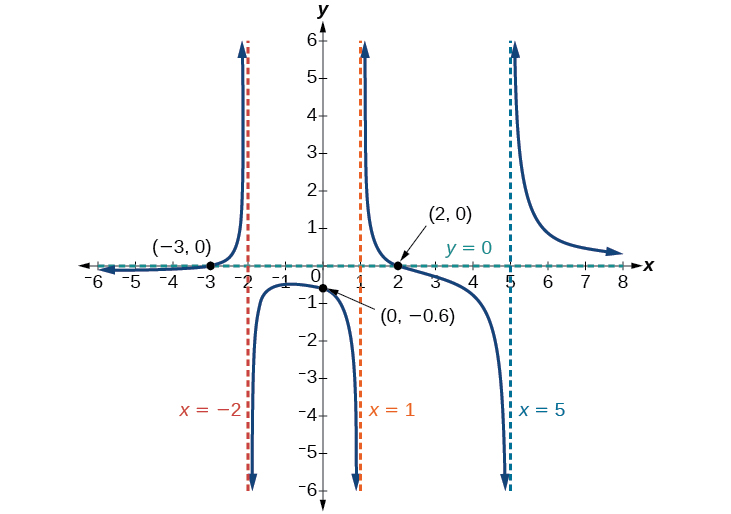
Figure \(\PageIndex{17}\).
![]() \(\PageIndex{7}\)
\(\PageIndex{7}\)
Given the reciprocal squared function that is shifted right 3 units and down 4 units, write this as a rational function. Then, find the \(x\)- and \(y\)-intercepts and the horizontal and vertical asymptotes.
- Answer
-
For the transformed reciprocal squared function, we find the rational form.
\(f(x)=\dfrac{1}{{(x−3)}^2}−4=\dfrac{1−4{(x−3)}^2}{{(x−3)}^2}=\dfrac{1−4(x^2−6x+9)}{(x−3)(x−3)}=\dfrac{−4x^2+24x−35}{x^2−6x+9}\)
Because the numerator is the same degree as the denominator we know that as \(x\rightarrow \pm \infty\), \(f(x)\rightarrow −4\); so \(y=–4\) is the horizontal asymptote. Next, we set the denominator equal to zero, and find that the vertical asymptote is \(x=3\), because as \(x\rightarrow 3\), \(f(x)\rightarrow \infty\). We then set the numerator equal to \(0\) and find the x-intercepts are at \((2.5,0)\) and \((3.5,0)\). Finally, we evaluate the function at 0 and find the \(y\)-intercept to be at \((0,−\frac{35}{9})\).
Graphing Rational Functions
Note: just as when we discussed graphing polynomial functions in Section 4.5, we will need tools from calculus in order to find the exact location of any turning points; so while our sketch will be as correct as we can make it, it may not be entirely accurate.
In Example \(\PageIndex{10}\), we see that the factors of the numerator of a rational function reveal the \(x\)-intercepts of the graph, whereas the factors of the denominator reveal the vertical asymptotes of the graph. The factors of the numerator may have multiplicity greater than one. The effect on the shape of the graph at those \(x\)-intercepts is the same as we saw with polynomial functions. Factors with odd multiplicity will cause the graph to cross the \(x\)-axis, while factors with even multiplicity will cause the graph to "bounce off" the \(x\)-axis.
The vertical asymptotes associated with the factors of the denominator will mirror one of the two toolkit reciprocal functions. When the degree of the factor in the denominator is odd, the distinguishing characteristic will be like the function \(\dfrac{1}{x}\). On one side of the vertical asymptote the graph heads towards positive infinity, and on the other side the graph heads towards negative infinity. See Figure \(\PageIndex{18}\).

Figure \(\PageIndex{18}\).
When the degree of the factor in the denominator is even, the distinguishing characteristic will be like the function \(\dfrac{1}{x^2}\). The graph either heads toward positive infinity on both sides of the vertical asymptote or heads toward negative infinity on both sides. See Figure \(\PageIndex{19}\).

Figure \(\PageIndex{19}\).
For example, the graph of \(f(x)=\dfrac{{(x+1)}^2(x−3)}{{(x+3)}^2(x−2)}\) is shown in Figure \(\PageIndex{20}\).

Figure \(\PageIndex{20}\).
- At the \(x\)-intercept \(x=−1\) corresponding to the \({(x+1)}^2\) factor of the numerator, the graph "bounces", consistent with the quadratic nature of the factor.
- At the \(x\)-intercept \(x=3\) corresponding to the \((x−3)\) factor of the numerator, the graph passes through the axis as we would expect from a linear factor.
- At the vertical asymptote \(x=−3\) corresponding to the \({(x+3)}^2\) factor of the denominator, the graph heads towards positive infinity on both sides of the asymptote, consistent with the behavior of the function \(f(x)=\frac{1}{x^2}\).
- At the vertical asymptote \(x=2\), corresponding to the \((x−2)\) factor of the denominator, the graph heads towards positive infinity on the left side of the asymptote and towards negative infinity on the right side, consistent with the behavior of the function \(f(x)=\frac{1}{x}\).
![]() Given a rational function, sketch a possible graph.
Given a rational function, sketch a possible graph.
- Evaluate the function at \(x=0\) (if zero is in the domain) to find the\(y\)-coordinate of the \(y\)-intercept.
- Factor the numerator and denominator.
- For factors in the numerator not common to the denominator, determine where each factor of the numerator is zero to find the \(x\)-coordinates of the \(x\)-intercepts.
- Find the multiplicities of the \(x\)-intercepts to determine the behavior of the graph at those points.
- For factors in the denominator, note the multiplicities of the zeros to determine the local behavior. For those factors not common to the numerator, find the vertical asymptotes by setting those factors equal to zero and then solve.
- For factors in the denominator common to factors in the numerator, find the removable discontinuities by setting those factors equal to zero and then solve.
- Compare the degrees of the numerator and the denominator to determine the horizontal or slant asymptotes.
- Sketch the graph.
Example \(\PageIndex{11}\): Graphing a Rational Function
Sketch a possible graph of \(f(x)=\dfrac{(x+2)(x−3)}{{(x+1)}^2(x−2)}\).
Solution:
We start by noting that the function is already factored, saving us a step.
Next, we find the intercepts. Evaluating the function at zero gives the \(y\)-coordinate of the \(y\)-intercept:
To find the \(x\)-coordinates of the \(x\)-intercepts, we determine when the numerator of the function is zero. Setting each factor equal to zero, we find \(x\)-intercepts at \(x=–2\) and \(x=3\). At each, the behavior will be linear (multiplicity 1), with the graph passing through the intercept.
We have a \(y\)-intercept at \((0,3)\) and \(x\)-intercepts at \((–2,0)\) and \((3,0)\).
There are no common factors in the numerator and denominator. This means there are no removable discontinuities.
To find the vertical asymptotes, we determine where the denominator is equal to zero. This occurs when \(x+1=0\) and when \(x - 2=0\), giving us vertical asymptotes with equations \(x=-1\) and \(x=2\).
Finally, the degree of denominator is larger than the degree of the numerator, telling us this graph has a horizontal asymptote whose equation is \(y =0\).
To sketch the graph, we might start by plotting the three intercepts. Since the graph has no \(x\)-intercepts between the vertical asymptotes, and the \(y\)-intercept is positive, we know the function must remain positive between the asymptotes, letting us fill in the middle portion of the graph as shown in Figure \(\PageIndex{21}\). Note that we have no method to find the exact location of the local minimum, but by testing a few \(x\)-values, we might notice that \(f(1)=1.5\), so there is a local minimum somewhere between \(x=0\) and \(x=2\).
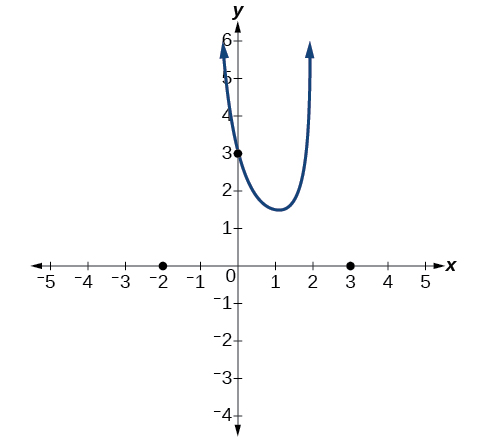
Figure \(\PageIndex{21}\).
The factor associated with the vertical asymptote at \(x=−1\) was squared, so we know the behavior will be the same on both sides of the asymptote. The graph heads toward positive infinity as the inputs approach the asymptote on the right, so the graph will head toward positive infinity on the left as well.
For the vertical asymptote at \(x=2\), the factor was not squared, so the graph will have opposite behavior on either side of the asymptote. Since the graph goes up as \(x \rightarrow 2^-\), the graph must go down as \(x \rightarrow 2^+\). See Figure \(\PageIndex{22}\).
After passing through each of the \(x\)-intercepts, the graph will eventually tend to a \(y\)-value of zero as \(x \rightarrow -\infty\) and as \(x \rightarrow \infty\), as indicated by the horizontal asymptote. Note: The scale of this graph does not allow us to see all of the details. Can you explain why there must be one more local minimum (in Quadrant III), and a local maximum (in Quadrant I)? (If you can, write it up and give it to your instructor for extra credit!)

Figure \(\PageIndex{22}\).
![]() \(\PageIndex{8}\)
\(\PageIndex{8}\)
Given the function \(f(x)=\frac{{(x+2)}^2(x−2)}{2{(x−1)}^2(x−3)}\), use the characteristics of polynomials and rational functions to describe its behavior and sketch a possible graph of the function.
- Answer
-
Horizontal asymptote at \(y=\frac{1}{2}\). Vertical asymptotes at \(x=1\) (multiplicity 2) and \(x=3\) (multiplicity 1). \(y\)-intercept at \((0,\frac{4}{3})\).
\(x\)-intercepts at \((2,0)\) and \((–2,0)\). \((–2,0)\) is a zero with multiplicity \(2\), and the graph bounces off the \(x\)-axis at this point. \((2,0)\) is a single zero and the graph crosses the \(x\)-axis at this point.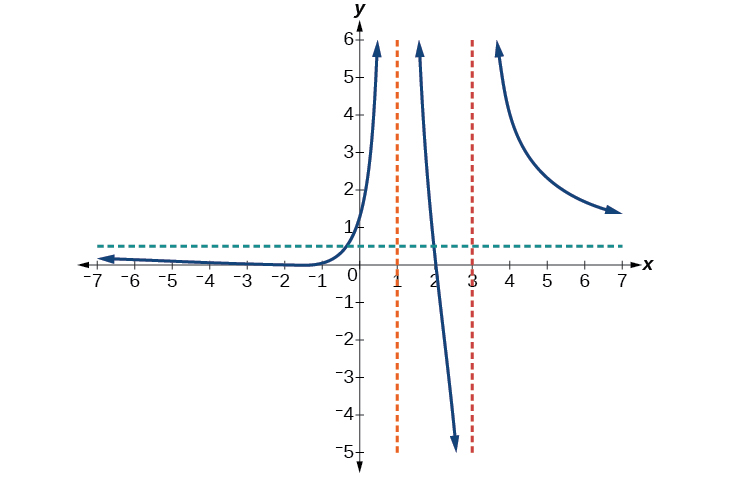
Figure \(\PageIndex{23}\)
Writing Rational Functions
Now that we have analyzed some important equations for rational functions and how they relate to a graph of the function, we can use information given by a graph to write a possible function. A rational function written in factored form will have an \(x\)-intercept where each factor of the numerator is equal to zero. (An exception occurs in the case of a removable discontinuity.) As a result, we can form a numerator of a function whose graph will pass through a set of \(x\)-intercepts by introducing a corresponding set of factors. Likewise, because the function will have a vertical asymptote where each factor of the denominator is equal to zero (again excluding the case of a removable discontinuity), we can form a denominator that will produce the vertical asymptotes by introducing a corresponding set of factors.
WRITING RATIONAL FUNCTIONS FROM INTERCEPTS AND ASYMPTOTES
If a rational function has \(x\)-intercepts at \(x=x_1,x_2,...,x_n\), and vertical asymptotes at \(x=v_1,v_2,…,v_m\), then a possible function can be written in the form:
where the powers \(p_i\) or \(q_i\) on each factor can be determined by the behavior of the graph at the corresponding intercept or asymptote, and the stretch factor \(a\) can be determined either from an input-output pair of the function other than an \(x\)-intercept or from the horizontal asymptote if it is nonzero.
![]() Given a graph of a rational function, write a possible function.
Given a graph of a rational function, write a possible function.
- Determine the factors of the numerator. Examine the behavior of the graph at the \(x\)-intercepts to determine the zeroes and their possible multiplicities. (Try to find the “simplest” function with small multiplicities—such as 1 or 3—rather than choosing larger multiplicities—such as 5 or 7, for example.)
- Determine the factors of the denominator. Examine the behavior on both sides of each vertical asymptote to determine the factors and their multiplicity, again keeping things simple by choosing small multiplicities.
- Use any clear point on the graph to find the stretch factor; or, if there is a horizontal asymptote \(y=k\) with \(k \neq 0\), the stretch factor will be equal to \(k\).
Example \(\PageIndex{12}\): Writing a Rational Function from Intercepts and Asymptotes
Write a possible equation for the rational function shown in Figure \(\PageIndex{24}\).
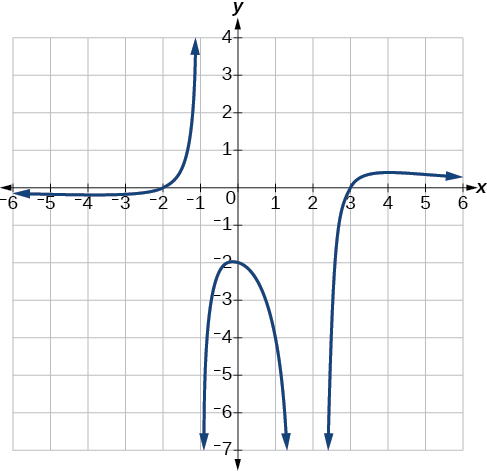
Figure \(\PageIndex{24}\).
Solution:
The graph appears to have \(x\)-intercepts at \(x=-2\) and \(x=3\). At both, the graph passes through the intercept, suggesting linear factors. The graph has two vertical asymptotes. The one at \(x=-1\) exhibits the basic behavior similar to \(\dfrac{1}{x}\), with the graph heading toward positive infinity on one side and heading toward negative infinity on the other. The asymptote at \(x=2\) is exhibiting a behavior similar to \(\dfrac{1}{x^2}\), with the graph heading toward negative infinity on both sides of the asymptote. See Figure \(\PageIndex{25}\).
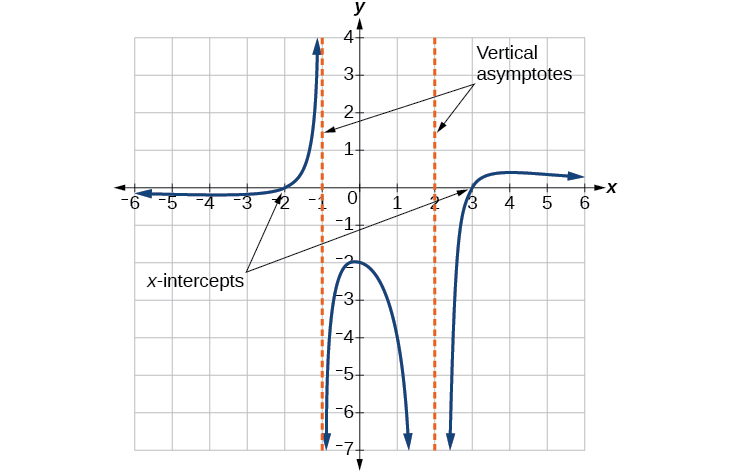
Figure \(\PageIndex{25}\).
We can use this information to write a function of the form
To find the stretch factor, we can use another clear point on the graph, such as the \(y\)-intercept \((0,–2)\).
\[\begin{align*} -2 &= a\dfrac{(0+2)(0−3)}{(0+1)(0−2)^2}\\[2pt] -2 &= a\dfrac{−6}{4}\\[2pt] a &= \dfrac{-8}{-6}=\dfrac{4}{3}\end{align*}\]
This gives us a final function of \(f(x)=\dfrac{4(x+2)(x−3)}{3(x+1){(x−2)}^2}\).
Media
Access these online resources for additional instruction and practice with rational functions.
Key Equations
| Rational Function | \(f(x)=\dfrac{P(x)}{Q(x)}=\dfrac{a_px^p+a_{p−1}x^{p−1}+...+a_1x+a_0}{b_qx^q+b_{q−1}x^{q−1}+...+b_1x+b_0}\), Domain \(= \{x \vert Q(x) \neq 0\}\) |
Key Concepts
- Arrow notation can be used to describe local behavior and end behavior of the toolkit functions \(f(x)=\frac{1}{x}\) and \(f(x)=\frac{1}{x^2}\).
- A function that tends toward positive or negative infinity as the input values approach an \(x\)-value that is not in the domain has a vertical asymptote.
- A function can have more than one vertical asymptote.
- A function that levels off at a horizontal value has a horizontal asymptote.
- The domain of a rational function includes all real numbers except those that cause the denominator to equal zero.
- The vertical asymptotes of a rational function will occur where the denominator of the function is equal to zero and the numerator is not zero.
- A rational functions behavior at a vertical asymptote will mirror \(y=\frac{1}{x}\) if the degree of the factor in the denominator is odd, and will mirror \(y=\frac{1}{x^2}\) if the degree of the factor in the denominator is even.
- A removable discontinuity might occur in the graph of a rational function if an input causes both numerator and denominator to be zero.
- A rational function’s end behavior will mirror that of the ratio of the leading terms of the numerator and denominator functions.
- Graph rational functions by finding the intercepts, behavior at the intercepts and asymptotes, and end behavior.
- If a rational function has \(x\)-intercepts at \(x=x_1,x_2,…,x_n\), vertical asymptotes at \(x=v_1,v_2,…,v_m\), and no \(x_i\) equals any \(v_j\), then the function can be written in the form \[f(x)=a\frac{(x-x_1)^{p_1}(x-x_2)^{p_2}\cdots (x-x_n)^{p_n}}{(x-v_1)^{q_1}(x-v_2)^{q_2}\cdots (x-v_m)^{q_m}}. \nonumber\]
Contributor
Jay Abramson (Arizona State University) with contributing authors. Textbook content produced by OpenStax College is licensed under a Creative Commons Attribution License 4.0 license. Download for free at https://openstax.org/details/books/precalculus.


