4.3: Rational Inequalities and Applications

Math 370 Learning Objectives

• Solve rational inequalities using graphs and/or sign charts
• Build models to solve applications involving rational functions

---

Solving Rational Inequalities

In this section, we solve equations and inequalities involving rational functions and explore associated application problems. Our first example showcases the critical difference in procedure between solving a rational equation and a rational inequality.

Example \( \PageIndex{1} \)

1. Solve \(\frac{x^3-2x+1}{x-1} = \frac{1}{2}x-1\).
2. Solve \(\frac{x^3-2x+1}{x-1} \geq \frac{1}{2}x-1\).
3. Use graphing technology to check your answers to 1 and 2.

Solution

1. To solve the equation, we clear denominators\[\begin{array}{rclr}
   \dfrac{x^3-2x+1}{x-1} & = & \dfrac{1}{2}x-1 & \\
    \left(\dfrac{x^3-2x+1}{x-1}\right) \cdot 2(x-1) & = & \left( \dfrac{1}{2}x-1 \right) \cdot 2(x-1) & \\
   2x^3 - 4x + 2 & = & x^2-3x+2 & \left( \text{expand} \right) \\
   2x^3 -x^2 - x & = & 0 & \\
   x(2x+1)(x-1) & = & 0 & \left( \text{factor} \right) \\
   x & = & -\dfrac{1}{2}, \, 0, \, 1 & \\
   \end{array}\nonumber\]Since we cleared denominators, we need to check for extraneous solutions. Sure enough, we see that \(x=1\) does not satisfy the original equation and must be discarded. Our solutions are \(x=-\frac{1}{2}\) and \(x=0\).
2. To solve the inequality, it may be tempting to begin as we did with the equation \(-\) namely by multiplying both sides by the quantity \((x-1)\). The problem is that, depending on \(x\), \((x-1)\) may be positive (which doesn’t affect the inequality) or \((x-1)\) could be negative (which would reverse the inequality). Instead of working by cases, we collect all of the terms on one side of the inequality with \(0\) on the other and make a sign diagram using the technique given Section 4.2.\[\begin{array}{rclr}
   \dfrac{x^3-2x+1}{x-1} & \geq & \dfrac{1}{2}x-1 & \\
   \dfrac{x^3-2x+1}{x-1} - \dfrac{1}{2} x + 1& \geq & 0& \\
   \dfrac{2\left(x^3-2x+1\right)-x(x-1)+1(2(x-1))}{2(x-1)} & \geq & 0 & \left( \text{get a common denominator} \right) \\
   \dfrac{2x^3-x^2-x}{2x-2} & \geq & 0 & \left( \text{expand} \right) \\
   \end{array}\nonumber\]Viewing the left hand side as a rational function \(r(x)\) we make a sign diagram. The only value excluded from the domain of \(r\) is \(x=1\) which is the solution to \(2x-2=0\). The zeros of \(r\) are the solutions to \(2x^3-x^2-x=0\), which we have already found to be \(x=0\), \(x=-\frac{1}{2}\) and \(x=1\), the latter was discounted as a zero because it is not in the domain. Choosing test values in each test interval, we construct the sign diagram below.

   

   We are interested in where \(r(x) \geq 0\). We find \(r(x) > 0\), or \((+)\), on the intervals \(\left(-\infty, -\frac{1}{2}\right)\), \((0,1)\) and \((1, \infty)\). We add to these intervals the zeros of \(r\), \(-\frac{1}{2}\) and \(0\), to get our final solution: \(\left( - \infty, -\frac{1}{2} \right] \cup [0,1) \cup (1, \infty)\).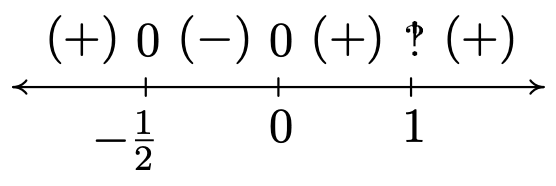
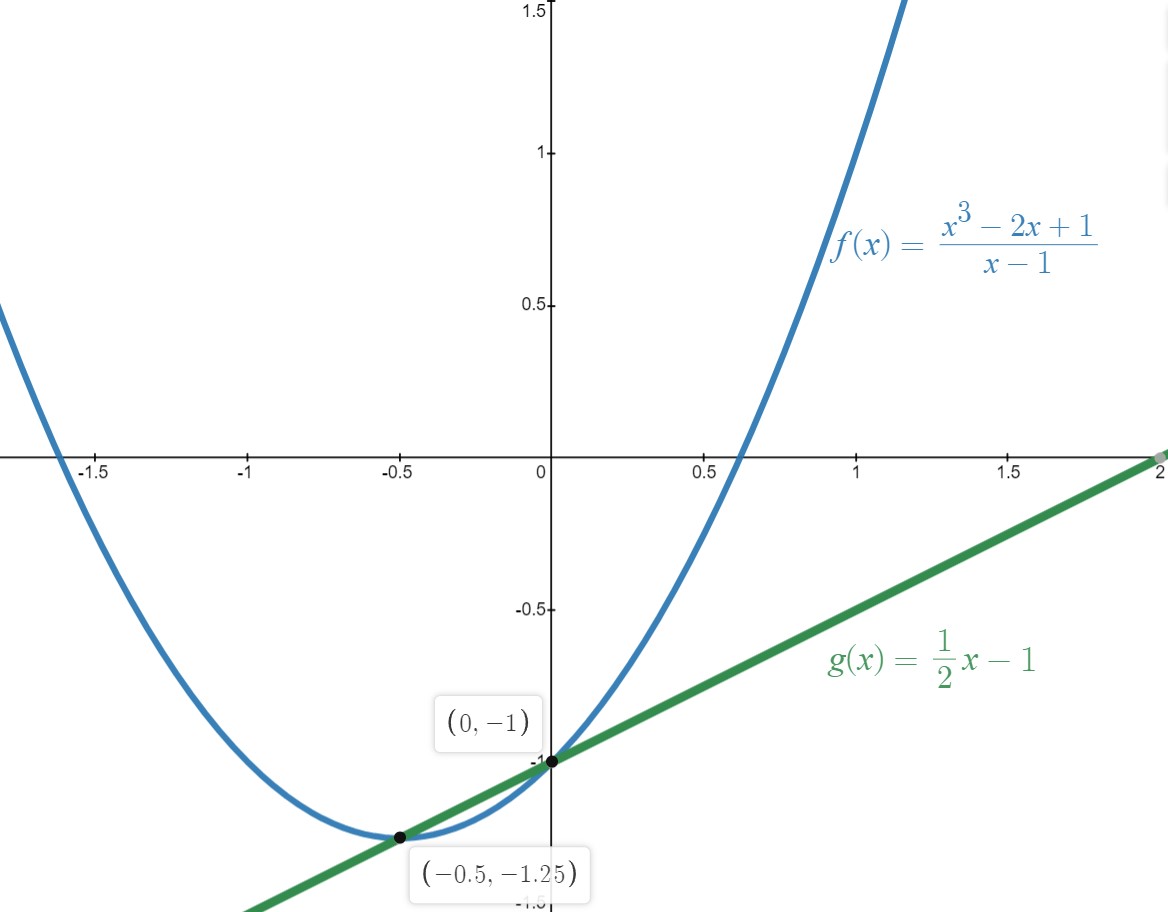
3. Geometrically, if we set \(f(x) = \frac{x^3-2x+1}{x-1}\) and \(g(x) = \frac{1}{2} x -1\), the solutions to \(f(x)=g(x)\) are the \(x\)-coordinates of the points where the graphs of \(y=f(x)\) and \(y=g(x)\) intersect. The solution to \(f(x) \geq g(x)\) represents not only where the graphs meet, but the intervals over which the graph of \(y=f(x)\) is above (\(>\)) the graph of \(g(x)\). We obtain the graph below.

   

   It is clear from Desmos that the graph of \(y=f(x)\) is above the graph of \(y=g(x)\) on \(\left(-\infty, -\frac{1}{2}\right)\) as well as on \((0,\infty)\). According to Desmos, our solution is then \(\left(-\infty, -\frac{1}{2}\right] \cup [0, \infty)\) which almost matches the answer we found analytically. We have to remember that \(f\) is not defined at \(x=1\), and, even though it isn’t shown on Desmos, there is a hole in the graph of \(y=f(x)\) when \(x=1\) which is why \(x=1\) is not part of our final answer.¹

Subsection Footnotes

¹ There is no asymptote at \(x = 1\) since the graph is well behaved near \(x = 1\). According to Theorem 4.1.1, there must be a hole there.

---

Applications Involving Rational Equations

Next, we explore how rational equations can be used to solve some classic problems involving rates.

Example \( \PageIndex{2} \)

Carl decides to explore the Meander River, the location of several recent Sasquatch sightings. From camp, he canoes downstream five miles to check out a purported Sasquatch nest. Finding nothing, he immediately turns around, retraces his route (this time traveling upstream), and returns to camp 3 hours after he left. If Carl canoes at a rate of 6 miles per hour in still water, how fast was the Meander River flowing on that day?

Solution

We are given information about distances, rates (speeds) and times. The basic principle relating these quantities is: \[\text{distance} = \text{rate} \cdot \text{time}\nonumber\] The first observation to make, however, is that the distance, rate and time given to us aren’t "compatible": the distance given is the distance for only part of the trip, the rate given is the speed Carl can canoe in still water, not in a flowing river, and the time given is the duration of the entire trip. Ultimately, we are after the speed of the river, so let’s call that \(R\) measured in miles per hour to be consistent with the other rate given to us. To get started, let’s divide the trip into its two parts: the initial trip downstream and the return trip upstream. For the downstream trip, all we know is that the distance traveled is \(5\) miles.

\[\begin{array}{rcl} \text{distance downstream} & = & \text{rate traveling downstream} \cdot \text{time traveling downstream} \\ 5 \, \text{miles} & = & \text{rate traveling downstream} \cdot \text{time traveling downstream} \\ \end{array}\nonumber\]

Since the return trip upstream followed the same route as the trip downstream, we know that the distance traveled upstream is also 5 miles.

\[\begin{array}{rcl} \text{distance upstream} & = & \text{rate traveling upstream} \cdot \text{time traveling upstream} \\ 5 \, \text{miles} & = & \text{rate traveling upstream} \cdot \text{time traveling upstream} \\ \end{array}\nonumber\]

We are told Carl can canoe at a rate of \(6\) miles per hour in still water. How does this figure into the rates traveling upstream and downstream? The speed the canoe travels in the river is a combination of the speed at which Carl can propel the canoe in still water, 6 miles per hour, and the speed of the river, which we’re calling \(R\). When traveling downstream, the river is helping Carl along, so we add these two speeds:

\[\begin{array}{rcl} \text{rate traveling downstream} & = & \text{rate Carl propels the canoe} + \text{speed of the river} \\ & = & 6 \dfrac{\text{miles}}{\text{hour}} + R \dfrac{\text{miles}}{\text{hour}} \\ \end{array}\nonumber\]

So our downstream speed is \((6+R) \frac{\text{miles}}{\text{hour}}\). Substituting this into our "distance-rate-time" equation for the downstream part of the trip, we get:

\[\begin{array}{rcl} 5 \, \text{miles} & = & \text{rate traveling downstream} \cdot \text{time traveling downstream} \\ 5 \, \text{miles} & = & (6+R) \dfrac{\text{miles}}{\text{hour}} \cdot \text{time traveling downstream} \\ \end{array}\nonumber\]

When traveling upstream, Carl works against the current. Since the canoe manages to travel upstream, the speed Carl can canoe in still water is greater than the river’s speed, so we subtract the river’s speed from Carl’s canoeing speed to get:

\[\begin{array}{rcl} \text{rate traveling upstream} & = & \text{rate Carl propels the canoe} - \text{river speed} \\ & = & 6 \dfrac{\text{miles}}{\text{hour}} - R \dfrac{\text{miles}}{\text{hour}} \\ \end{array}\nonumber\]

Proceeding as before, we get

\[\begin{array}{rcl} 5 \, \text{miles} & = & \text{rate traveling upstream} \cdot \text{time traveling upstream} \\ 5 \, \text{miles} & = & (6 - R) \dfrac{\text{miles}}{\text{hour}} \cdot \text{time traveling upstream} \\ \end{array}\nonumber\]

The last piece of information given to us is that the total trip lasted \(3\) hours. If we let \(t_{\text{down}}\) denote the time of the downstream trip and \(t_{\text{up}}\) the time of the upstream trip, we have: \(t_{\text{down}} + t_{\text{up}} = 3 \, \text{hours}\). Substituting \(t_{\text{down}}\) and \(t_{\text{up}}\) into the "distance-rate-time" equations, we get (suppressing the units) three equations in three unknowns:² \[\left\{\begin{array}{lrcl} E1 & (6+R) \, t_{\text{down}} & = & 5 \\ E2 & (6-R) \, t_{\text{up}} & = & 5 \\ E3 & t_{\text{down}} + t_{\text{up}} & = & 3 \end{array} \right.\nonumber\]

Since we are ultimately after \(R\), we need to use these three equations to get at least one equation involving only \(R\). To that end, we solve \(E1\) for \(t_{\text{down}}\) by dividing both sides³ by the quantity \((6+R)\) to get \(t_{\text{down}} = \frac{5}{6+R}\). Similarly, we solve \(E2\) for \(t_{\text{up}}\) and get \(t_{\text{up}} = \frac{5}{6-R}\). Substituting these into \(E3\), we get:⁴ \[\dfrac{5}{6+R} + \dfrac{5}{6 - R} = 3.\nonumber\] Clearing denominators, we get \(5(6-R) + 5(6+R) = 3(6+R)(6-R)\) which reduces to \(R^2 = 16\). We find \(R = \pm 4\), and since \(R\) represents the speed of the river, we choose \(R = 4\). On the day in question, the Meander River is flowing at a rate of \(4\) miles per hour.

One of the important lessons to learn from Example \( \PageIndex{2} \) is that speeds, and more generally, rates, are additive. As we see in our next example, the concept of rate and its associated principles can be applied to a wide variety of problems - not just "distance-rate-time" scenarios.

Example \( \PageIndex{3} \)

Working alone, Taylor can weed the garden in 4 hours. If Carl helps, they can weed the garden in 3 hours. How long would it take for Carl to weed the garden on his own?

Solution

The key relationship between work and time which we use in this problem is: \[\text{amount of work done} = \text{rate of work} \cdot \text{time spent working}\nonumber\]

We are told that, working alone, Taylor can weed the garden in 4 hours. In Taylor’s case then: \[\begin{array}{rcl} \text{amount of work Taylor does} & = & \text{rate of Taylor working} \cdot \text{time Taylor spent working} \\ 1 \, \text{garden} & = & (\text{rate of Taylor working}) \cdot (4 \, \text{hours}) \\ \end{array}\nonumber\]

So we have that the rate Taylor works is \(\frac{1 \, \text{garden}}{ 4 \, \text{hours}} = \frac{1}{4} \frac{\text{garden}}{\text{hour}}\). We are also told that when working together, Taylor and Carl can weed the garden in just 3 hours. We have:

\[\begin{array}{rcl} \text{amount of work done together} & = & \text{rate of working together} \cdot \text{time spent working together} \\ 1 \, \text{garden} & = & (\text{rate of working together}) \cdot (3 \, \text{hours}) \\ \end{array}\nonumber\]

From this, we find that the rate of Taylor and Carl working together is \(\frac{1 \, \text{garden}}{3 \, \text{hours}} = \frac{1}{3} \frac{\text{garden}}{\text{hour}}\). We are asked to find out how long it would take for Carl to weed the garden on his own. Let us call this unknown \(t\), measured in hours to be consistent with the other times given to us in the problem. Then:

\[\begin{array}{rcl} \text{amount of work Carl does} & = & \text{rate of Carl working} \cdot \text{time Carl spent working} \\ 1 \, \text{garden} & = & (\text{rate of Carl working}) \cdot (t \, \text{hours}) \\ \end{array}\nonumber\]

In order to find \(t\), we need to find the rate of Carl working, so let’s call this quantity \(R\), with units \(\frac{\text{garden}}{\text{hour}}\). Using the fact that rates are additive, we have:

\[\begin{array}{rcl} \text{rate working together} & = & \text{rate of Taylor working} + \text{rate of Carl working} \\[4pt] \dfrac{1}{3} \dfrac{\text{garden}}{\text{hour}} & = & \dfrac{1}{4} \dfrac{\text{garden}}{\text{hour}} + R \dfrac{\text{garden}}{\text{hour}} \\ \end{array}\nonumber\]

so that \(R = \frac{1}{12} \frac{\text{garden}}{\text{hour}}\). Substituting this into our ‘work-rate-time’ equation for Carl, we get:

\[\begin{array}{rcl} 1 \, \text{garden} & = & (\text{rate of Carl working}) \cdot (t \, \text{hours}) \\[4pt] 1 \, \text{garden} & = & \left(\dfrac{1}{12} \dfrac{\text{garden}}{\text{hour}} \right) \cdot (t \, \text{hours}) \\ \end{array}\nonumber\]

Solving \(1 = \frac{1}{12} t\), we get \(t = 12\), so it takes Carl 12 hours to weed the garden on his own.

As is common with "word problems" like Examples \( \PageIndex{2} \) and \( \PageIndex{3} \), there is no short-cut to the answer. We encourage the reader to carefully think through and apply the basic principles of rate to each (potentially different!) situation. It is time well spent. We also encourage the tracking of units, especially in the early stages of the problem. Not only does this promote uniformity in the units, it also serves as a quick means to check if an equation makes sense.

Subsection Footnotes

² This is called a system of equations. No doubt, you’ve had experience with these things before, and we will study systems in greater detail in Chapter 9.

³ While we usually discourage dividing both sides of an equation by a variable expression, we know \((6+R) \neq 0\) since otherwise we couldn’t possibly multiply it by \(t_{\text {down }}\) and get 5.

⁴ The reader is encouraged to verify that the units in this equation are the same on both sides. To get you started, the units on the "3" is "hours."

---

Applications Involving Rational Inequalities

Our next example deals with the average cost function, first introduced as applied to PortaBoy Game systems from Example 2.1.5 in Section 2.1.

Example \( \PageIndex{4} \)

Given a cost function \(C(x)\), which returns the total cost of producing \(x\) items, recall that the average cost function, \(\overline{C}(x) = \frac{C(x)}{x}\) computes the cost per item when \(x\) items are produced. Suppose the cost \(C\), in dollars, to produce \(x\) PortaBoy game systems for a local retailer is \(C(x) = 80x + 150\), \(x \geq 0\).

1. Find an expression for the average cost function \(\overline{C}(x)\).
2. Solve \(\overline{C}(x) < 100\) and interpret.
3. Determine the behavior of \(\overline{C}(x)\) as \(x \to \infty\) and interpret.

Solution

1. From \(\overline{C}(x) = \frac{C(x)}{x}\), we obtain \(\overline{C}(x) = \frac{80x+150}{x}\). The domain of \(C\) is \(x \geq 0\), but since \(x=0\) causes problems for \(\overline{C}(x)\), we get our domain to be \(x>0\), or \((0, \infty)\).
2. Solving \(\overline{C}(x) < 100\) means we solve \(\frac{80x+150}{x} < 100\). We proceed as in the previous example.\[\begin{array}{rclr}
   \dfrac{80x+150}{x} & < & 100 & \\
   \dfrac{80x+150}{x} - 100 & < & 0 & \\
   \dfrac{80x + 150 - 100x}{x} & < & 0 & \left( \text{common denominator} \right) \\
   \dfrac{150 - 20x}{x} & < & 0 & \\
   \end{array}\nonumber\]If we take the left hand side to be a rational function \(r(x)\), we need to keep in mind that the applied domain of the problem is \(x > 0\). This means we consider only the positive half of the number line for our sign diagram. On \((0, \infty)\), \(r\) is defined everywhere so we need only look for zeros of \(r\). Setting \(r(x)=0\) gives \(150-20x =0\), so that \(x = \frac{15}{2}= 7.5\). The test intervals on our domain are \((0, 7.5)\) and \((7.5, \infty)\). We find \(r(x) < 0\) on \((7.5, \infty)\).

   

   In the context of the problem, \(x\) represents the number of PortaBoy games systems produced and \(\overline{C}(x)\) is the average cost to produce each system. Solving \(\overline{C}(x) < 100\) means we are trying to find how many systems we need to produce so that the average cost is less than \(\$100\) per system. Our solution, \((7.5, \infty)\) tells us that we need to produce more than \(7.5\) systems to achieve this. Since it doesn’t make sense to produce half a system, our final answer is \([8, \infty)\).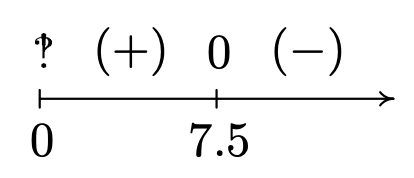
3. When we apply Theorem 4.1.2 to \(\overline{C}(x)\) we find that \(y=80\) is a horizontal asymptote to the graph of \(y=\overline{C}(x)\). To more precisely determine the behavior of \(\overline{C}(x)\) as \(x \to \infty\), we first use long division⁵ and rewrite \(\overline{C}(x) = 80+\frac{150}{x}\). As \(x \to \infty\), \(\frac{150}{x} \to 0^{+}\), which means \(\overline{C}(x) \approx 80+\text { very small }(+)\). Thus the average cost per system is getting closer to \(\$ 80\) per system. If we set \(\overline{C}(x) = 80\), we get \(\frac{150}{x} = 0\), which is impossible, so we conclude that \(\overline{C}(x) > 80\) for all \(x > 0\). This means that the average cost per system is always greater than \(\$ 80\) per system, but the average cost is approaching this amount as more and more systems are produced. Looking back at Example 2.1.5, we realize \(\$ 80\) is the variable cost per system \(-\) the cost per system above and beyond the fixed initial cost of \(\$150\). Another way to interpret our answer is that "infinitely" many systems would need to be produced to effectively "zero out" the fixed cost.

Our next example is another classic "box with no top" problem.

Example \( \PageIndex{5} \)

A box with a square base and no top is to be constructed so that it has a volume of \(1000\) cubic centimeters. Let \(x\) denote the width of the box, in centimeters as seen below.

1. Express the height \(h\) in centimeters as a function of the width \(x\) and state the applied domain.
2. Solve \(h(x) \geq x\) and interpret.
3. Find and interpret the behavior of \(h(x)\) as \(x \to 0^{+}\) and as \(x \to \infty\).
4. Express the surface area \(S\) of the box as a function of \(x\) and state the applied domain.
5. Use graphing technology to approximate (to two decimal places) the dimensions of the box which minimize the surface area.

Solution

1. We are told that the volume of the box is \(1000\) cubic centimeters and that \(x\) represents the width, in centimeters. From geometry, we know \(\mbox{Volume} = \mbox{width} \times \mbox{height} \times \mbox{depth}\). Since the base of the box is a square, the width and the depth are both \(x\) centimeters. Using \(h\) for the height, we have \(1000 = x^2h\), so that \(h = \frac{1000}{x^2}\). Using function notation, \(h(x) = \frac{1000}{x^2}\) As for the applied domain, in order for there to be a box at all, \(x > 0\), and since every such choice of \(x\) will return a positive number for the height \(h\) we have no other restrictions and conclude our domain is \((0, \infty)\).
2. To solve \(h(x) \geq x\), we proceed as before and collect all nonzero terms on one side of the inequality in order to use a sign diagram.\[\begin{array}{rclr}
   h(x) & \geq & x & \\
   \dfrac{1000}{x^2} & \geq & x & \\
   \dfrac{1000}{x^2} - x & \geq & 0 \\
   \dfrac{1000-x^3}{x^2} & \geq & 0 & \left( \text{common denominator} \right) \\
   \end{array}\nonumber\]We consider the left hand side of the inequality as our rational function \(r(x)\). We see \(r\) is undefined at \(x=0\), but, as in the previous example, the applied domain of the problem is \(x > 0\), so we are considering only the behavior of \(r\) on \((0, \infty)\). The sole zero of \(r\) comes when \(1000-x^3 = 0\), which is \(x=10\). Choosing test values in the intervals \((0,10)\) and \((10, \infty)\) gives the following diagram.

   

   We see \(r(x) > 0\) on \((0,10)\), and since \(r(x) = 0\) at \(x=10\), our solution is \((0,10]\). In the context of the problem, \(h\) represents the height of the box while \(x\) represents the width (and depth) of the box. Solving \(h(x) \geq x\) is tantamount to finding the values of \(x\) which result in a box where the height is at least as big as the width (and, in this case, depth.) Our answer tells us the width of the box can be at most \(10\) centimeters for this to happen.
3. As \(x \to 0^{+}\), \(h(x) = \frac{1000}{x^2} \to \infty\). This means that the smaller the width \(x\) (and, in this case, depth), the larger the height \(h\) has to be in order to maintain a volume of \(1000\) cubic centimeters. As \(x \to \infty\), we find \(h(x) \to 0^{+}\), which means that in order to maintain a volume of \(1000\) cubic centimeters, the width and depth must get bigger as the height becomes smaller.
4. Since the box has no top, the surface area can be found by adding the area of each of the sides to the area of the base. The base is a square of dimensions \(x\) by \(x\), and each side has dimensions \(x\) by \(h\). We get the surface area, \(S = x^2+4xh\). To get \(S\) as a function of \(x\), we substitute \(h = \frac{1000}{x^2}\) to obtain \(S = x^2+4x \left( \frac{1000}{x^2}\right)\). Hence, as a function of \(x\), \(S(x) = x^2 + \frac{4000}{x}\). The domain of \(S\) is the same as \(h\), namely \((0, \infty)\), for the same reasons as above.
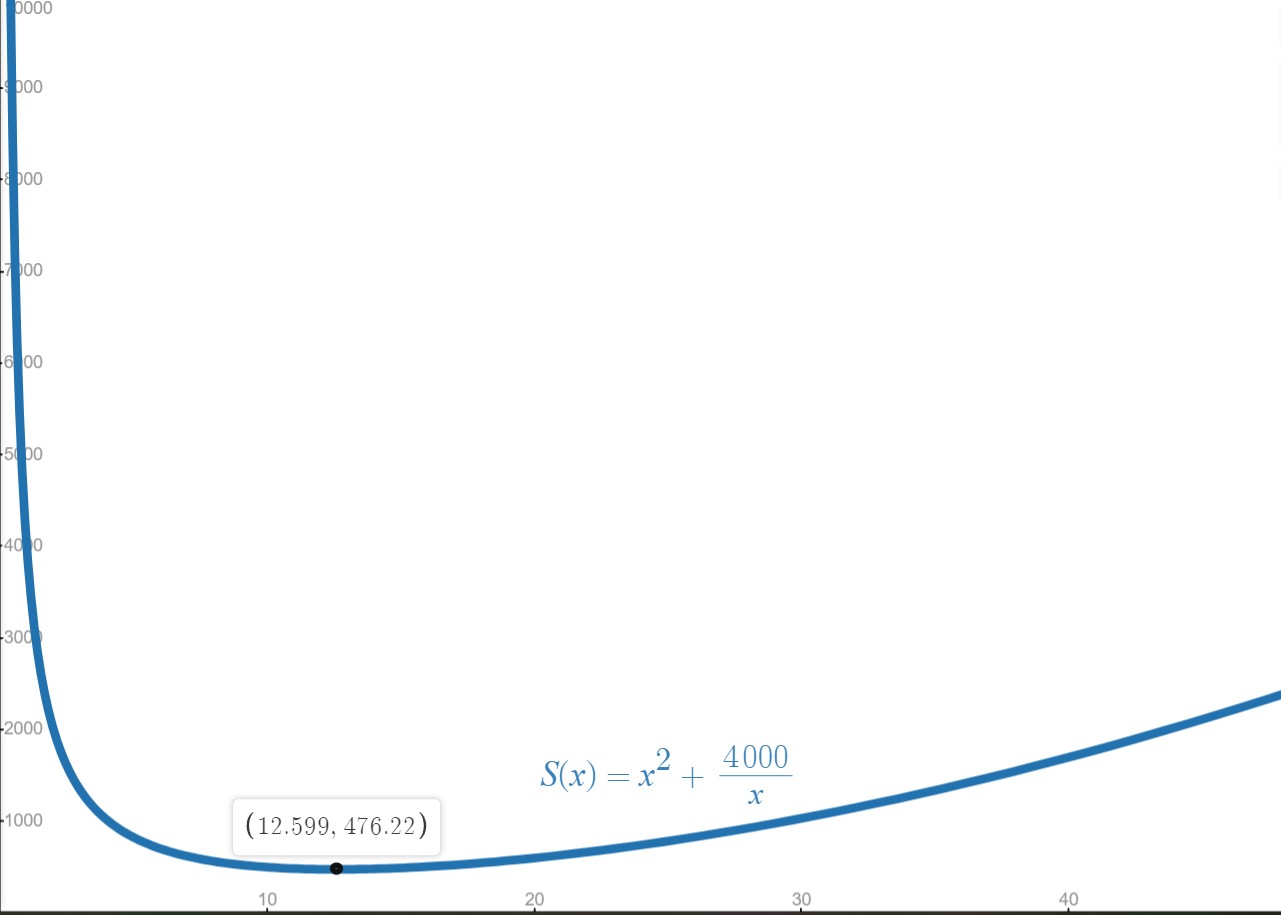
5. A first attempt at the graph of \(y=S(x)\) on the calculator may lead to frustration. Chances are good that the first window chosen to view the graph will suggest \(y=S(x)\) has the \(x\)-axis as a horizontal asymptote. From the formula \(S(x) = x^2 + \frac{4000}{x}\), however, we get \(S(x) \approx x^2\) as \(x \to \infty\), so \(S(x) \to \infty\). Readjusting the window, we find \(S\) does possess a relative minimum at \(x \approx 12.60\). Without calculus, all we can say is that we belive this is the only relative extremum, so it is the absolute minimum as well. This means that the width and depth of the box should each measure approximately \(12.60\) centimeters. To determine the height, we find \(h(12.60) \approx 6.30\), so the height of the box should be approximately \(6.30\) centimeters.

Subsection Footnotes

⁵ In this case, long division amounts to term-by-term division.