1.R: Trigonometric Functions (Review)

Last updated
Save as PDF

Page ID: 60912

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$ \newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\id}{\mathrm{id}}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\kernel}{\mathrm{null}\,}$

$ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$

$ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$

$ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\AA}{\unicode[.8,0]{x212B}}$

$ \newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$ \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$ \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vectorC}[1]{\textbf{#1}} $

$ \newcommand{\vectorD}[1]{\overrightarrow{#1}} $

$ \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} $

$ \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} $

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$\newcommand{\avec}{\mathbf a}$ $\newcommand{\bvec}{\mathbf b}$ $\newcommand{\cvec}{\mathbf c}$ $\newcommand{\dvec}{\mathbf d}$ $\newcommand{\dtil}{\widetilde{\mathbf d}}$ $\newcommand{\evec}{\mathbf e}$ $\newcommand{\fvec}{\mathbf f}$ $\newcommand{\nvec}{\mathbf n}$ $\newcommand{\pvec}{\mathbf p}$ $\newcommand{\qvec}{\mathbf q}$ $\newcommand{\svec}{\mathbf s}$ $\newcommand{\tvec}{\mathbf t}$ $\newcommand{\uvec}{\mathbf u}$ $\newcommand{\vvec}{\mathbf v}$ $\newcommand{\wvec}{\mathbf w}$ $\newcommand{\xvec}{\mathbf x}$ $\newcommand{\yvec}{\mathbf y}$ $\newcommand{\zvec}{\mathbf z}$ $\newcommand{\rvec}{\mathbf r}$ $\newcommand{\mvec}{\mathbf m}$ $\newcommand{\zerovec}{\mathbf 0}$ $\newcommand{\onevec}{\mathbf 1}$ $\newcommand{\real}{\mathbb R}$ $\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$ $\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$ $\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$ $\newcommand{\laspan}[1]{\text{Span}\{#1\}}$ $\newcommand{\bcal}{\cal B}$ $\newcommand{\ccal}{\cal C}$ $\newcommand{\scal}{\cal S}$ $\newcommand{\wcal}{\cal W}$ $\newcommand{\ecal}{\cal E}$ $\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$ $\newcommand{\gray}[1]{\color{gray}{#1}}$ $\newcommand{\lgray}[1]{\color{lightgray}{#1}}$ $\newcommand{\rank}{\operatorname{rank}}$ $\newcommand{\row}{\text{Row}}$ $\newcommand{\col}{\text{Col}}$ $\renewcommand{\row}{\text{Row}}$ $\newcommand{\nul}{\text{Nul}}$ $\newcommand{\var}{\text{Var}}$ $\newcommand{\corr}{\text{corr}}$ $\newcommand{\len}[1]{\left|#1\right|}$ $\newcommand{\bbar}{\overline{\bvec}}$ $\newcommand{\bhat}{\widehat{\bvec}}$ $\newcommand{\bperp}{\bvec^\perp}$ $\newcommand{\xhat}{\widehat{\xvec}}$ $\newcommand{\vhat}{\widehat{\vvec}}$ $\newcommand{\uhat}{\widehat{\uvec}}$ $\newcommand{\what}{\widehat{\wvec}}$ $\newcommand{\Sighat}{\widehat{\Sigma}}$ $\newcommand{\lt}{<}$ $\newcommand{\gt}{>}$ $\newcommand{\amp}{&}$ $\definecolor{fillinmathshade}{gray}{0.9}$

1.1: Review Exercises

For the exercises 1-2, convert the angle measures to degrees.

1) $\dfrac{π}{4}$

Answer: $45°$

2) $−\dfrac{5π}{3}$

For the exercises 3-6, convert the angle measures to radians.

3) $-210°$

Answer: $−\dfrac{7π}{6}$

4) $180°$

5) Find the length of an arc in a circle of radius $7$ meters subtended by the central angle of $85°$.

Answer: $10.385$ meters

6) Find the area of the sector of a circle with diameter $32$ feet and an angle of $\dfrac{3π}{5}$ radians.

For the exercises 7-8, find the angle between $0°$ and $360°$ that is coterminal with the given angle.

7) $420°$

Answer: $60°$

8) $−80°$

For the exercises 9-10, find the angle between $0$ and $2π$ in radians that is coterminal with the given angle.

9) $− \dfrac{20π}{11}$

Answer: $\dfrac{2π}{11}$

10) $\dfrac{14π}{5}$

For the exercises 11-, draw the angle provided in standard position on the Cartesian plane.

11) $-210°$

Answer: $alt$

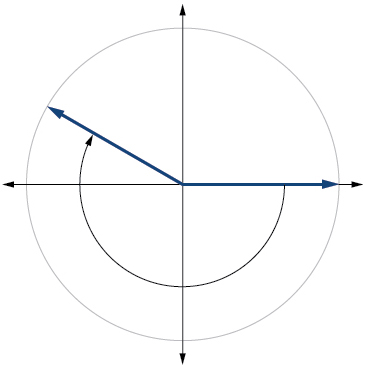
12) $75°$

13) $\dfrac{5π}{4}$

Answer: $CNX_Precalc_Figure_05_04_219.jpg$

14) $−\dfrac{π}{3}$

15) Find the linear speed of a point on the equator of the earth if the earth has a radius of $3,960$ miles and the earth rotates on its axis every $24$ hours. Express answer in miles per hour.

Answer: $1036.73$ miles per hour

16) A car wheel with a diameter of $18$ inches spins at the rate of $10$ revolutions per second. What is the car's speed in miles per hour?

1.2: Review Exercises

1) Find the exact value of $ \sin \dfrac{π}{3}$.

Answer: $\dfrac{\sqrt{3}}{2}$

2) Find the exact value of $ \cos \dfrac{π}{4}$.

3) Find the exact value of $ \cos π $.

Answer: $-1$

4) State the reference angle for $300°$.

5) State the reference angle for $ \dfrac{3π}{4}$.

Answer: $ \dfrac{π}{4}$

6) Compute cosine of $330°$.

7) Compute sine of $\dfrac{5π}{4}$.

Answer: $−\dfrac{\sqrt{2}}{2}$

8) State the domain of the sine and cosine functions.

9) State the range of the sine and cosine functions.

Answer: $[–1,1]$

1.3: Review Exercises

For the exercises 1-4, find the exact value of the given expression.

1) $ \cos \dfrac{π}{6} $

2) $ \tan \dfrac{π}{4} $

Answer: $1$

3) $ \csc \dfrac{π}{3}$

4) $ \sec \dfrac{π}{4} $

Answer: $\sqrt{2}$

For the exercises 4-12, use reference angles to evaluate the given expression.

5) $ \sec \dfrac{11π}{3}$

6) $ \sec 315°$

Answer: $ \sqrt{2}$

7) If $ \sec (t)=−2.5$, what is the $ \sec (−t)$?

8) If $ \tan (t)=−0.6 $, what is the $ \tan (−t)$?

Answer: $0.6$

9) If $ \tan (t)=\dfrac{1}{3}$, find $ \tan (t−π)$.

10) If $ \cos (t)= \dfrac{\sqrt{2}}{2}$, find $ \sin (t+2π)$.

Answer: $\dfrac{\sqrt{2}}{2}$ or $−\dfrac{\sqrt{2}}{2}$

11) Which trigonometric functions are even?

12) Which trigonometric functions are odd?

Answer: sine, cosecant, tangent, cotangent

1.4: Review Exercises

For the exercises 1-5, use side lengths to evaluate.

1) $ \cos \dfrac{π}{4}$

2) $ \cot \dfrac{π}{3}$

Answer: $\dfrac{\sqrt{3}}{3}$

3) $ \tan \dfrac{π}{6}$

4) $ \cos (\dfrac{π}{2}) = \sin ( \_\_°)$

Answer: $0$

5) $ \csc (18°)= \sec (\_\_°)$

For the exercises 6-7, use the given information to find the lengths of the other two sides of the right triangle.

6) $ \cos B= \dfrac{3}{5}, a=6$

Answer: $ b=8,c=10$

7) $ \tan A = \dfrac{5}{9},b=6 $

For the exercises 8-9, use Figure below to evaluate each trigonometric function.

$alt$

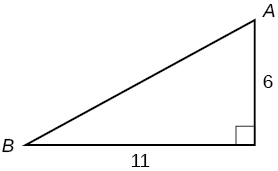
8) $ \sin A $

Answer: $ \dfrac{11\sqrt{157}}{157}$

9) $ \tan B $

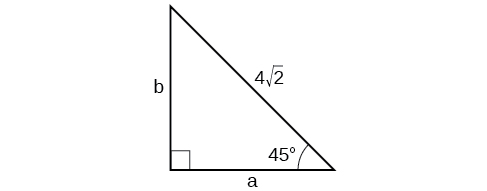
For the exercises 10-11, solve for the unknown sides of the given triangle.

10)

$alt$

Answer: $a=4, b=4 $

11)

$alt$

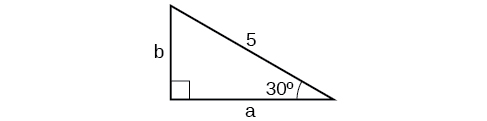
12) A $15$-ft ladder leans against a building so that the angle between the ground and the ladder is $70°$. How high does the ladder reach up the side of the building?

Answer: $14.0954$ ft

13) The angle of elevation to the top of a building in Baltimore is found to be $4$ degrees from the ground at a distance of $1$ mile from the base of the building. Using this information, find the height of the building.

Practice Test

1) Convert $ \dfrac{5π}{6}$ radians to degrees.

Answer: $150°$

2) Convert $−620°$ to radians.

3) Find the length of a circular arc with a radius $12$ centimeters subtended by the central angle of $30°$.

Answer: $6.283$ centimeters

4) Find the area of the sector with radius of $8$ feet and an angle of $\dfrac{5π}{4}$ radians.

5) Find the angle between $0°$ and $360°$ that is coterminal with $375°$.

Answer: $15°$

6) Find the angle between $0$ and $2π$ in radians that is coterminal with $−\dfrac{4π}{7}$.

7) Draw the angle $315°$ in standard position on the Cartesian plane.

Answer: $alt$

8) Draw the angle $−\dfrac{π}{6}$ in standard position on the Cartesian plane.

9) A carnival has a Ferris wheel with a diameter of $80$ feet. The time for the Ferris wheel to make one revolution is $75$ seconds. What is the linear speed in feet per second of a point on the Ferris wheel? What is the angular speed in radians per second?

Answer: $3.351$ feet per second, $ \dfrac{2π}{75}$ radians per second

10) Find the exact value of $ \sin \dfrac{π}{6}$.

11) Compute sine of $240°$.

Answer: $−\dfrac{\sqrt{3}}{2}$

12) State the domain of the sine and cosine functions.

13) State the range of the sine and cosine functions.

Answer: $[ –1,1 ]$

14) Find the exact value of $ \cot \dfrac{π}{4}$.

15) Find the exact value of $ \tan \dfrac{π}{3}$.

Answer: $ \sqrt{3}$

16) Use reference angles to evaluate $ \csc \dfrac{7π}{4}$.

17) Use reference angles to evaluate $ \tan 210°$.

Answer: $\dfrac{\sqrt{3}}{3}$

18) If $ \csc t=0.68$, what is the $ \csc (−t)$?

19) If $ \cos t= \dfrac{\sqrt{3}}{2}$, find $ \cos (t−2π)$.

Answer: $\dfrac{\sqrt{3}}{2}$

20) Which trigonometric functions are even?

21) Find the missing angle: $\cos \left(\dfrac{\pi }{6} \right)= \sin (\;)$

Answer: $\dfrac{π}{3}$

22) Find the missing sides of the triangle $ ABC: \sin B= \dfrac{3}{4},c=12$

23) Find the missing sides of the triangle.

$alt$

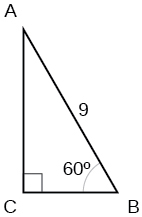
Answer: $a=\dfrac{9}{2},b=\dfrac{9\sqrt{3}}{2}$

24) The angle of elevation to the top of a building in Chicago is found to be $9$ degrees from the ground at a distance of $2000$ feet from the base of the building. Using this information, find the height of the building.

Contributors and Attributions

Jay Abramson (Arizona State University) with contributing authors. Textbook content produced by OpenStax College is licensed under a Creative Commons Attribution License 4.0 license. Download for free at https://openstax.org/details/books/precalculus.

Search

Text Color

Text Size

Margin Size

Font Type

1.2: Review Exercises

1.3: Review Exercises

1.4: Review Exercises

Practice Test