2.R: Periodic Functions (Review)

Last updated
Save as PDF

Page ID: 60919

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$ \newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\id}{\mathrm{id}}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\kernel}{\mathrm{null}\,}$

$ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$

$ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$

$ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\AA}{\unicode[.8,0]{x212B}}$

$ \newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$ \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$ \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vectorC}[1]{\textbf{#1}} $

$ \newcommand{\vectorD}[1]{\overrightarrow{#1}} $

$ \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} $

$ \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} $

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$\newcommand{\avec}{\mathbf a}$ $\newcommand{\bvec}{\mathbf b}$ $\newcommand{\cvec}{\mathbf c}$ $\newcommand{\dvec}{\mathbf d}$ $\newcommand{\dtil}{\widetilde{\mathbf d}}$ $\newcommand{\evec}{\mathbf e}$ $\newcommand{\fvec}{\mathbf f}$ $\newcommand{\nvec}{\mathbf n}$ $\newcommand{\pvec}{\mathbf p}$ $\newcommand{\qvec}{\mathbf q}$ $\newcommand{\svec}{\mathbf s}$ $\newcommand{\tvec}{\mathbf t}$ $\newcommand{\uvec}{\mathbf u}$ $\newcommand{\vvec}{\mathbf v}$ $\newcommand{\wvec}{\mathbf w}$ $\newcommand{\xvec}{\mathbf x}$ $\newcommand{\yvec}{\mathbf y}$ $\newcommand{\zvec}{\mathbf z}$ $\newcommand{\rvec}{\mathbf r}$ $\newcommand{\mvec}{\mathbf m}$ $\newcommand{\zerovec}{\mathbf 0}$ $\newcommand{\onevec}{\mathbf 1}$ $\newcommand{\real}{\mathbb R}$ $\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$ $\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$ $\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$ $\newcommand{\laspan}[1]{\text{Span}\{#1\}}$ $\newcommand{\bcal}{\cal B}$ $\newcommand{\ccal}{\cal C}$ $\newcommand{\scal}{\cal S}$ $\newcommand{\wcal}{\cal W}$ $\newcommand{\ecal}{\cal E}$ $\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$ $\newcommand{\gray}[1]{\color{gray}{#1}}$ $\newcommand{\lgray}[1]{\color{lightgray}{#1}}$ $\newcommand{\rank}{\operatorname{rank}}$ $\newcommand{\row}{\text{Row}}$ $\newcommand{\col}{\text{Col}}$ $\renewcommand{\row}{\text{Row}}$ $\newcommand{\nul}{\text{Nul}}$ $\newcommand{\var}{\text{Var}}$ $\newcommand{\corr}{\text{corr}}$ $\newcommand{\len}[1]{\left|#1\right|}$ $\newcommand{\bbar}{\overline{\bvec}}$ $\newcommand{\bhat}{\widehat{\bvec}}$ $\newcommand{\bperp}{\bvec^\perp}$ $\newcommand{\xhat}{\widehat{\xvec}}$ $\newcommand{\vhat}{\widehat{\vvec}}$ $\newcommand{\uhat}{\widehat{\uvec}}$ $\newcommand{\what}{\widehat{\wvec}}$ $\newcommand{\Sighat}{\widehat{\Sigma}}$ $\newcommand{\lt}{<}$ $\newcommand{\gt}{>}$ $\newcommand{\amp}{&}$ $\definecolor{fillinmathshade}{gray}{0.9}$

2.1: Graphs of the Sine and Cosine Functions

For the exercises 1-8, graph the functions for two periods and determine the amplitude or stretching factor, period, midline equation, and asymptotes.

1) $f(x)=-3\cos x+3$

Answer

amplitude: $3$; period: $2\pi $; midline: $y=3$no asymptotes

$6R6.1.1.png$

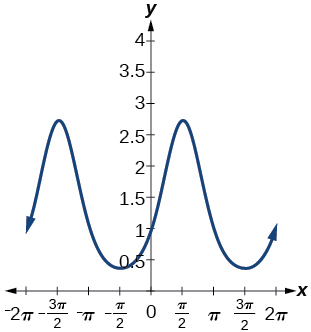
2) $f(x)=\dfrac{1}{4}\sin x$

3) $f(x)=3\cos\left ( x+\dfrac{\pi }{6} \right )$

Answer

amplitude: $3$; period: $2\pi $; midline: $y=0$; no asymptotes

$6R6.1.3.png$

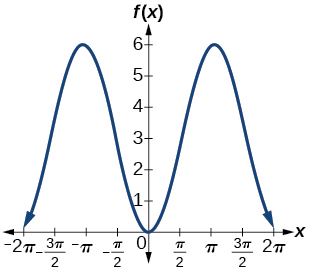
4) $f(x)=-2\sin\left ( x-\dfrac{2\pi }{3} \right )$

5) $f(x)=3\sin\left ( x-\dfrac{\pi }{4} \right )-4$

Answer

amplitude: $3$; period: $2\pi $; midline: $y=-4$; no asymptotes

$6R6.1.5.png$

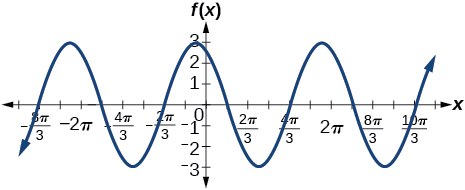
6) $f(x)=2\left (\cos\left ( x-\dfrac{4\pi }{3} \right )+1 \right )$

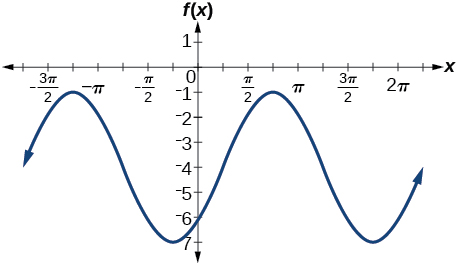
7) $f(x)=6\sin\left ( 3x-\dfrac{\pi }{6} \right )-1$

Answer

amplitude: $6$; period: $dfrac{2\pi }{3}$; midline: $y=-1$; no asymptotes

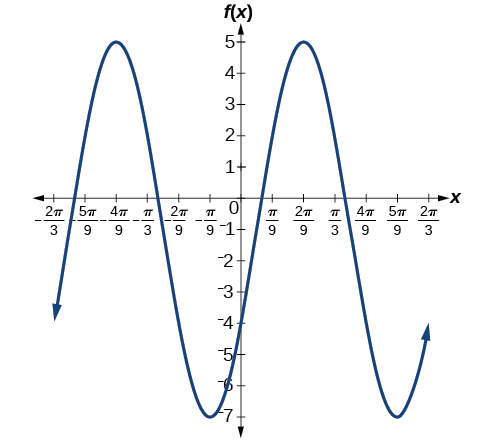
$6R6.1.7.png$

8) $f(x)=-100\sin(50x-20)$

2.2: Graphs of the Other Trigonometric Functions

For the exercises 1-4, graph the functions for two periods and determine the amplitude or stretching factor, period, midline equation, and asymptotes.

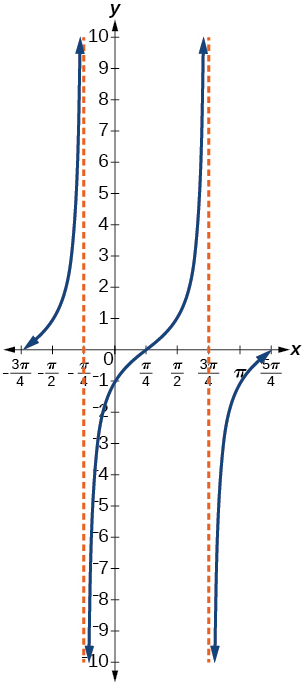
1) $f(x)=\tan x-4$

Answer

stretching factor: none; period: $\pi $midline: $y=-4$asymptotes: $x=\dfrac{\pi }{2}+\pi k$, where $k$ is an integer

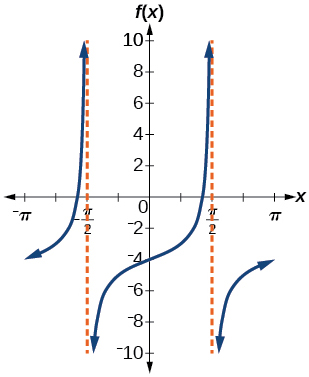
$6R6.2.1.png$

2) $f(x)=2\tan \left ( x-\dfrac{\pi }{6} \right )$

3) $f(x)=-3\tan (4x)-2$

Answer

stretching factor: $3$; period: $\dfrac{\pi }{4}$; midline: $y=-2$; asymptotes: $x=\dfrac{\pi }{8}+\dfrac{\pi }{4}k$, where $k$ is an integer

$6R6.2.3.png$

4) $f(x)=0.2\cos(0.1x)+0.3$

For the exercises 5-10, graph two full periods. Identify the period, the phase shift, the amplitude, and asymptotes.

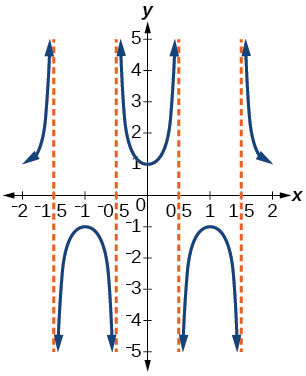
5) $f(x)=\dfrac{1}{3}\sec x$

Answer

amplitude: none; period: $2\pi $; no phase shift; asymptotes: $x=\dfrac{\pi }{2}k$, where $k$ is an integer

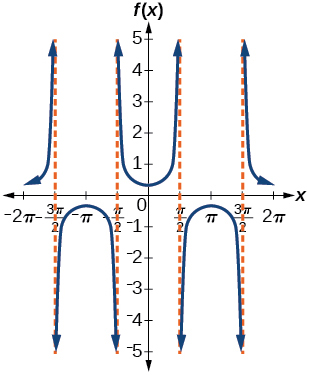
$6R6.2.5.png$

6) $f(x)=3\cot x$

7) $f(x)=4\csc (5x)$

Answer

amplitude: none; period: $\dfrac{2\pi }{5}$; no phase shift; asymptotes: $x=\dfrac{\pi }{5}k$, where $k$ is an integer

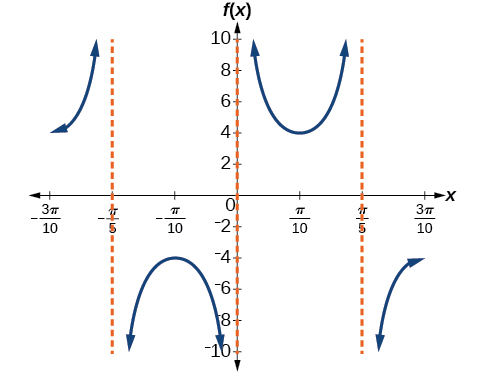
$6R6.2.7.png$

8) $f(x)=8\sec \left (\dfrac{1}{4}x \right )$

9) $f(x)=\dfrac{2}{3}\csc \left (\dfrac{1}{2}x \right )$

Answer

amplitude: none; period: $4\pi $; no phase shift; asymptotes: $x=2\pi k$, where $k$ is an integer

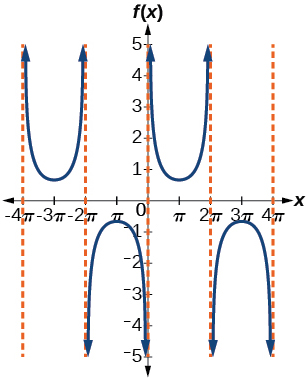
$6R6.2.9.png$

10) $f(x)=-\csc (2x+\pi)$

For the exercises 11-15, use this scenario: The population of a city has risen and fallen over a $20$-year interval. Its population may be modeled by the following function: $y=12,000+8,000\sin(0.628x)$, where the domain is the years since 1980 and the range is the population of the city.

11) What is the largest and smallest population the city may have?

Answer: largest: $20,000$; smallest: $4,000$

12) Graph the function on the domain of $[0,40]$.

13) What are the amplitude, period, and phase shift for the function?

Answer: amplitude: $8,000$; period: $10$; phase shift: $0$

14) Over this domain, when does the population reach $18,000$? $13,000$?

15) What is the predicted population in 2007? 2010?

Answer: In 2007, the predicted population is $4,413$. In 2010, the population will be $11,924$.

For the exercises 16a-16d, suppose a weight is attached to a spring and bobs up and down, exhibiting symmetry.

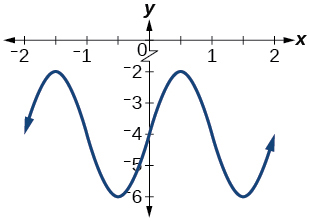
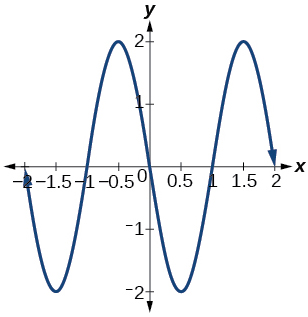
16) Suppose the graph of the displacement function is shown in the Figure below, where the values on the $x$-axis represent the time in seconds and the $y$-axis represents the displacement in inches.

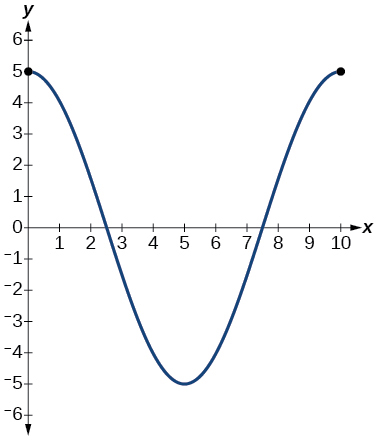
$6R6.2.16.png$

Give the equation that models the vertical displacement of the weight on the spring.
At $\text{time} = 0$, what is the displacement of the weight?

Answer: $5$ in.

At what time does the displacement from the equilibrium point equal zero?
What is the time required for the weight to return to its initial height of $5$ inches? In other words, what is the period for the displacement function?

Answer: $10$ seconds

2.3: Inverse Trigonometric Functions

For the exercises 1-11, find the exact value without the aid of a calculator.

1) $\sin ^{-1}(1)$

2) $\cos ^{-1}\left ( \dfrac{\sqrt{3}}{2} \right )$

Answer: $\dfrac{\pi }{6}$

3) $\tan ^{-1}(-1)$

4) $\cos ^{-1}\left ( \dfrac{1}{\sqrt{2}} \right )$

Answer: $\dfrac{\pi }{4}$

5) $\sin ^{-1}\left ( \dfrac{-\sqrt{3}}{2} \right )$

6) $\sin ^{-1}\left (\cos \left (\dfrac{\pi }{6} \right ) \right )$

Answer: $\dfrac{\pi }{3}$

7) $\cos ^{-1}\left (\tan \left (\dfrac{3\pi }{4} \right ) \right )$

8) $\sin \left (\sec^{-1} \left (\dfrac{3}{5} \right ) \right )$

Answer: No solution

9) $\cot \left (\sin^{-1} \left (\dfrac{3}{5} \right ) \right )$

10) $\tan \left (\cos^{-1} \left (\dfrac{5}{13} \right ) \right )$

Answer: $\dfrac{12}{5}$

11) $\sin \left (\cos^{-1} \left (\dfrac{x}{x+1} \right ) \right )$

12) Graph $f(x)=\cos x$ and $f(x)=\sec x$ on the interval $[0,2\pi )$ and explain any observations.

Answer

The graphs are not symmetrical with respect to the line $y=x$.They are symmetrical with respect to the $y$-axis.

$6R6.3.12.png$

13) Graph $f(x)=\sin x$ and $f(x)=\csc x$ and explain any observations.

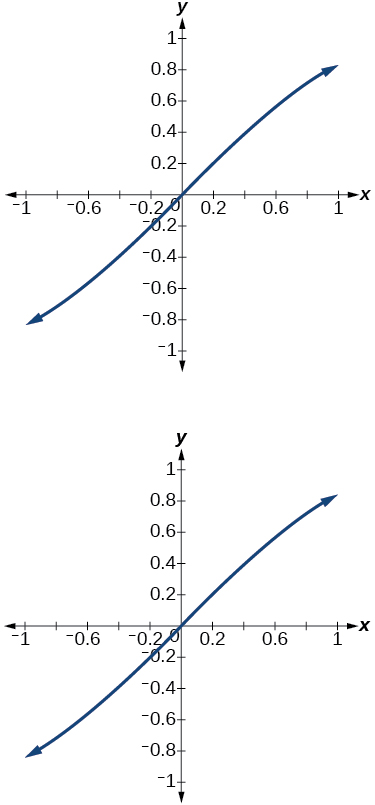
14) Graph the function $f(x)=\dfrac{x}{1}-\dfrac{x^3}{3!}+\dfrac{x^5}{5!}-\dfrac{x^7}{7!}$ on the interval $[-1,1]$ and compare the graph to the graph of $f(x)=\sin x$ on the same interval. Describe any observations.

Answer

The graphs appear to be identical.

$6R6.3.14.png$

Practice Test

For the exercises 1-13, sketch the graph of each function for two full periods. Determine the amplitude, the period, and the equation for the midline.

1) $f(x)=0.5\sin x$

Answer

amplitude: $0.5$; period: $2\pi $midline $y=0$

2) $f(x)=5\cos x$

3) $f(x)=5\sin x$

Answer

amplitude: $0.5$; period: $2\pi $; midline $y=0$

$6RP 3.png$

4) $f(x)=\sin (3x)$

5) $f(x)=-\cos \left ( x+\dfrac{\pi }{3} \right )+1$

Answer

amplitude: $1$; period: $2\pi $; midline $y=1$

$6RP 5.png$

6) $f(x)=5\sin \left (3\left ( x-\dfrac{\pi }{6} \right ) \right )+4$

7) $f(x)=3\cos \left ( \dfrac{1}{3}x-\dfrac{5\pi }{6} \right )$

Answer

amplitude: $3$; period: $6\pi $; midline $y=0$

$6RP 7.png$

8) $f(x)=\tan (4x)$

9) $f(x)=-2\tan \left ( x-\dfrac{7\pi }{6} \right )+2$

Answer

amplitude: none; period: $\pi $; midline $y=0$, asymptotes: $x=\dfrac{2\pi }{3}+\pi k$ where $k$ is an integer

$6RP 9.png$

10) $f(x)=\pi \cos(3x+\pi)$

11) $f(x)=5\csc(3x)$

Answer

amplitude: none; period: $\dfrac{2\pi }{3}$; midline $y=0$, asymptotes: $x=\dfrac{\pi }{3}k$ where $k$ is an integer

$6RP 11.png$

12) $f(x)=\pi \sec \left ( \dfrac{\pi }{2}x \right )$

13) $f(x)=2\csc \left ( x+\dfrac{\pi }{4} \right )-3$

Answer

amplitude: none; period: $2\pi $; midline $y=-3$

$6RP 13.png$

For the exercises 14-16, determine the amplitude, period, and midline of the graph, and then find a formula for the function.

14) Give in terms of a sine function.

$6RP 14.png$

15) Give in terms of a sine function.

$6RP 15.png$

Answer: amplitude: $2$; period: $2$; midline: $y=0$; $f(x)=2\sin(\pi (x-1))$

16) Give in terms of a tangent function.

$6RP 16.png$

For the exercises 17-20, find the amplitude, period, phase shift, and midline.

17) $y=\sin\left(\dfrac{\pi}{6}x+\pi \right)-3$

Answer: amplitude: $1$; period: $12$; phase shift: $-6$; midline: $y=-3$

18) $y=8\sin\left(\dfrac{7\pi}{6}x+\dfrac{7\pi}{2} \right)+6$

19) The outside temperature over the course of a day can be modeled as a sinusoidal function. Suppose you know the temperature is $68^{\circ}$F at midnight and the high and low temperatures during the day are $80^{\circ}$F and $56^{\circ}$F, respectively. Assuming $t$ is the number of hours since midnight, find a function for the temperature, $D$, in terms of $t$.

Answer: $D(t)=68-12\sin\left(\dfrac{\pi}{12}x \right)$

20) Water is pumped into a storage bin and empties according to a periodic rate. The depth of the water is $3$ feet at its lowest at 2:00 a.m. and $71$ feet at its highest, which occurs every $5$ hours. Write a cosine function that models the depth of the water as a function of time, and then graph the function for one period.

For the exercises 21-25, find the period and horizontal shift of each function.

21) $g(x)=3\tan(6x+42)$

Answer: period: $\dfrac{\pi}{6}$; horizontal shift: $-7$

22) $n(x)=4\csc \left(\dfrac{5\pi }{3}x-\dfrac{20\pi }{3} \right)$

23) Write the equation for the graph in the Figure below in terms of the secant function and give the period and phase shift.

$6RP 23.png$

Answer: $f(x)=\sec(\pi x)$; period: $2$; phase shift: $0$

24) If $\tan x=3$find $\tan (-x)$.

25) If $\sec x=4$, find $\sec (-x)$.

Answer: $4$

For the exercises 26-28, graph the functions on the specified window and answer the questions.

26) Graph $m(x)=\sin(2x)+\cos(3x)$ on the viewing window $[-10,10]$ by $[-3,3]$Approximate the graph’s period.

27) Graph $n(x)=0.02\sin(50\pi x)$ on the following domains in $x:[0,1]$ and$[0,3]$Suppose this function models sound waves. Why would these views look so different?

Answer

The views are different because the period of the wave is $125$Over a bigger domain, there will be more cycles of the graph.

$6RP 27.png$

28) Graph $f(x)=\dfrac{\sin x}{x}$ on $[-0.5,0.5]$ and explain any observations.

For the exercises 29-31, let $f(x)=\dfrac{3}{5}\cos(6x)$.

29) What is the largest possible value for $f(x)$?

Answer: $\dfrac{3}{5}$

30) What is the smallest possible value for $f(x)$?

31) Where is the function increasing on the interval $[0,2\pi ]$?

Answer: On the approximate intervals $(0.5,1),(1.6,2.1),(2.6,3.1),(3.7,4.2),(4.7,5.2),(5.6,6.28)$

For the exercises 32-33, find and graph one period of the periodic function with the given amplitude, period, and phase shift.

32) Sine curve with amplitude $3$, period $\dfrac{\pi }{3}$and phase shift $(h,k)=\left(\dfrac{\pi }{4},2\right)$

33) Cosine curve with amplitude $2$, period $\dfrac{\pi }{6}$and phase shift $(h,k)=\left(-\dfrac{\pi }{4},3\right)$

Answer

$f(x)=2\cos\left ( 12\left ( x+\dfrac{\pi }{4} \right ) \right )+3$

$6RP 33.png$

For the exercises 34-35, graph the function. Describe the graph and, wherever applicable, any periodic behavior, amplitude, asymptotes, or undefined points.

34) $f(x)=5\cos(3x)+4\sin(2x)$

35) $f(x)=e^{(sint)}$

Answer

This graph is periodic with a period of $2\pi $

$6RP 35.png$

For the exercises 36-43, find the exact value.

36) $\sin^{-1}\left ( \dfrac{\sqrt{3}}{2} \right )$

37) $\tan^{-1}\left ( \sqrt{3} \right )$

Answer: $\dfrac{\pi }{3}$

38) $\cos^{-1}\left ( -\dfrac{\sqrt{3}}{2} \right )$

39) $\cos^{-1}\left ( \sin(\pi) \right )$

Answer: $\dfrac{\pi }{2}$

40) $\cos^{-1}\left ( \tan \left (\dfrac{7\pi}{4} \right ) \right )$

41) $\cos(\sin^{-1}(1-2x))$

Answer: $\sqrt{1-(1-2x)^2}$

42) $\cos^{-1}(-0.4)$

43) $\cos \left (\tan^{-1}\left(x^2\right) \right )$

Answer: $\dfrac{1}{\sqrt{1+x^4}}$

For the exercises 44-46, suppose $\sin t=\dfrac{x}{x+1}$ Evaluate the following expressions.

44) $\tan t$

45) $csc t$

Answer: $\dfrac{x+1}{x}$

46) Given Figure, find the measure of angle $\theta $ to three decimal places. Answer in radians.

$6RP 46.png$

For the exercises 47-49, determine whether the equation is true or false.

47) $\arcsin\left(\sin\left(\dfrac{5\pi }{6}\right)\right)=\dfrac{5\pi }{6}$

Answer: False

48) $\arccos\left(\cos\left(\dfrac{5\pi }{6}\right)\right)=\dfrac{5\pi }{6}$

49) The grade of a road is $7\%$. This means that for every horizontal distance of $100$ feet on the road, the vertical rise is $7$ feet. Find the angle the road makes with the horizontal in radians.

Answer: approximately $0.07$ radians

Search

Text Color

Text Size

Margin Size

Font Type

2.2: Graphs of the Other Trigonometric Functions

2.3: Inverse Trigonometric Functions

Practice Test

Contributors and Attributions